AP Stats - Unit 3

This is mostly vocab and things to remember in wording. Practice problems are not here.

Correlation Coefficient

This is “r”

• A quantitative assessment of the strength & direction of the linear relationship between bivariate, quantitative data

• Pearson’s sample correlation is used most

• parameter - ρ (rho)

• statistic - r

Does the value of r depend on which of the two variables is labeled x?

The value of r does not depend on which of the two variables is labeled x

Weak Correlation range

-.5 to .5

No Correlation range

No range. 0 is no correlation

Strong Correlation Range

-1 to -.8 and .8 to 1

Moderate Correlation Range

-.8 to -.5 and .5 to .8

Does the value of r depend on which of the two variables is labeled x?

The value of r does not depend on which of the two variables is labeled x

Is value of r resistant or non-resistant?

The value of r is non-resistant

Outliers affect the correlation coefficient

What is the value of r a measure of?

The value of r is a measure of the extent to which x & y are linearly related

A value of r close to zero does not rule out any strong relationship between x and y.

True or False?

True

Does correlation imply causation?

Correlation does not imply causation

x – variable

• the independent or explanatory variable

y- variable

• the dependent or response variable

What does LSRL stand for?

Least Squares Regression Line

(LSRL)

What is the LSRL formula?

y (with a hat, as pictured)= a + bx

y (y-hat) - means the predicted y

b  – is the slope

• it is the amount by which y increases when x increases by 1 unit

a  – is the y-intercept

• it is the height of the line when x = 0

• in some situations, the y-intercept has no meaning

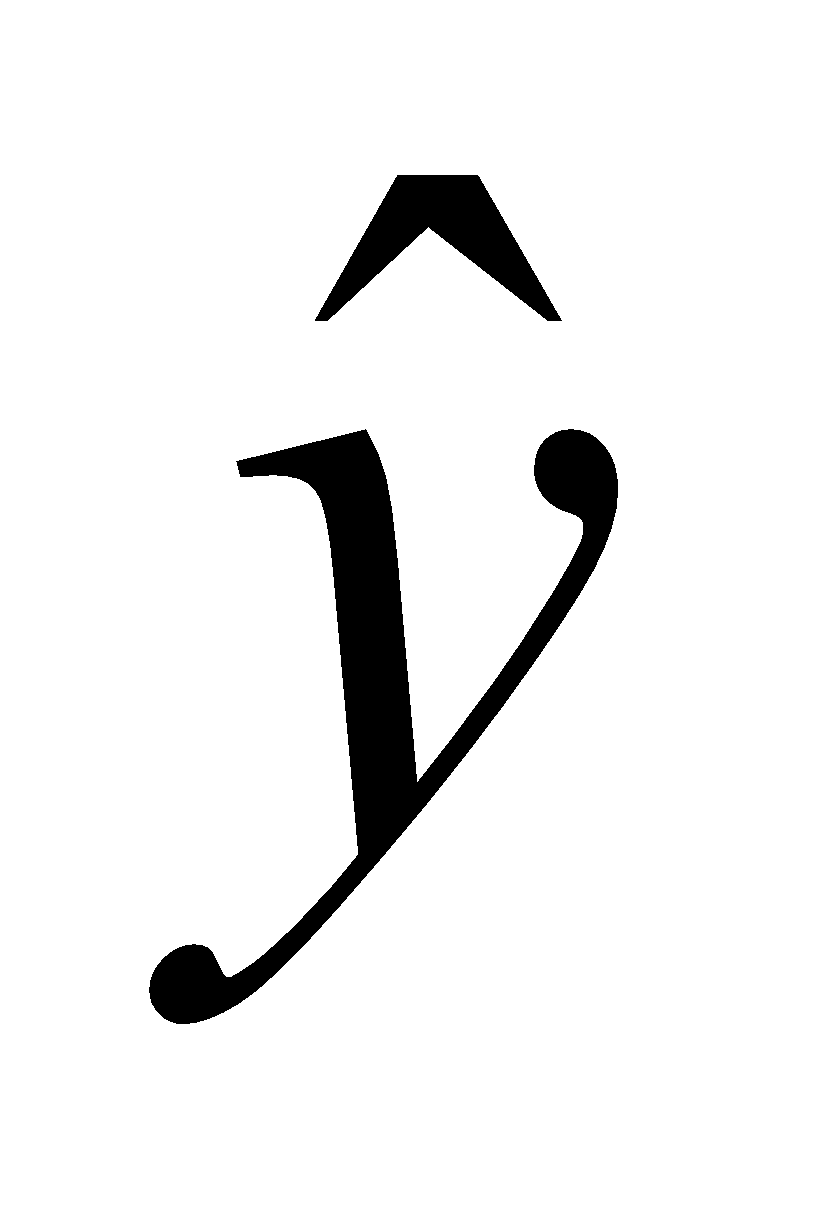
What exactly is the LSRL?

• The line that gives the best fit to the data set

The line that minimizes the sum of the squares of the deviations from the line

How do you interpret the slope?

For each unit increase in x, there is an approximate increase/decrease of b in y.

(Plug in the boldened words/letters).

How do you interpert the correlation coefficent?

There is a direction, strength, linear of association between x and y.

How does LSRL work with Extrapolation

• The LSRL should not be used to predict y for values of x outside the data set. 

It is unknown whether the pattern observed in the scatterplot continues outside this range.

The correlation coefficient and the LSRL are both non-resistant measures.  

True or false?

True.

Formulas (null)

y(-hat) = b₀ + b₁x

b₀ = y-hat - b₁x

b₁ = r(S_y/S_x)

Residual formula

Residual = y - y(-hat)

What are residuals?

Error

• The vertical deviation between the observations & the LSRL

• the sum of the residuals is always zero

• error = observed - expected

What are Residual plots?

• A scatterplot of the (x, residual) pairs.

• Residuals can be graphed against other statistics besides x

• Purpose is to tell if a linear association exist between the x & y variables

If no pattern exists between the points in the residual plot, then the association is linear.

If no pattern exists between the points in the residual plot, then the association is _____

If no pattern exists between the points in the residual plot, then the association is linear.

If a pattern exists between the points in the residual plot, then the association is ___.

non-linear

Are residual plots are the same no matter if plotted against x or y-hat?

Yes

Residual plots are the same no matter if plotted against x or y-hat.

Coefficient of determination

• r²

• gives the proportion of variation in y that can be attributed to an approximate linear relationship between x & y

remains the same no matter which variable is labeled x

Interperation of r²

Approximately r²% of the variation in y can be explained by the LSRL of x & y.

Outlier

In a regression setting, an outlier is a data point with a large residual

Influential point-

• A point that influences where the LSRL is located

If removed, it will significantly change the slope of the LSRL

Which of these measures are resistant?

• LSRL

• Correlation coefficient

• Coefficient of determination

NONE are resistant – all are affected by outliers

Computer-generated regression analysis of knee surgery data: 

What is the equation of the LSRL?

Find the slope & y-intercept.

Predictor Coef Stdev T P

Constant 107.58 11.12 9.67 0.000

Age 0.8710 0.4146 2.10 0.062

s = 10.42 R-sq = 30.6% R-sq(adj) = 23.7%

y(-hat) = 107.58 + .8710x

Computer-generated regression analysis of knee surgery data: 

What are the correlation coefficient and the coefficient of determination

Predictor Coef Stdev T P

Constant 107.58 11.12 9.67 0.000

Age 0.8710 0.4146 2.10 0.062

s = 10.42 R-sq = 30.6% R-sq(adj) = 23.7%

Never use adjusted R²

Be sure to convert r² to decimal before taking the square root!

r=.5532