populations final

population dynamics is…

population dynamics is…

• population is an important unit of organization

• basic unit w management and conservation

population definitions

1. a group of individuals of one species that live in a particular geographic area

2. a collection of inter-breeding organisms of a particular species

tell four basic population dynamic processes

why defining the unit is important

• bc it will affect the unit / manner of management

• bc it will affect the status of the population

evolutionary significant unit (ESU)

• from salmon managment

• commonly used for the purpose of conservation of “protected species”

Distinct population segment (DPS)

• language in Endangered Species Act

• smallest unit of species to be protected under ESA

Stock

• unit of management in fisheries

• the Magnuson-Stevens Fishery Conservation and Management Act

State Variables

variables that represent the state of a population

population dynamics (definition)

changes in the state of a population

what are the state variables

measures of the state of the population

• population abundance

• population density (number of individuals per unit area)

• biomass

• incidence (presence-absence)

Vital rates

facts that change population abundance

what are the vital rates

• birth

• death

• immigration

• emigration

Constant per-capita birth rate

• number of offspring born and survive between time t and t+1 per individual parent

• assume no immigration and no emigration

  • b (in equation)

Constant per=capita death rate

• a portion of individuals alive at time t dying before t+1

• rate of death of individuals from time t to time t+1

• d (in equation)

geometric growth equation

• n(t+1) = lambdan(1)

per-population meaning

2.2

natural log of density changes w geometric growth

• grows (declines) linearly ( straight line )

constant number or birth rates + constant number of death rates =

• geometric growth/decline

finite per-capita population growth rate

• lambda

• lambda > 1 = geometric growth

• lambda = 1 pop. density remains the same

• 1>lambda>(-) = geometric decline

fertility term

• fecundity (# of offspring per adult) x Offspring survival until age 1

lifecycle graph

• circle/node = age class

• arrows = potential transitions of individuals from t to t+1

• solid: aging/death

• dashed: reproduction / fecundity per parent c the survival of the offspring over one time unit

how to read/label a(ij)

• contribution of age class J to age class I

• OG age class is second script

how to deal with females and males in a model

how to write a matrix w a(ij)

• first subscript = row on matrix

• second subscript = column in matrix

vector

• matrix with one column

matrix

• 3 x 3 matrix

scalar

• a matrix with one row and one column (n)

ordinary product

• multiplication of matrix and vector product

component of a vector

• each parameter/variable in a vector

element of a matrix

• each parameter/variable in a matrix (vital rates)

• location is indicated by 2 numbers (i,j)

how to multiply matrices

• row 1 and column 1 x row 1 and column 1

• row 1 and column 2 x row 2 and column 1

• etc

• only matrices of same size can be multiplied

• number of columns of a matrix and the number of components of a vector must be the same

how to refer to an elemet of matrix (indices)

how to multiply a popultion matrix and population vector

n(t+1) = An(t) (?)

how to enter transition rates into a population matrix

• a(ij)

• i = the row

• j = the column

population vector

• the ith component of the vector is the number/density of individuals in the ith stage

• the number of components is the same as the number of age classes in the model

when does the natural log of population density grows/declines linearly with time?

• when the elements of a population matrix are constant over time = expo. growth

what happens to a population when per-capita rates are constant ?

geometric / exponential growth

what is the difference between transient and asymptotic dynamics when vital rates are constant?

• asymptotic = population grows/declines exponentially (here all stages have the same slope w same rate)

• transient = each stage grows/declines exponentially after some transient period.

difference between state variables and vital rates ?

what’s asymptotic per-capita population growth rate ?

• each stage density grows geometrically and the coefficient is the same for all stages

describe stable stage (age) distribution

• under asymptotic dynamics, distribution of individuals amongst stage remains constant

differences in the characteristics of lifecycle graphs associated with lesilie matrix (age structured) and lefkovitch mitch (stage structured)

• leslie matrix:

  • age progress w time

• lefkovitch mitch:

  • assume age is a special type of stage

  • stage does not necessarily progress w time

    • can remain in same stage for years (self-loops)

• both have asymptotic population growth rate and stable stage distribution (properties are the same)

how to draw lifecycle graphs for stage-structured populations

• time unit is the same for all

• they are stages that can hold multiple ages

how to write population matrix for stage-structured population

just know

3 things you can calculate from constant population projection matrix

1. asymptotic population growth rate (lambda)

2. stable stage distribution (w)

3. reproductive value (v)

stable stage distribution

• where the proportion of individuals in each stage remains constant

reproductive value

• measures relative expected contribution of individuals in different stages to future population abundance

• denoted by vector v

difference between reproductive value and fertility

• fertility = contributions of individuals in the present

• reproductive value = measure of future potential asymptotic contribution of individuals

what is sensitivity ?

• how sensitive lambda is to changes in population parameters aka transition rates

• shows measures of how important vital rates are to the asymptotic population growth rate

be able to calculate sensitivity from stable stage distribution and reproductive values

• W = stable stage distribution // V = reproductive value

• vw (T) / v (T)w

interpret the sensitivity results

• use (i,j) to see how sensitive lamda is to a(ij)

• the ith row of the sensitivity matrix is high if the “reproductive value” of a stage i is high

• the jth column of the sensitivity matrix is high if the stable stage distribution of stage j is high

loggerhead sea turtles basic info:

• 5 stages:

  • eggs

  • small juveniles

  • large juveniles

  • subadults

  • adults

• more younger individuals in the population

• reproductive value of older individuals is high

• we should allocate more effort to protect juveniles

how is sensitivity matrix related to reproductive value and stable stage distribution

• the product of reproductive value and stable stage distribution is the sensitivity matrix

high sensitivity

• if the sensitivity of lamda is to a(ij) is high

  • relatively large number of individuals is in stage j and or

    • reproductive value of stage i is high

instantaneous population growth rate:

• the natural log of the finite population growth rate (lambda)

• instantaneous and finite population growth rates are both per-capita population growth rates

what affects vital rates (transition rates) ?

• lack of food

• habitat deterioration

• human interventions (e.g conservation activity)

describe the steps in conducting matrix population model analysis?

v =

reproductive value

w =

• stable stage distribution

simpsons paradox

• a trend appears in several groups of data but disappears or reverses when the groups are combined

difference between longitudinal data and census data

• longitudinal data: the individual capture-recaptured data are a type data collected

  • identified individuals are followed over time

• census data: just count individuals

meaning of 95% confidence intervals

• if we repeat the analysis, the estimated value is expected to fall within the confidence intervals 95% of the time

the cause of increased morality of north atlantic right whales

• individuals are dying with a higher stage specific per-capita mortality rate

• changes in vital rates

  • collision with ships

the 4 qualities calculated from the population matrix

• growth rate

• reproductive values

• stable stage

• sensitivity matrix

why a population growing in its abundance can have a reduced survival rate?

• because stage distribution may be different

• because the population may consist of more individuals in a stage with lower survival rate

elasticity

• how lambda changes from proportional changes in transition rates a(ij)

what is the shape of a graph representing population abundance if the same proportion 0.3 of individuals ( 30% ) dies and each individual in the population contributes to the birth of 0.2 individuals on average and these per-capita rates remain constant ( the same ) ?

exponentially decreasing density

shape of the graph representing population density of both per capita birth rate and per. capita death rates are constant over time?

exponentially increasing density

which one of these statements are not true ?

there is no variable in statistical analysis

the proportional of individuals died over one year is 0.8. this value represents

per capita death/mortality rate

geometric/exponential growth results from

constant per-capita survival and per-capita birth rate

why do we need to structure a population based on age?

bc survival rate may be different among age classes and bc reproductive rate may be different among age classes

a(21)

• the first number = the destination / the row

• the second number = the OG / the column

the first step to build a population matrix

to draw a lifecycle graph

the size of leslie matrix for a population is determined by

the oldest individuals in the population

the number of rows of leslie matrix and the number of component of an associated population vector must be the same

true

which is the correct way of multiplying a population matrix and population vector

nt+1 = Ant

( lambda ) weird Y

represents population growth rate

if all of the per capita rates ( birth rate, death rate, developmental rate ) are constant over time, population density should change

exponentially with time

the asymptomatic population growth rate and stable stage distribution are the properties of age-structured populations but they are not the properties of stage - structured population?

false

sensitivity in the matrix population model analysis measures how sensitive lambda is to the same magnitude changes in vital rates whereas the elasticity measures how sensitive lambda is to the same proportional changes in vital rates

true

in each experiment, we start with the same number of individuals in each stage and introduce two additional individuals either adults or juveniles. on average which will have the greater number of individuals in the future?

both populations

what does lambda = .95 imply?

the population is expected to decrease by 5% per year on average

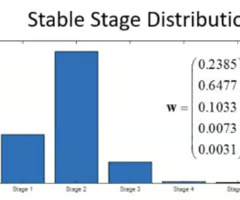
why does stage 2 have a greater number of individuals than stage 1 ?

bc stage 2 includes multiple age classes

why does stage 5 loggerhead sea turtles have high relative reproductive value?

• bc stage 5 have high survival rate

• bc stages 1-3 have low survival rate

• bc stage 5 has high fecundity

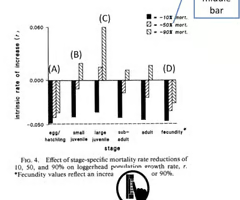
according to the figure, which of the following is expected to make the population to start growing?

50% reduction in mortality of large juveniles

what does reproductive value measure?

relative contribution of individuals in a given stage to future population abundance

according to the sensitivity matrix, which parameter has the most impact on the asymptotic population growth rate?

a (43)

you administer medication to individuals and keep track of their symptoms/fate over time ( a record for each individual is kept ) the data are

longitudinal data

the average survival rate for population can be decline when a population abundance is increasing (assuming no immigration and emigration)

TRUE

case mortality rate appears to be declining. it is still plausible that that disease is becoming worse ( according to simspons paradox ) bc

more younger people ( who previously did not develop symptoms before ) are developing symptoms and they tend to recover.

population is

a group of individuals of one species that live in a particular geographic area

semelparous means

individuals reproduce at most once in their life time

suppose a population of frogs consisted of 100 individuals on March 1, 2021 and 94 individuals on April 2021. We know there was no birth. we also know there is no immigration and emigration because the population is isolated. Which of the following is the best answer?

The monthly mortality rate was 0.06

suppose X=500-Ns where Ns is the variable in the script. what is X Suppose you survey deer in national park ( all of the individuals in population is in the park ) and count how many individuals died in a given year. the total number of death is

not sufficient information to calculate a per-capita annual death rate

which of the following is correct

individuals can start reproducing at age 4, and some individuals can live beyond age 5

fertility rate for matrix population model is

the product of fecundity per parent and the survival rate of offspring

lifecycle graph tells

number of stage/age classes and how individuals can be transition among them

why does population density fluctuate over time ?

is it because vital rates are not constant

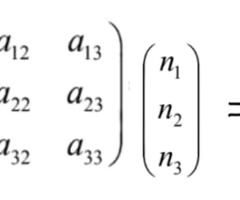
which of the following is correct

x(1) = a11n1 + a12n2+a13n3