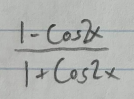Trig Identities

(Basic Trig Identity) sin²x + cos²x =

1

(Basic Trig Identity) 1 + tan²x =

sec²x

(Basic Trig Identity) 1 + cot²x =

csc²x

(Quotient Identity) tanx

sinx / cosx

(Quotient Identity) cotx

cosx / sinx

(Reciprocal Identity) cscx

1 / sinx

(Reciprocal Identity) secx

1 / cosx

(Reciprocal Identity) cotx

1 / tanx

Basic Trig Identities

The Pythagorean Identities. It comes from the unit circle, where the hypotenuse is 1. Since sine is the y-value and cosine is the x-value, using the Pythagorean Theorem gives this equation.

Reciprocal Identity

Shows how each trig function is the reciprocal of another. Basically how one side of trig functions relate to the other side.

Quotient Identity

Focused on the third trig functions of both sides, this describes how the other two functions of the pair relate to them.

(Cofunction Identity) cos(pi/2 - x)

Sinx

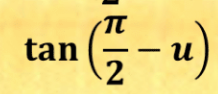
(Cofunction Identity) tan(pi/2 - x)

Cotx

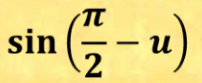
(Cofunction Identity) sin(pi/2 - x)

Cosx

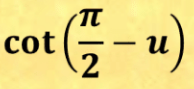
(Cofunction Identity) cot(pi/2 - x)

Tanx

Cofunction Identity

states that trigonometric functions of complementary angles are equal, allowing for transformations between functions like sine, cosine, tangent, and cotangent. Only happens when it’s multiplied by (pi/2 - x)

(Half Angle Formula) Sin²x

1 - Cos2x / 2

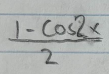
(Half Angle Formula) Cos²x

1 + Cos2x / 2

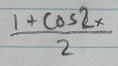
(Half Angle Formula) Tan²x

1 - Cos2x / 1 + Cos2x