Photogrammetry Exam 1

Wavelenght

distance between 2 peaks or 2 troughs

inversely proportional to frequency

inversely proportional to energy

lambda

units are micrometers

frequnecy

inversely proportional to wavelength

proportional to energy

atmospheric walls

opaque to solar or other electromagnetic radiation

white spaces between atmospheric transmissions

obstructs transmission

wavelength ranges

ultraviolet

blue

green

red

near infrared

photographic infrared

mid-infrared

thermal infrared

microwave

visible light

0.4 μm to 0.7 μm

blue

green

red

ultraviolet

less than 0.4 μm

blue

0.4 - 0.5 μm

band 1

green

0.5 - 0.6 μm

band 2

red

0.6 - 0.7 μm

band 3

near infrared

0.7 - 1.3 μm

band 4

photographic infrared

0.7 - 0.9 μm

mid infrared

1.3 - 3 μm

thermal infrared

greater than 3 μm

microwave

1 mm - 1 m

emitted

energy coming from inherent heat or energy absorbed

energy coming off something

thermal

metabolic processes

absorbed radiation

reflected

light that bounces off something and then comes back

speed of light equation

c=νλ

speed of light

constant

slower in water or air than in a vacuum

3×10^8 m/s in a vacuum

electromagnetic radiation

electromagnetic energy composed of discrete units called protons or quanta

energy of quanta

Q=hc/λ

joules pf energy = (Planck’s constant x speed of light)/ wavelength

quantum energy

inversely proportional to wavelength

E=hc/λ

flux

how many particles an area is getting

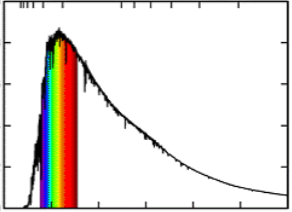
photon flux (Φ)

number of photons per second per unit area

doesn’t incorporate energy or wavelength

number of particles/ m² x s

power density (H)

incorporates energy and wavelength

photon flux times energy

Φ x quantum energy (hc/λ)

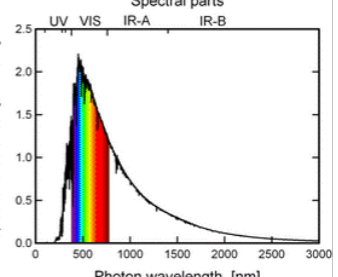
spectral irradiance

power density / Δλ

energy from the sun

there is a rapid decrease in spectral irradiance as the wavelength increases

there is a more gradual decrease in photon flux as the wavelength increases

yellow has the most spectral irradiance and highest proton flux

waves and particles

distinct but related

energy per quantum decreases as wavelength increases

when wave-packets are spatially localized, they are considered particle like

energy per unit basis

based on how many photons and the amount of energy per photon

sunlight

electromagnetic radiation

visible light is a small subset of the electromagnetic spectrum

light

wave with particular wavelength

packets of particles of energy, photons

both wave-like and particle-like

either absorbed, reflected, or transmitted

quanta

total energy of light is made up of indistinguishable energy elements

blue light

has higher energy but shorter wavelength

red light

has lower energy but longer wavelength

the photon flux of a higher energy photon to give a specific power density

will be lower than the photon flux of a lower energy photon required to give the same power density

spectral irradiance (F)

gives the power density at a particular wavelength

F = Φ x E x (1/Δλ)

short wavelength

high energy

high frequency

long wavelength

low energy

low frequency

photon flux units

photons per square meter per second

quantum energy units

joules per photon

spectral irradiance units

watts per square meter per micrometer

power density units

watts per square meter

spectral irradiance graph

photon flux graph

2 parts of optical wavelengths

-wavelength band

-spectral band

defined regions

1 micrometer

1000 nanometers

atmospheric windows

atmosphere is largely transparent

put sensors here

spectral bands through time

are very similar

landsat data continuity act

short wave infrared 1

band 5

short wave infrared 2

better to separate vegetation from soil

standard false color composite

NIR → Red

Red → Green

Green → Blue

432

true color composite

actual colors we would see

pixel

picture element

ground resolution cell

spatial resolution

horizontal dimension

side of a ground resolution cell

identify feature

shows you the 6 wavelength bands the satellite records

spectral signiture

relates to color/hue

reflections as a function of wavelength

material type and condition

Incident energy

=reflected energy + absorbed energy +transmitted energy

variation of wavelenght

gives us color

how we see in response to reflected light

1

= reflectance + absorptance + transmittance

unitless

scattering

when you hit something and it moves off in all directions

unpredictable diffusion of radiation by particles in the atmosphere

how we see light in our shadow

every bit of light we see has some of this, have to correct for remote sensing

doesn’t change wavelength

absorption

sucks energy in

effective loss of energy

taking in of energy at particular wavelengths by atmospheric constitutes

causes object to heat up

Rayleigh Scattering

Particle diameter is larger than wavelength

why sky is blue and sunsets are red

cause of haze in imagery

caused by aerosols (small molecules)

wavelength specific

shorter wavelength have more scattering

Nonselective Scattering

Particle diameter is smaller than wavelength

all wavelengths are effected equally (not wavelength specific)

water droplets cause this

why clouds are white

Mie Scattering

particle diameter equals wavelength

dominates in slightly overcast or hazy conditions

water vapor and dust cause this

diffuse reflectance

scatters in the atmosphere and interferes with reading

path radiance

Top atmospheric gases that are absorbers

1. Water (H2O)

2. Carbon Dioxide (CO2)

3. Methane (CH4)

Carbon Dioxide units in atmosphere

Parts per million

Methane units in atmopshere

parts per billion

H2O units in atmosphere

Percent

Atmospheric water vapor feedback

Positive: clouds

Negative: humidity

When something gets hotter

more energy is emitted

the energy emitted out is a shorter wavelength

Ozone

short wavelengths are absorbed

long wavelengths are released out

keeps UV light out of atmosphere

chlorophyll

absorbs red and blue light

leaves green

sources of emitted energy

human endothermic system

solar fusion

thermal energy

blackbody

no light is coming out

unreflective and absorbs across the spectrum

as it heats up it emits more energy

emitted energy falls along Planck’s curve

emissivity = 1

hot fires

have a wavelength peak of 3 micrometers

can see with sensors

thermal scanners see 3000 - 15000 nm

spectral resolution

how finely we divide the electromagnetic spectrum

color picture has 3 bands

where you are in respect to where sun emits, smaller wavelengths more ideal

as you get longer wavelengths, you get less solar radiation

more bands: less energy

Panchromatic band

giant band 8

the more you divide up spectral resolution

higher resolution

you lose energy

spatial resolution becomes more coarse

finer spatial resolution

decreased energy

how to get more photons to increase signal/noise ratio

use an active sensor

coarsen spectral resolution

active sensor

sends energy out to illuminate objects

flash photography

passive sensor

relies on just light emitted or reflected from the environment

graybody

not completely black but still has a Planck Curve

emissivity less than one

same peak and shape of blackbody

doesn’t vary by wavelength

selective radiator

if emissivity varies with wavelength

geometric manner of reflectance

if something is smooth, you will see hotspots

specular reflector

most smooth

one angle of incidence and one angle of reflectance

mirror

Lambertian surface

diffuse reflector

most rough

one angle of incidence and many equal angles of reflection

rough surfaces

are better for remote sensing

with respect to wavelength of energy

leaves

have lots of infrared reflecting off it

most visible wavelengths are absorbed in leaves

overall reflectance is low

higher green reflectance than red or blue

needles less reflecting (NIR)

variability in spectral signals

atmospheric effects

phenology or other temporal changes (snow…)

differential illumination (shadows)

feature conditions (drought…)

mixed pixels/ spectral mixture

mixtures of soil, veg, or water in a pixel

can lighten or darken spectral signature

dirt added to a pond would increase the pond’s NIR reflectance

radiometric resolution

ability of remote senses system to record many levels of a value

more bins = more resolution

100% vs A-F vs Pass Fail

Landsat 1-3

6 bit

Landsat 4-7

8 bit

Landsat 8-9

12 bit

Base 10

(_ x 10^0) + (_ x 10^1) + (_ x 10²) …

Base 2

(_ x 2^0) + (_ x 2^1) + (_ x 2²) …

feature space

n-dimensional space with all possible combinations of DN’s/e’s ???

Classification schemes

informational classes

use an existing one

principles of classification schemes

hierarchical

exhaustive

mutually exclusive