Physics A. Space, time and motion

That the motion of bodies through space and time can be described and analysed in terms of position, velocity, and acceleration

• position: an object's distance and direction from a reference point, the coordinate on a number line

Velocity is the rate of change of position, and acceleration is the rate of change of velocity

• velocity: the rate of change of displacement. velocity = change of displacement / time taken (v/u). Vector quantity

• acceleration: the rate of change of velocity. acceleration = change of velocity / time taken (a). Vector quantity

The change in position is the displacement

displacement is the change in position, vector quantity. The displacement gives information on the direction of the change in position relative to the starting position.

displacement = final position - intial position

The difference between distance and displacement

• Displacement: Vector quantity. The displacement gives information on the direction of the change in position relative to the starting position.

• Distance: Scalat quantity. Distance shows the length of the path followed

The difference between instantaneous and average values of velocity, speed and acceleration, and how to determine them

• Velocity

  • Average Velocity = total displacement / total time

  • Instantaneous velocity is the velocity (speed with direction) at a particular instant.

• Speed

  • Average speed = total distance / total time

  • Instantaneous speed is how fast an object is moving at a particular point in time

• Acceleration

  • Average acceleration = change in velocity / total time

  • Instantaneous acceleration is the rate of change of velocity at a single point in time.

To find instantaneous values, you look at a graph and find the value at a certain point (using the gradient or reading it directly from the graph)

Displacement-time graphs

The y-axis represents displacement, while the x-axis represents time.

The slope of the graph indicates the object's velocity.

A flat line represents zero velocity, a positive slope indicates constant forward motion, and a negative slope indicates constant backward motion.

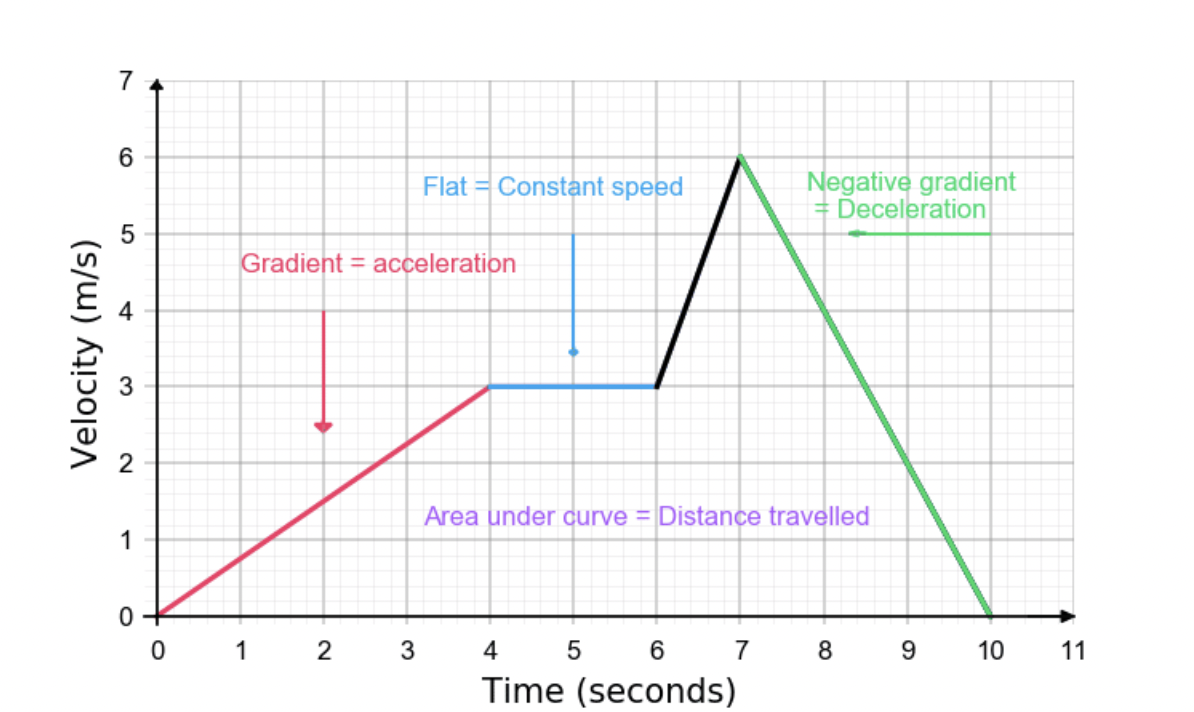
Velocity-time graphs

Graph that shows how an object's velocity changes over time.

Steeper slope = acceleration.

Flat line = constant speed.

Negative slope = moving backwards.

Area under the curve represents displacement.

acceleration-time graphs

The slope of the graph indicates the object's acceleration.

A steeper slope represents a higher acceleration, while a flatter slope indicates a lower acceleration.

The area under the graph represents the object's change in velocity.

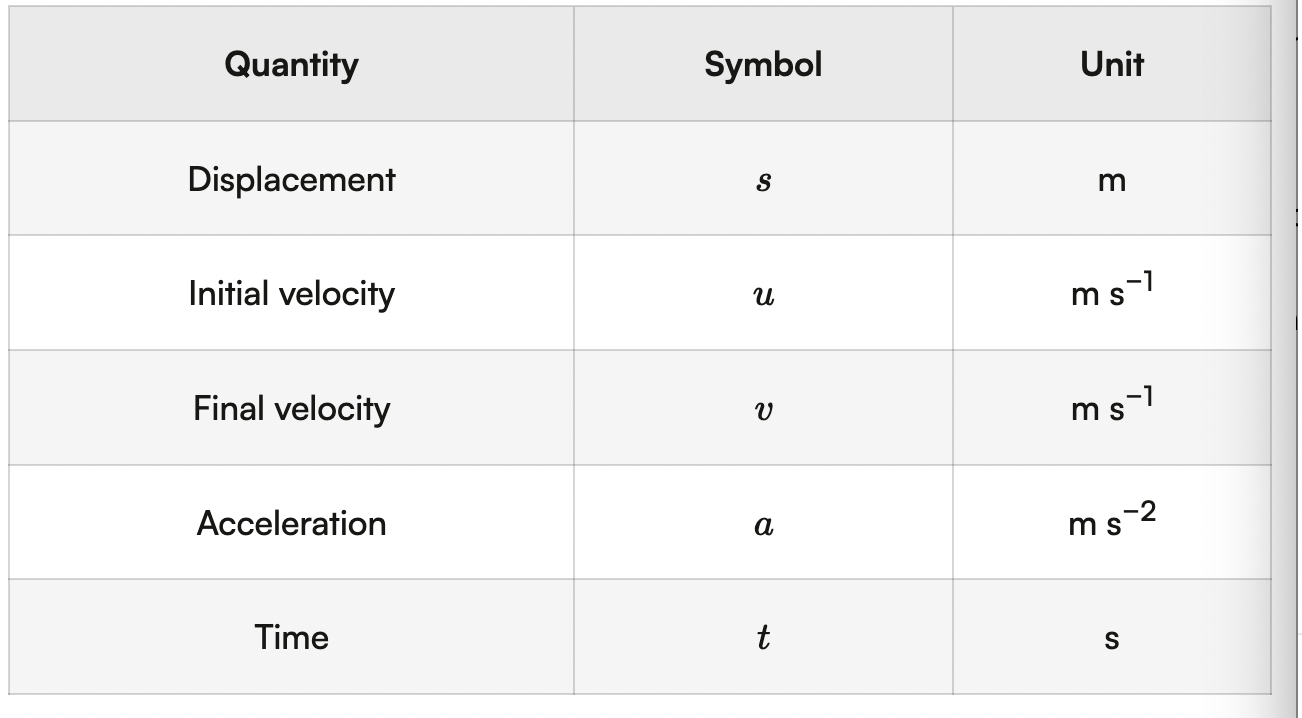
The equations of motion for solving problems with uniformly accelerated motion as given by s = (u + v)/2 *t, v = u + at, s = ut + 1/2 at^2 and v^2 = u^2 + 2as

u - initial velocity

v - final velocity

a - acceleration (constant)

t - time taken

s- distance travelled

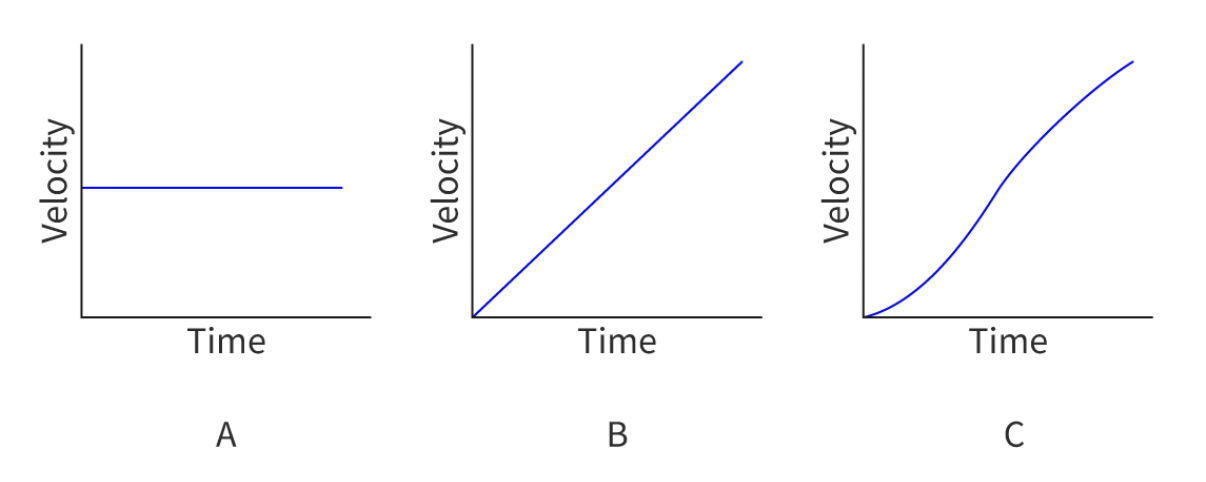
Motion with uniform and non-uniform acceleration

a. uniform motion is motion with constant velocity

b. uniform acceleration = Velocity changes at a constant rate.

c. non-uniform acceleration = Velocity changes at a rate that is not constant.

The behaviour of projectiles in the absence of fluid resistance, and the application of the equations of motion resolved into vertical and horizontal components

When a body is allowed to fall freely ignoring the effects of air resistance, it is called free fall. Bodies falling freely on the Earth fall with an acceleration of about 9.81 ms^-2 (g)

A projectile is a particle moving freely (non-powered), under gravity, in a two-dimensional plane. It is assumed that:

• Resistance from the air or liquid (known as fluid resistance) the object is travelling through is negligible

• Acceleration due to free-fall, g is constant as the object is moving close to the surface of the Earth

An object is sent into a projectile motion trajectory with a resultant velocity, u at an angle, θ to the horizontal. The trajectory of an object undergoing projectile motion consists of a vertical component and a horizontal component. These quantities are independent of each other

• Vertical component = opposite = sinθ × hyp = u sinθ

• Horizontal component = adjacent = cosθ × hyp = u cosθ

• time of flight - uses vertical component when v=0 doesnt use s * by 2

• maximum height - uses vertical component when v=0 finding s

• range - uses horizontal component and the time of the flight (2u sin θ / g) and acceleration is zero. distance = speed * time

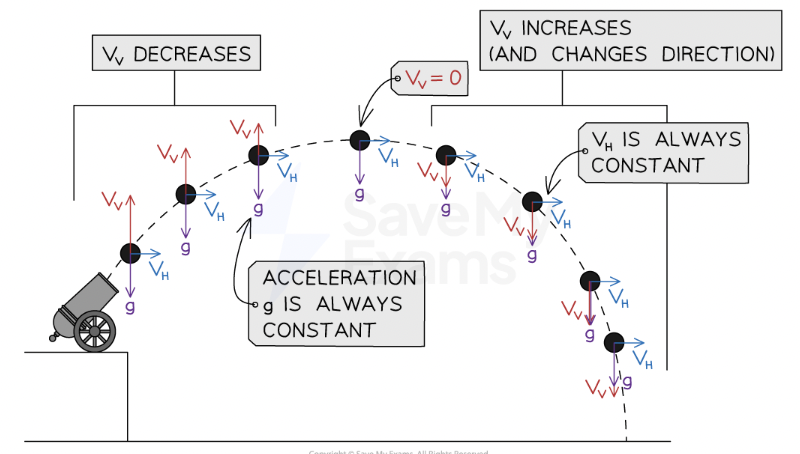
The qualitative effect of fluid resistance on projectiles, including time of flight, trajectory, velocity, acceleration, range and terminal speed

Fluid resistance refers to the effects of gases and liquids on the motion of a body. When an object moves through a fluid (a gas or a liquid), there are resistive forces for that movement, frictional force. Frictional forces:

• Always act in the opposite direction to the motion of the object

• Always slow down an object or keep them moving at a constant speed

- Time of flight: With air resistance, the object spends less time in the air.
It slows down the upward motion faster, and though it also slows the fall, the total time is usually shorter

- Trajectory: Without air resistance, the path is a perfect parabola. With air resistance, the trajectory becomes steeper and less symmetric

- Velocity: Air resistance reduces velocity in both horizontal and vertical directions. So, the object slows down more than it would without resistance.

- Acceleration: Gravity still pulls downward, but air resistance adds an upward or backward force, depending on the direction of motion.
This means acceleration is no longer constant — it changes throughout the motion.

- Range: The projectile travels a shorter horizontal distance because the horizontal velocity is reduced by air resistance.

- Terminal speed: As the object falls, air resistance increases until it balances the force of gravity. At this point, the object reaches terminal speed, a constant downward velocity where acceleration = 0.

Newton’s three laws of motion

• First law: If the net force on an object is 0 on an axis, the acceleration is 0 on that axis and vice versa (if there is a nonzero net force on an axis, there is a nonzero acceleration on that axis). it tells us that if an object is slowing down there must be a nonzero net force slowing it if there were no forces acting on an object it would keep the same velocity forever

  • inertia means an objects tendency to keep its same velocity unless acted on by a force. objects with more mass have more inertia because it takes more force to change their velocity by the same amount.

• second law: the net force on an object is equal to the mass of the object multiplies by the acceleration that the force creates. the acceleration always point in the same direction as the net force.

  • F = ma (Fx=max, Fy=may) (only works for net force, so find the forces acting on the object in the x and y direction (which equals the net force in that direction) and set them equal to ma)

  • this connects with first law since if net force is 0 acceleration is 0

• Third law: if object 1 puts a force on object 2, object 2 puts a force with an equal magnitude and opposite direction on object 1

  • ma = ma (if one has a bigger mass than the other it would mean that the acceleration on the smaller object would be much larger than the acceleration on the larger object)

  • Force is the same but acceleration is different due to different masses

  • action-reaction pair is a set of 2 equal and opposite forces that are created by 2 objects interacting with each other. (x pushes on y, y pushes on x)

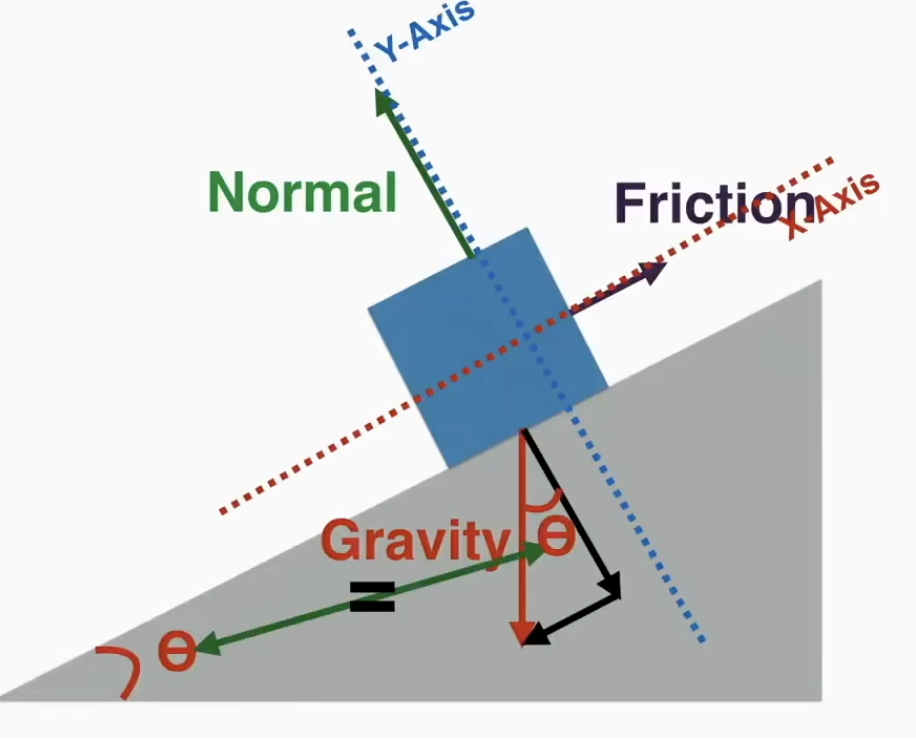
ramp problemss

• normal

• friction

• gravity (mg)

rotate x and y axis so only gravity has a x and y component

if not moving then normal = y component of gravity and friction = x component of gravity

forces as interactions between bodies

A force is a push or a pull on an object, vector, measured in newtons (N)

add multiple forces together for net force (sum of all forces acting on an object)

that forces acting on a body can be represented in a free-body diagram

that free-body diagrams can be analysed to find the resultant force on a system

free-body diagrams use a box with vectors as arrows showing magnitude and direction of all the forces acting on an object (taking all x components and y components and using pathagoras to find net force)

Net force: total net force in the x direction and in the y direction (missing forces found is net force equals zero)

normal force FN is the component of the contact force acting perpendicular to the surface that counteracts the body

the force created by a surface to oppose a force pushing on it

normal force always acts at a 90 angle to the surface that is creating it

always matches the net force that is pushing he object into the surface

surface frictional force Ff acting in a direction parallel to the plane of contact between a body and a surface, on a stationary body as given by Ff ≤ μsFN or a body in motion as given by Ff = μdFN where μs and μd are the coefficients of static and dynamic friction respectively

The force created by a surface on an object that opposes the object's motion. Friction always acts parallel to the surface and in the opposite direction of the object's motion. Its strength depends on the material of the surface and the object itself.

Friction depends on the normal force acting on the object from the surface, and the material the surface and object are made of. the bigger the normal force the bigger the force of friction.

So the coefficient of friction (μ) is the ratio of the force of friction to the normal force. It depends on the materials the surface and object are made of. bigger coefficient of friction means bigger force of friction.

so: μ = Ff/FN meaning Ff = μ*FN

2 types of friction:

• Static friction: prevents an object from moving. Balances out forces acting parallel to the surface of the object as long as those forces are below a certain maximum. Ff ≤ μsFN. (normal force can be found from weight and coefficient is given to find static friction. this is the maximum amount that the static friction can push back before it begins to move)

• Kinetic friction: acts against a moving object's velocity. Always points opposite the velocity. Only depends on the normal force. Ff = μdFN (if velocity is more it will speed up and if kinetic friction if more it will slowly come to a stop)

The coefficient of static friction is greater than the coefficent of kinetic friction for any surface

Applied force (Fa) (dont have to know)

the force of a push or pull from a person or another object

Spring force (Fs) (dont have to know)

the force exerted on an object by a spring. the more the spring the stretched or compresses the more force acts on the object. a spring does not apply any force if it is at its natural length

Air resistance (Fair) (dont have to know)

When an object is moving through air, the air applies a force in the opposite direction. An object experiences more air resistance if it is moving faster or if it has more surface area.

A falling object reaches "terminal velocity" or its maximum velocity when the force of air resistance up equals the force of gravity down.

tension

the force created by a pull of a rope or string. tension always pulls in the exact opposite direction of the rope or string itself.

tension pulls with equal force and opposite direction on both ends of the rope

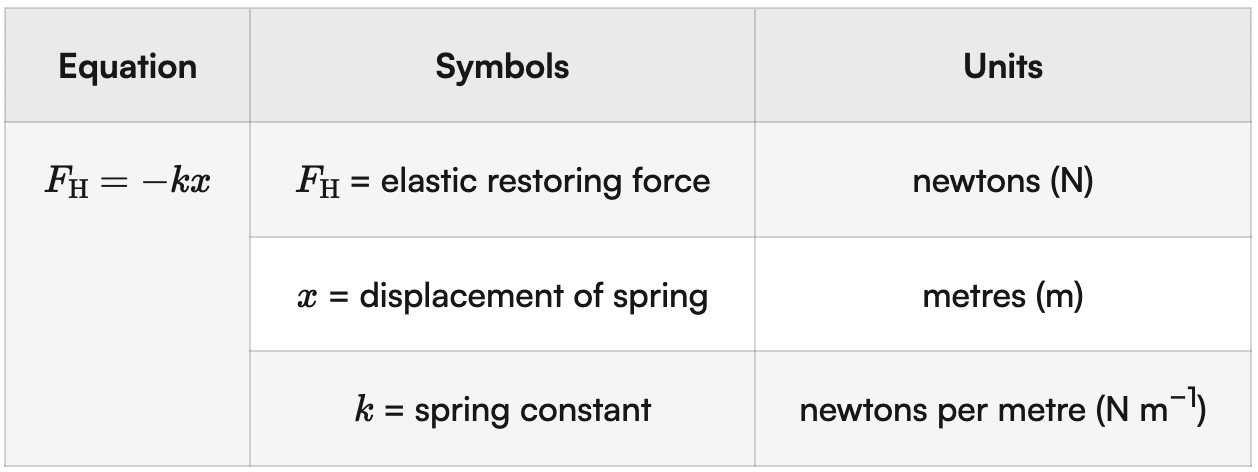
elastic restoring force FH following Hooke’s law as given by FH = –kx where k is the spring constant

When a spring is stretched, it exerts an equal and opposite force on the objects attached to each end of the spring. The force is directly proportional to the extension, according to Hooke's law

Elastic restoring force (FH) is the force that counteracts the force extending or compressing a spring and restores the spring to its natural length.

viscous drag force Fd acting on a small sphere opposing its motion through a fluid as given by Fd = 6πηrv where η is the fluid viscosity, r is the radius of the sphere and v is the velocity of the sphere through the fluid

Viscous drag force (Fd) is the resistive force opposing the motion of a body inside a fluid. Viscous drag force depends on the viscosity of a fluid. Viscosity is the property of a fluid that describes the fluid’s resistance to flow.

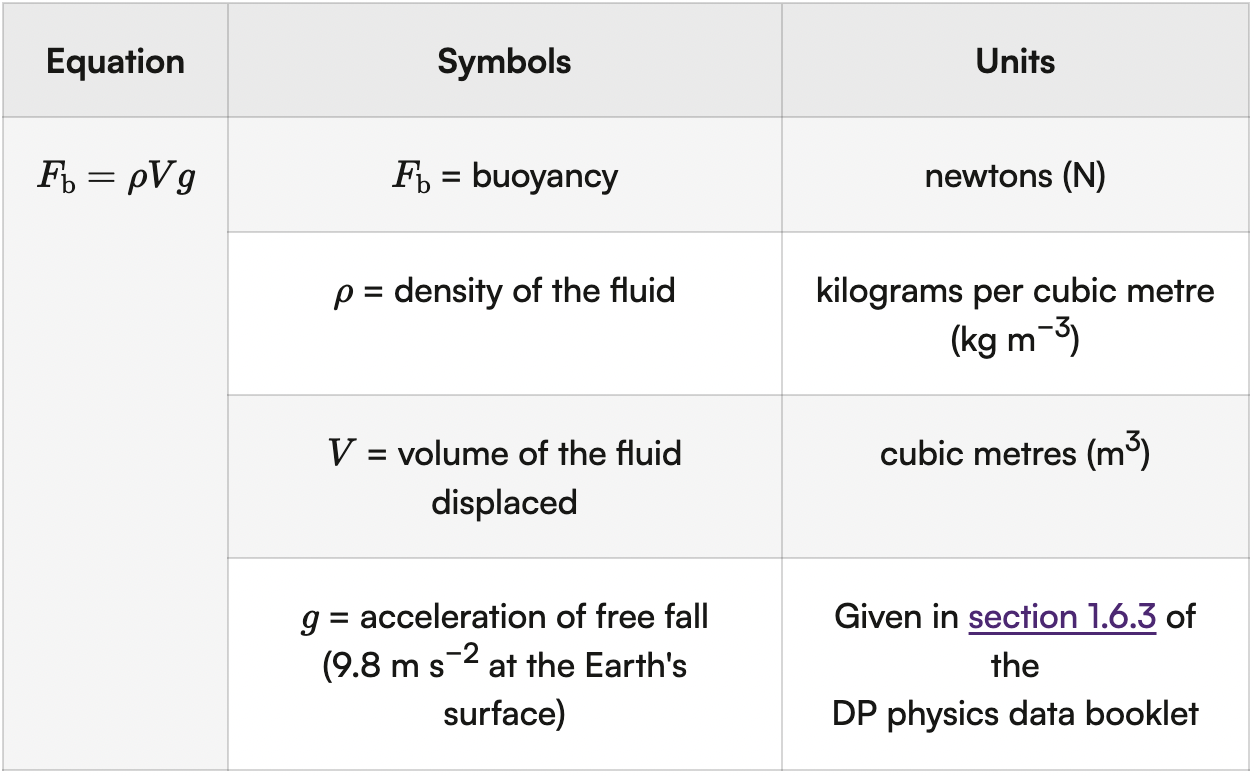
buoyancy Fb acting on a body due to the displacement of the fluid as given by Fb = ρVg where V is the volume of fluid displaced

Buoyancy (Fb) is the force experienced by a body when it is partly or fully immersed in a fluid (The force exerted by a fluid on an object partly or wholly immersed in the fluid, that counteracts the weight of the body.)

gravitational force Fg is the weight of the body and calculated is given by Fg = mg

the force of gravity is created between any 2 objects with mass (more mass = more gravitational force)

always points towards the centre of earth (on a ramp straight down)

also known as weight (weight = mass x acceleration of gravity (weight = m*g))

mass vs weight

mass is the amount of matter in an object (Kg), scalar. would stay the same in space as there is no gravity

weight is the force of gravity acting on an object (N), vector, would be no weight in space as there is no gravity

Force = mass * acceleration

Weight = mass * acceleration of gravity

electric force Fe

Electric force (Fe) is the interaction between bodies that can change the motion of a body, or change the shape or size of a body.

• Like charges (two positive charges or two negative charges) repel each other.

• Opposite charges (positive charge and negative charge) attract each other.

• The greater the charge, the greater the electric force.

magnetic force Fm

Magnetic force (Fm) is a non-contact force caused by the interaction between a magnet and a magnetic material, or between two magnets.

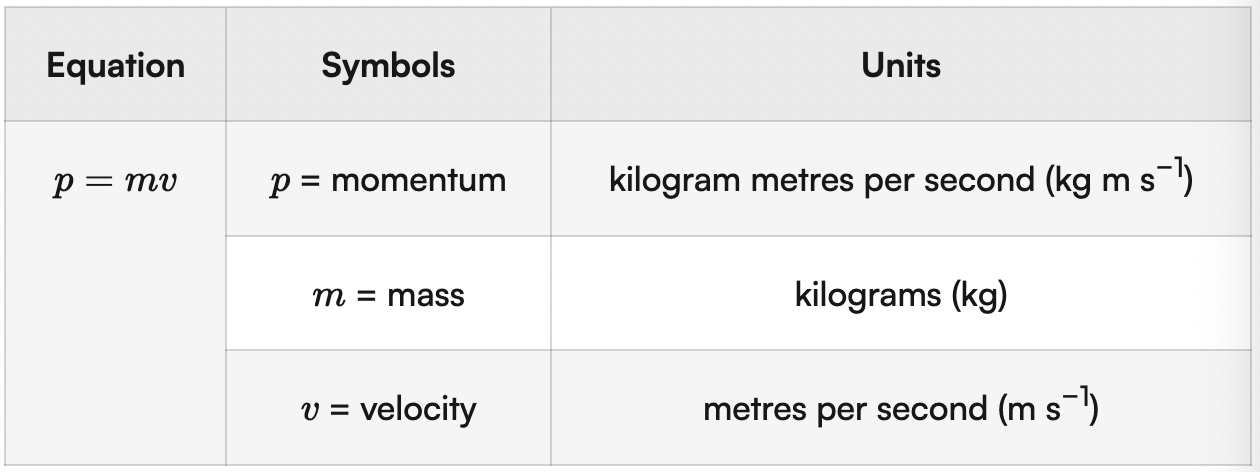
that linear momentum as given by p = mv remains constant unless the system is acted upon by a resultant external force

The linear momentum, p,  of a body is the product of the mass of the body and its velocity. Linear momentum is a vector quantity. The direction of linear momentum is the same as the direction of the velocity of the body. Linear momentum remains constant unless the system is acted on by a resultant external force.

momentum (p) is how difficult it is to make an object stop (Ns), vector

• more mass = more momentum

• more velocity = more momentum

so: p = mv

that a resultant external force applied to a system constitutes an impulse J as given by J = FΔt where F is the average resultant force and Δt is the time of contact

that the applied external impulse equals the change in momentum of the system

Impulse (Δp) is a change in momentum (final momentum - initial momentum) (Ns)

• Δp = ΣFt (=m(v-u)) or J = FΔt

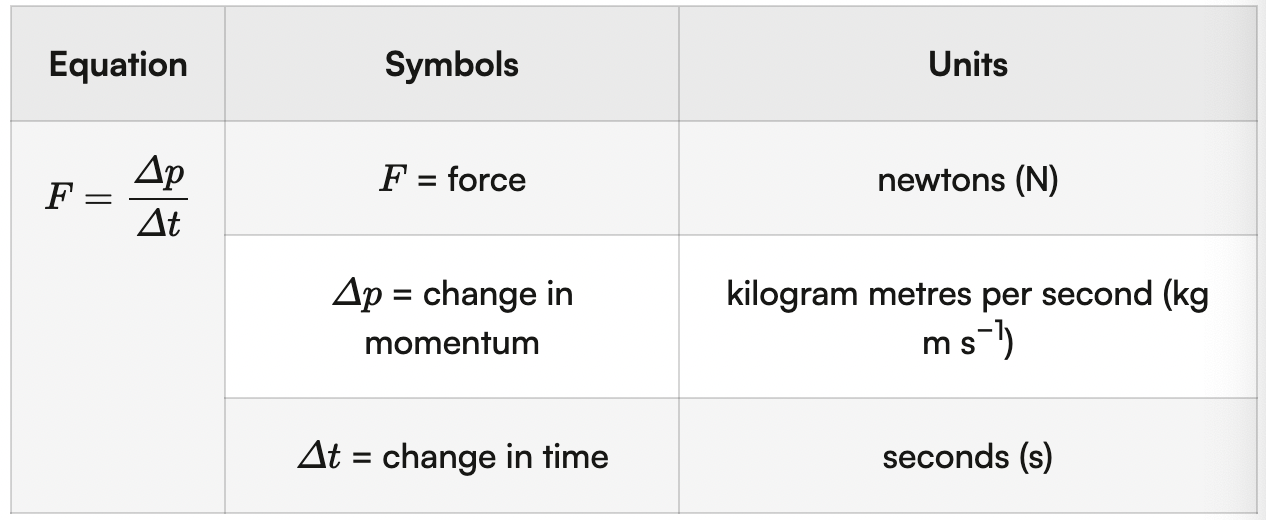
• F = Δp/t is identical to F=ma

a force-time graph shows to net force applied on an object on the graph’s y-axis and the time on the x-axis. the area under the curve of a force-time graph is equal to the impulse on the object. Can be used for a constantly changing force

Conservation of momentum

During a collision: 

• Newton's 3rd Law says the magnitudes of these two forces are the same, and their directions are opposite.

• The time is the same amount for both, because the collision always lasts the same time for both objects.

So Ft = -Ft or Δp1 = -Δp2

Σp = p1 + p2

The Law of Conservation of Momentum says that when two objects put forces on each other, the individual momentums of the objects change, but the total momentum of the two objects stays the same. This means that momentum can never be created or destroyed, only transferred from one object to another object.

this can be used in calculations by finding the total momentum in the system as this must stay and can only be transferred

open & closed system

• a system is a group of objects

• A Closed System is a group of objects that do not have a net force on them from any object outside the system. They can still put net forces on each other. In a closed system, the total momentum does not change.

• An Open System is a group of objects with a nonzero net force acting on them from some object outside of the system. In an open system, the total momentum changes. The total momentum after = the total momentum before + the impulse given by the outside object.

that Newton’s second law in the form F = ma assumes mass is constant whereas F = Δp allows for situations where mass is changing

Objects we encounter every day undergo changes in momentum so we use this:

the elastic and inelastic collisions of two bodies

• An elastic collision is when two objects collide and do not attach to each other. Σp = m1v1 + m2v2 = m1v1 + m2v2

  • if an objects hits a wall with an elastic collision, it bounces off with a velocity of the same magnitude and opposite direction that had pointed directly into the wall (total momentum changes since it is an open system (wall connected to earth) & because the velocity is now in the opposite direction

  • if it hits the wall at an angle only the part of the velocity that flipped is the component perpendicular to the wall (so angle an object impacts a wall will be equal to the angle it leaves the wall)

• An inelastic collision is when two objects collide and attach to each other. After the collision, they behave as one single object. Σp = m1v1 + m2v2 = (m1+m2)v

The total momentum in the system is the same before and after the collision meaning m1v1=m2v2

explosions

an explosion is when one object splits into two object. The total momentum in the system is the same before and after the collision

Σp = MV = m1v1 + m2v2

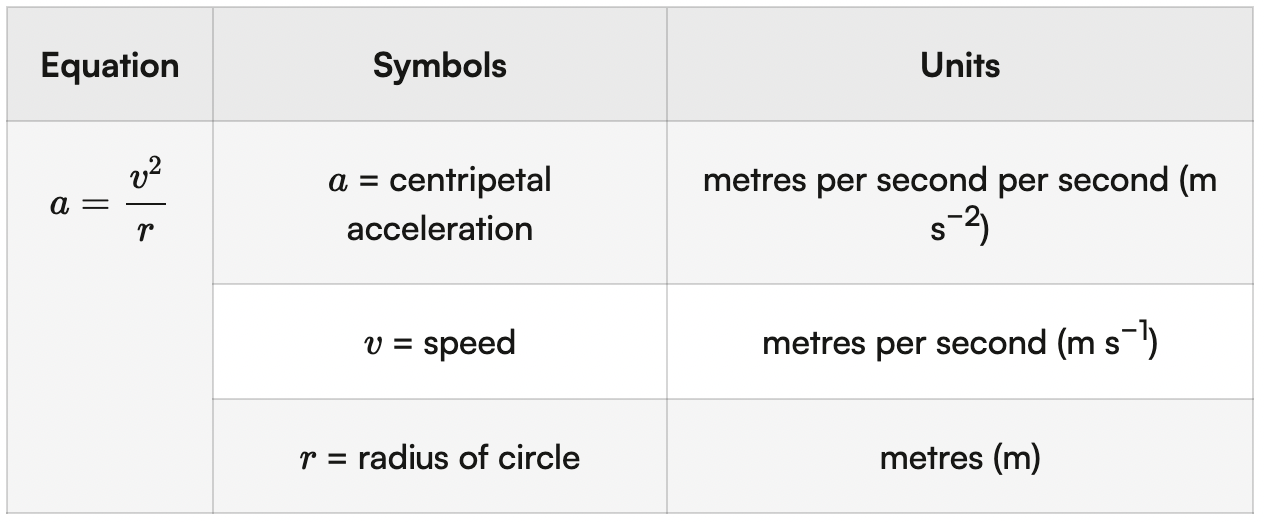
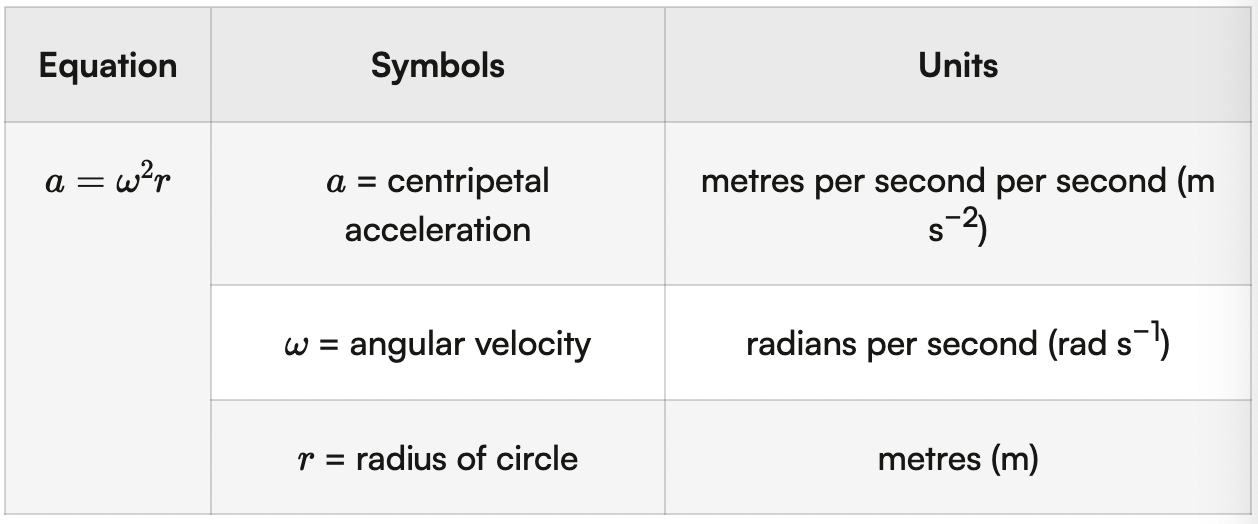
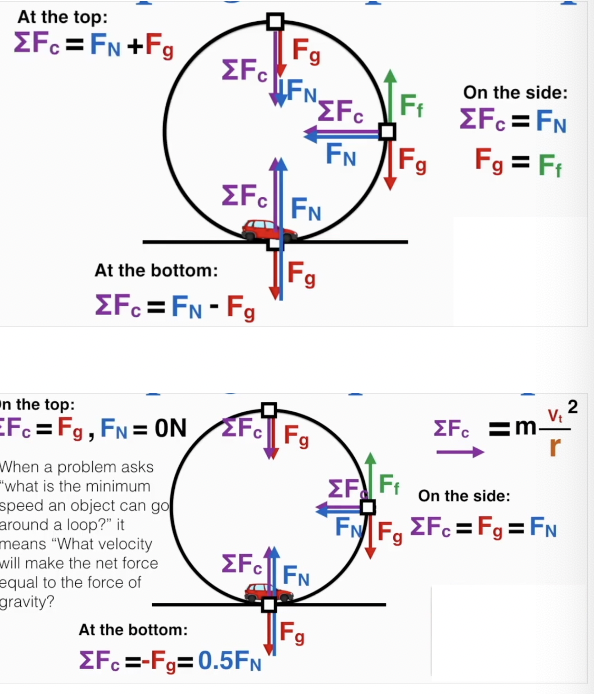
that bodies moving along a circular trajectory at a constant speed experience an acceleration that is directed radially towards the centre of the circle—known as a centripetal acceleration as given by

• a= v2 =ω2r= 4π2r r T2

1. The net force has to be constant and always pointing toward the centre

2. There has to be a constant velocity at a 90 degree angle to the net force

Centripetal acceleration: (ac) the acceleration of the object (points in the same direction as the centripetal force (ms^-2). Accelerations shows the direction of the change in velocity which is how the object is kept moving in a circle. Ac = Vt^2/r

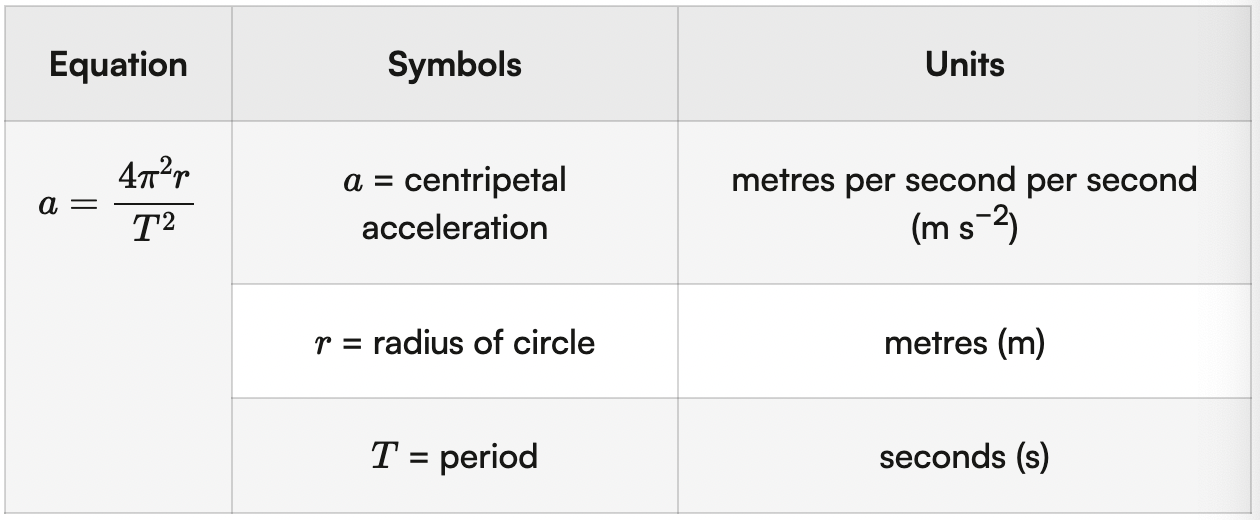
that circular motion is caused by a centripetal force acting perpendicular to the velocity

that a centripetal force causes the body to change direction even if its magnitude of velocity may remain constant

Centripetal force: (ΣFc) the net force on the object. It always points towards the centre of motion (N). Any combination of forces can add to make it. ΣFc = mac = m*(Vt^2/r)

Radius: (r) the radius of the circular path (m)

Tangential velocity: (Vt) the distance the object moves around the circle over change in time (m/s) Vt = d/t so Vt = 2πr/T = 2πrf

Vt = ωr

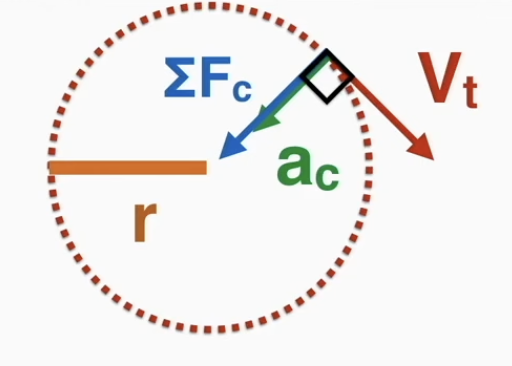
that the motion along a circular trajectory can be described in terms of the angular velocity ω which is related to the linear speed v by the equation as given by v = 2πr = ωr.

Angular velocity: (ω) the change in angle around a circle over the change in time (rad/s). This is different from tangential velocity as Vt takes the distance travelled into consideration (v=d/t) whilst ω tracks the angle from the starting position (meaning the circle could be much smaller but the ω is still the same, goes around the circle at the same rate) ω = Δθ/t = 2π/T = 2πf

Period and frequency

Period (T): the amount of time an object takes to complete one full cycle (s) 

Frequency (f): the amount of repetitions a cycle completes in 1 second (Hz)

T=1/f

spinning surface

p

turning roads

p

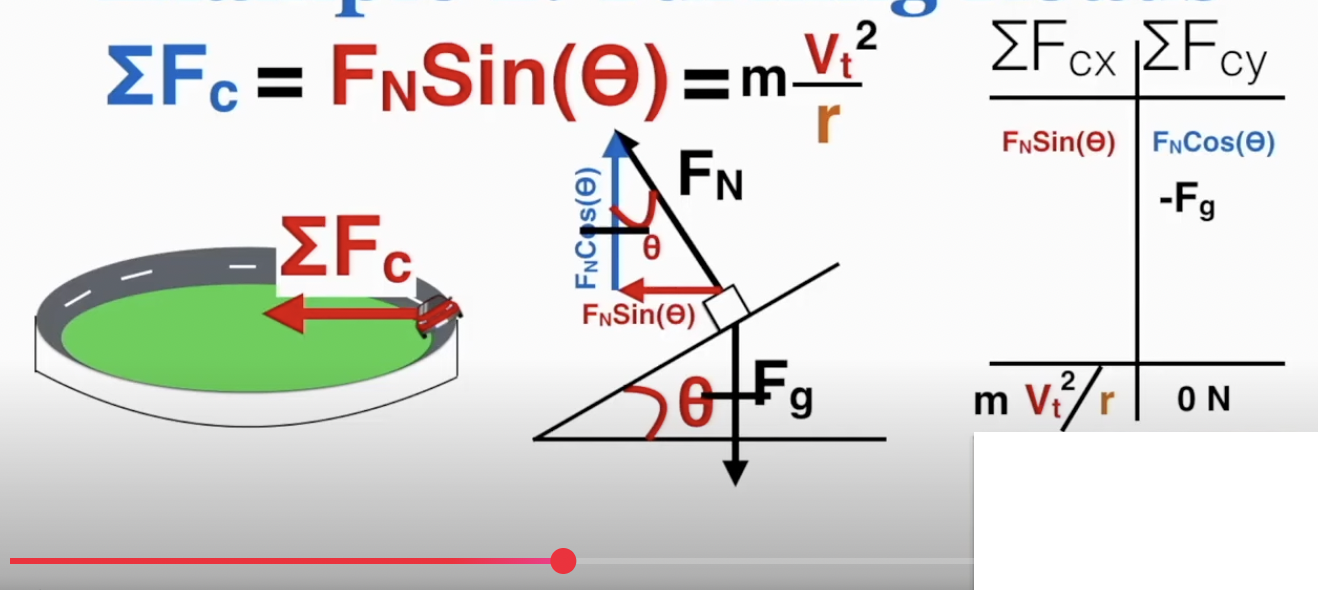
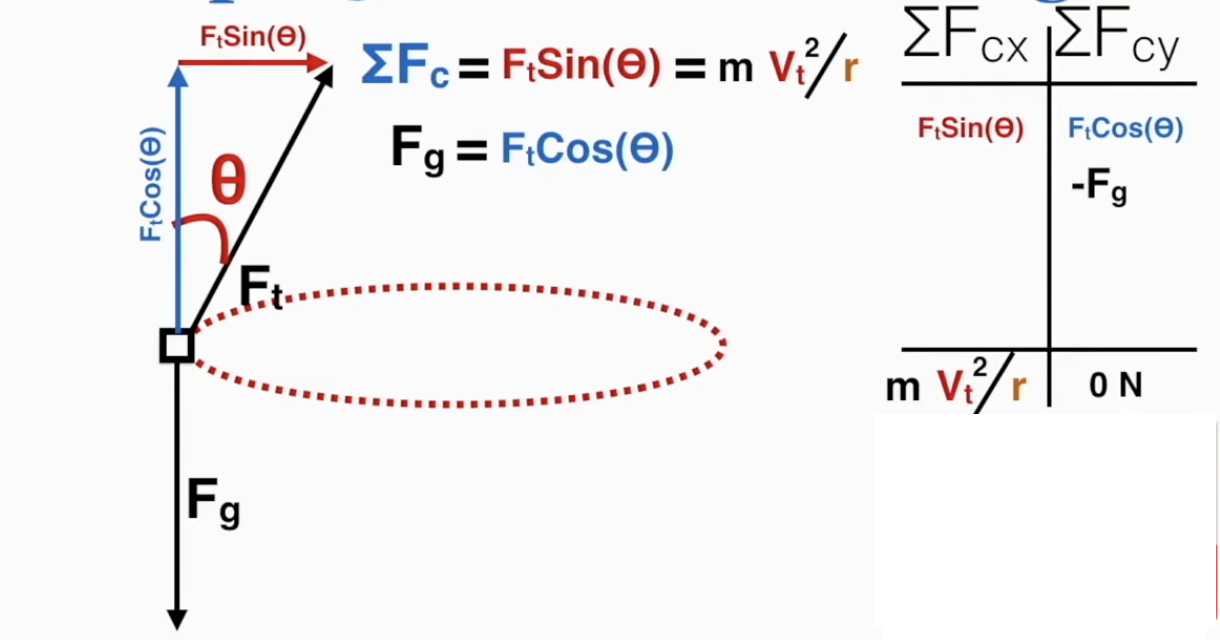
tension at an angle

p

hills

p

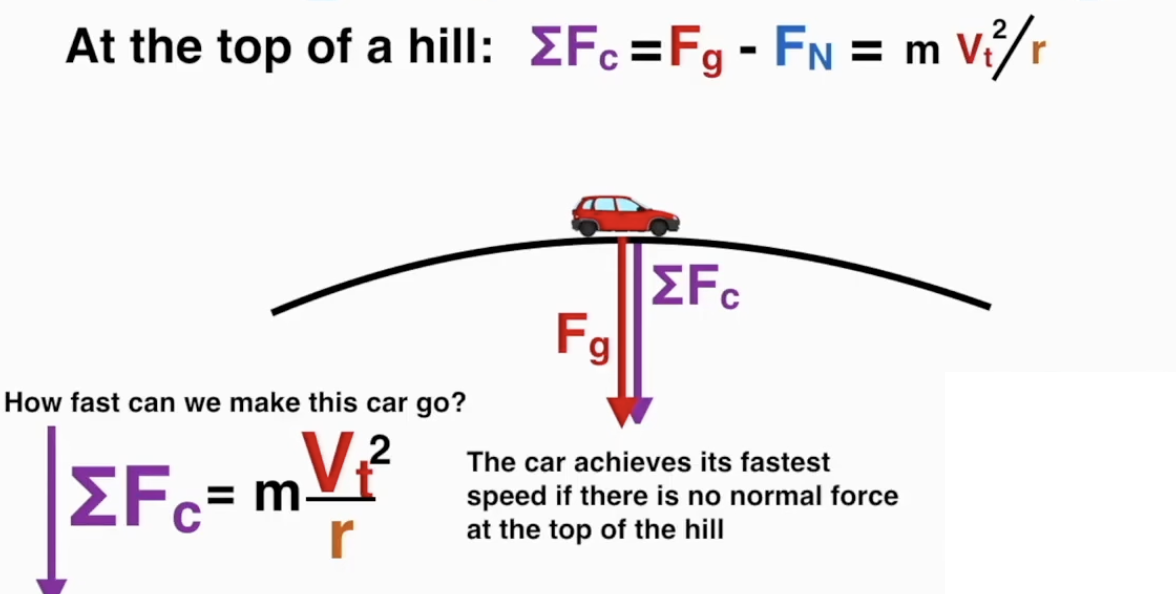
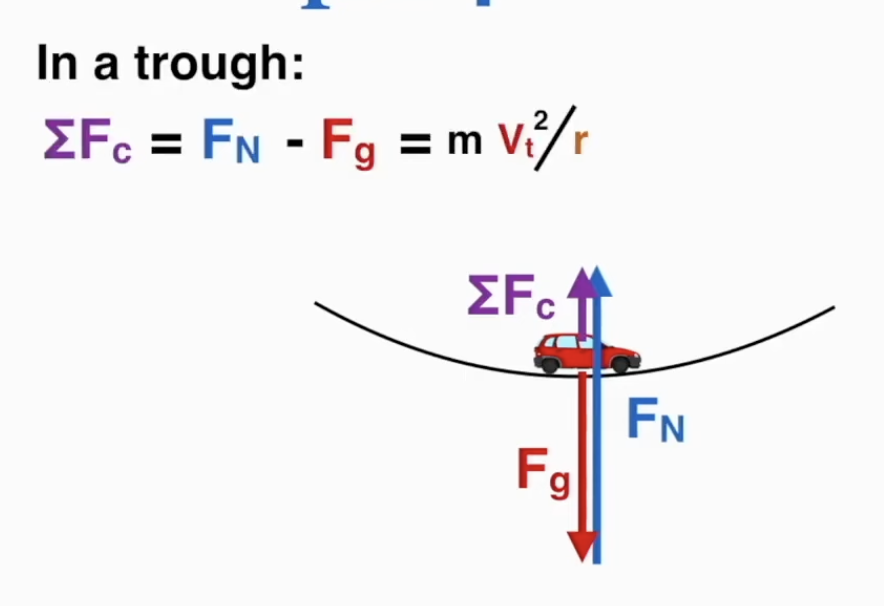
throughs

p

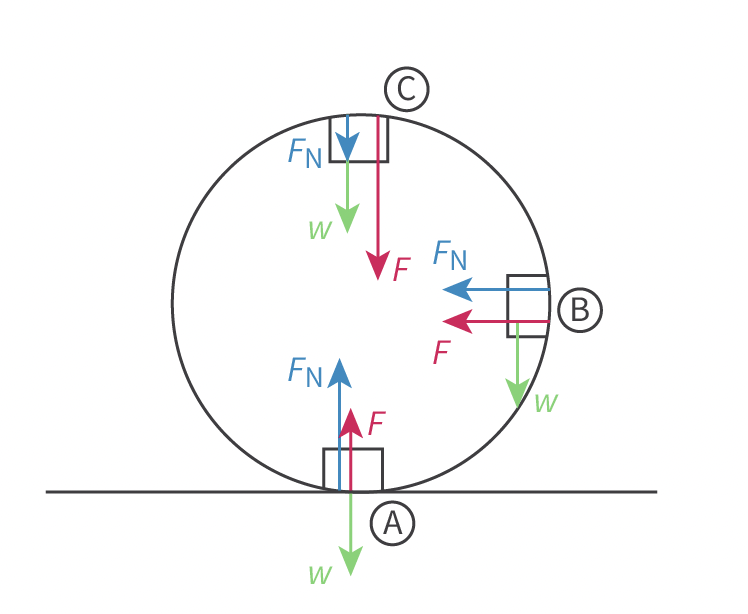
Loop

Fc would need to be bigger than Fg to stay on the circle

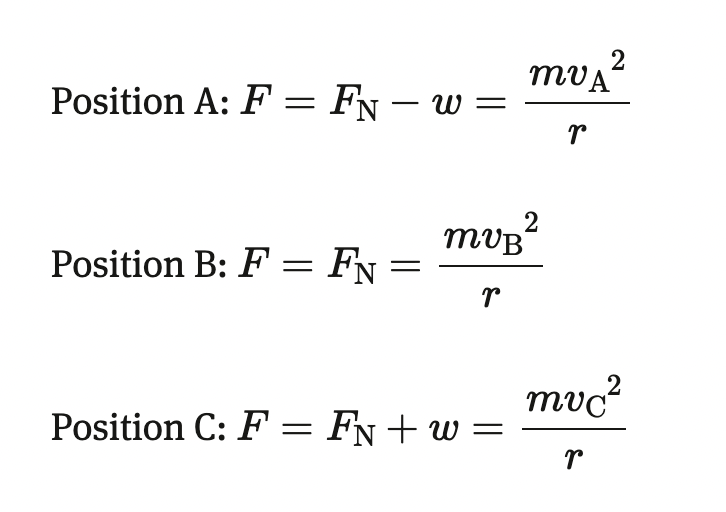
The principle of the conservation of energy

The Law of Conservation of Energy says that energy can NEVER be created or destroyed, only transferred from one object to another or transformed from one type of energy to another type of energy.

that work done by a force is equivalent to a transfer of energy

A force is said to do work when it acts on an object, and it transfers energy to it. Work done and energy transferred are both measured in  joules (J), and they are equivalent.

that energy transfers can be represented on a Sankey diagram

A sankey diagram is a scale diagram used to represent the total input, the useful output and the wasted output energies within a system. The thickness of each arrow is proportional to the amount of energy in each store.

• that work W done on a body by a constant force depends on the component of the force along the line of displacement as given by W = Fs cos θ

Work (W) is an objects displacement multiplied by the component of force parallel to the displacement (J), scalar

• W = Fs cos θ

if force is perpendicular no work is being done

force and displacement in the same direction the work done is positive, if they point in opposite directions work is negative

total work done use net force (or net force in the x component and y component)

that work done by the resultant force on a system is equal to the change in the energy of the system

Every time a force does work on an object, energy is transferred to the object. So the work done by the resultant force on the car is equal to the change in energy of the system. If the resultant force on the system is zero, the kinetic energy of the system will remain constant, and the change in energy will be equal to zero.

energy (E) is a measurement of the amount of work and object can do (J), scalar. object uses energy when it does work.

• W = ΔE

that mechanical energy is the sum of kinetic energy, gravitational potential energy and elastic potential energy

Mechanical Energy: Types of energy associated with the motion & position of an object that we can easily calculate and predict.

• Kinetic

• Gravitational Potential

• Elastic Potential

Non-Mechanical Energy: Other types of energy that are difficult to calculate and predict.

• Thermal (Thermal energy (Eint) is the energy associated with heat and temperature. Thermal energy appears as a result of friction, air resistance, impacts, and other interactions between objects. Thermal energy is actually a measurement of the random kinetic energy of vibrating particles in an object. (Eint = internal energy) (J). almost always lost from the system. So find for example Ep and Ek and the difference is lost as thermal energy. (perfectly elasatic nothing is lost))

• Electrical (Electrical energy is the energy carried by electrons. People normally use electricity to deliver energy created in power plants to buildings where we convert it into types of energy we use: light energy, heat energy, kinetic energy, etc.)

• Sound (Sound energy is the energy carried by sound waves. In almost all collisions at least some kinetic energy is transformed into sound energy. Sound itself is a wave of energy moving through a medium like air. The energy moves through without carrying the matter along with it.)

• Chemical (Chemical potential energy is the energy stored in the bonds between atoms or ions. It can be released or stored in chemical reactions.)

• Nuclear (Radiant energy is the energy carried by light and other forms of electromagnetic radiation (x-rays, microwaves, radio waves, gamma waves etc).)

• Radiant (Nuclear energy is the energy released during nuclear fission or fusion. It is contained within the nuclei of atoms until released.)

that in the absence of frictional, resistive forces, the total mechanical energy of a system is conserved

A Closed System in energy is a system where the only energy transfer and transformation that happens involves mechanical energy. Energy is not transferred to objects outside the system. The total energy of the objects in the system stays the same.

An Open System in energy is a system where energy is transformed from mechanical to non-mechanical or vice versa, or mechanical energy is transferred from or to an object outside the system. The total energy in the system changes.

that if mechanical energy is conserved, work is the amount of energy transformed between different forms of mechanical energy in a system, such as:

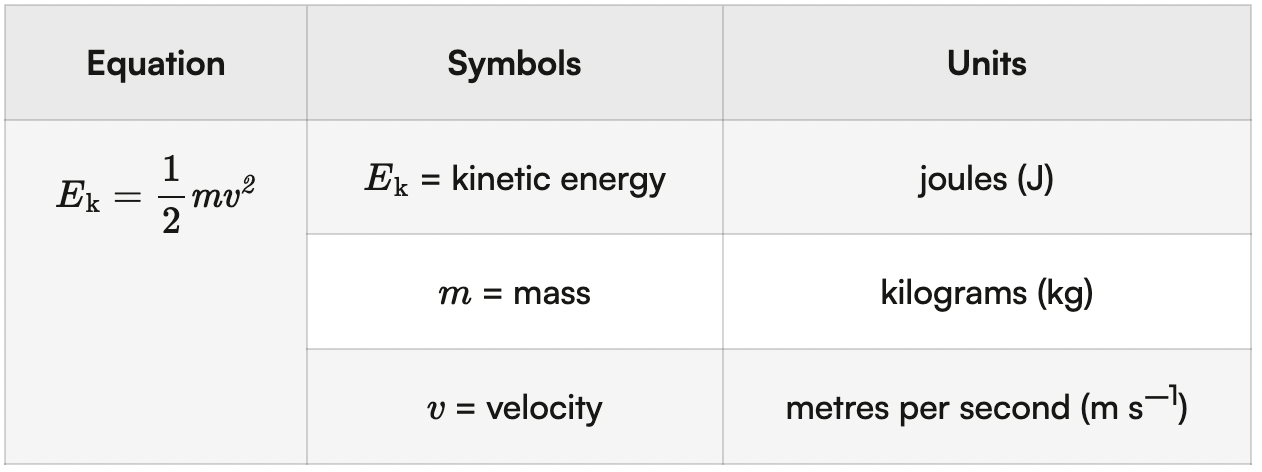
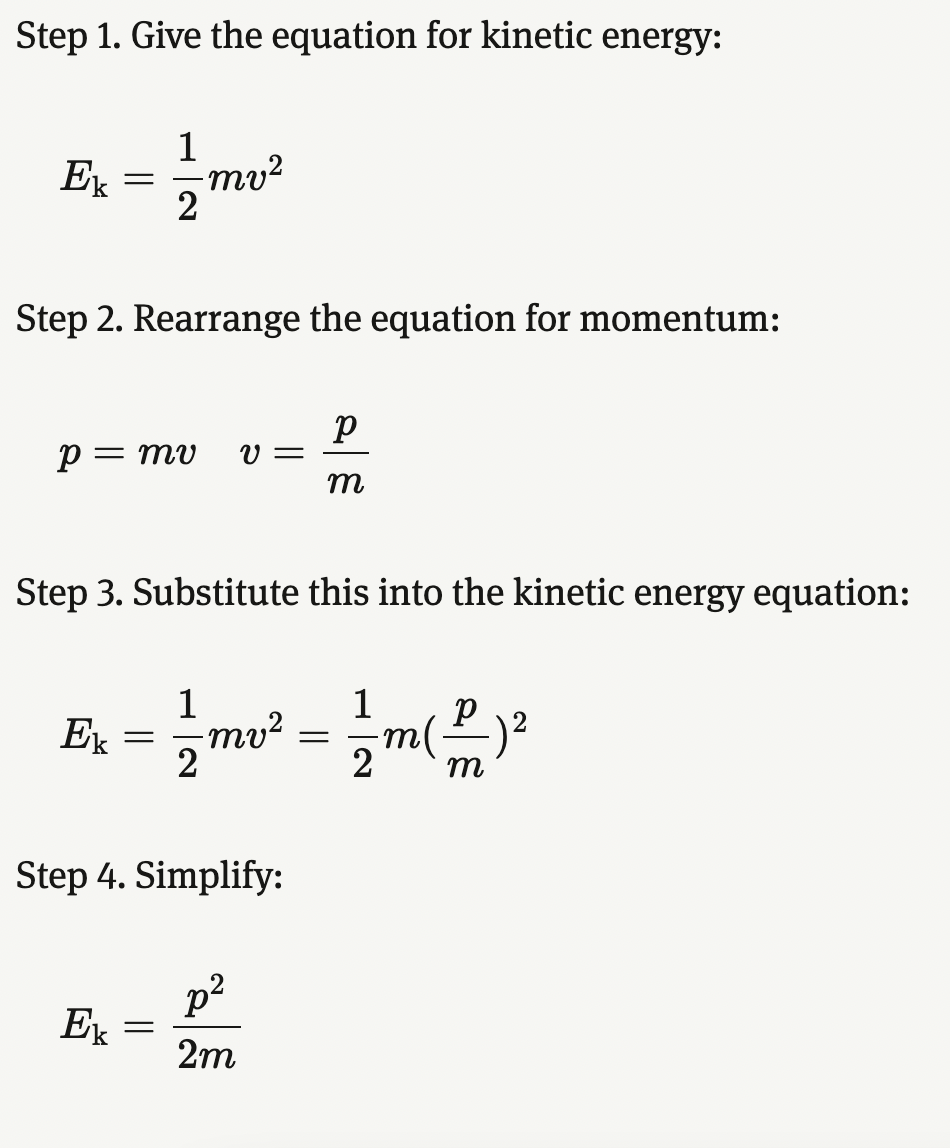
• the kinetic energy of translational motion as given by Ek = 1 mv2 = p2 2 2m

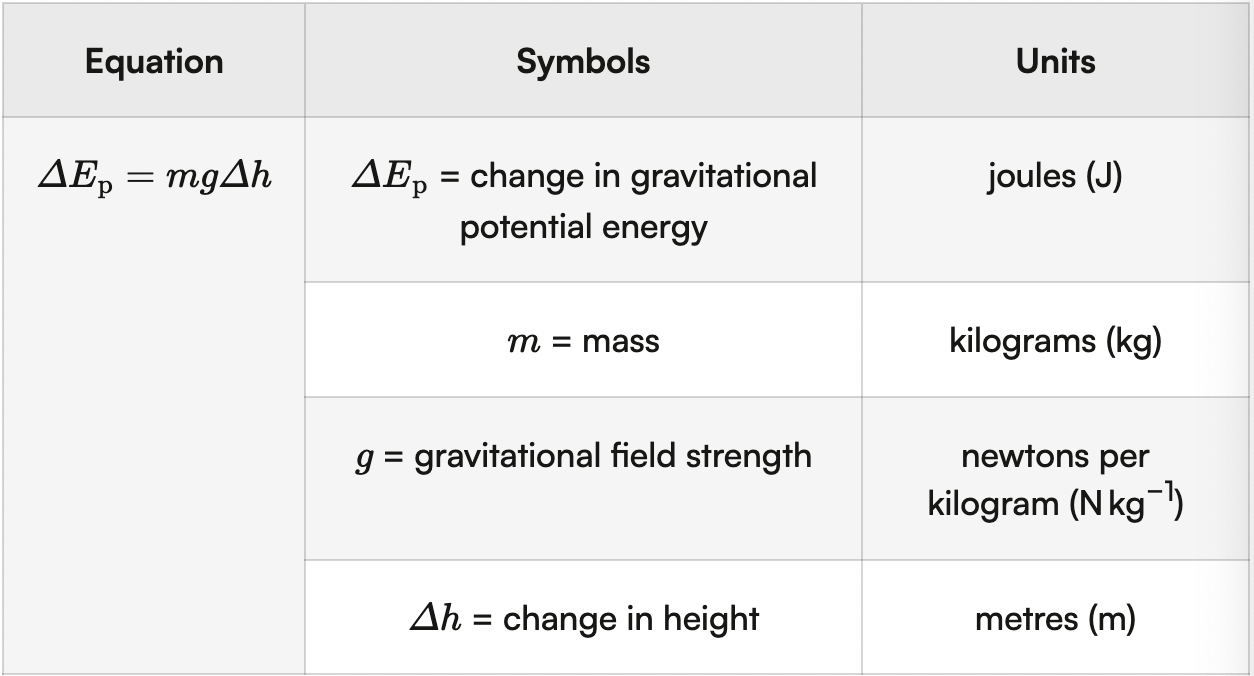
  •  the gravitational potential energy, when close to the surface of the Earth as given by

    ΔEp = mgΔh

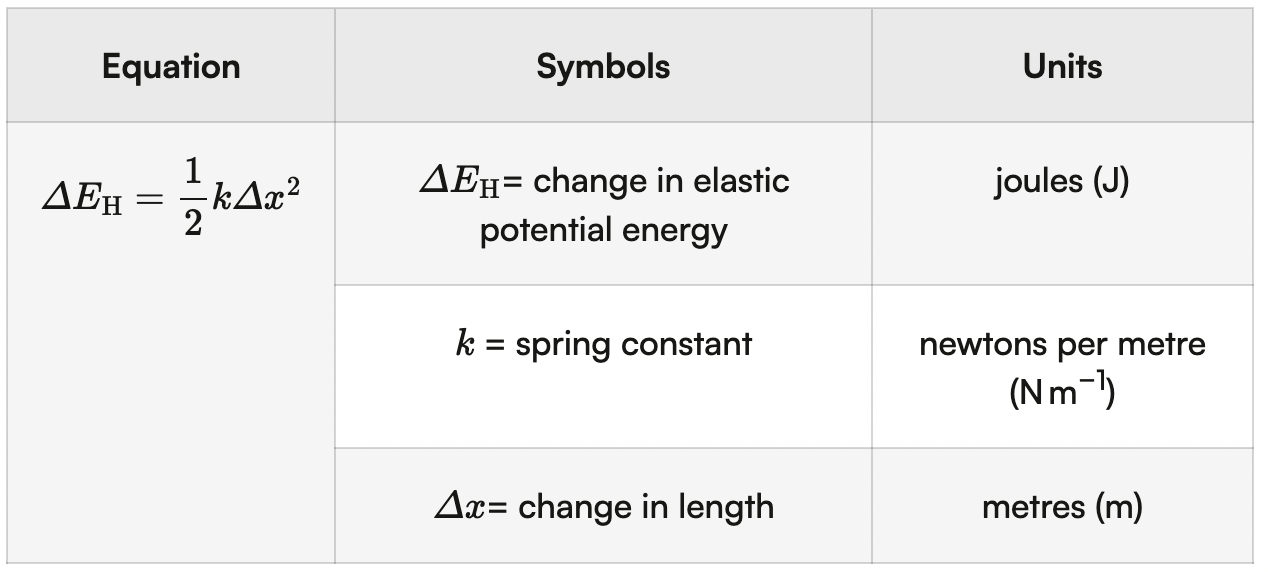
  •  the elastic potential energy as given by EH = 12k(Δx)2

Kinetic Energy (Ek) is the energy an object has as a result of moving. Thus, moving gives an object the ability to apply a force for a displacement, which is the ability to do work (J), can never be negative

Gravitational Potential Energy (Ep) is the energy an object has as a result of its ability to fall. As long as it has a vertical displacement (height) that it can move down, it can push or pull objects with its weight until it hits the bottom (J)

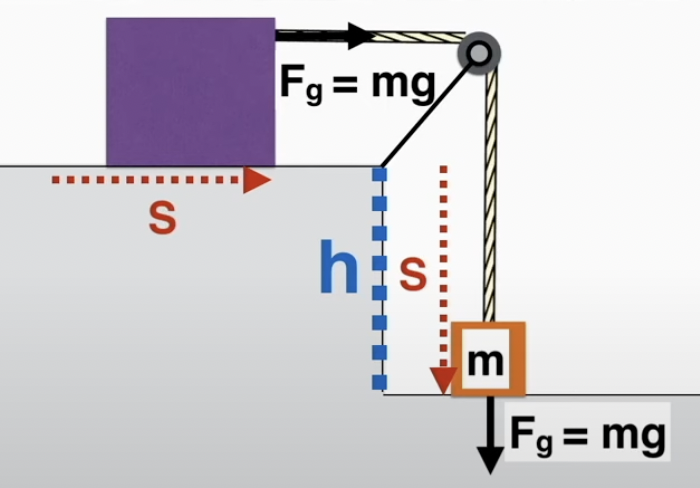
Elastic potential energy (Ep) is the energy stored in a spring that has been stretched or compressed from its natural length. When the spring is released, it will apply a force for a displacement until it returns to its natural length, so it has the potential to do work, so it has energy (J)

force displacement graph has slope of k and the area under the curve is the work done. 

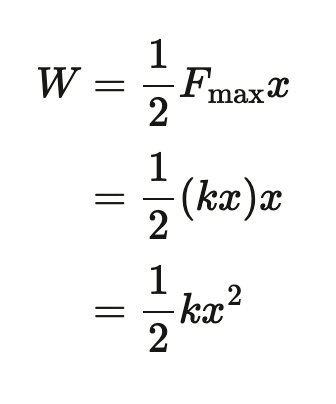
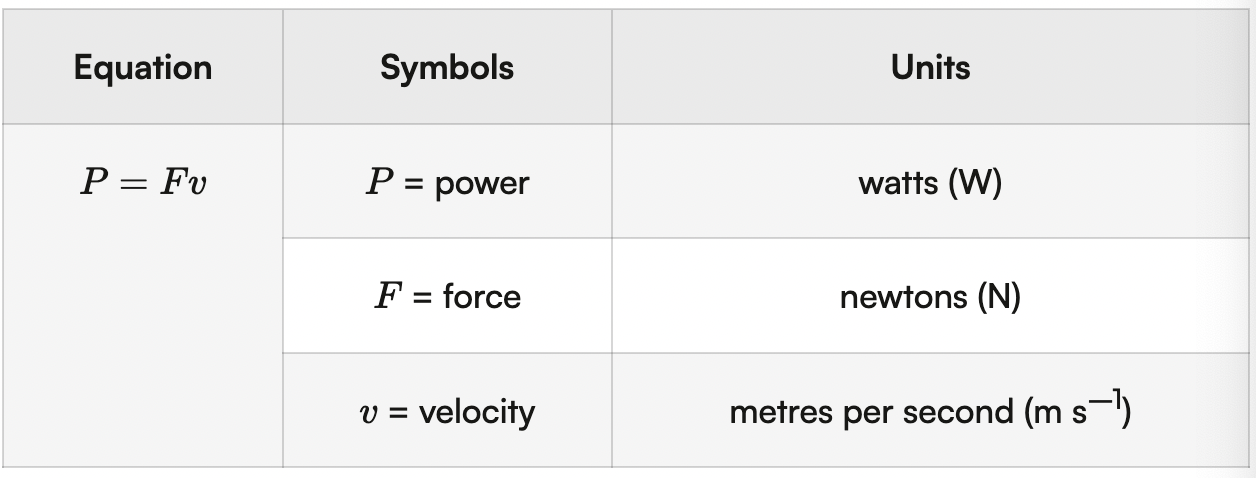
that power developed P is the rate of work done, or the rate of energy transfer, as given by P = ΔW/Δt = Fv

Power (P) is a measurement of a change in energy per time (The rate of work done). It's measured in units of watts. scalar. Power supplied to an object is also equal to the force applied to an object times the velocity of the object. (W)

• P=ΔE/t = W/t = Fs/t = Fv

efficiency η in terms of energy transfer or power as given by η = Eoutput = Poutput

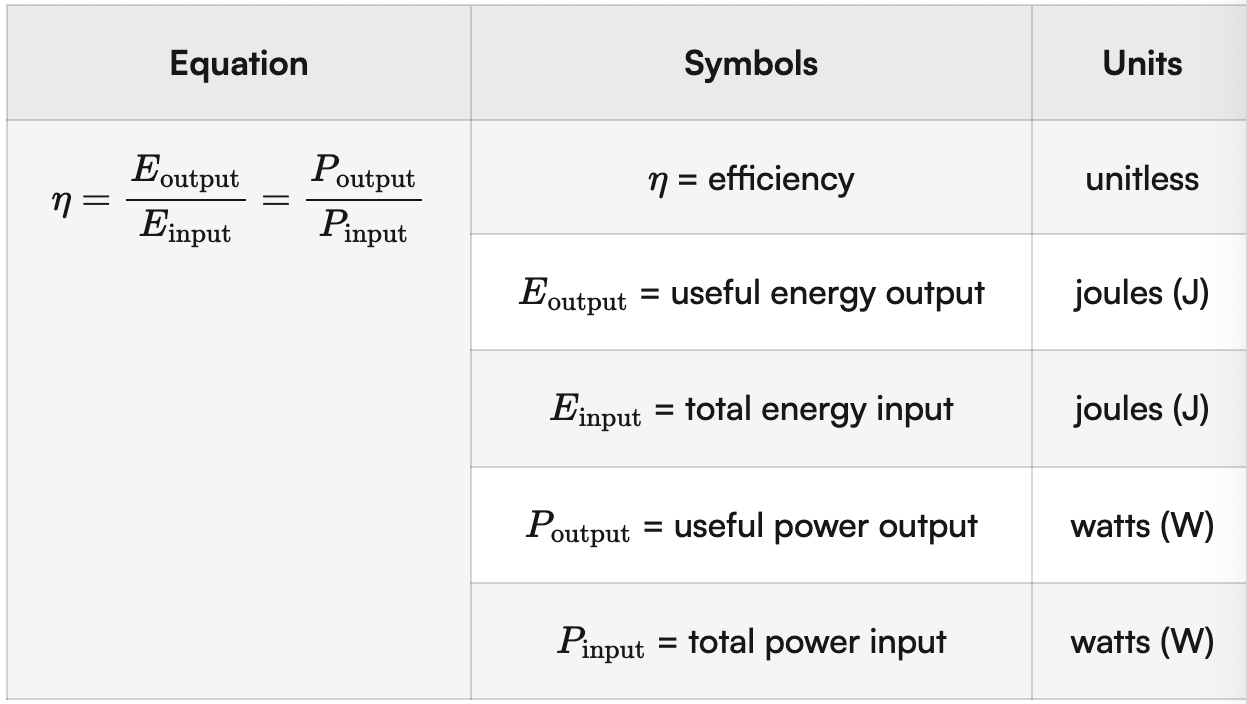
Efficiency is the ratio of how much useful energy or power we get out of a system vs. how much energy we put into the system.

• Efficiency = Useful work out / Total work in = Useful power out / Total power in

energy density of the fuel sources.

A fuel is a material that can be made to react with other substances in order to produce thermal energy or mechanical energy that can be used to do work. One of the things to consider when choosing a suitable fuel is energy density, which is defined as the amount of energy in a fuel per unit volume. Energy density is measured in joules per cubic metre (J m^–3)