Chapter 2 Tabular and Graphical Methods

Useful way of interpreting data is through

data visualization

• Help organize and present data

For a categorical variable:

frequency distribution, bar chart, pie chart

For two (or more) categorical variables:

contingency table, stacked bar chart

For a numerical variable:

frequency distribution, histogram, polygon, ogive, line chart

For two numerical variables:

scatterplot

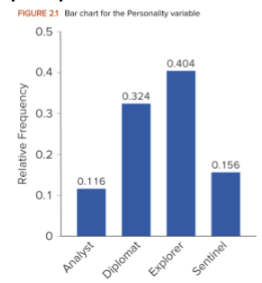
A frequency distribution for a categorical variable

groups the data into categories and records the number of observations that fall into each category

• ex) Myers-Briggs Assessment Results (1,000 employees)

A bar chart (column chart)

depicts the frequency or the relative frequency for each category of the categorical variable as a series of horizontal or vertical bars, the lengths of which are proportional to the values

A pie chart is a

segmented circle whose segments portray the relative frequency of each category for a categorical variable

When constructing or interpreting charts or graphs:

• The simplest graph should be used

• Each axis should be clearly marked and labeled

• Each bar/rectangle should be of the same width for creating bar charts or histograms

• The vertical axis should not be given a very high value

• The vertical axis should not be stretched

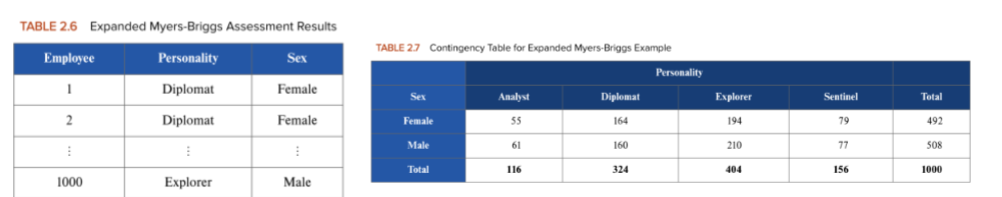
A contingency table shows

the frequencies for two categorical variables x and y, where each cell represents a mutually exclusive combination of the pair of x and y values

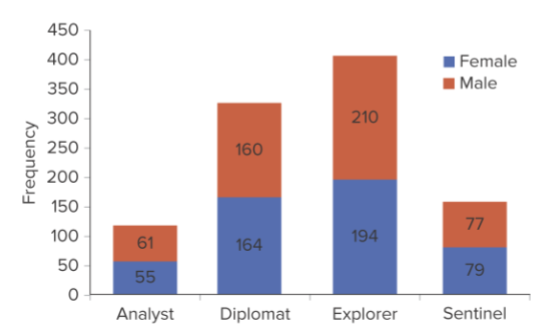
• ex) Expanded Myers-Briggs Assessment Results

A stacked column chart

graphically depicts a contingency table designed to visualize more than one categorical variable

A frequency distribution for a numerical variable

groups the data into nonoverlapping intervals and records the number of observations that fall into each interval

• ex) The price of a house for a sample of 40 houses in FL

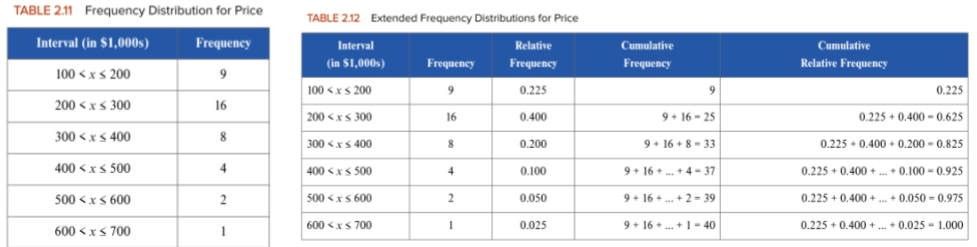
Guidelines when constructing frequency distribution

• Intervals are mutually exclusive

• Intervals are exhaustive

• Interval limits are easy to recognize and interpret

• The total number of intervals in a frequency distribution usually ranges from 5 to 20.

To calculate the width if 6 intervals

(Max - Min) / # of intervals

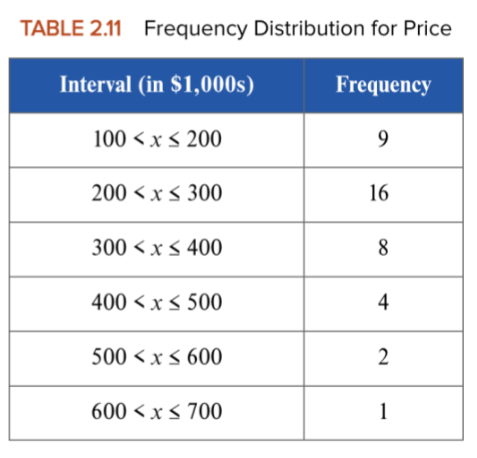
How many of the houses sold in the range of $300,000 up to $500,000?

8 + 4 = 12 houses

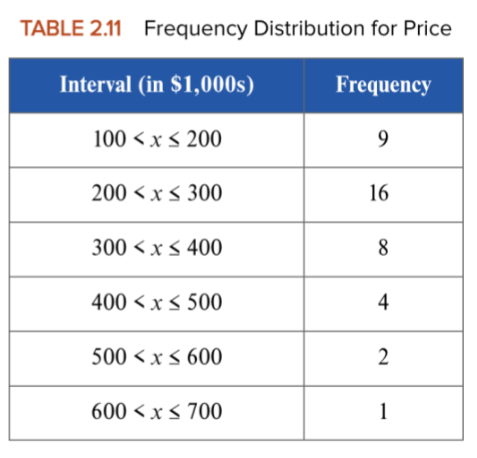
How many of the houses sold in the range of $200,000 up to $700,000?

16 + 8 + 4 + 2+ 1 = 31 houses

A relative frequency distribution records

the proportion of observations that falls into each interval

A cumulative frequency distribution identifies

the number of observations that fall below the upper limit of a particular interval

A cumulative relative frequency distribution shows

the proportion of observations that falls below the upper limit of a particular interval

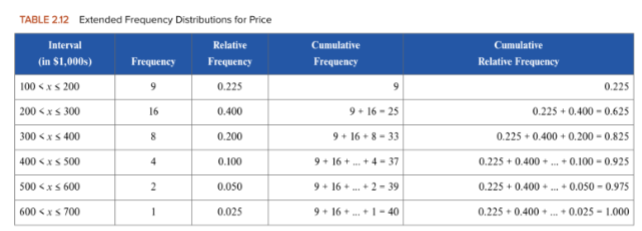
What proportion of the houses sold in the $200,000 to $400,000 range?

0.4+0.2 = 0.6

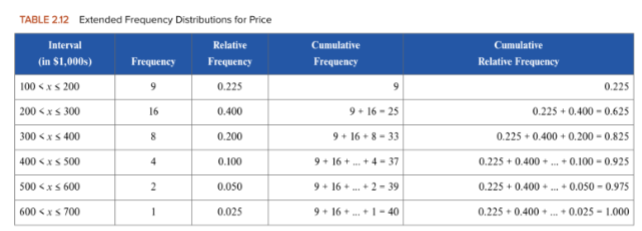
What percentage of the houses sold for more than $400,000?

0.1+0.05+0.025 = 0.175, 17.5%

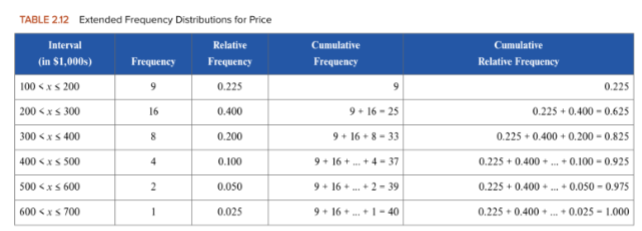
How many of the houses sold for $300,000 or less?

9+16 = 25 houses

A histogram is

a series of rectangles where the width and height of each rectangle represent the interval width and frequency (or relative frequency) of the respective interval

• The counterpart to the vertical bar chart for a categorical variable

• ex) A histogram of housing prices

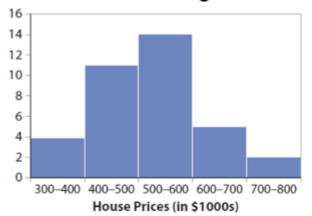
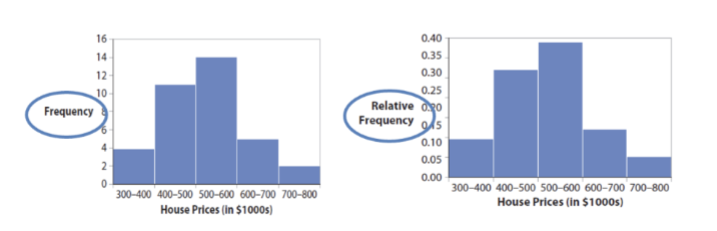
Here are the frequency and relative frequency histograms for the house-price data

Note that the only difference is the y-axis scale

Histogram Shapes of Distribution

typically, symmetric or skewed

Symmetric—

mirror image on both sides of its center

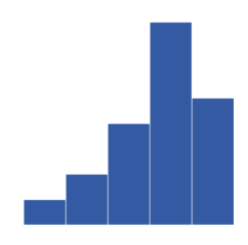
Positively skewed:

skewed to the right, long tail extends to the right

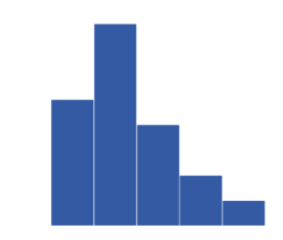
Negatively skewed:

skewed to the left, long tail extends to the left

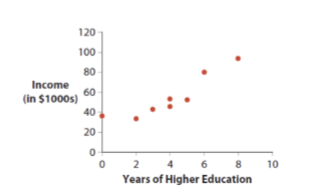
A scatterplot is

a graphical tool that helps in determining whether or not two numerical variables are related in some systematic way

• Each point in the diagram represents a pair of observations of the two variables: (x1,y1), (x2,y2), etc.

• Three relationships: Linear, Nonlinear, and No relationships

• ex) Incomes vs. Education

A linear relationship:

upward or downward sloping trend of data

• Positive linear relationship

• Negative linear relationship

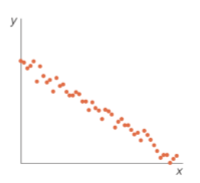
Positive linear relationship:

as x increases, y increases (positive slope)

Negative linear relationship:

as x increases, y decreases (negative slope)

Nonlinear Relationship

As x increases, y increases at an increasing or decreasing rate

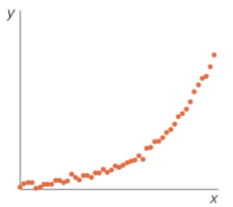
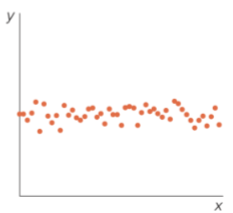
No Relationship

There is no apparent pattern between x and y

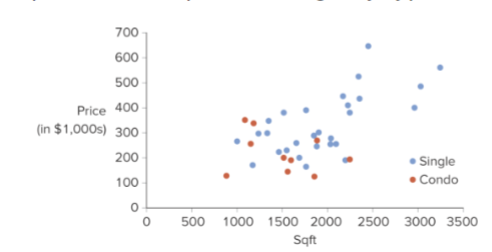
A scatter plot with a categorical variable modifies a basic scatterplot by

incorporating a categorical variable

• It is common to encode the categorical variable through point color

• ex) House prices and square footage by type in FL

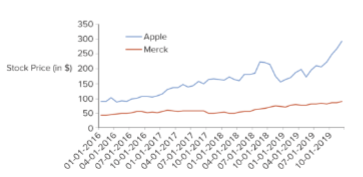
A line chart connects

the consecutive observations of a numerical variable with a line

• Useful for tracking changes or trends over time

• Easy to identify any major changes happened in the past

• Multiple lines can be plotted

• Ex) Monthly stock prices for Apple and Merck