Conic Sections Geometry Honors

distance formula (write it out)

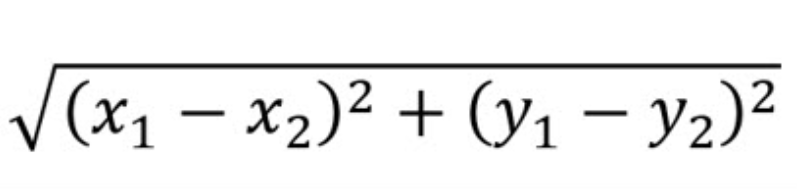
midpoint formula (write it out)

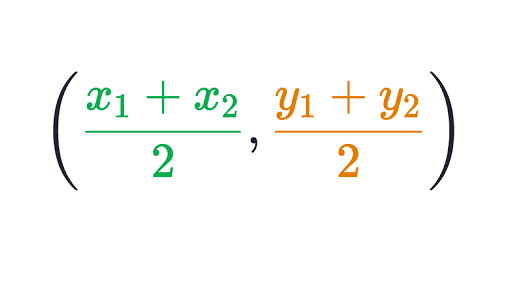
parabola vertical (0,0) equation

y=1/4p(x)²

parabola horizontal (0,0) equation

x=1/4p(y)²

directrix parabola (0,0) horizontal

x=-p

where is p on a parabola

distance from the vertex to the focus

directrix parabola (0,0) vertical

y=-p

if there isn’t both a x² and y² in a general equation, which conic section is it

parabola

if x² and y² are opposing signs in a general equation, what conic section is it

hyperbola

if the x² and y² values have the same sign and number in a general equation, what conic section is it

circle

if x² and y² have the same sign and different numbers in a general equation, what conic section is it

ellipse

when completing the square, when do you subtract at the end instead of add

only in parabola equations

vertical parabola equation

y=1/4p (x-h)²+k

horizontal parabola equation

x=1/4p (y-k)²+h

standard form for conic sections

y=a(x-h)²+k

general form for conic sections

y=ax²+bx+c

circle equation

(x-h)²+(y-k)²=r²

circle equation (0,0) center

x²+y²=r²

What is c distance to the center in ellipse and hyperbola

foci

Vertex (9,9) Vertex (9,-5) Focus (9,6)

Is this ellipse/hyperbola/parabola horizontal or vertical?

Vertical because the y value changed

Vertex (8,-2) Vertex (-4,-2) Focus (12,-2)

Is this ellipse/hyperbola/parabola horizontal or vertical?

Horizontal because the x value changed

Horizontal ellipse equation standard form

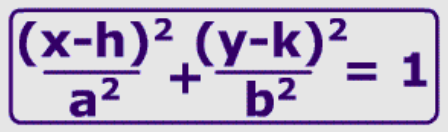
Vertical ellipse equation standard form

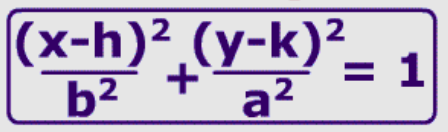
Whats the foci equation for an ellipse

c²=a²-b²

What is the one rule about the ellipse foci equation

a>b ALWAYS.

Vertices for a horizontal ellipse

(h+a,k) (h-a,k)

Co Vertices for a horizontal ellipse

(h,k+b) (h,k-b)

Foci for a horizontal ellipse

(h+c,k) (h-c,k)

Vertices for a vertical ellipse

(h,k+a) (h,k-a)

Co Vertices for a vertical ellipse

(h+b,k) (h-b,k)

Foci for a vertical ellipse

(h,k+c) (h,k-c)

Foci equation for hyperbolas

c²=a²+b²

Horizontal hyperbola equation standard form

Vertical hyperbola equation standard form

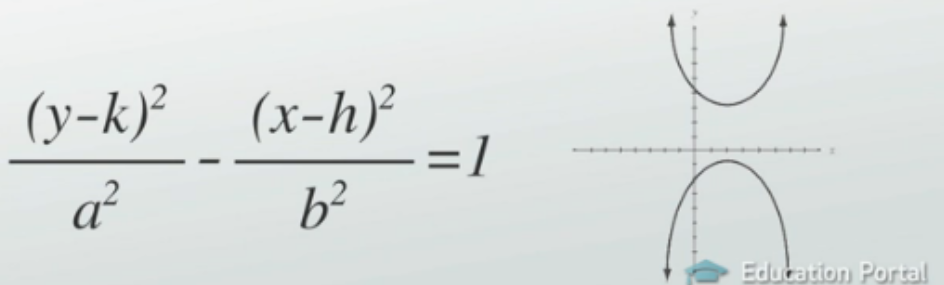
Vertices for horizontal hyperbolas

(h+a,k) (h-a,k)

Co Vertices for horizontal hyperbolas

(h,k+b) (h,k-b)

Foci for horizontal hyperbolas

(h+c,k) (h-c,k)

Vertices for vertical hyperbolas

(h,k+a) (h,k-a)

Co Vertices for vertical hyperbolas

((h+b,k) (h-b,k))

Foci for vertical hyperbolas

(h,k+c) (h,k-c)

Horizontal asymptotes

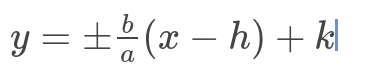
Vertical asymptotes

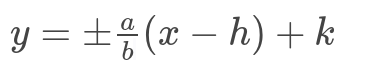
What is the equation between a and c in hyperbolas

c>a ALWAYS

Is a>b all the time

No, only in ellipses

What do we know about the relationships between a, b, and c in ellipses and hyperbolas

ellipses =

a>c

a>b

c≥b

hyperbolas=

c>a

c>b

Hyperbola

the locus (set) of all points in a plane such as that the difference of the distances from a point to two fixed points, F1 and F2 is a constant

Circle

the locus (set) of all points equidistant from a given point (the center)

Parabola

The set of all points in a plane that are the same distance form a given point, called a focus and a given line, called a directrix

Ellipse

the locus (set) of all points in a plane such that the sum of the distance from a point to two fixed points, F1 and F2 is a constant

What is b distance from the center in ellipse and hyperbola

covertices

What is a distance from the center in ellipse and hyperbola

vertices

parabola directrix horizontal

x=h-p

parabola directrix vertical

y=k-p