Nuclear Physics

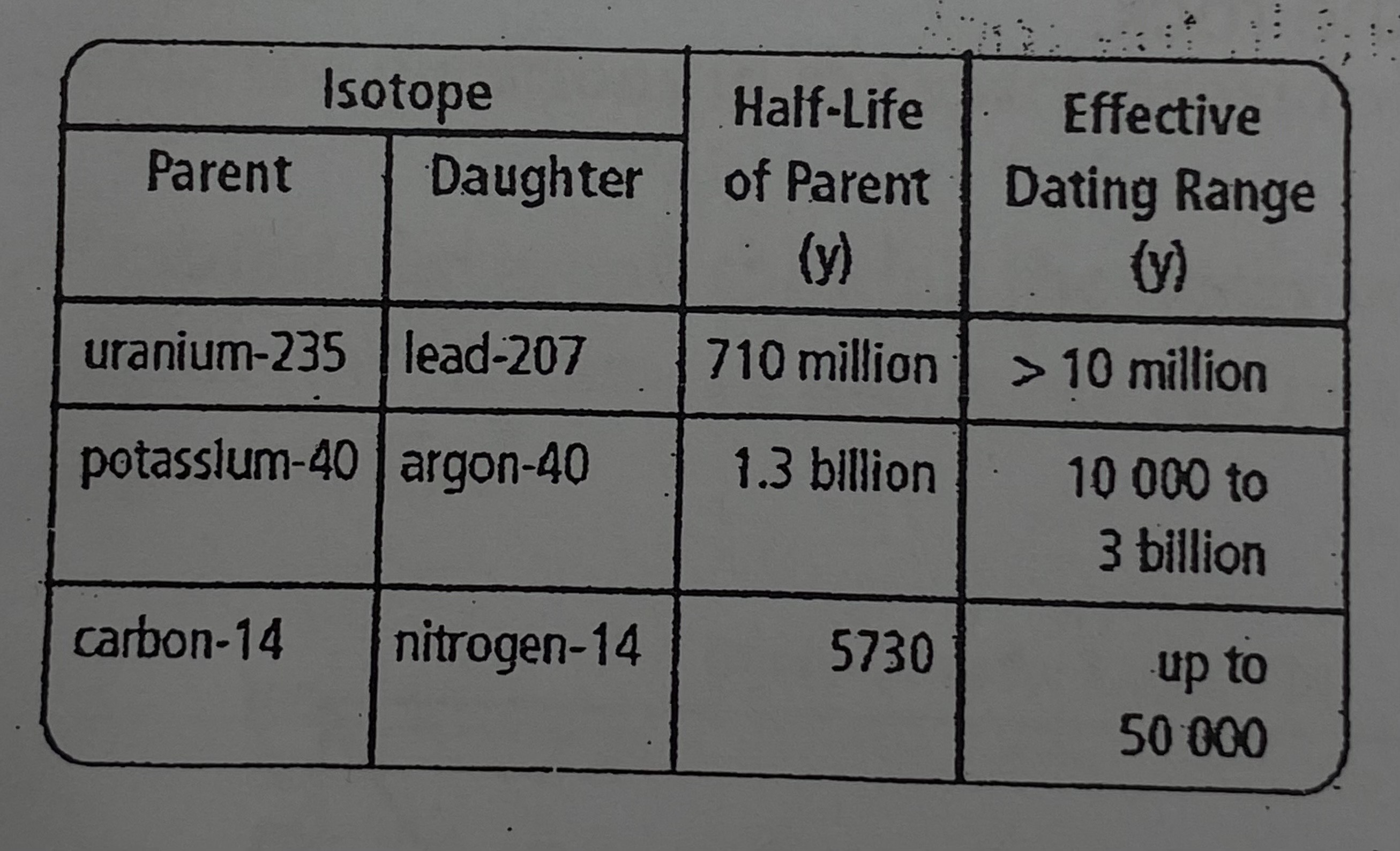
One half life of uranium-235 is 710 million years old. Estimate how many half-lives of uranium-235 would have passed in a rock sample that is between 3.7 and 3.8 billion years.

~Slightly more than 5 half-lives of uranium-235 would have passed.

EXPLANATION: Since one half-life of uranium-235 is 710 million years old, dividing 3.7 billion years by 710 million years gives approximately 5.2 half-lives/dividing 3.8 billion years by 710 million years equals approximately 5.3 half-lives. As a result, it is possible to determine that slightly more than 5 half-lives of uranium-235 have passed.

Estimate the percentage of uranium-235 that would remain after 5 half-lives have passed.

~3.125% of uranium-235 will remain after 5 half-lives.

EXPLANATION: To calculate the remaining amount of uranium-235, divide 100 by 2 five times or multiply 100 by 0.5 five times.

If a sample of rock was analyzed using the potassium-40 clock, what information could be given about the rock’s age?

The potassium-40 clock can provide information about the rock’s age.

EXPLANATION: Since one half life of potassium-40 is 1.3 billion years old, it is possible to conclude that the rock sample is more than 1.3 billion years old if the use of a potassium-40 clock is necessary.

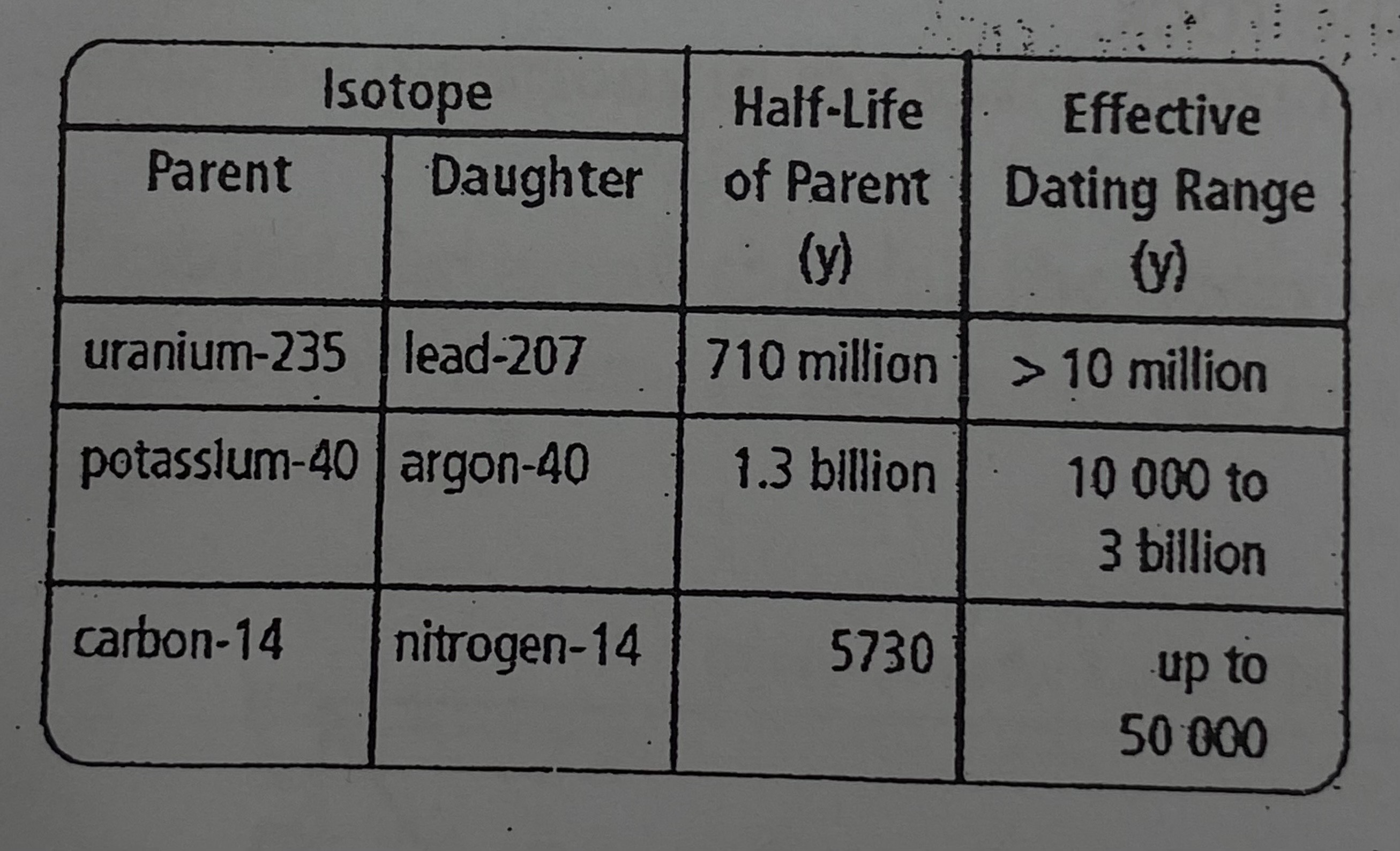
Could carbon-14 dating be used to estimate the age of a rock in the Canadian shield? (~3.7-3.8 billion years old)

Carbon-14 dating would not be efficient in estimating the age of a rock in the Canadian shield.

EXPLANATION: The effective dating range of Carbon-14 is limited to about 50,000 years therefore, it is not effective when dating an object older.