16.5 Gibbs free energy

What is the equation for Gibbs free energy?

• For a spontaneous process: ∆G __ 0

• For a non-spontaneous process: ∆G __ 0

• A process at equilibrium: ∆G __ 0

• Gibbs free energy represents the _____________; it is a ______ function

< , > , = , energy available to do work , state

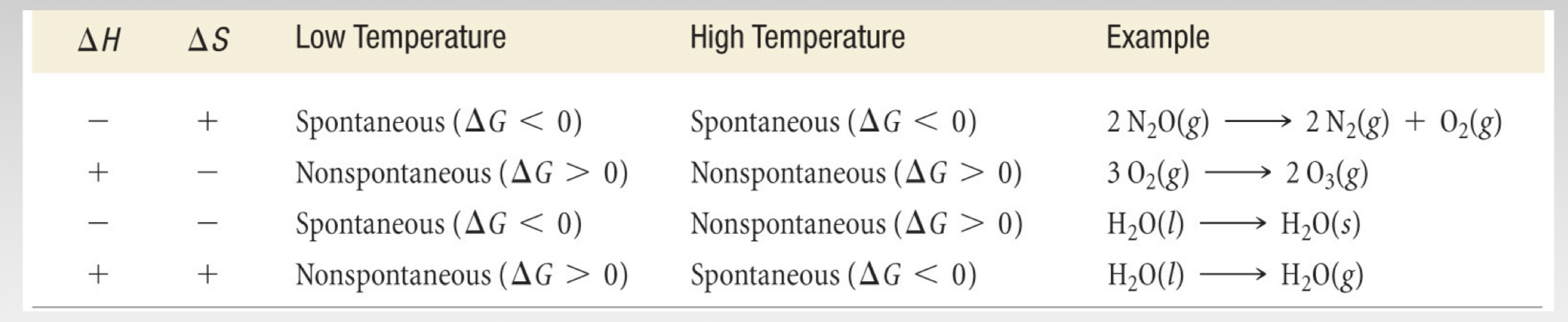
State the equation for calculating the ∆H of a chemical reaction.

∆H = ∑∆H_products - ∑∆H_reactants

State the equation for calculating the ∆S of a chemical reaction.

∆S = ∑∆S_products - ∑∆S_reactants

The entropy of a perfect crystal of a pure substance approaches 0 as the temperature approaches 0 K.

• Every substance that’s not a perfect crystal at _________ has _________ from entropy → absolute entropy of a substance is always ________.

3rd Law of Thermodynamics

Ans: absolute zero, some energy, positive

Conditions for standard state:

• Standard entropy (Sº): _______

• ∆Gº (∆G = ∆Gº only when reactants and products are in their standard states): _______

• Entropies for 1 mole at 298 K (25º C) for a particular state, particular allotrope, particular molecular complexity, particular molar mass, and a particular degree of dissolution

• Normal state at that temperature, partial pressure of gas = 1 atm, concentration = 1 M

∆G and ∆S are ______ properties.

extensive (dependent upon the amount of substance)

What is are the equations for ∆G_reaction at 298 K and temperatures other than 298 K (i.e. calculating Gibbs free energy of a reaction using ∆Gº_f for both situations)?

• ∆Gº_reaction = ∑n∆Gº_f(products) - ∑n∆Gº_f(reactants)

  • ∆Gº_f is the Gibbs free energy of formation: the energy associated with forming 1 mole of a compound from its components in their standard state

• Assuming the change in ∆Hº_reaction and ∆Sº_reaction is negligble: ∆Gº_reaction = ∆Hº_reaction - T∆Sº_reaction

  • determine ∆Hº_rxn and ∆Sº_rxn separately to determine ∆Gº_rxn

What is the equation for ∆G under non-standard conditions (hint: related to equilibrium constant K)?

∆G = ∆Gº + RT ln(Q)

∆G = non-standard Gibbs free energy (J / mol)

∆Gº = standard Gibbs free energy (J / mol)

R = ideal gas constant

T = temperature in K

Q = reaction quotient (note: at EQ, Q = K and ∆G = 0)

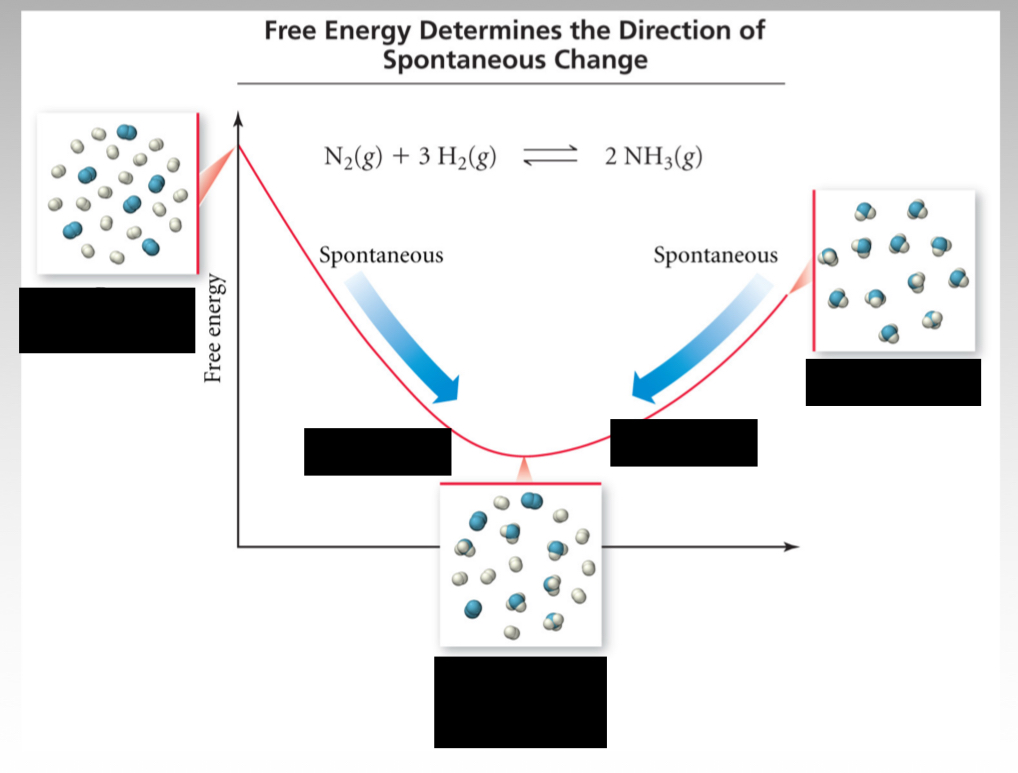
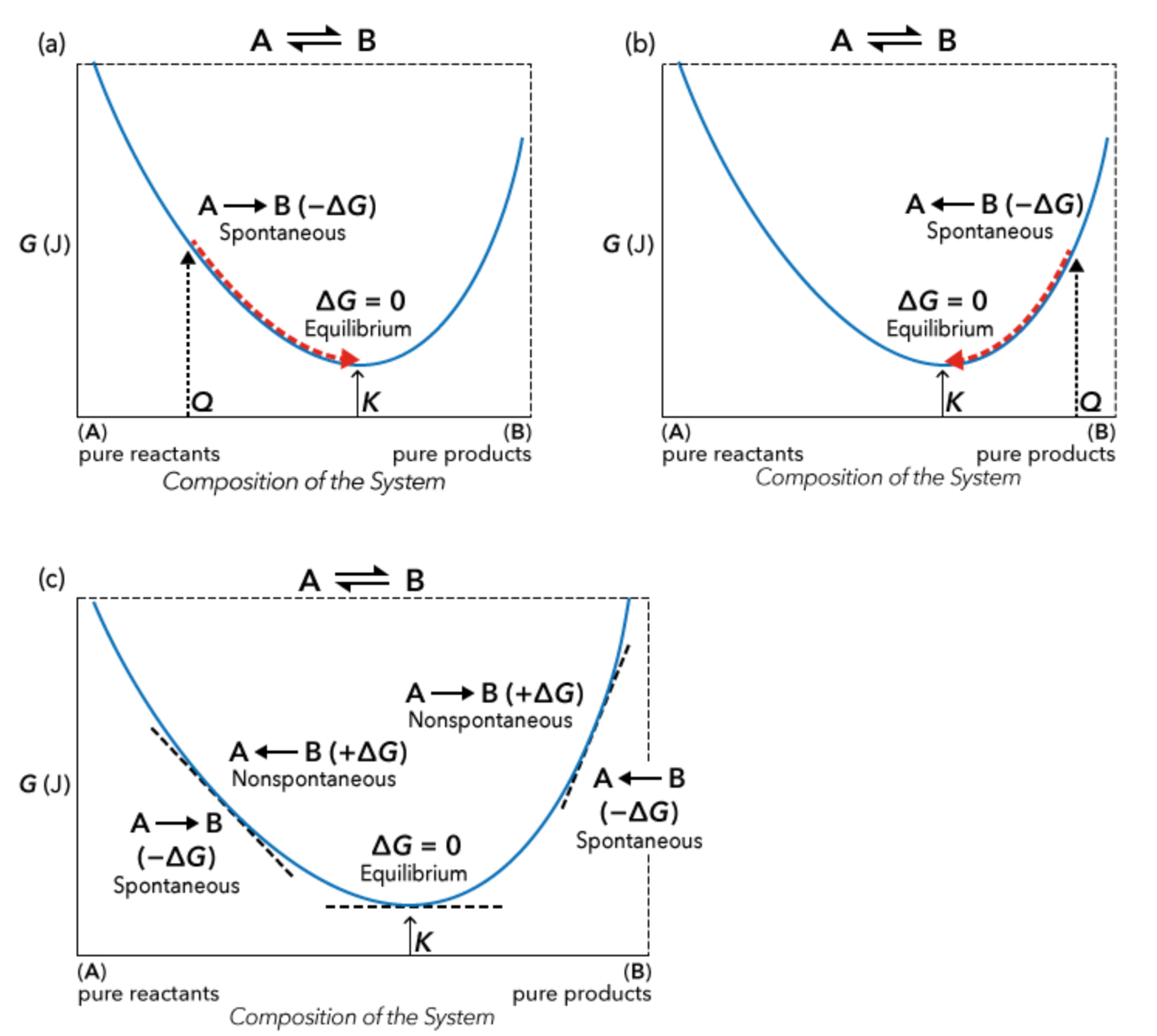
At equilibrium, what is the relationship between standard Gibbs free energy and the equilibrium constant?

At equilibrium, ∆G = 0, so ∆G = ∆Gº + RTln(K) → ∆Gº = -RTln(K)

What is the significance of ∆Gº = 0?

The reaction is at equilibrium under standard conditions. This means that the concentrations of all reactants and products = 1 M (or 1 atm for gas-phase reactions).

Problem-solving: relating Gibbs free energy, the equilibrium constant (K), and the reaction quotient (Q)

• Use the sign on ΔG to determine spontaneity and the relative value of Q vs K

• Use the value of Q to calculate ΔG (or vice versa)

• Use the sign on ΔG° to determine the relative magnitude of K

• Use the value of K to calculate ΔG° (or vice versa)