Physics- Topic 23 ( Nuclear Physics)

Energy and Mass Equivalence

E = mc² where E= energy, m= mass and c = speed of light

Representing simple nuclear reactions

^A_ZX where A: nucleon number/mass number, Z= atomic number/ proton number and X= chemical symbol of the element

Mass defect

The difference between an atom’s mass and the sum of the masses of its protons and neutrons

∆m = Z_mp + (A-Z) m_n - m_total

where:

• Z = proton number

• A = nucleon number

• M_p= mass of a proton

• M_n = mass of a neutron

• M_total = measured mass of a nucleus

Binding energy

The energy required to break a nucleus into it’s constituent protons and neutrons

Energy released in nuclear reaction

E = c²m

The formation of a nucleus from a system of isolated protons and neutrons is therefore an exothermic reaction

Binding energy per nucleon

The binding energy of a nucleus divided by the number of nucleons in their nucleus

A higher binding energy per nucleon indicates a higher stability

Graph of number of nucleons vs the binding energy per nucleon

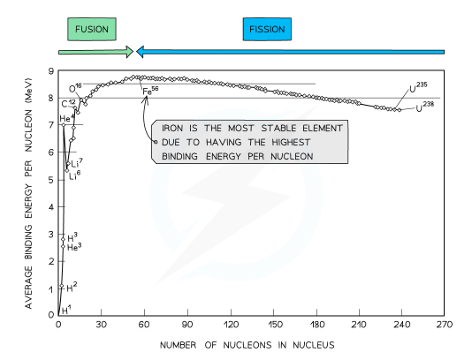
• At high values of nucleon number

  • The general binding energy per nucleon is high & gradually decreases with nucleon number

  • This means the heaviest elements are volatile and undergo fission

• At low values of nucleon number

  • The nuclei tend to have a lower binding energy per nucleon, hence they are generally less stable

  • This means thatthe lightest elements have weaker electrostatic forces and most likely undergo fusion

NOTE: Oxygen, Carbon, and Helium do not fit the trend

Nuclear fusion

Fusion is the fusing together of two small nuclei to produce a large nuclei nucleus

For two nuclei to fuse, both nuclei must have high kinetic energy

• This is because protons repel each other

Eg: Deutrium + tritium → Helium + energy

Nuclear fission

Fission is the splitting of large atomic nuclei into smaller nuclei

• Fission must be induced by firing neutrons at a nucleus

• When the nucleus is struck by a neutron, it splits into 2 or more daughter nuclei and ejects neutrons which can react with another nuclei causing a cascade effect

  • This reaction needs to be controlled otherwise it can cause a nuclear bomb effect

Significance of binding energy per nucleon

• At Lower values of A

  • Attractive nuclear forces between nucleons outweigh repulsive electrostatic forces between protondprotons

  • In the right conditions, nuclei undergo fusion

    • In fusion, the mass of nucleus that is created is slightly less than the total mass of the original nuclei

    • The mass defect is equal to the binding energy released since the nucleus formed is more stable

• At higher values of A

  • Repulsive electrostatic forces begin to dominate, and these forces tend to break apart the nucleus rather than hold together

  • In the right conditions, nuclei will undergo fission

    • In fission, an unstable nucleus is converted to a more stable nuclei with a smaller total mass

    • Mass defect = binding energy

Radioactive decay

Is spontaneous disintegration of a nucleus to form a more stable nucleus, resulting in the emission of an alpha, beta or gamma particle

Evidence for the random nature of radioactive decay

This can be observed with the count rate of a Geiger-Muller(GM) tube

When a GM tube is placed near a radioactive source, the counts are found to be irregular and can’t be perdicted

Each count represents a decay of an unstable nucleus

These fluctuations in count rate on the GM tube provide evidence for the randomness of radioactive decay

Characteristics of radioactive decay

• Spontaneous: A process which can not be influenced by environmental factors

• Random: A process in which the exact time of decay of a nucleus can not be predicted

Decay constant

Is the probability that an individual nucleus will decay per unit time

Average number of nuclei which are expected to decay per unit time

Activity

Is the rate at which radioactive decay occurs

A = ΔN/ Δt = -λN

Where:

• A = activity

• λ = decay constant

• ΔN = number of undecayed nuclei

• Δt = time interval

• N = number of nuclei remaining in a sample

The minus sign shows that the number of undecayed nuclei decreases over time

Half-life

Is the time taken for the initial number of nuclei to reduce by half

This means when a time equal to half-life has passed, the activity of the sample will also have decreased by half.

A ∝ N

λ = 0.693 / t_1/2

Exponential nature of radioactive decay

In radioactive decay, the number of nuclei falls very rapidly without ever reaching zero

• Such a model is known as exponential decay

The steeper the graph is, the larger the decay constant

General Equation

X = X₀e^-λt where X can be activity, number of undecayed nuclei or received count rate