Complex Numbers and Vectors

What is the gradient of a line?

The gradient of a line or the slope or steepness of a line is the rise over run or change in y over the change in x on the x-y coordinate plane.

_____________and _________ have neither a positive of negative slope. These lines have a slope of zero.

Flat and Tangental

The gradient of tangent vertical lines is ___________________.

undefined

What is the equation of a line?

The equation of a line is a mathematical expression that describes all the points that lie on a straight line in a coordinate plane.

Parallel lines have the same _______________ but different y - intercepts.

Gradient

Perpendicular lines have _______________ that are the negative reciprocal of each other.

gradients

What is the slope intercept form?

What is the point slope form?

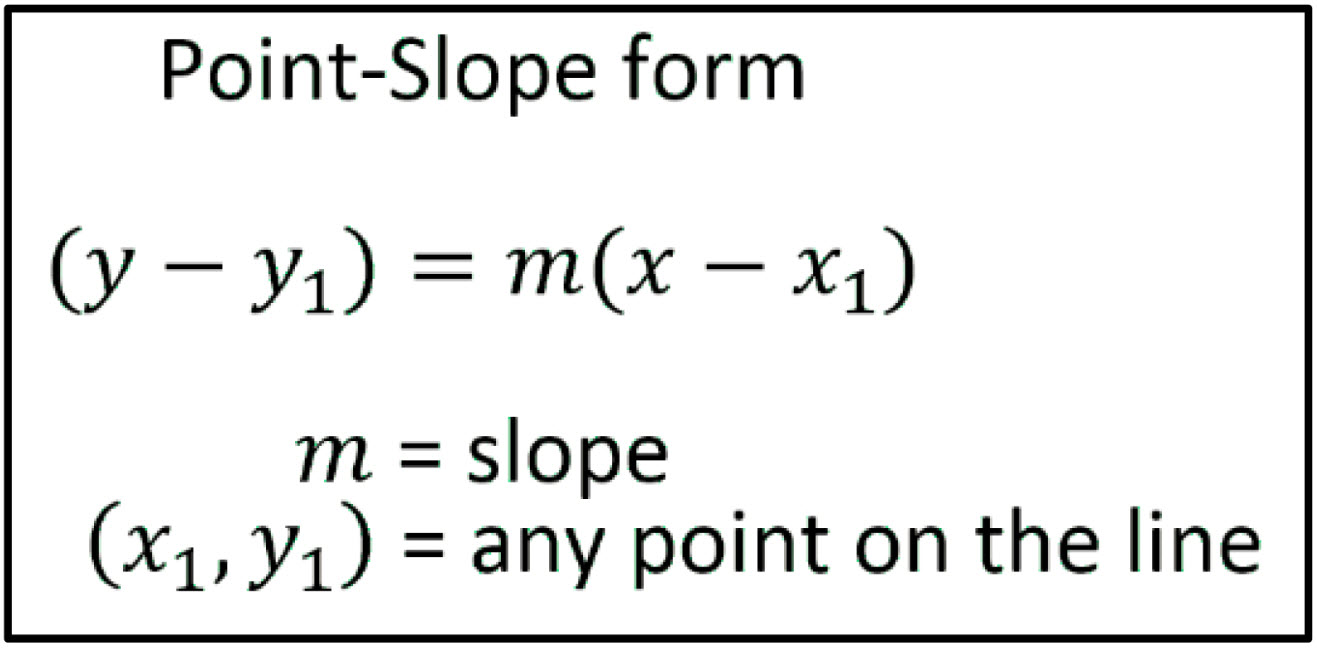
What are oblique lines?

Lines that are neither horizontal nor vertical but are slanted at an angle. In other words, they have a non- zero slope.

How do you calculate the x - intercept using the slope intercept form.

Substitute the y for zero and solve for x

What is the standard form of a linear equation?

When you multiply the reciprocal of perpendicular lines the product is?

-1 ( except for horizontal perpendicular lines)

What is a vector quantity?

quantity that has magnitude and direction

What are collinear vectors?

Collinear vectors are vectors that lie along the same line or along parallel lines. This means that one vector is a scalar multiple of the other.

• Two or more vectors are proportional or related to each other by a scalar. These points are on the same line.

What are properties of collinear vectors?

1. Collinear vectors are always parallel to each other

2. If k > 0, the vectors have the same direction. ( K is the scalar)

3. If k < 0, the vectors have opposite directions.

What is the Greek letter used to represent a scalar?

lambda

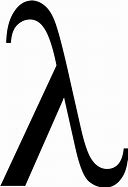
What is the difference between a free vector and a position vector?

A free vector is a vector that is not fixed at a specific location and can be moved anywhere while keeping the same magnitude and direction. A position vector is at the origin and points to a specific location in space. Free vectors are represented by v and position vectors are represented by r.

What does it mean when lines converge?

Lines converge when they get closer to each other as they extend in a certain direction. This means that at some point, they will eventually intersect (meet).

What are properties of Converging lines?

• They move toward a common point of intersection.

• The angle between them decreases as they extend.

• If they meet at a single point, they are not parallel.

What does it mean when lines diverge?

Lines diverge when they move away from each other as they extend. This means they will never meet and will continue spreading apart.

What are properties of diverging lines?

• The angle between them increases as they extend.

• They do not have a common point of intersection (unless extended backward).

• If they are straight and never meet, they are simply diverging non-parallel lines.