PARABOLA/ELLIPSE/HYPERBOLA

Ellipse

A locus of points (x,y) such that the sum of the distances from two fixed points (foci) is constant.

Foci

Two fixed points in an ellipse used to define its shape.

Major Axis

The longer axis of an ellipse.

Minor Axis

The shorter axis of an ellipse.

Vertices

Endpoints of the major axis of an ellipse.

Co-vertices

Endpoints of the minor axis of an ellipse.

Center of an ellipse

The midpoint of both the major and minor axes.

Eccentricity (e)

A measure of how much an ellipse deviates from being circular, calculated as e = c/a.

Standard form of an ellipse

The equation format for an ellipse, which varies depending on the orientation and center of the ellipse.

Constant sum

The sum of the distances from the foci to any point on the ellipse, which remains constant.

Latus Rectum

A line segment perpendicular to the major axis that passes through a focus and whose endpoints lie on the ellipse.

Equation of an ellipse

A mathematical representation of an ellipse in coordinate form, often represented as (x^2/a^2) + (y^2/b^2) = 1.

Locus of an ellipse

The set of points that form the shape of an ellipse based on its definition.

Distance from center to foci (c)

The distance from the center of an ellipse to each of its foci; c is calculated via the relationship c^2 = a^2 - b^2.

Length of Major Axis

Twice the value of 'a', expressed as 2a.

Length of Minor Axis

Twice the value of 'b', expressed as 2b.

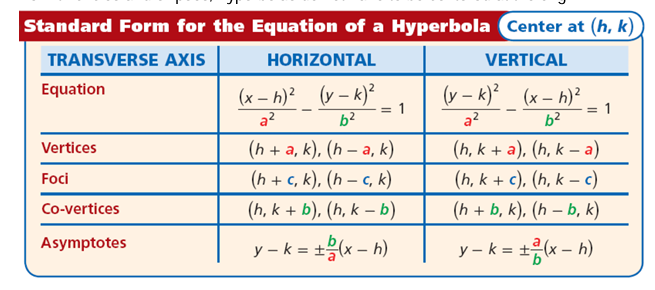
hyperbola

is a set of points P(x, y) in a plane such that the difference of the distances from P to fixed points F1 and F2, the foci, is constant.

c^2 = a^2 + b^2.

The values a, b, and c in hyperbola are related by the equation

Standard form for Hyperbola

Parabola

is the set of all points in a plane that are equidistant from a fixed point and

fixed line of the plane.

Standard form for Parabola opening to the Horizontally

(y – k)^2 = 4a(x – h)

Standard form for Parabola opening vertically

(x – h)^2 = 4a(y – k)

Parabola length of latus rectum

|4a| (absolute value of 4a)