Fluids Part 1

Inviscid flow

Flow where the following assumptions lie:

• No friction

• No thermal conduction

• No diffusion

Essentially viscous effects are neglected

High speed external flows where inertial forces dominate over viscous forces.

Far from boundaries where shear layers have minimal influence

Used In simplifying fluid dynamics problems (eg Bernoullis eq)

Assumes no shear stress, hence no viscous energy loss

inertial energy losses are large or when flow is external to a body (as viscous effects are pronounced at
boundaries in particular)

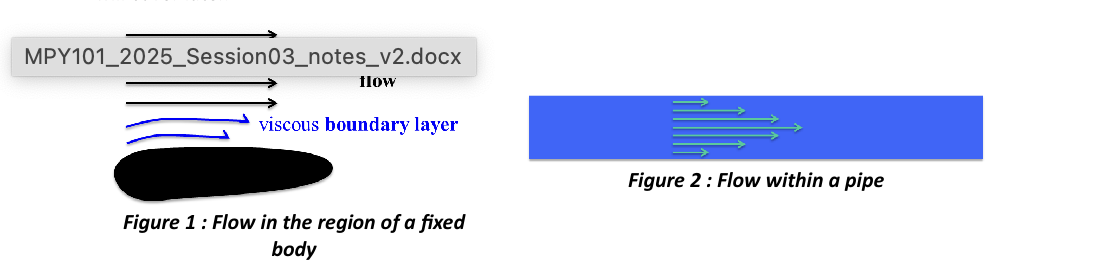
Viscous flow

Flow where the following assumptions lie:

• Friction

• Thermal conduction

• Diffusion

Velocity gradients exist within the flow field

Viscous stresses affect energy dissipation

No slip condition: Fluid velocity at a solid boundary is zero

Steady Flow

The velocity, pressure and other flow parameters at any given location in the fluid remain constant with respect to time. This means that although the individual fluid particles move through space, the overall pattern of flow does not vary. Mathematically ∂u​ / ∂t=0..Steady: all time derivatives are zero.

• If the flow is steady:

  • Streamlines = Pathlines = Streaklines (all the same)\

    Steady inlet velocity (VinVin​) ➔ constant.

• Steady outlet velocity (VoutVout​) ➔ constant.

• Flow goes smoothly around the cylinder, no weird behavior.

• Streamlines are clean and don't move with time.

Transient Flow

✅ Definition:
A transient flow is a fluid flow where the velocity, pressure, or other properties change with time at any given location.

✅ Key Characteristics:

• Time-dependent behavior:
  ∂∂t≠0∂t∂​=0 (time derivatives are NOT zero)

• Velocity field changes over time.

• Streamlines vary with time — they are not fixed.

• Pathlines (particle history) and streamlines (instantaneous flow lines) are different.

✅ Common causes:

• Sudden changes in boundary conditions (e.g., valves opening or closing).

• Instabilities in the flow (e.g., vortex shedding behind objects like cylinders).

• Pulsating inlets or outlets (e.g., heartbeat in blood flow).

✅ Examples:

• Flow around a cylinder creating alternating vortices (vortex shedding).

• Blood flow during heartbeat cycles.

• Wind gusts affecting smoke trails.

✅ Important Equations:

• Navier-Stokes equations include time-dependent terms when analyzing transient flow.

• Must solve unsteady (time-dependent) versions of fluid equations.

✅ In contrast to steady flow:

Steady Flow	Transient Flow
No change with time	Changes with time
Streamlines = Pathlines	Streamlines ≠ Pathlines

✅ Extra Tip:
In transient flow, the history of the particle matters, because the forces acting on it vary over time.

Streamlines

✅ Streamlines:

• Definition:
  Lines that show the instantaneous direction of the velocity field at a single moment in time.

• Properties:

  • Always tangent to the local velocity vector.

  • Snapshot view of the flow.

  • In steady flow, streamlines are fixed.
    In transient flow, streamlines change over time.

Streamlines = Instant

Pathlines

✅ Pathlines:

• Definition:
  The actual path a particle follows as it moves through the flow field over time.

• Properties:

  • History of a fluid particle.

  • Takes into account changes in velocity with time.

  • Always tied to the motion of a particular particle.

Pathlines = Journey

Compressible v Incompressible and Mach number

✅ Incompressible Flow:

• Definition:
  The density ρρ of the fluid does not change significantly with time or position.

• Mathematically:

  dρdt=0dtdρ​=0

• ✅ Assumption: fluid elements do not expand or shrink as they move.

• ✅ This makes fluid equations much simpler!

✅ Compressible Flow:

• Definition:
  The density ρρ changes significantly due to changes in pressure or temperature.

• You cannot ignore compressibility effects.

• Typically happens at very high speeds (e.g., airflows around jets, explosions).

📚 How do we know if a flow is compressible?

We use the Mach Number (MaMa):

Ma=ua

where:

• uu = flow velocity relative to the medium

• aa = speed of sound in the medium

✅ Mach number = how fast the flow is compared to the speed of sound.

📚 Mach Number Ranges:

Mach Number	Flow Type
Ma<0.3	Incompressible (density changes negligible)
0.8<Ma<1.20.8<Ma<1.2	Transonic (partly compressible)
1.2<Ma<3.01.2<Ma<3.0	Supersonic (strong compressibility)
Ma>3.0Ma>3.0	Hypersonic (extreme compressibility)

✅ If Ma<0.3Ma<0.3 ➔ safe to assume incompressible.

---

📚 Cardiovascular System Context:

• In blood flow and water-based systems,

• Speed of sound in water ≈ 1,484 m/s,

• Blood flow speed is only about 1–2 m/s in arteries.

Thus:

Ma=21484≈0.0013Ma=14842​≈0.0013

✅ Way less than 0.3 ➔ blood flow is incompressible!

---

🎯 Final Quick Summary:

Property	Incompressible	Compressible
Density ρρ	Constant	Varies
Mach Number MaMa	<0.3<0.3	>0.3>0.3
Cardiovascular blood flow	Incompressible (small MaMa)