General Relativity

General Relativity Theory

Einstein's theory of gravity (accelerated motion)

Especially important for fast-moving objects and very dense objects

Proposed by Einstein in 1915

Predictions of General Relativity

1. Space and time are not independent but form a single entity called "spacetime".
2. Orbits of masses follow shortest pathways (geodesics) in spacetime.
3. Gravity is a geometrical distortion of spacetime, explains why inertial mass (m in F = ma) same as gravitational mass (m in F = GmM/r2 ).
4. Time slows down when a clock is in a gravitational field.
5. Additional precession of elliptical orbits (Mercury's orbit)
6. Black holes can exist & have a singularity point in their interiors.
7. Accelerating masses generate gravitational waves that propagate at speed of light.

Spacetime

Special relativity showed that space and time are not absolute nor separable

Space and time but form a single entity called spacetime
• 4-dimensional

Gravity is a distortion of spacetime

Travelers going in opposite directions in straight lines will eventually meet
Because they meet, the travelers know earth's surface cannot be flat - it must be curved.

Gravity can cause two space probes moving around Earth to meet.

General relativity says this happens because spacetime is curved.

Einstein's theory of gravity

Masses can reshape distort the fabric of spacetime, much like weights on a rubber sheet
Small mass produces a small divet in the space around it
Marble will orbit the divet
Gravity is a weak force; you need a lot of mass
He turned gravity into a distortion of the space

Curved space
• Gravity can cause two space probes moving around the earth to meet
• Masses can re-shape (distort) the "fabric" of spaceitme, much like weights on a rubber sheet

Motion is considered relative but only for no-accelerating reference frames

Equivalence principle

The effects of gravity are exactly equivalent to the effects of acceleration

Gravity and acceleration are the same thing in effect

Gravity - accelerating reference frame
• Uniting graviy and acceleration

A closed room accelerating through space

You cannot tell the difference between gravity and an accelerating reference frame

Additional precession of elliptical orbits - explains Mercury's orbits
There's no planet Vulcan
Black holes can exist and have a singularity point in their interiors
• All mass is essentially at a point
Accelerating masses generate gravitational waves that propagate at speed of light

Einstein's equivalence principle depends on

A sufficiently small region of space

Perspectives in space

• A book has a definite three-dimensional shape
• But the book looks different in two-dimensional pictures of the book taken from different perspectives
• Similarly, space and time look different from different perspectives in spacetime

Perspectives in spacetime

• Observers in relative motion do not
share the same definitions of x, y, z,
and t, taken individually

Space is different for different observers.
Time is different for different observers.
but Spacetime is the same for everyone.

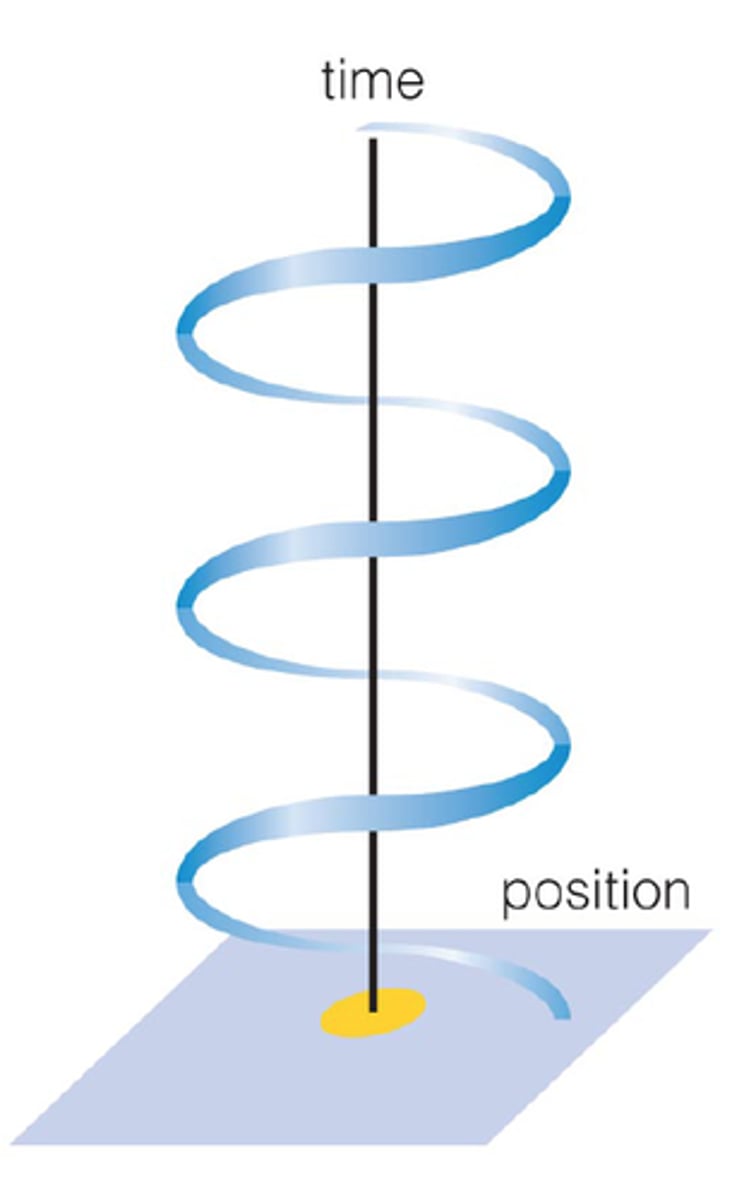
Spacetime diagram of a car

Plots an object's position in space at different moments in time

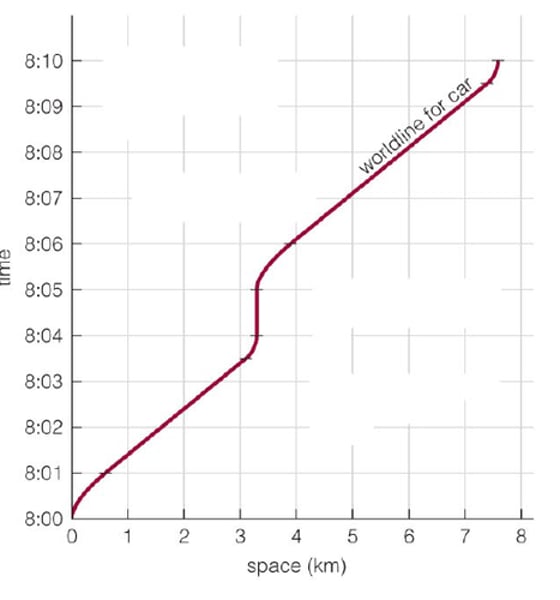
Wordline

• A worldline shows an
object's path through space-time in a spacetime diagram
- Vertical worldline: no motion
- Diagonal worldline: constant-velocity motion
- Curved wordline: accelerating motion

Worldlines for light

• Worldlines for light go at 45° angles in diagrams with light-seconds on one axis and seconds on the other

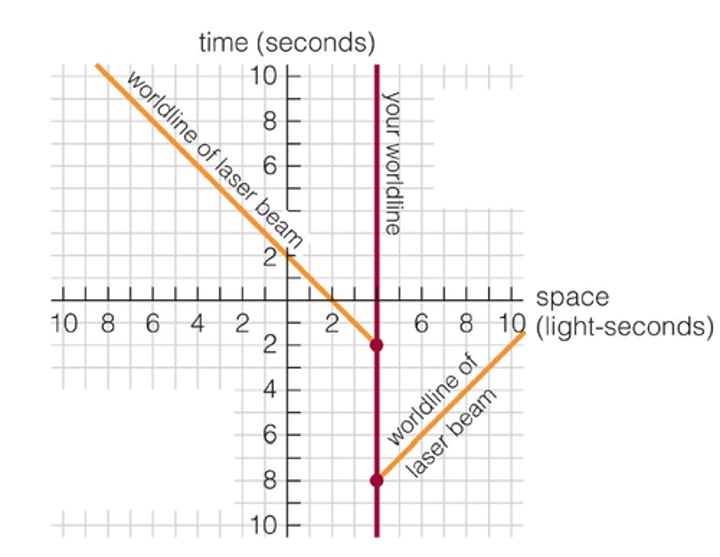
Worldlines and relativity

Look different in different reference frames

Distance between events in space time

Constant
x- and y- coordinates of two points can be different in different coordinate systems
But the distance between them (red line) is the same

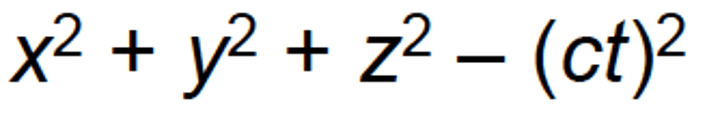
Geometry on curved surface

• The straightest
lines on a sphere
are great circles
sharing the same
center as the
sphere
• Great circles
intersect, unlike
parallel lines in flat
space
• Straight lines are
shortest paths
between two points
in flat space
• Great circles are
the shortest paths
between two points
on a sphere

Rules of spherical geometry

• Great circle is
shortest distance
between two points
• Parallel lines
eventually
converge
• Angles of a triangle
sum to > 180°
• Circumference of
circle is < 2πr

Rules of saddle shaped geometry

• Piece of hyperbola
is shortest distance
between two points
• Parallel lines
diverge
• Angles of a triangle
sum to < 180°
• Circumference of
circle is > 2πr

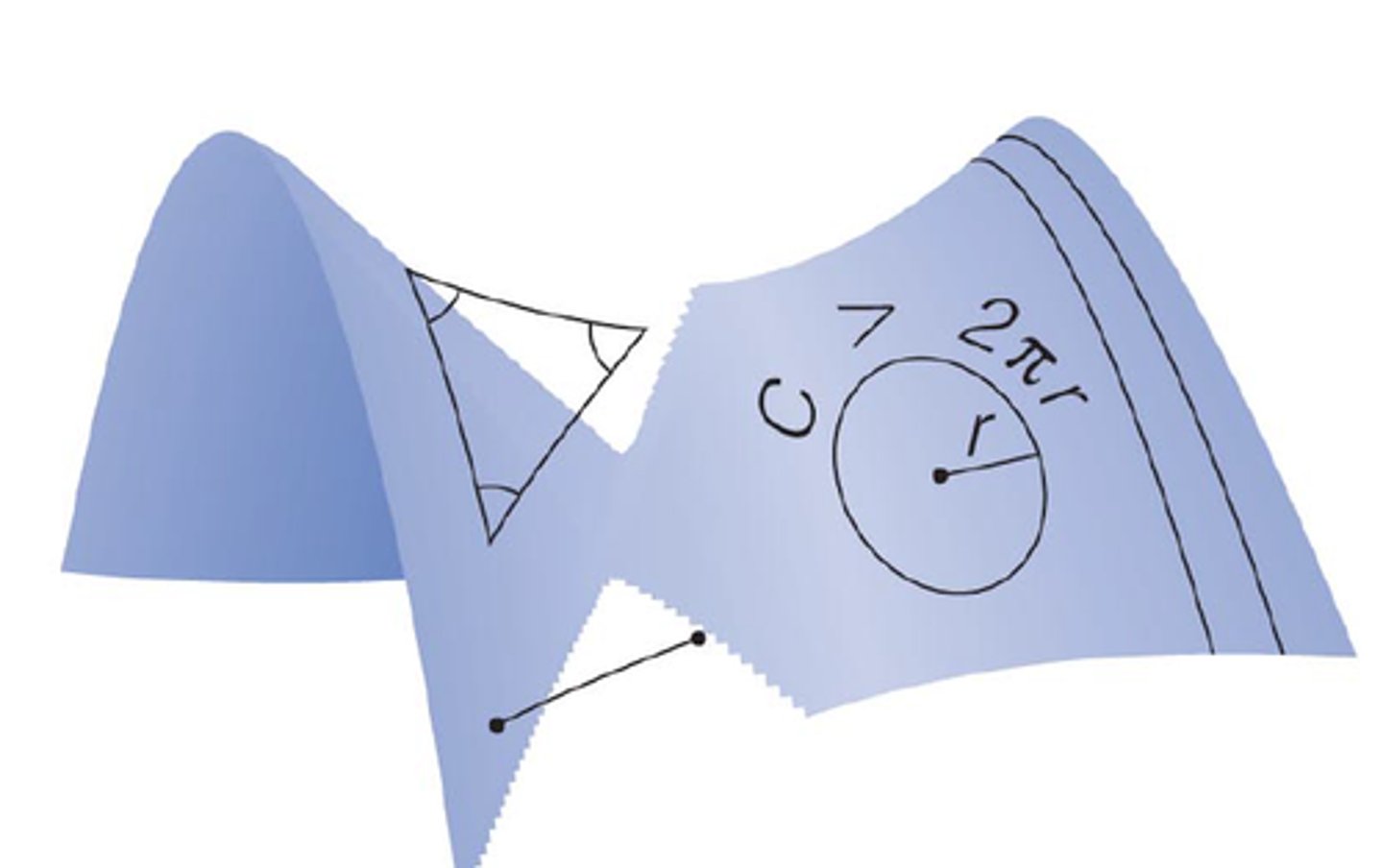
Difference between flat, saddle shaped, and spherical universses

The Universe may be either flat, spherical,
or saddle-shaped depending on how
much matter (and energy) it contains.
- Flat and saddle-shaped universe are infinite in extent
- Spherical universe is finite in extent

No center and no edge to the universe is
necessary in any of these cases

How did Newton view gravity?

Action at a distance

How did Einstein view gravity?

removed the mystery by showing
that what we perceive as gravity arises
from the curvature of spacetime

Why does the apple fall, according to A, N, and E?

Aristotle: It's seeking its natural place
toward the center of the universe.
Newton: The earth exerts a force,
pulling the apple downward.
Einstein: It's moving along the
straightest possible path through curved
space-time.

Space time is curved

Because it is distorted by the earth's mass

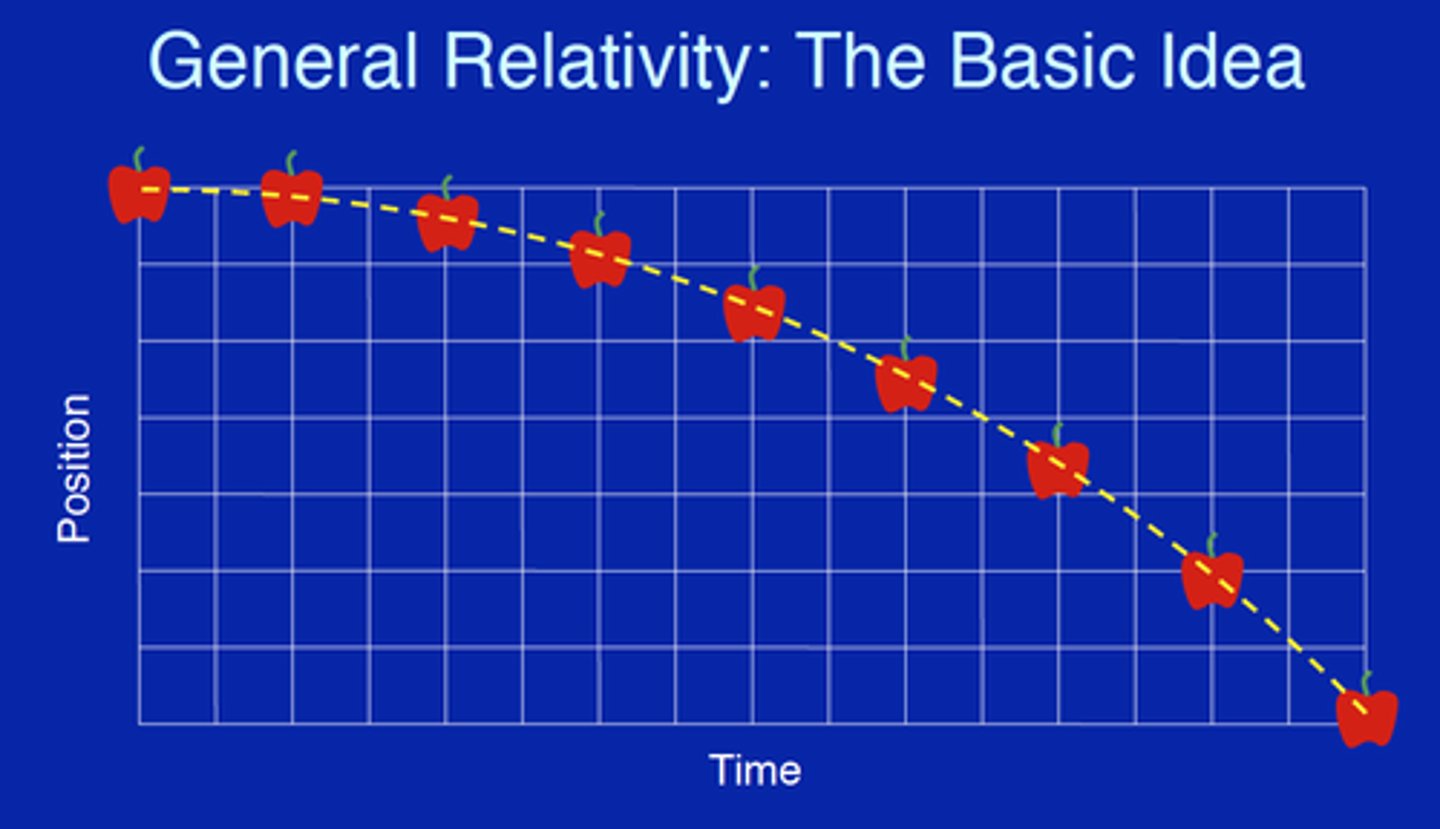
Trajectories are a property of ...

space time, not the object

General relativity in a nutshell

Matter tells spacetime how to curve.
Spacetime tells matter how to move.

Rubber sheet with sun in the middle

QUESTION Look this up in text book

Free-falling objects follow curved paths - why?
Circles have circumference less than 2(pi)r

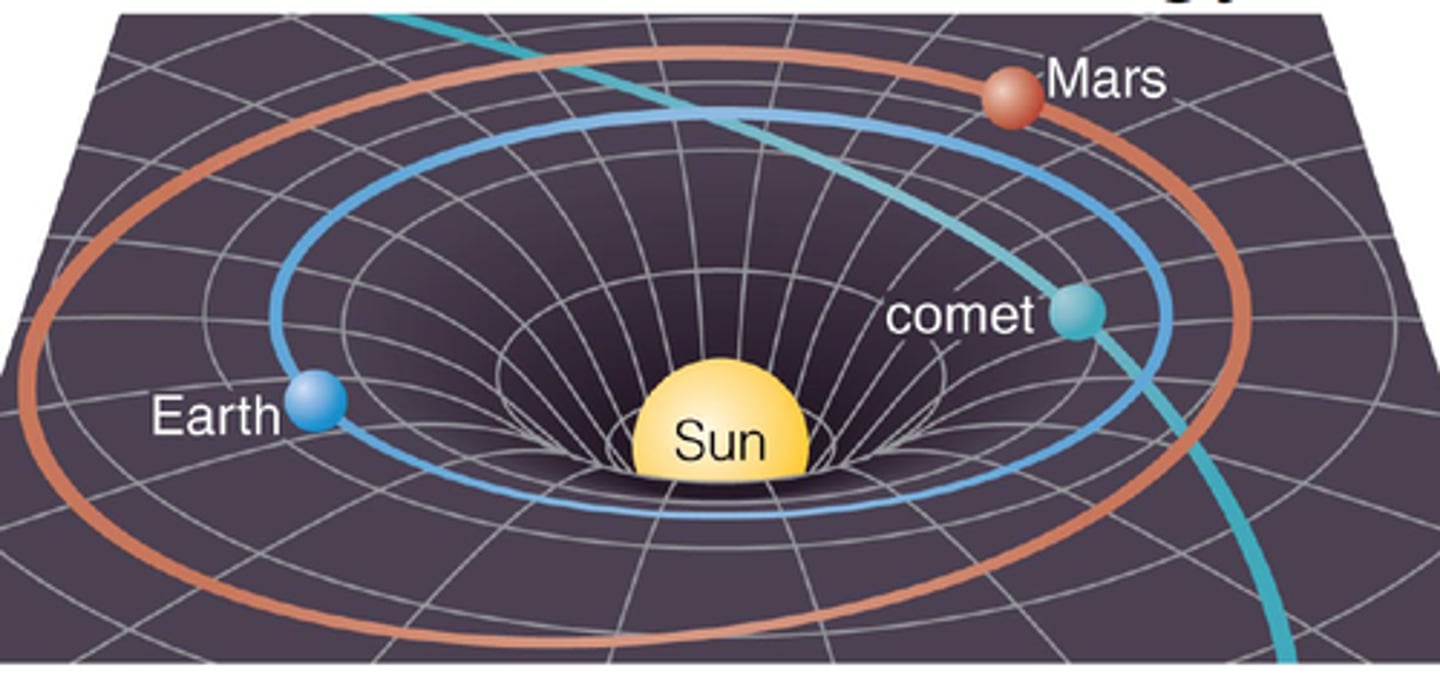
Limitations of rubber sheet analogy

• Masses do not rest "upon" the spacetime
like they rest on a rubber sheet
• Rubber sheet shows only two dimensions
of space (not time?)

QUESTION Look this up in textbook

Curvature near the sun

QUESTION: What does it mean that the sun's mass curves space-time near its surface?

If we could shrink the Sun without changing its
mass, curvature of spacetime would become greater
near its surface, as would the strength of gravity

Effect of gravity on clocks. Explain the Thought Experiment.

Time slows down in gravity field

Imagine you and Jackie are in the opposite ends of a spaceship that is floating in space.
Both you and her have watches that flash brightly each second.
And both see each other's watches flash at the same rate.
Now...suppose the spaceship engines fire and it begins to accelerate.

Because you are in the front of the spaceship, your frame of reference is carrying you away from Jackie and the flashes from her watch.
Thus, the light flashes from her watch will take a little longer to reach you than it would if the spaceship were not accelerating.
So you'll see her watch flashes more than 1 second apart.

From Jackie's point of view at the back of the accelerating spaceship, her frame of reference is carrying her toward you and the flashes from your watch.

Thus, the light flashes from your watch will take a little less time to reach her, so she sees them faster than 1 second apart.

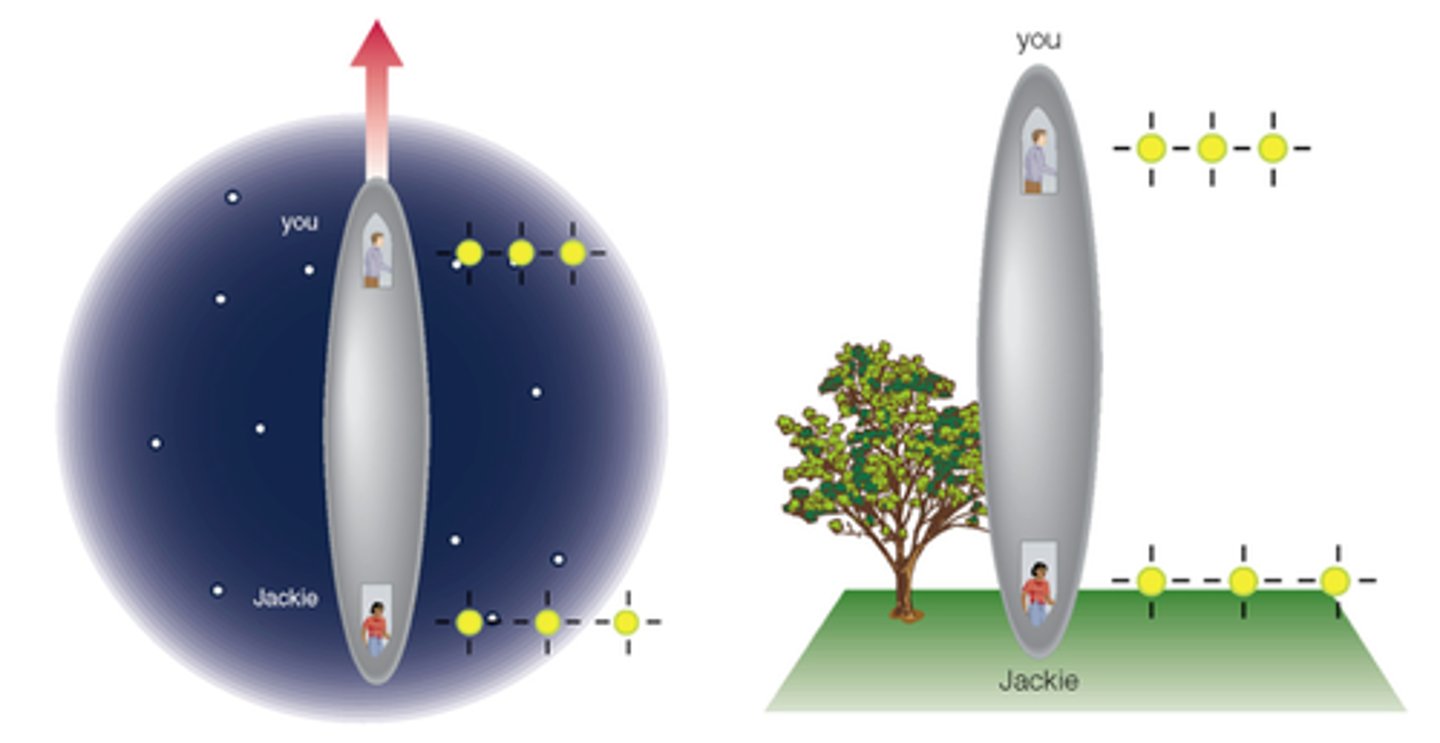
Time in an accelerating ship

• Light pulses travel
more quickly from
front to back of an
accelerating
spaceship than in
other direction
• Everyone on ship
agrees that time
runs faster in front
than in back

• Effects of gravity
are exactly
equivalent to those
of acceleration
• Time must run
more quickly at
higher altitudes in a
gravitational field
than at lower
altitudes

GPS - how accurate are they?

• GPS systems provide
positions accurate to 5 or 10
meters in real time.

How does GPS work?

• Its a network of 24 satellites in high orbits around the Earth. Each satellite in the GPS
constellation orbits at an altitude of about 20,000 km from the ground, and has an
orbital speed of about 14,000 km/hour (the orbital period is roughly 12 hours).
• The satellite orbits are distributed so that at least 4 satellites are always visible from
any point on the Earth at any given instant (with up to 12 visible at one time). Each
satellite carries with it an atomic clock that "ticks" with an accuracy of 1 nanosecond
(1 billionth of a second). A GPS receiver determines its current position and heading
by comparing the time signals it receives from a number of the GPS satellites.
(usually 6 to 12) and triangulating on the known positions of each satellite.

How does relativity correct GPS?

• To get positions accurate to 10 meters, the GPS clock ticks must be
known to an accuracy of 30 nanoseconds (3 x 10-8 sec) (d = ct).
• Satellites are moving at a velocity of 14,000 km/hour; special
relativity predicts that the satellite clocks will fall behind ground based
clocks at a rate of 7 microseconds/day (7 x 10-6 sec/day).
• Satellites are farther from the Earth, and so in a weaker gravitational
field than the ground based clocks; general relativity predicts that this
will cause the satellite based clocks to gain 45 microseconds/day
(4.5 x 10-5 sec/day) compared to the ground based clocks.
• Net effect is satellite clock should tick faster than identical clocks on
the ground by about 38 microseconds per day (45 - 7 =38)! This
sounds small, but the high-precision required of the GPS system
requires nanosecond accuracy, and 38 microseconds is 38,000
nanoseconds.
• If these effects were not properly taken into account, a navigational
fix based on the GPS constellation would be false after only 2
minutes, and errors in global positions would continue to accumulate
at a rate of about 10 kilometers each day!
• Next time you use a GPS system, you can thank Albert Einstein.

Mercury's elliptical orbit

Small part of the precession = due to relativistic corrections to Newtonian gravity (43 arc seconds per century)

Most of it is due to gravitational pull of other planets

Inverse-square force isn't exact; minor corrections to solar system trajectories

Arthur Eddington

Found that gravitational deflection of light was twice the amount that Newton would have predicted

QUESTION - look up 1919 eclipse in textbook

An unusual number of bright stars from the Hyades cluster conveniently filled
the sky on all sides of the Sun during the 1919 eclipse. In this artist's
rendering, deflection of starlight from the Sun's gravity shifts the original
stellar positions (blue) radially away from the center of the Sun; those closest
to the center suffer the greatest shift.

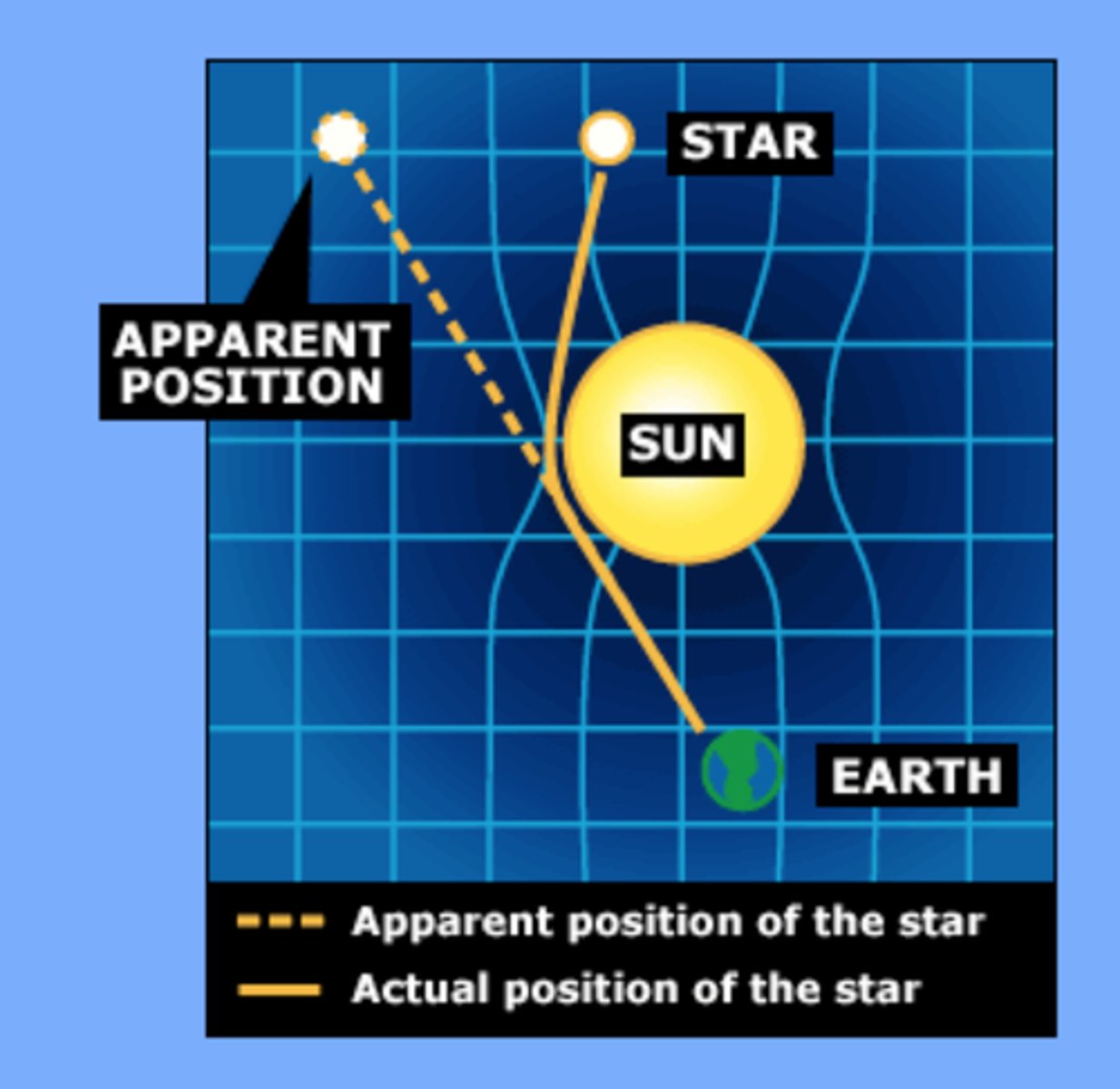
Three different possible mathematical paths for a pulse of light following around the sun

the path with no
gravity, the path as
predicted by
Newtonian gravity,
and the path as
predicted by
Einstein's General
Theory of Relativity.

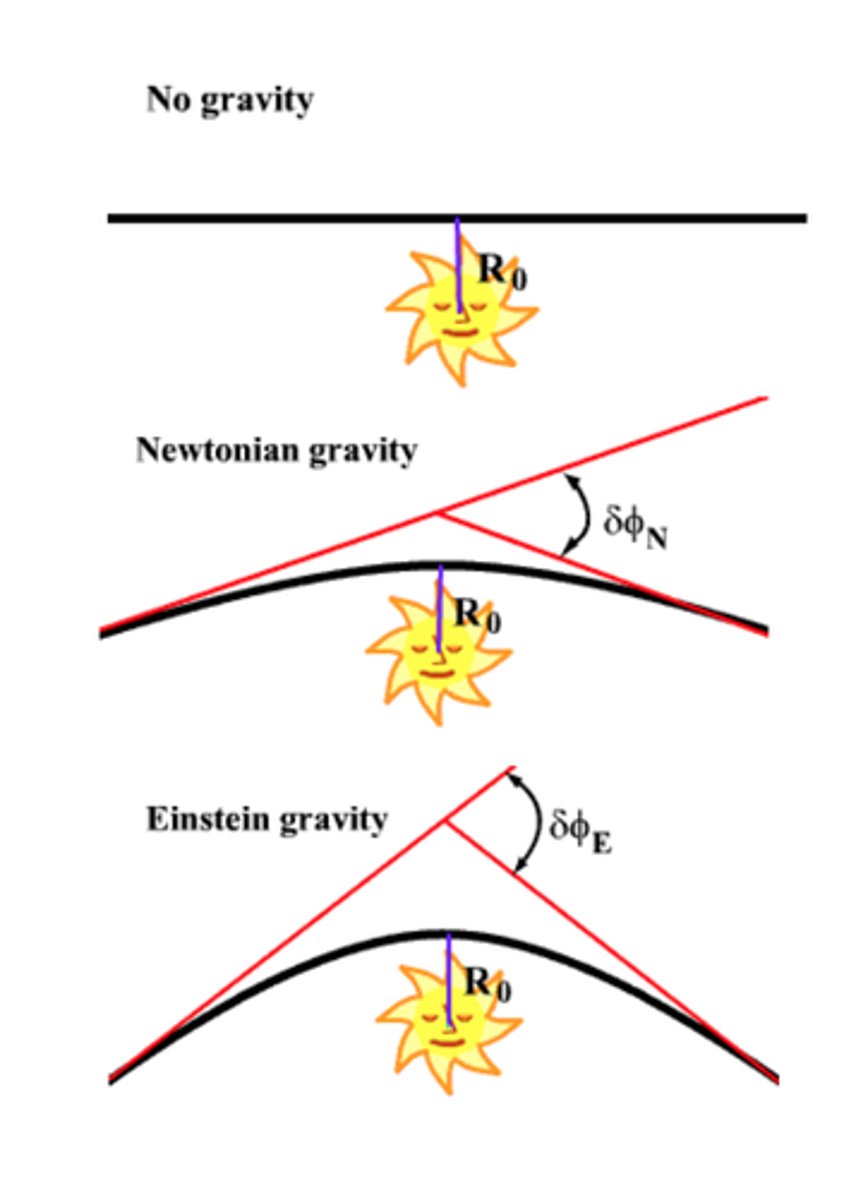
What did Eddington see on May 29, 1919?

measured stars that appeared near the
Sun and found that their positions were shifted slightly, just
as Einstein's equations predicted.

infinite warp

If mass warps space-time, enough mass packed into a small space could create an infinite warp.

In other words, it would warp space so much that nothing could escape from it, including light, creating what would come to be known as a black hole.

Black holes

created by objects so dense that not even light can escape

A consequence of general relativity

spherical "horizion" surrounds the region through which nothing can escape

What is the radius of the horizon (of the black hole) proportional to? Give examples.

The black hole's mass

Surface area is 4(pi)r^2

• For a solar-mass black hole, the horizon radius is 3 km
(only a few times smaller than a neutron star)!
• Neutron stars, like white dwarfs, shrink with increasing
mass; maximum mass is 2-3 solar masses!
• So a collapsing stellar core more massive than this
should form a black hole!

How can one detect a black hole?

• Only through its gravitational pull on other objects!!
• An isolated black hole would be virtually impossible to detect.
• In a close binary system, a black hole can suck gas off the
companion star...as the gas spirals inward it becomes extremely
hot and emits x-rays!

Black holes are at the center of galaxies

What is an example of a black hole that we have detected?

• Most famous example: Cygnus X-1. Binary system (8000 ly
away) of a blue supergiant star and a compact object, both 20-30 solar masses--too massive for a neutron star.