Final Exam

Mostly population genetics and Hardy-Weinburg equations

Motoo Kimura

• 1968

• proposed Neutral Theory

Neutral Theory

there is a natural mutation rate leading to changes in alleles

some alleles will get selected again while others may drift in frequency due to random genetic drift. It emphasizes that many genetic variations are not driven by natural selection but occur by chance.

How are Hardy-Weinberg equations predicted?

predicts how alleles flow/are maintained in a population

what does p equal?

the frequency of the 1st allele in a population

what does q equal?

the frequency of the 2nd allele in a population

what is the first rule of Hardy-Weinburg equations?

p + q = 1.0

Represents allele frequencies

what is the second rule of Hardy-Weinburg equations?

The genotype frequencies can be calculated using p² + 2pq + q² = 1.

conditions that need to be met in a Hardy-Weiberg equation

1. the population must be infinitely large

2. must be random mating

3. population isn’t subject to evolutionary forces

if p = 0.7 and q = 0.3 in a population, what is the probability that you will have a homozygous disease (aa)?

To find the probability of being homozygous recessive (aa), use the formula q². Therefore, if q = 0.3, then q² = 0.3² = 0.09, which means there is a 9% chance that the population is homozygous for this disease

if p = 0.7 and q = 0.3 in a population, what is the probability that you will have a homozygous disease (AA)?

To find the probability of being homozygous dominant (AA), use the formula p². Therefore, if p = 0.7, then p² = 0.7² = 0.49, which means there is a 49% chance that the population is homozygous for this trait.

if p = 0.7 and q = 0.3 in a population, what is the probability that you will have a heterozygous disease (Aa)?

To find the probability of being heterozygous (Aa), use the formula 2pq. Therefore, if p = 0.7 and q = 0.3, then 2pq = 2(0.7)(0.3) = 0.42, which means there is a 42% chance that the population is heterozygous for this trait.

PKU is a rare metabolic disorder and is homozygous recessive. It occurs in 1/14,000 live births.

What percentage of the population is a carrier (assuming homozygous recessive disease)?

(7.14 × 10^-5) 1/14,000 = q² (frequency that is homozygous recessive)

q = √1/14,000 = 0.00845

p = 1 - q = 1 - 0.00845 = 0.992

Heterozygous = 2pq = 2(0.992)(0.00845) = 0.0168

Therefore, there is approximately a 1.68% chance that an individual in the population is a carrier for PKU.

what is another method for figuring out equilibrium regarding p?

p = p² +1/2 (2pq)

p² = whatever AA is

1/2(2pq) = ½ of Aa

what is another method for figuring out equilibrium regarding q?

q = q²+ 1/2(2pq)

q² = frequency of aa

AA = 0.2

Aa = 0.6

aa = 0.2

what is genotype frequency of the population in relation to p?

p = 0.2 + ½ (0.6) = 0.5

half the population is AA (p)

AA = 0.2

Aa = 0.6

aa = 0.2

what is genotype frequency of the population in relation to q?

q = 0.2 + ½ (0.6) = 0.5

half the population is aa (q)

Blood typing

MM = 1101

MN = 1406

NN = 503

What is the allele frequency, and is the population in equilibrium?

1101 + 1406 + 503 = 3100

1101/3100 = 0.355 (p²)

1406/3100 = 0.483 (2pq)

503/3100 = 0.162 (q²)

Allele frequency:

p = 0.355 + ½ (0.483) = 0.597 (M)

q = 0.162 + ½ (0.483) = 0.403 (N)

0.597 + 0.403 = 1

MM = (0.597)² = 0.357

MN = 2(0.597)(0.403) = 0.481

NN = (0.403)² = 0.162

0.357 × 3100 = 1107

0.481 × 3100 = 1491

0.162 × 3100 = 502

Chi square:

(O-e) = -6, 5, 1

(O-e)² = 36, 25, 1

(O-e)² /e = 0.0325, 0.168, 0.002

X² = 0.0513

d.F = 2

0.95 < p < 0.99

Data fits what we expected, the population is in equilibrium

Fish

AA = 80

Aa = 280

aa = 640

Allele frequency? Is the population in equilibrium?

80 + 280 + 640 = 1000

80/1000 = 0.08 (p²)

280/1000 = 0.28 (2pq)

640/1000 = 0.64 (q²)

Allele frequency:

p = 0.08 + ½ (0.28) = 0.22

q = 0.64 + ½ (0.28) = 0.78

0.22 + 0.78 = 1

p² = (0.22)² = 0.0484

2pq = 2(0.22)(0.78) = 0.3432

q² = (0.78)² = 0.6084

0.0484 × 1000 = 48.4

0.3432 × 1000 = 343.2

0.6084 × 1000 = 608.4

Chi square:

(O-e) = 31.6, -63.2, 31.6

(O-e)² = 998.56, 3994.24, 998.56

(O-e)² /e = 20.63, 11.64, 1.64

X² = 33.91

d.F = 2

p < 0.01

The population is not in equilibrium

what are some reasons what can cause changes in allele frequencies?

• mutations

  • relatively rare

  • random

  • look at development of new allele

• migrations

  • how much goes on

• genetic drift

  • Founder effect

• Genetic bottleneck

Founder effect

big population at first → small group breaks away

Achondroplasia (dwarfism)

1.4 × 10^-5 mutations/allele

population of 500,000 individuals = 1,000,000 gametes

how long will it take for p and q to roughly equal 0.5

p = 1.0

q = 0.0

1,000,000 × 1.4 × 10^-5 = 14 alleles in the next generation

p = 0.999986

q = 0.000014

Next gen = 14 dominant alleles and 999,986 recessive alleles

It will take roughly 70,000 generations for q and p to roughly equal 0.5

• really slow mutation rates

In a population with 100 individuals

Frequency of A (p) = 0.5

Frequency of a (q) = 0.5

100% AA - survive and reproduce

90% Aa - survive and reproduce

80% aa - survive and reproduce

Assume population is in equilibrium

W_AA = 1.0

W_Aa = 0.9

W_aa = 0.9

AA = (0.5)² = 0.25 = 25

Aa = 2(0.5)(0.5) = 0.5 = 50

aa = (0.5)² = 0.25 = 25

A = 2(25)(1) + 1(50)(0.9) = 95

a = 1(50)(0.9) + 2(25)(0.8) = 85

95 + 85 = 180

95/180 = 0.53 = p

85/180 = 0.47 = q

These are an Individual’s fitness

directional selection

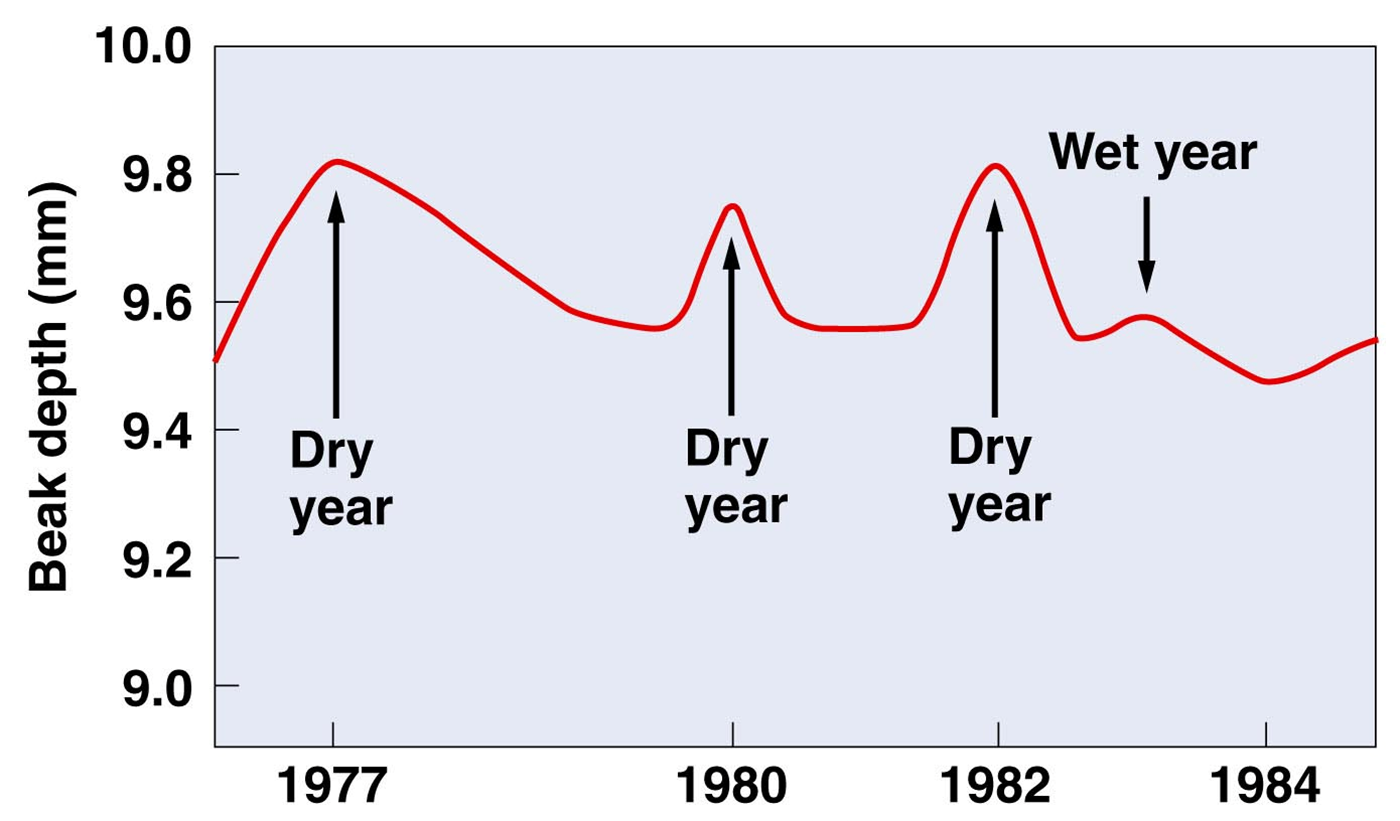
some sort of force pushing a bell curve in one direction

ex: beaks in finches

stabilizing selection

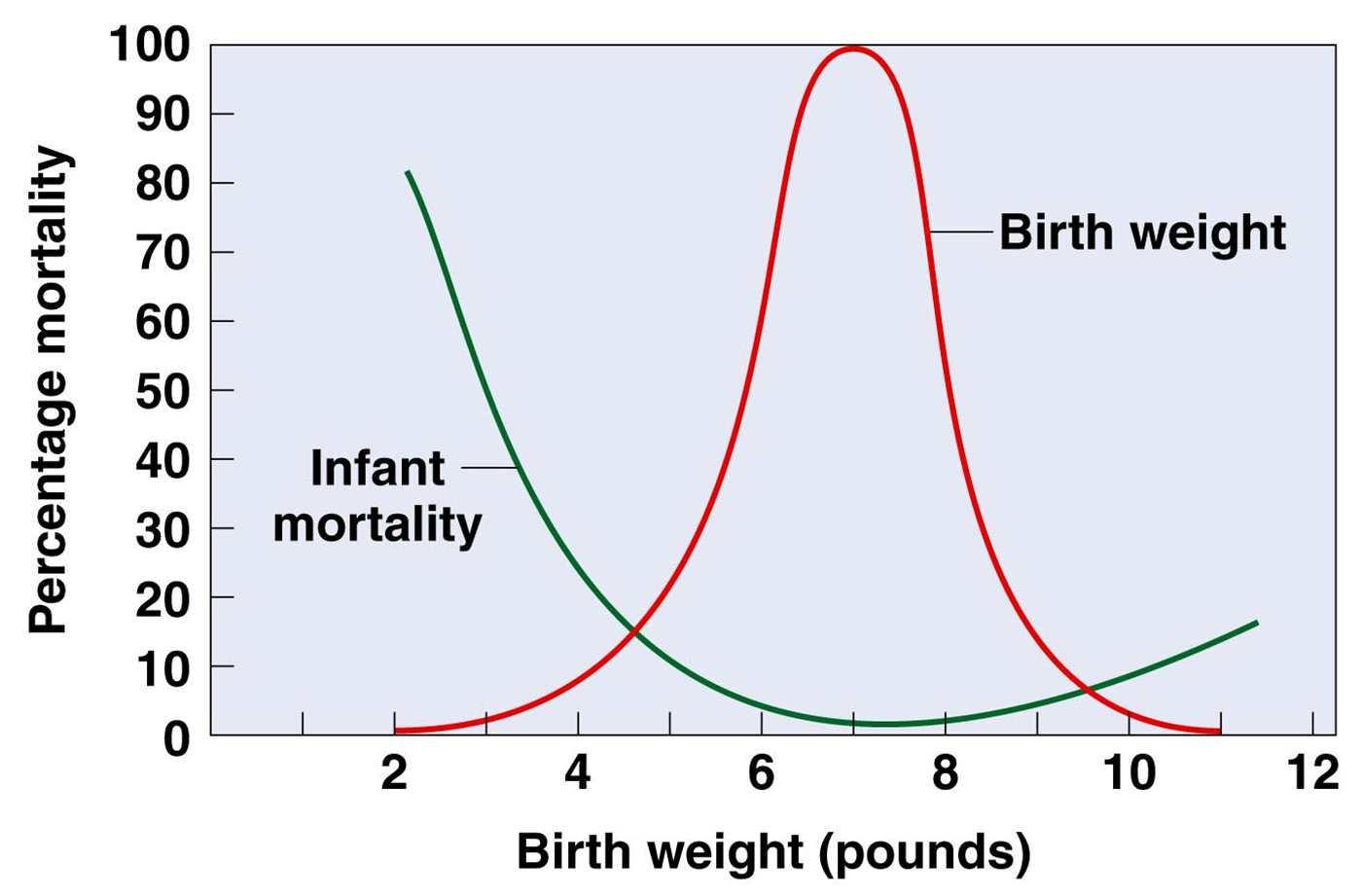
selects against the extremes

ex: human birth weight

• disadvantage for heaviest and lightest babies

disruptive selection

selection favors the extremes

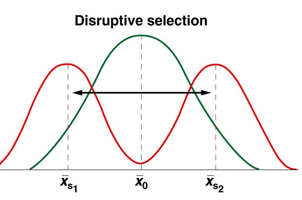
what drives speciation?

disruptive selection

what drives evolution?

directional selection

what prevents speciation?

migration

prezygotic isolating mechanism

things that prevent 2 individuals in a population to mate

postzygotic isolating mechanism

if members are willing/able to mate but aren’t able to produce viable offspring

ex: mule, liger

The Pacific Ocean and the Caribbean Sea are connected at Panama. There were 7 different populations of shrimp in the Pacific and Caribbean respectfully. When the Panama Canal opened, both populations evolved and were a different species. Could they still mate?

3/7 - could not mate

4/7 - could mate

• 60% of the eggs are viable

what lake had 1 species of fish evolve into over 500 species?

Lake Victoria

The more divergence there is in DNA sequences…

the less related a species is to another

BRCA1 and BRCA2

• associated with breast cancer

• DNA damage repair

• very big genes

  • 10,000 nucleotides

• conserved

  • critical for BRCA1 and BRCA2

what does polygenetic inheritance show

shows some sort of quantitative inheritance

meristatic traits

phenotype is described in whole numbers

threshold traits

phenotype is when you either have it or you don’t

multiple genes determine if you have it or not