Convergence, Divergence, More Integration Basics

improper integrals

• a form of DEFINITE integrals (has a lower (a) and upper bound (b))

2 types of improper integrals

1. infinite interval (upper or lower bound is infinitely large/small)

2. discontinuous integrands (there is a break in the domain in the interval [a,b]; there’s a real num where the INTEGRAND is discontinuous)

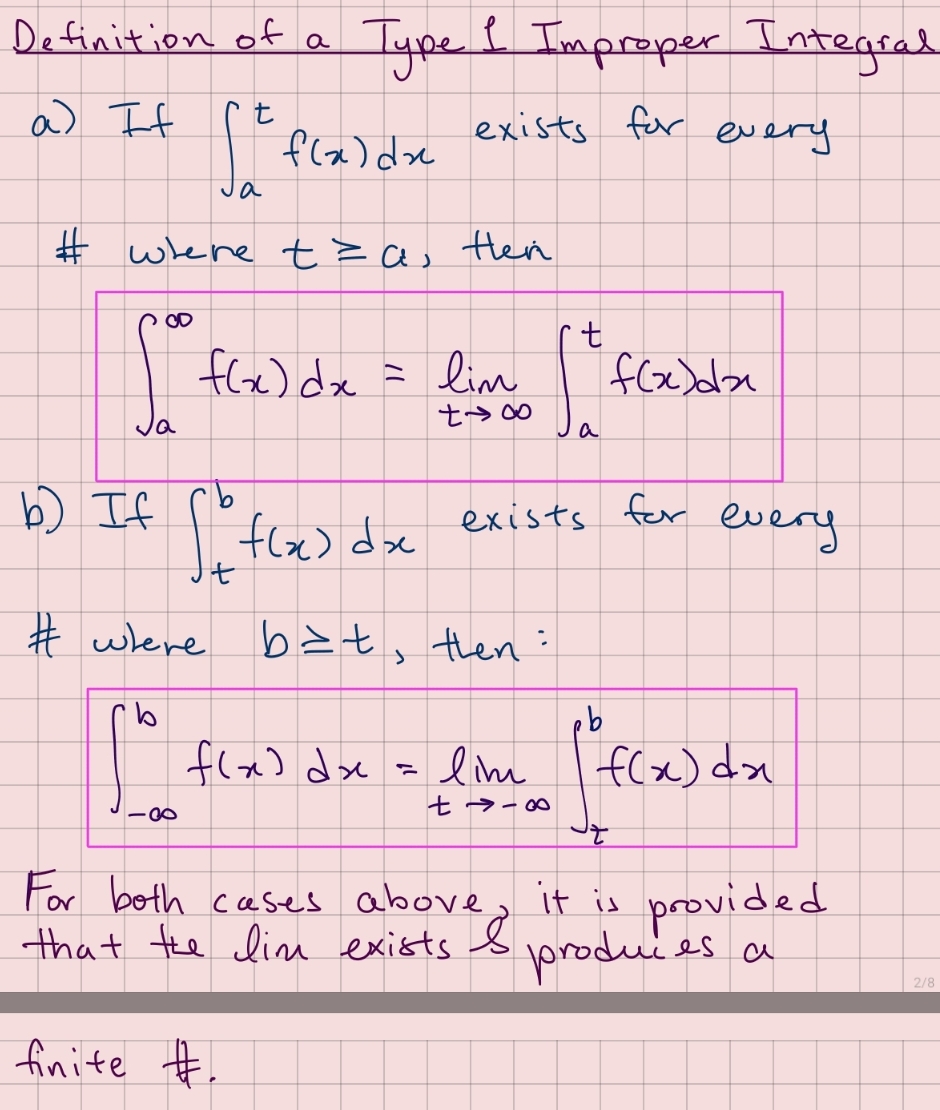
improper integral: infinite interval defn

convergent

to describe an improper integral whose limit is a real tangible number and not infinity

divergent

to describe an improper integral whose limit is pos or neg infinity

improper integrals: infinite interval (case where the upper and lower bounds are infinite)

infinite interval improper integral: some fns can be BOTH convergent AND divergent since it depends on:

which real num is the upper/lower bound

improper integrals: discontinous integrand (type 2) defn

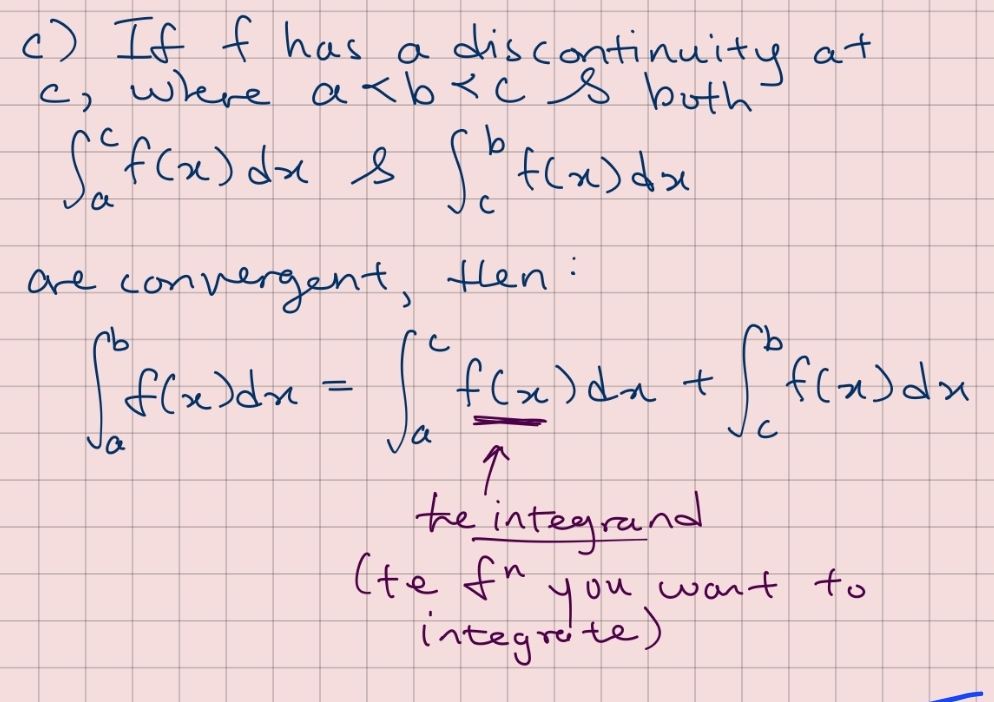
improper integrals: discontinous integrand (type 2) case where the integrand is discontinuous at some value c which is in the interval [a,b]

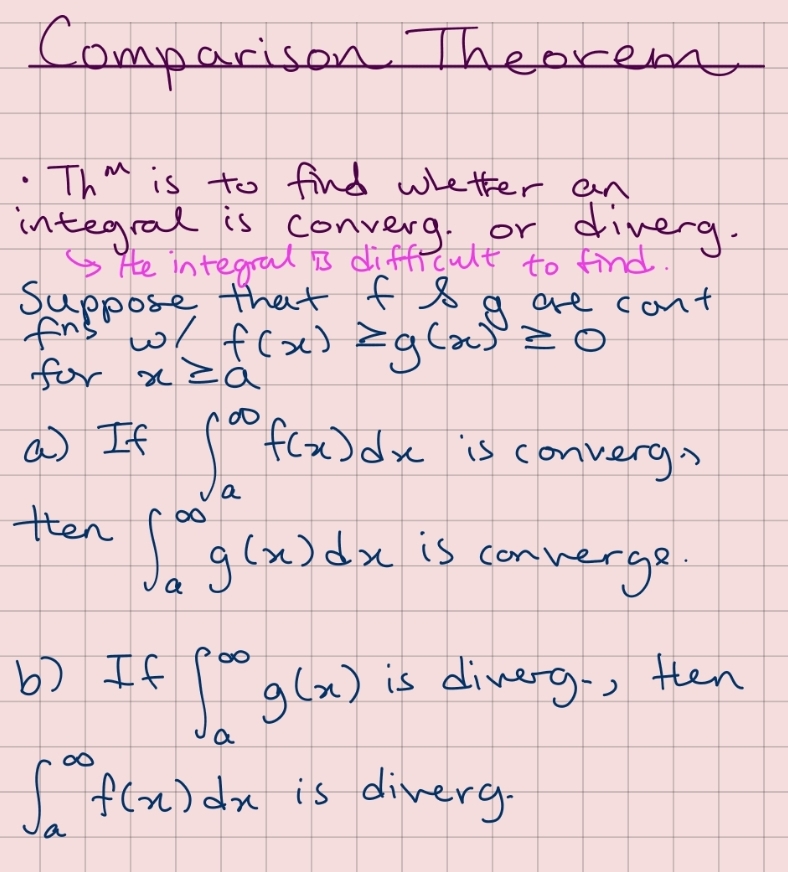
comparison Thm

finding area between two curves formula

area of a complex region

• for the case where f(x) is not greater than g(x) for all x values in the interval OR where you are given more than two curves

  • draw all the curves listed and split up the interval and individually compute the area sections

area w.r.t y

• for the case where f(x) is not greater than g(x) for all x values in the x interval, BUT f(y) IS greater than g(y) for all y values in the y interval

  • each aspect of the graph (functions, upper and lower bound values) must be converted to be w.r.t y

  • compute the integral of f(y) - g(y)

for interval [0,1], 1/x^p is _____________________ if p ___ 0

convergent; less than (so look for the x term w the exponent of less than 1 in this case)

for interval [1,infinity], 1/x^p is _______________ if p ___ 1

convergent; greater than