AP Calc Exam

A limit exists at x=c if

the limit as x —> c- of f(x) = limit x —> c+ of f(x)

A function is continuous at x=c if

limit as x—>c- f(x) = lim x—>c+ f(x) = f(c )

Three types of discontinuity

point (removable), jump, infinite

f is differentiable at x=c if

f is continuous at x=c and limit x—>c- f’(x) = limit x—>c+ f’(c )

Formal definition of derivative

f’(x) = limit h—> 0 f(x+h) - f(x) / h

formal definition of derivative at x=a

f’(a) = limit h—>0 f(a+h) - f(a) / h

alternate definition of derivative at x=a

f’(a) = limit x—>a f(x) -f(a) / x-a

average rate of change on [a,b]

AROC = f(b) - f(a) / b-a

d/dx (x²)

nx^n-1

d/dx [f(x) x g(x)]

f’(x) x g(x) + g’(x) x f(x)

d/dx [f(x)/g(x)]

g(x) x f’(x) - f(x) x g’(x) / g(x)²

d/dx (f(g(x))

f’(g(x)) x g’(x)

d/dx sin

cos

d/dx cos

-sin

d/dx tan

sec²

d/dx cot

-csc²

d/dx sec

sec x tan

d/dx csc

-csc x cot

d/dx e^x

e^x

d/dx a^x

a^x x lna

d/dx lnx

1/x

d/dx sin^-1

1/√1-x²

d/dx tan^-1

1/1+x²

L’Hospital’s Rule

If the limit of f(x)/g(x) gives you 0/0 or infinity over infinity, the limit of f’(x)/g’(x) works

Position

s(t) or x(t)

Velocity

s’(t) or x’(t)

Acceleration

s’’(t) or x’’(t)

Speed

|v(t)|

Speeding up

velocity and acceleration are same sign

slowing down

velocity and acceleration are opposite signs

linearization or linear approximation

y-f(a) = f’(a)(x-a) OR finding equation for tangent line near point and using it to approximate point

Horizontal asymptotes

limit x—> + or - infinity f(x)

Vertical asymptotes

denominator of f(x) = 0

horizontal tangent

f’(x) = 0 or numerator of f’(x) = 0

vertical tangent

denominator of f’(x) = 0

first derivative test for relative extrema

f’(x) = 0 OR f’(x) fails or dne OR number line

second derivative test for relative extrema

evaluate f’’(x) at each critical point to determine concavity

f’’(+) —> U —> minimum

f’’(-) —> n —> maximum

absolute max and min

f’(x) = 0 OR f’(x) fails or dne OR endpoints

f’ is positive

f is increasing

f’ is negative

f is decreasing

f’’ is positive

f’ is increasing and f is concave up

f’’ is negative

f’ is decreasing and f is concave down

relative maximum

f’ changes from + to -

relative minimum

f’ changes from - to +

point of inflection

f’’ changes sign, f’ changes inc to dec or dec to inc, f changes concavity

∫u^n du

1/n+1 (u^n+1) + C

∫sin

-cos + C

∫cos

sin + C

∫sec²

tan + C

∫csc²

-cot + C

∫sec x tan

sec + C

∫csc x cot

-csc + C

∫ du/u

ln|u| + C

∫e^u

e^u + C

∫f(x)

F(x) + C

∫a^b f(x)

F(b) - F(a)

∫a^b f’(x)

f(b) - f(a)

∫ du/√a² - u²

sin^-1 + C

∫du/a² + u²

1/a tan^-1 (u/a) + C

average value

1/b-a ∫a^b f(x) dx

displacement

∫a^b v(t) dt

total distance

∫a^b |v(t)| dt

definite integral

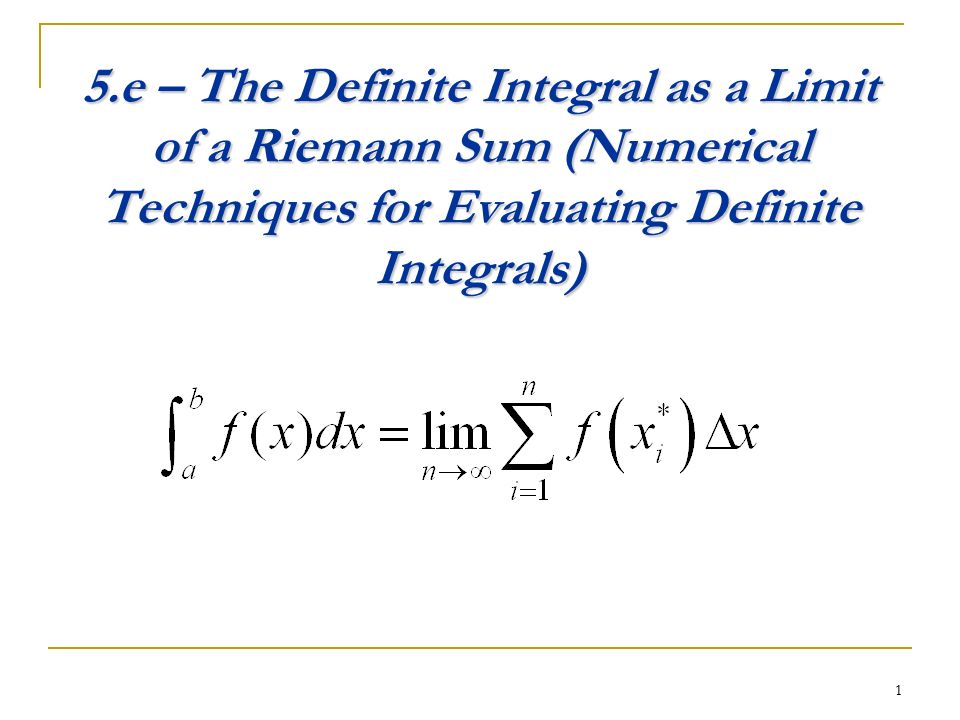
intermediate value theorem

if f is continuous on [a,b] and k is between f(a) and f(b), then there exists at least one c between a and b such that f(c ) = k

extreme value theorem

if f is continuous on [a.b] then there is at least one maximum value and one minimum value on the interval

Mean Value Theorem

if f is continuous on [a.b] and differentiable on (a,b), then there exists at least one c between a and b such that IROC = AROC (derivative = slope)

Rolle’s Theorem

if f is continuous on [a.b] and differentiable on (a,b), and if f(a) = f(b), then there is at least one c between a and b where f’(c ) = 0

1st Fundamental Theorem of Calculus

if f is continuous on [a,b] and F is the antiderivative of f on [a.b], then ∫a^b f(x) = F(b) - F(a)

2nd Fundamental Theorem of Calculus

if f is continuous of [a,b], then G(x) = ∫a^x f(t) dt has a derivative at every point on [a,b] and dG/dx = d/dx ∫a^x f(t) dt = f(x)

Mean value theorem for integrals

if f is continuous on [a,b] then at some point c on [a,b], f(c ) = 1/b-a ∫ a^b f(x)dx, which is the average value of f

volume of revolution - discs

π ∫a^b [r(x)]² dx

volume of revolution - washers

π ∫ a^b ([R(x)]² - [r(x)']²) dx