Trigonometric Proofs
1/10
Earn XP
Description and Tags
Trigonometry
Name | Mastery | Learn | Test | Matching | Spaced |
|---|
No study sessions yet.
11 Terms
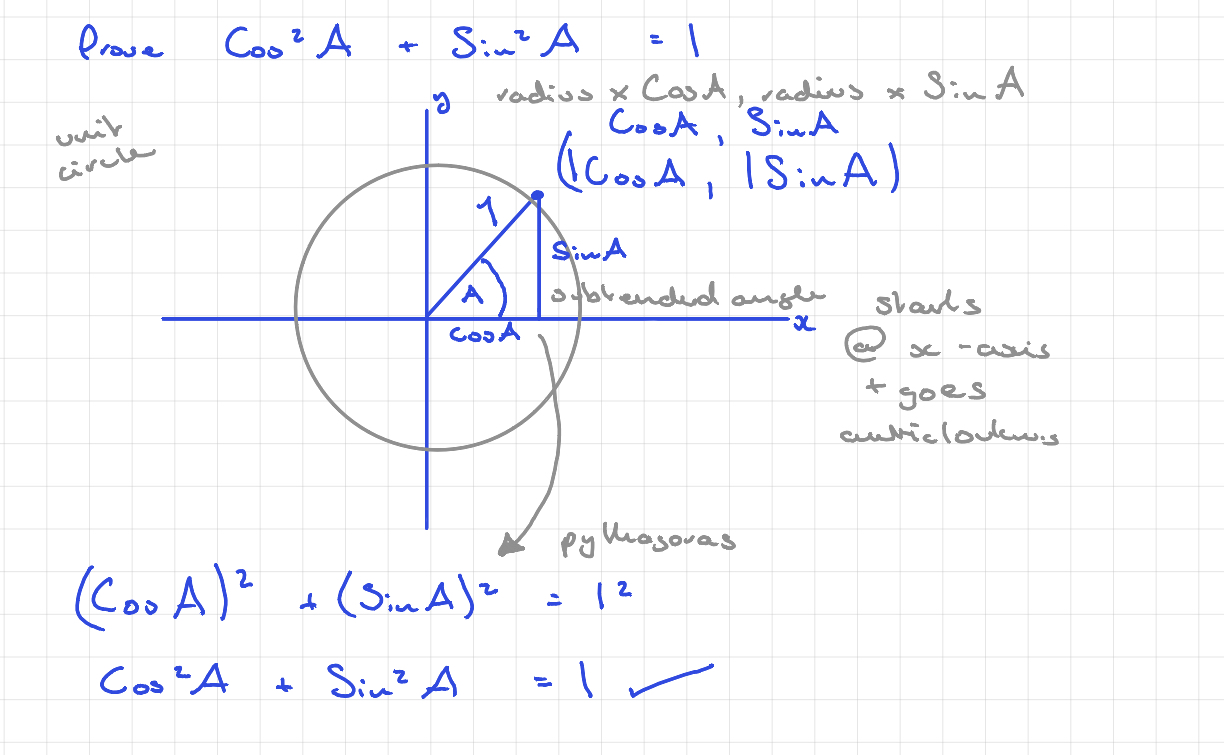
1 - Prove Cos2 A + Sin2A = 1
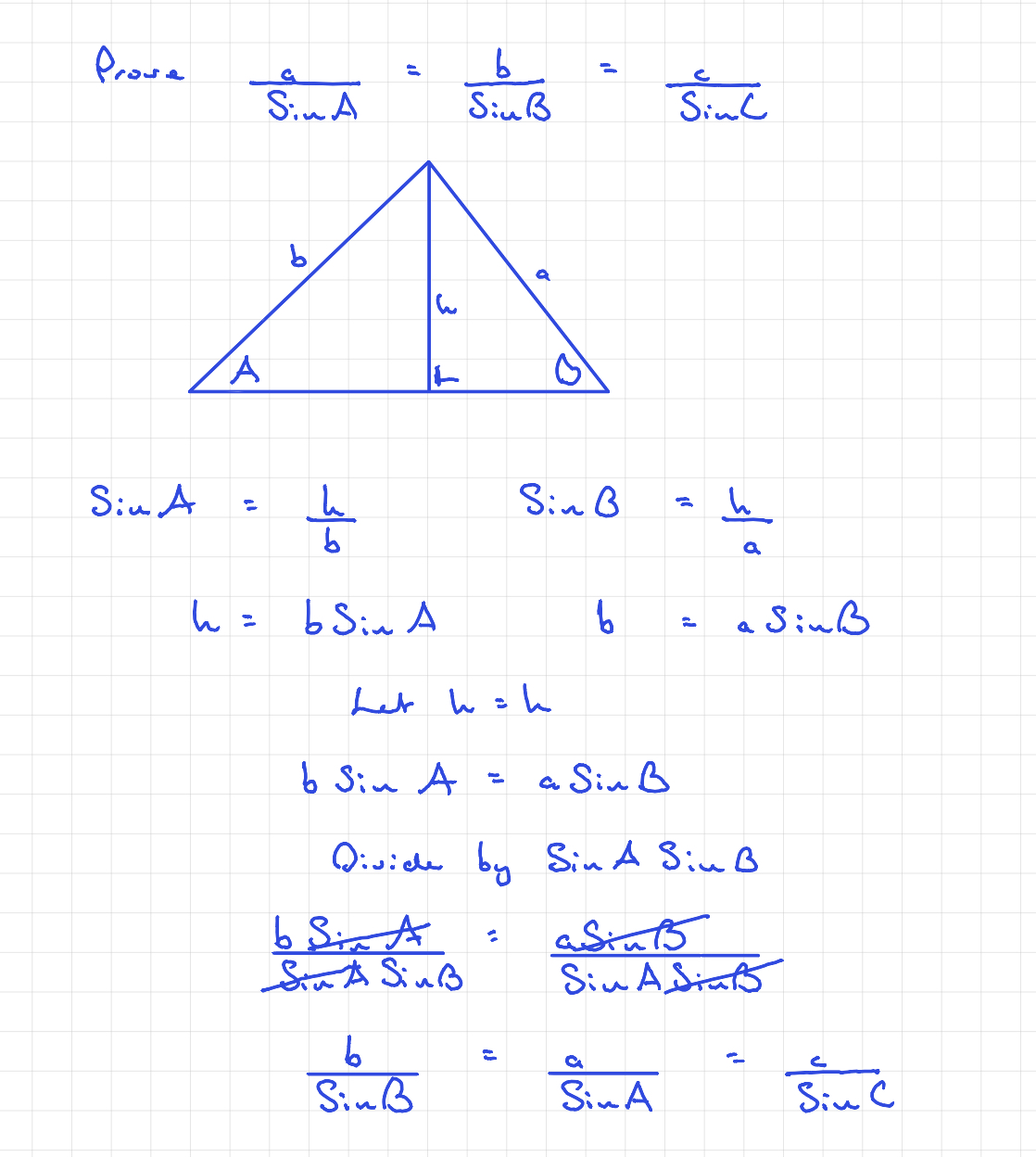
2 - Prove a/SinA = b/SinB = c/SinC,
when given a general triangle
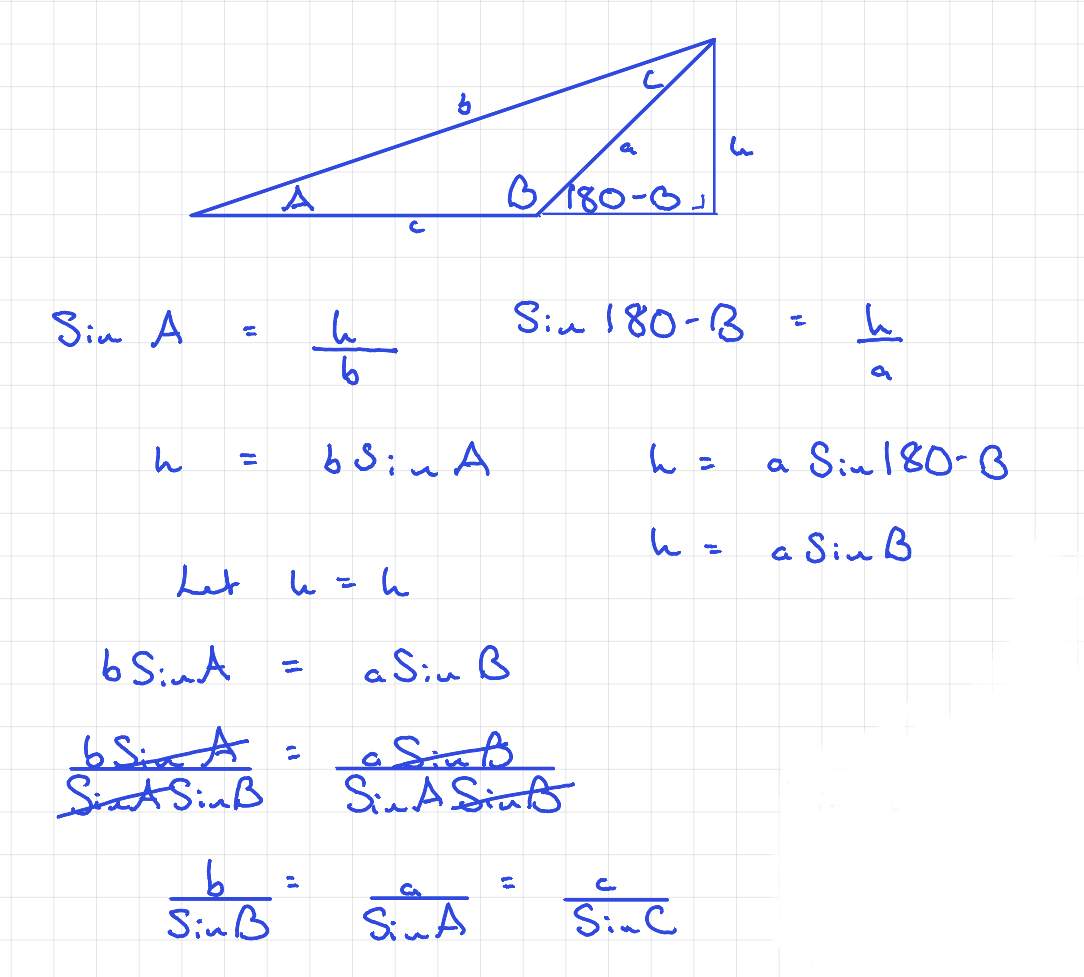
2 - Prove a/SinA = b/SinB = c/SinC
when given an obtuse-angled triangle
When SinA = SinB,
what do the angles add up to?
when Sin of an angle is equal to Sin of an angle, the angles add up to 180o
Sin108o = Sin72o
When CosA = SinB,
what do the angles add up to?
when Cos of an angle is equal to Sin of an angle, the angles add up to 90o
Cos60o = Sin30o
When CosA = - CosB,
what do the angles add up to?
when Cos of an angle is equal to negative Cos of an angle, the angles add up to 180o
Cos100o = - Cos80o
3 - Prove a2 = b2 + c2 -2bcCosA
Big c in the proof is supposed to be little c

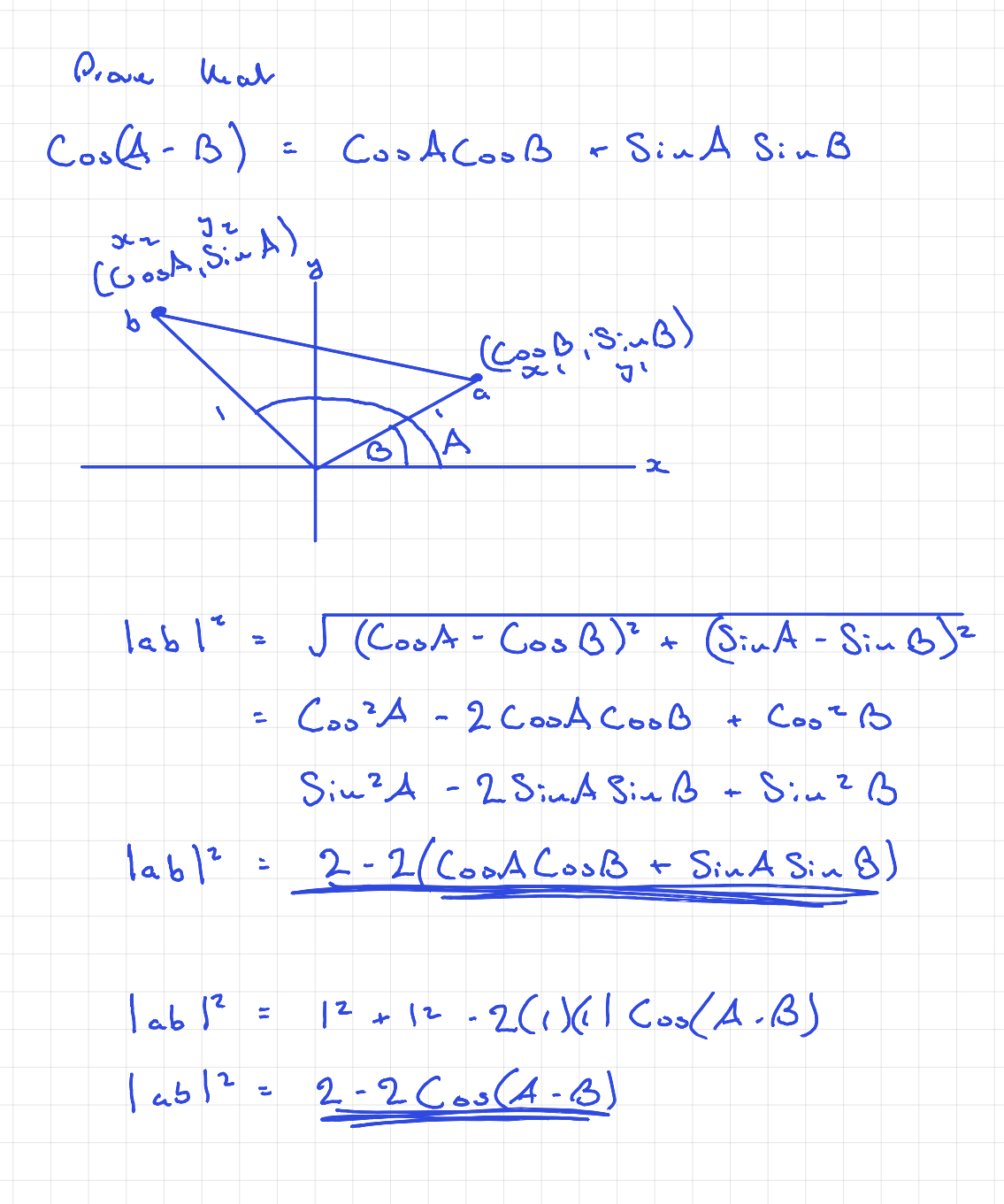
4 - Prove Cos(A-B) = CosACosB + SinASinB
5- Prove Cos(A+B) = CosACosB - SinASinB
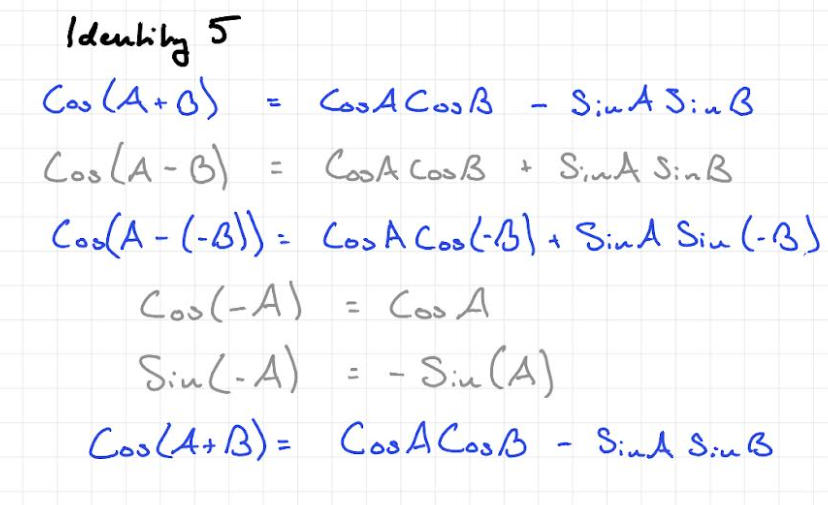
6- Prove Cos2A = Cos2A - Sin2A
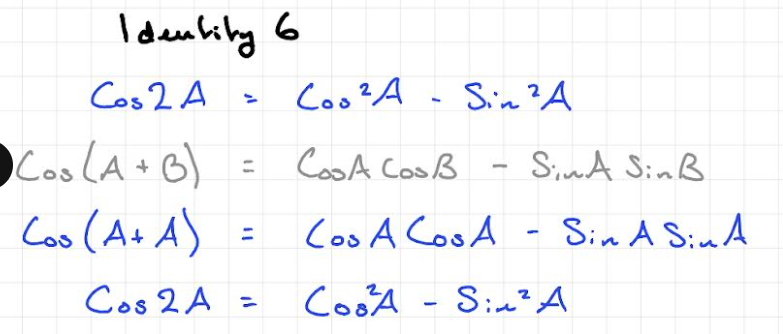
7- Prove Sin(A+B) = SinACosB + CosASinB
