From patterns to generalizations: sequences and series

Un

n is the position of the term in the sequence

U1

first term

Finding the general term

Un = n

recursive sequences

uses the previous term or terms to find the next term. The general term will include the notation Un-1 which means the previous term.

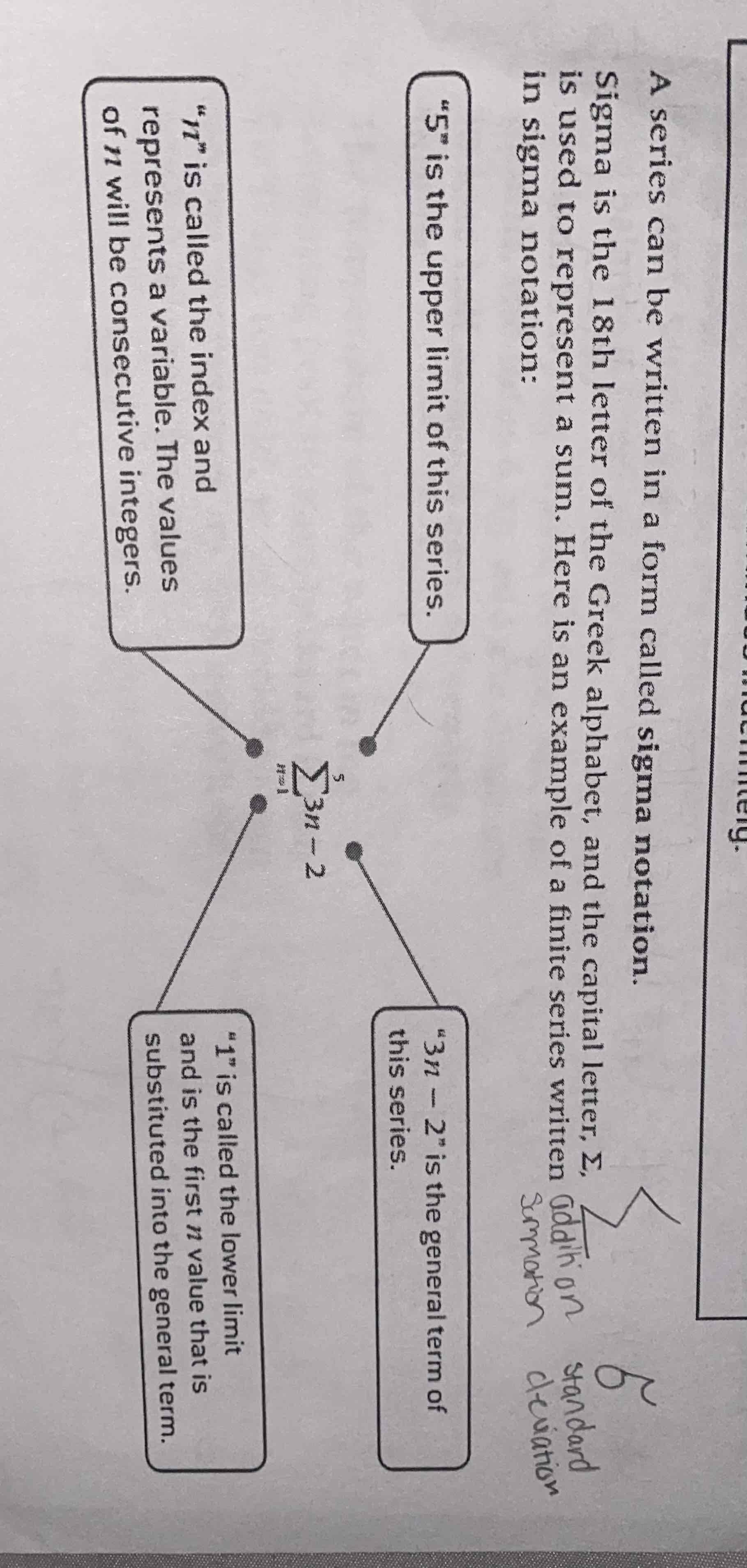
Sigma notation

upper limit can be infinite

The formula for any term in an arithmetic sequence is also known as explicit formula

un= u1 + (n-1) d

to find a term of an arithmetic sequence given two other terms

change the -1 to whatever term number you want to find!!!! then find the difference and substitute that into the equation

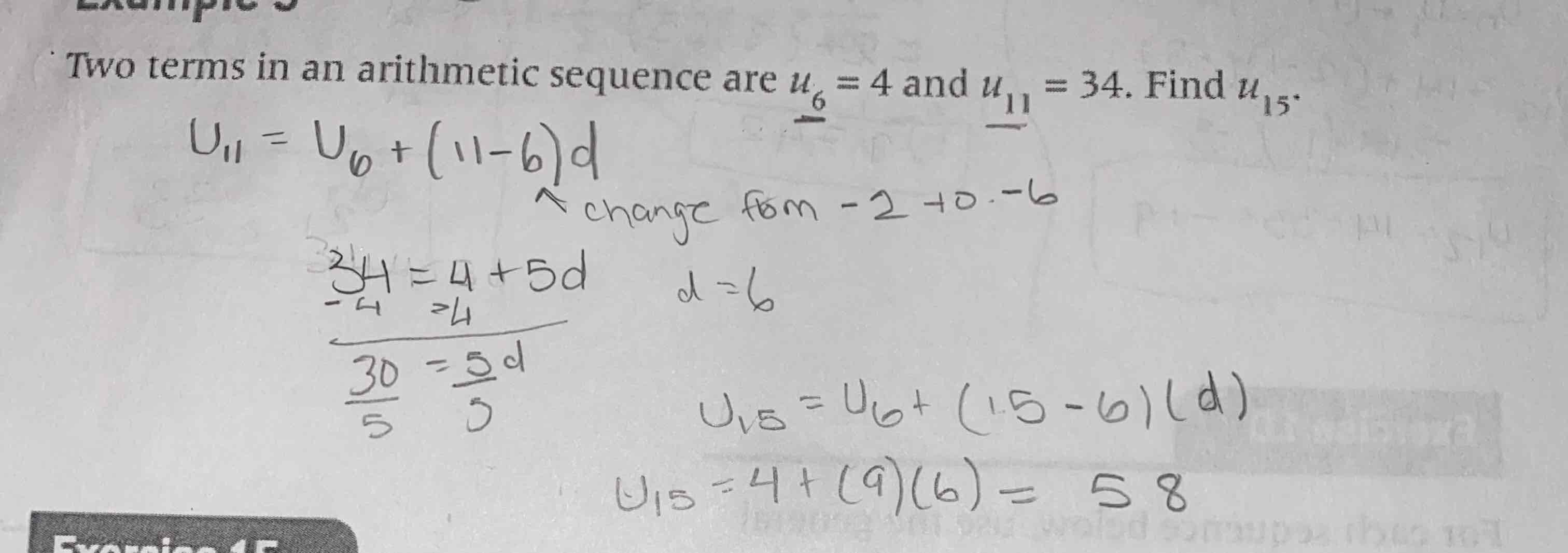
Formula to find any term in a geometric sequence is also know as the explicit formula

Un = U1r^n-1

The formula for the sum of an arithmetic series is

S_n = (n/2) [2u₁ + (n - 1) d]

The formula for the sum of a geometric series

S_n = u1(r^n - 1)/ r-1

sum of a converging (r between -1 and 1) infinite geometric series

S_n = u1/1-r

Meaning of variables in interest equations

A= accumulated amount: future amount

P= Principal

r= annual rate

n= years

t= total number of years

BUTT for compound interest over a non year period

n is equal to the how often do if it’s semi annually n=2, quarterly n= 4, monthly = 12, weekly = 52 etc

Formula for simple interest

A= P(1+nr)

formula for compound interest

A= P(1+r)^n

Compound interest over non year period

A= P(1+ r/n) ^nt

Recursive formula

Un = Un-1

add a number or subtract if arithmetic, multiply or divide a number if geometric