SAT Math

When do we flip inequality < > signs?

Multiplying/dividing by negative, multiplying by reciprocals

What is the “mean”?

The average (sum/total #)

What is the “median”?

The middle number in a set of numbers from least to greatest. (If it’s there’s 0 and 10, and 5 is present, 5 is not always the median)

What is the “mode”?

Most common number in a list

What is “range”?

The span of a data set, like standard deviation (High - low value)

What is considered the “middle” for median?

The middle if odd. If even, it’s the average of the two middle numbers.

What’s another way to describe median?

50% of the repeated values are below and above it

What is range in data?

The max value minus the minimum

How does the median differ in odd/even lists?

In odd lists, it is the middle number. In even lists, it is the average of the two middle numbers.

What is linear change?

X or y changes by the same amount (ex: +5)

What is exponential change?

X or y changes by the same ratio (ex: multiply by 1.05 each year) Graph looks like a J

What does slope tell us?

Rise over run. If the slope is 15, then Y increases by 15 and X increases by 1

What is a constant in algebra?

A number in an expression that doesn’t change. Ex: a=7

a^m*aⁿ

a^m+n

a^m /aⁿ

a^m-n

(a^m )ⁿ

a^m*n

(ab)^m

a^m b^m

(a/b)^m

a^m/b^m

a⁰

1

a^-m

1/a^m

When can you cancel out terms in division?

When they multiply. (If times 5 on num and dom, can cancel out). The cancelled terms become a 1!

If fractions have the same denominator, can you simply add or subtract the numerator?

YEP!

What is a function?

An expression that has a variable and produces an output

What is a composite function?

A function that uses the output of another for its input

How do we solve problems like g(-6)? (Notation)

The -6 replaces the x, evaluate from there!

What does y in terms of x mean? Or its derivatives

y = x + whatever else is there

What is true about the results of even roots?

Their answers can be + or - Ex: 3×3 or -3x-3 equals 9, so the square root of 9 can be plus or minus 3

How do we solve systems of equations?

When the two equations equal each other with the same x (intersect)

What is the algebraic way to solve a linear and quadratic system?

Set both equations equal to each other and try to get x (or a quadratic)

Point slope equation

Y - y1 = m(x - x1)

When do extraneous solutions occur?

Only in radical and rational equations

What does undefined in terms of fractions mean?

The denominator is 0

What is considered a x-intercept?

A point can be an x intercept if y = 0

What is considered an exponential equation?

An equation where the exponent or part of it is a variable. (So basically the graph goes up or down fasttttt)

What is a rational function?

A function defined by a rational fraction

The polynomial remainder theorem

f(x) divided by x-a.. the remainder is f(a). So f(x) divided by x-1, the remainder is f(1)

If we know something like p/q equals 56…

We can say q times 56 equals P.

When SAT asks you what equation is equal to another, what is it likely asking you?

To simply/factor/rearrange

Zero Product Property

(x+1)(x+20) = 0 One of the two parentheses = 0

What can odd roots have that even roots can’t?

They can have negative answers

If you have something like a^6 times b^-2, what do you do?

a^6 divided by b^2, because of the 1

What do exponential graphs often have approaching?

X or Y approaching some value but never exceeding it (like 4^x-2)

What’s the correct way to multiply the discriminant?

4 times a times c

What must we always remember when performing any operation on a variable?

The same mathematical operation will occur to its coefficient (its square rooted too, as an example) (2x)² = 4x²

How do we calculate the volume of an item dropped in water?

L x w x h (The H increase is the new H, like 0.25)

Corresponding angles

Angles with the same position relative to each other

Finding the angles in an “X”

This uses the properties of vertical lines and supplementary angles

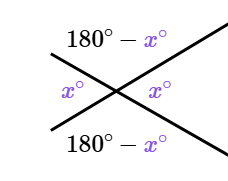
What do the “feathers” on lines and angles mean?

Congruent

Pythagorean theorem

For a right triangle, a² +b² = c²
Actually necessary to remember c is hypotenuse

SOHCAHTOA

• Sine is Opposite over Hypotenuse, Cosine is Adjacent over Hypotenuse, Tangent is Opposite over Adjacent

What is SOHCOATOA always based off of?

An angle and its sides

Complementary angles

Angles that add up to 90

Explain an angle like <BAC

A is the vertex, B and C are the rays

What are rays?

A line with one endpoint

What do the inverse functions of sin/cos/tan tell us?

The measure of the angle (which is based off the ratio of the sides)

What does “rationalize” the denominator mean?

No square roots! Ever!

What is 1 radian?

180 divided by pi

Circle equation

In the xy-plane, a circle with center (h, k) and radius (r ) has the equation:

(x-h)²+(y-k)²=r²

How do we know when slope is increasing/decreasing?

Line goes up as it goes right = increase Line goes down as it goes right = decrease. Steepness indicates level of this

How do we make parallel lines?

They have the same slope

How do we make perpendicular lines?

The lines have negative reciprocal slopes

How do we solve y/9 - x/6 = 1 ?

Multiply by the least common denominator of the denominators (18)

What is the least common denominator?

The lowest number all numbers involved in simplifying can multiply to (for 6 and 9, it’s 18)

When does a system of equations have “infinitely many solutions”?

When you can multiply/divide the equations to be exactly equal (like x+2y = 0 and 2x+4y = 0)

How do we solve systems of equations word problems?

Define variables separately if possible as well as what the two variables equal to

How do go about graphing linear inequalities and finding solutions?

If y is greater than, the solution is above the line. If y is less than, the solution is below the line. (Slope intercept form)

If a is negative in vertex form..

The parabola is pointing down (minimum value)

How do we find the best fit line equation?

Draw a best fit line, get two points then use y-y1=m(x-x1)

If you have x and y (numbers too), to the third root, can you simplify by multiplying them?

Yes, multiply their coefficients and then the radicals, adding them separately and leaving a radical with a coefficient.

How do you rewrite as “fraction multiplication” when there is a fraction of two fractions?

Multiply fractions but get the reciprocal of the second one

Addition

Algebraic long division

Divide the highest degree by the car, take the amount it goes into it and distribute to the divisor as well as putting on top, then subtract that from the dividend and so on

What do you do with the remainder in algebraic long division?

Divide it by the divisor and add it to the answer

What should you do once you’ve set up the fraction multiplication equation?

Multiply! THEN cancel out

What’s the biggest thing with simplifying rational expressions?

Getting the same denominator

For multiplying fractions, do the denominators need to be the same?

Nope! Just multiply both num and dom

What does b need to be in the quadratic equation?

It must be the single degree x.

Do you need to use the quadratic formula to solve a quadratic equation?

No, you can factor or just solve if there’s only x² and no x

What is i?

The square root of -1

Discriminants and solutions

0 = 1 real

Greater than 0 but not a perfect square = 2 irrational

Perfect square, greater than 0 = 2 real

Less than 0 = 2 irrational

What is 10x times x?

10x²

What does a positive slope indicate?

Line goes up, and vice versa

What is the margin of error?

If the margin of error is 12 bpm for something like the avg heart rate of 100 beats per minute, the true value could be 88-112

What is 1.07? (Percentage decimal terms)

107%. So a 7% increase or decrease

Vertex form

y=(x-h)²+k

A way to simplify tough fractional division

Multiply via reciprocal, then factor

When something is cancelled out, what does it become?

1

Can you multiply a denominator onto the other side if it’s only the denominator of one part of one side?

No

If you divide a fraction, can you multiply the denominator by what its multiplied by?

Absolutely

When you can’t combine in a fraction, what do you do?

Rewrite with common denominator. Multiply both denominators by the other denominator

Ways to solve systems of equations

Substitution and elimination

Dividing by a fraction is the same as multiplying by its..

reciprocal. Dividing by 1/3 is the same as multiplying by 3

What’s a good way to deal with fractions and many variables?

Isolate and treat both sides separately, multiplication or division to both affects the entirety of that side

When factoring out a quadratic equation with y…

Be warned that it does not give you the x-intercepts (it gives you their y values). Important for systems of equations

Quadratic/exponential word problems

Break down what you’re solving for, if need be for quadratics define changing things in separate parentheses (which multiply). Break down

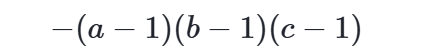
Does the negative sign apply to all terms?

Yes, as a rule. No parentheses are needed, it is assumed.

What is 0/14?

0! A real number/solution

Should you multiply a fraction by -1 if the denominator is negative?

Yes. Denominators must be positive.

V = s³

Volume of a cube. Lxwxh

Multiplying by the reciprocal..

makes it 1