Maths Unit 1

WJEC A-Level Maths

How to solve simultaneous equations?

1. rearrange linear equation

2. set equal to one another

3. find value and sub back in

4. find other value

How to complete the square?

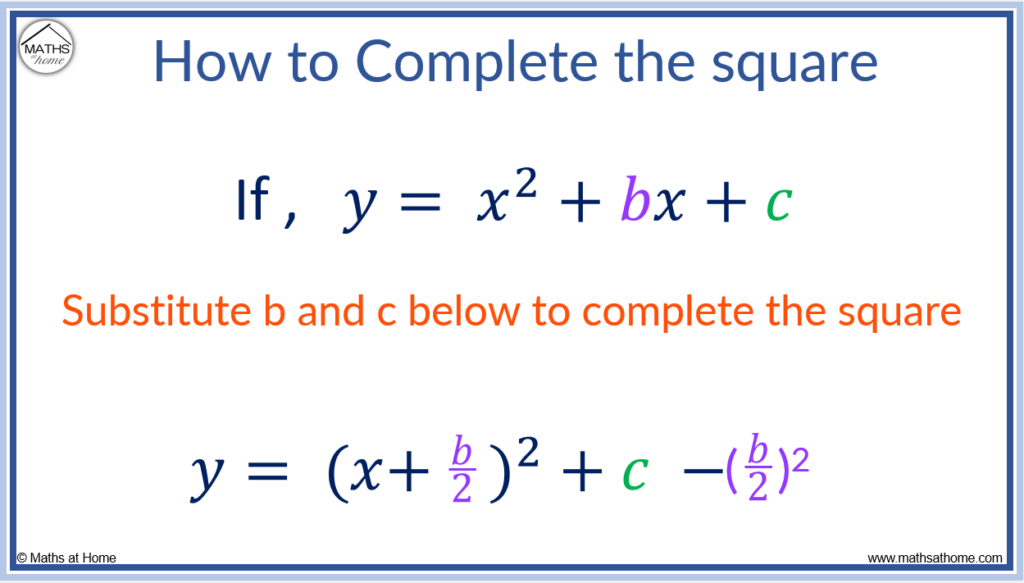
How to find the min/max of a value?

1. complete the square

2. sketch graph

How to solve quadratic inequalities?

1. solve normally

2. state critical values

3. sketch graph to visualise

4. shade region by looking at inequality

5. state inequalities range

Discriminant when 2 real roots

b2-4ac > 0

Discriminant when 1 real root

b²-4ac = 0

Discriminant when no real roots

b²-4ac < 0

Distance between 2 points

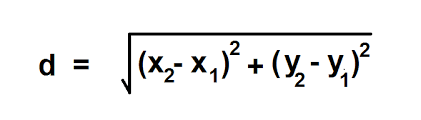
Midpoint of a line

Gradient of a straight line

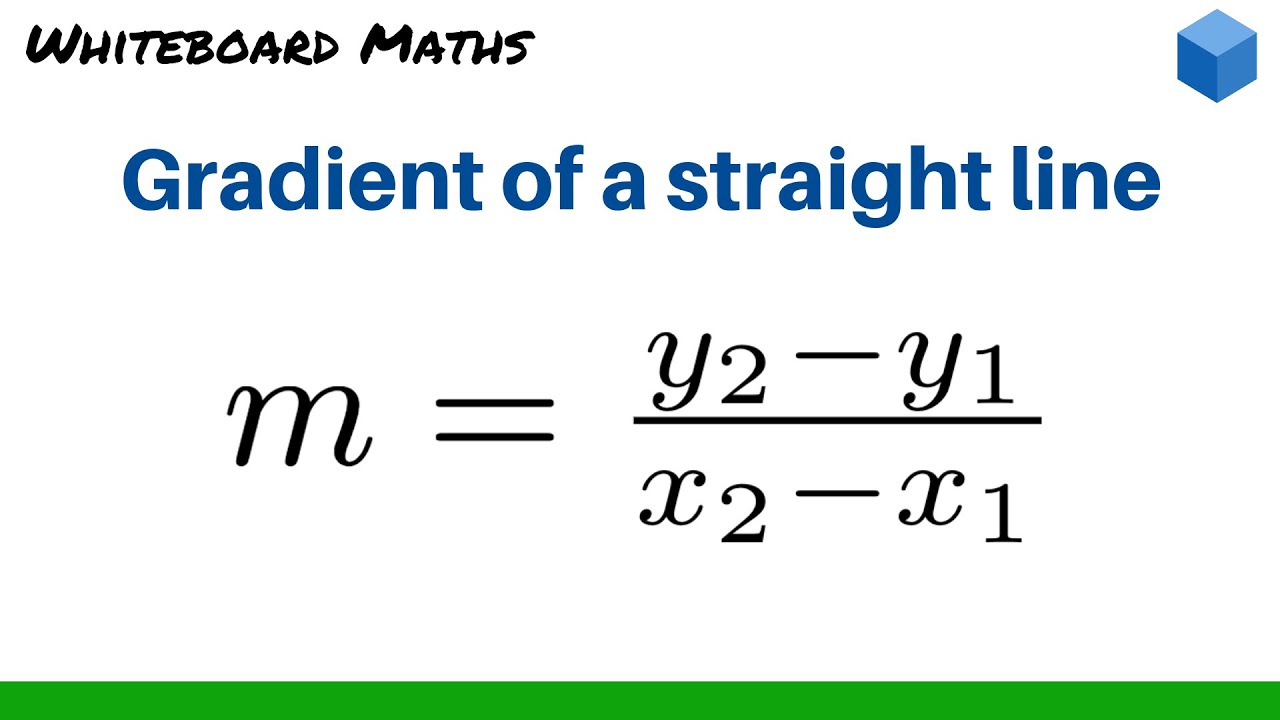
Equation of a straight line passing 2 points

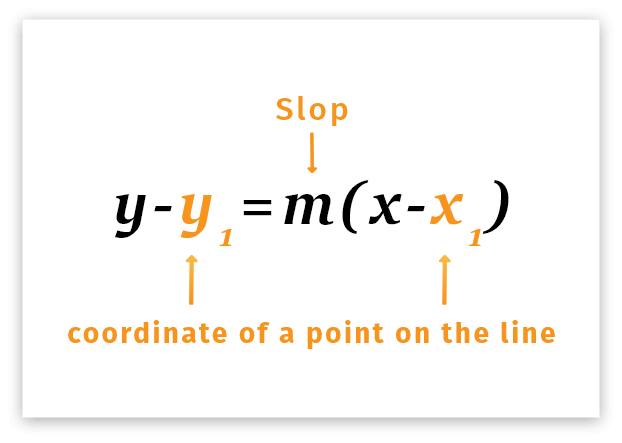
How to know if lines are perpendicular?

if m₁ x m₂ = -1

When are lines parallel?

If they have the same gradient

How to find intersection of lines?

solve equations simultaneously

Equation of circle

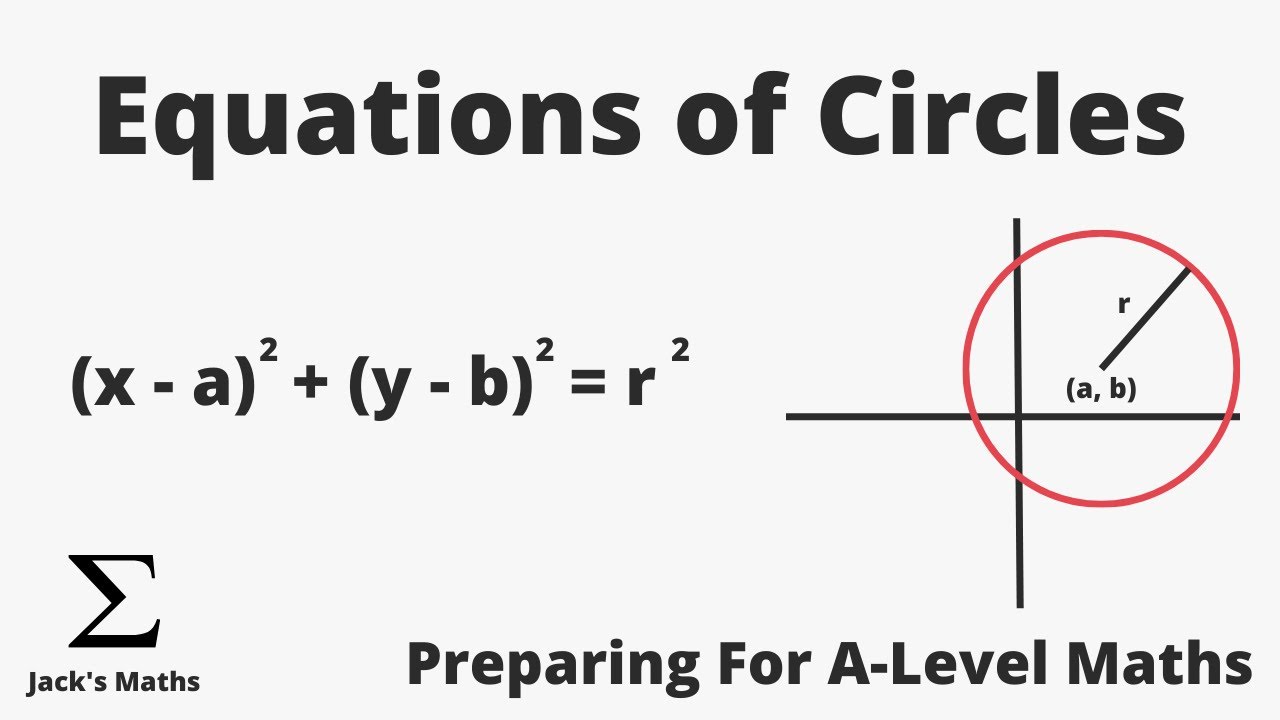
General form of equation of circle

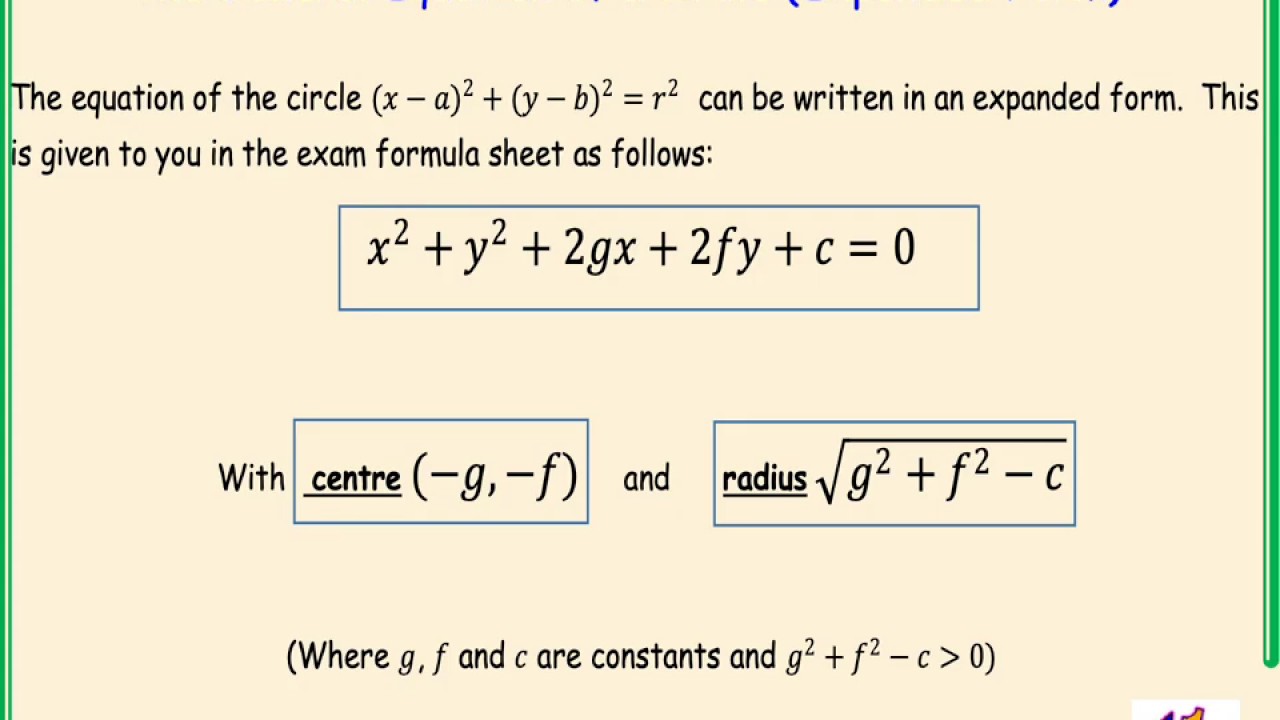
How to find equation of a tangent to a circle?

1. find gradient of radius

2. find gradient of tangent (perpendicular)

3. sub into equation of line using point co-ordinate

Point outside circle

CP > radius

Point inside circle

CP < radius

Point on circle

CP = radius

Circles touch externally

Distance of C_1>C₂ = R₁₊R₂

Circles do not touch

Distance of C_1>C₂ > R₁₊R₂

Circles intersect at 2 points

Distance of C_1>C₂ < R₁₊R₂

Circles touch internally

Distance of C_1>C₂ = R₁—R₂

Line intersects at 2 points

chord

Line intersects at 1 point

tangent

How to differentiate?

1. multiply by power

2. minus 1 from power

3. gradient function

Differentiation from first principles

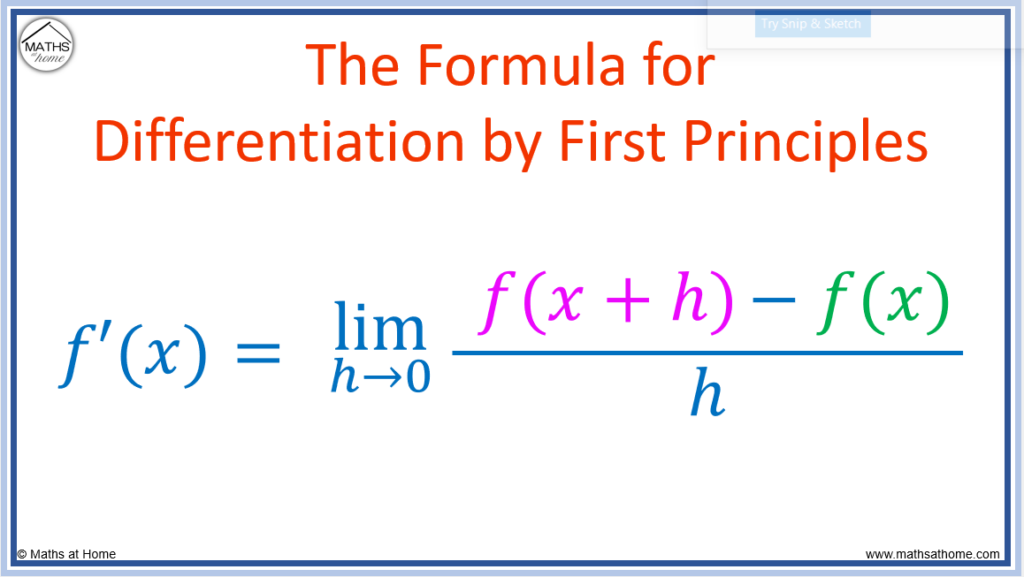
Gradient of normal

perpendicular to gradient of tangent

How to integrate?

1. gradient function

2. increase power by 1

3. divide by new power

4. must have +c

Quadratic formula

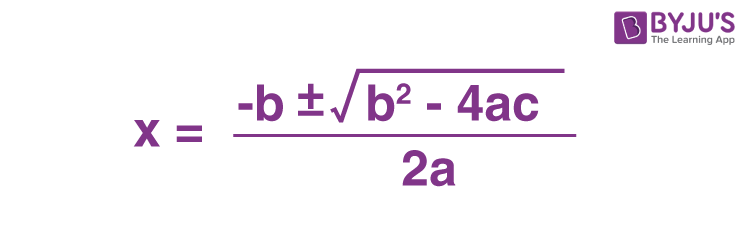
a^m/n

ⁿ√a^m

a^m x aⁿ

a^m+n

a⁰

1

a^-n

1/aⁿ

(a^m)ⁿ

a^mn

a^m / aⁿ

a^m-n

When is a stationary point a maximum?

d²y / dx² < 0
positive to negative gradient

When is a stationary point a minimum?

d²y / dx² > 0
negative to positive gradient

How to classify stationary points?

1. find second derivative

2. sub in x values

When is y increasing?

dy / dx > 0

When is y decreasing?

dy / dx < 0

Sine rule

a / sinA = b / sinB = c / sinC

Cosine rule

a² = b² + c² - 2bc x cosA

Cosine rule for angle

A = cos^-1 ( a² - b² - c² / -2bc )

Area of a triangle

A = ½ x ab x sinC

tanθ

sinθ / cosθ

cos²θ + sin²θ =

1

sine graph

cos graph

tan graph

General binomial theorem

(a+b)ⁿ = aⁿ + ⁿC₁ x a^n-1 x b + ⁿC₂ x a^n-2 x b² + ⁿC₃ x a^n-3 x b³ + … + ⁿC_n x a⁰ x bⁿ

When does a polynomial f(x) have a remainder f(a)?

when divided by (x-a)

When does a polynomial f(x) have a factor?

If f(a)=0 then (x-a) is a factor

|r| =

√a² + b²

What is the magnitude of a vector?

length of it, |r|

Write (2 / 4 ) in terms of i and j

2i + 4j

Finding vectors from coordinates

( x₂ / y₂ ) - ( x₁ / y₁ )

B - A

Dividing a line in a given ratio

μa + λb / μ + λ

What is an exponential

y = e^x

Inverse of y=e^x

y=ln(x)

Inverse of log_a(x)=p

a^p=x

log_a(xy) =

log_ax + log_ay

log_a(x/y) =

log_ax - log_ay

log_a(x)ⁿ =

nlog_a(x)

What is a real number

when squared they give a positive number

What is an imaginary number

when squared they give a negative number

What is a rational number

expressed as a/b when a and b are whole numbers

What is an irrational number

• cant be a fraction

• when decimals they are endless and non repeating

y=f(x) to y=f(x-a)

• inside bracket

• x direction

• opposite

y=f(x) to y=f(x) + a

• outside bracket

• y direction

• same

y=f(x) to y=af(x)

• outside bracket

• y values multiplied by a

y= f(x) to y=f(xa)

• inside bracket

• x direction

• x values divided by a

y=f(x) to y=f(x/a)

• inside bracket

• x direction

• x values multiplied by a

y=f(x) to y=-f(x)

• outside bracket

• y direction

• reflect on x axis

y=f(x) to y=f(-x)

• inside bracket

• x direction

• reflect on y axis