Infinite Series Estimates/Error Bounds/Remainders

Alternating Series Remainder

|S-S_n| = R_n < a_n+1

Estimate an alternating series (as an interval) using the first n terms

1. Find S_n using given n

2. Find a_n+1 using given n

3. |S-S_n| < a_n+1, get rid of absolute value to find final interval by adding/subtracting S_n to both sides

Find the number of terms required to approximate the sum of an alternating series with an error less than x

1. Find a_n+1

2. Solve for n using a_n+1 < x, the given error

Lagrange form of the remainder (of a Taylor Series)

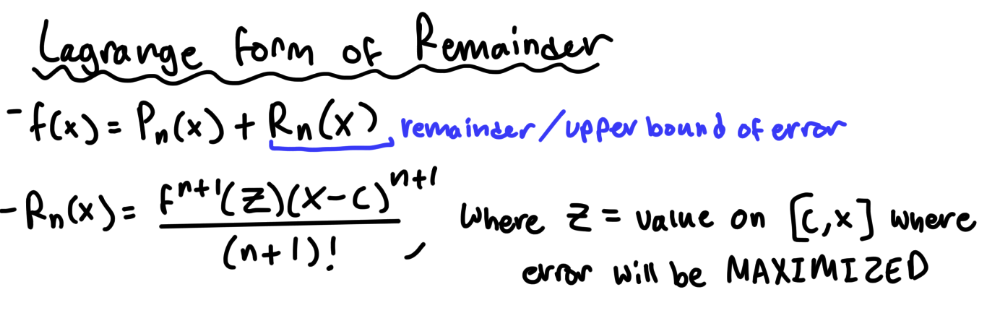
Find the upper bound Lagrange error for a given Taylor polynomial

1. Find fⁿ⁺¹(X), where X is the maximum endpoint (since we will only be using functions that are strictly increasing or decreasing, use first endpoint if function is decreasing)

2. Replace C with given center

3. After all of that R_n(x) = Upper Bound of Error