Geometry: Unit 10 Circles- Tangents, Chords, Arcs, Angles
0.0(0)
0.0(0)
New
Card Sorting
1/24
Earn XP
Description and Tags
Study Analytics
Name | Mastery | Learn | Test | Matching | Spaced |
|---|
No study sessions yet.
25 Terms
1
New cards
Circle
A set of all points a given distance (radius) from a given point, called the center
2
New cards
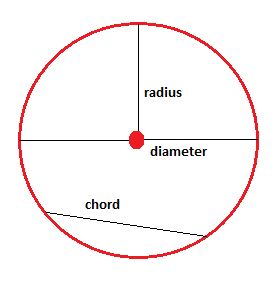
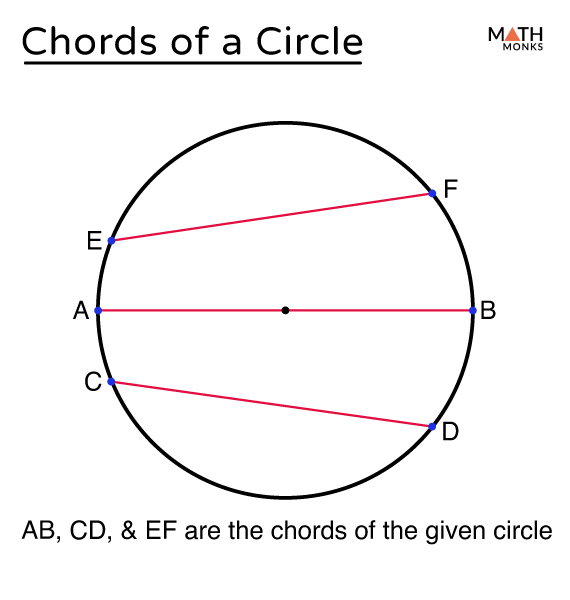
Chord
A segment with its endpoints on the circle
3
New cards
Diameter
The longest chord of a circle that always passes through the center

4
New cards
Arc
A continuous portion of between two points on the circle

5
New cards
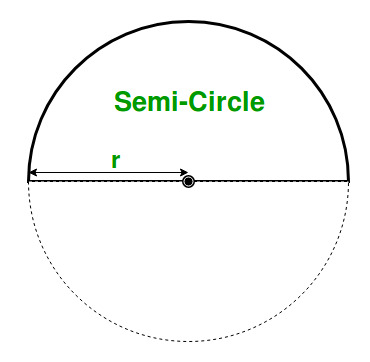
Semi-circle
An arc that is half a circle, the arc’s endpoints are at the diameter, arc measure is 180°
6
New cards
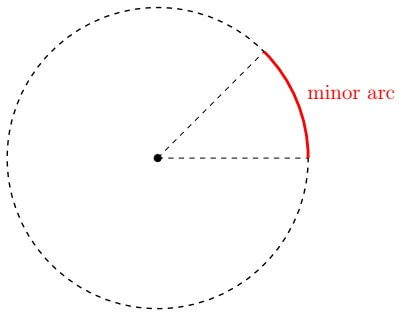
Minor Arc
An arc that is smaller than semi-circle, the arc measure is less than 180°
7
New cards
Major Arc
An arc that is larger than a semi-circle, the arc measure is greater than 180°

8
New cards
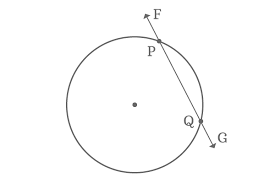
Secant
A line that intersects the circle at exactly two points
9
New cards
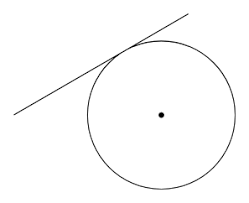
Tangent
A line that intersects the circle at exactly one point or touches the circle at 1 point
10
New cards
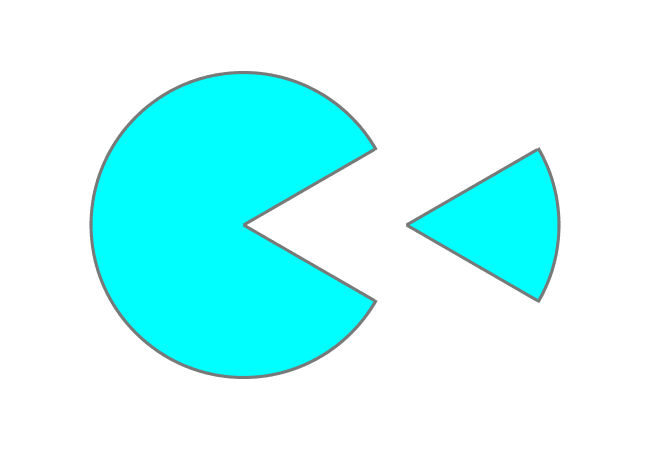
Sector
An area of circle bounded by two radii and an arc
11
New cards
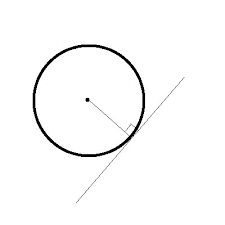
Tangent Conjecture
A tangent to a circle is perpendicular to the radius drawn to the point of tangency.
12
New cards
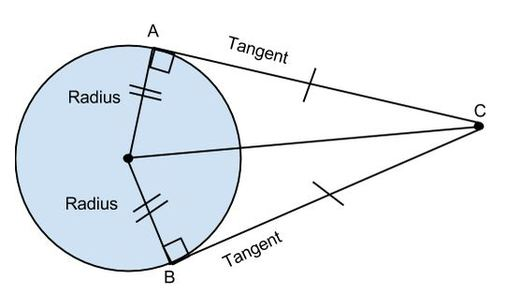
Tangent Segment Conjecture
Tangent segments to a circle from a point outside the circle are congruent.
13
New cards
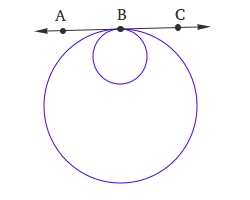
Internally Tangent Circle
14
New cards
Externally Tangent Circle

15
New cards
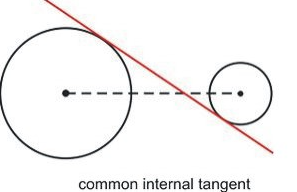
Common Internally Tangent Circle
16
New cards
Common Externally Tangent Circle
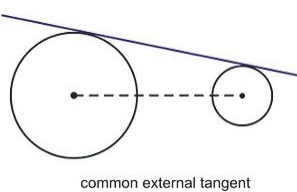
17
New cards
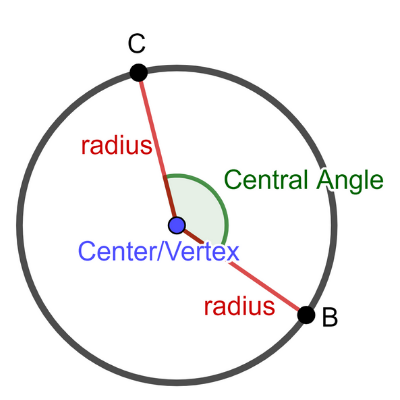
Central Angle
An angle made up of two radius on the circle’s circumference with its vertex at the circle’s center. The measure of the central angle is equal to the measure of its arc.
18
New cards
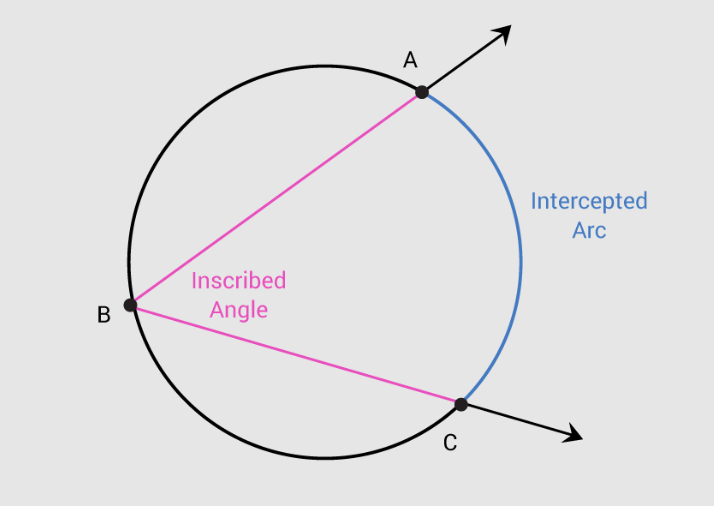
Inscribed Angle
An angle made up of two chords with its vertex on the circle’s circumference
19
New cards
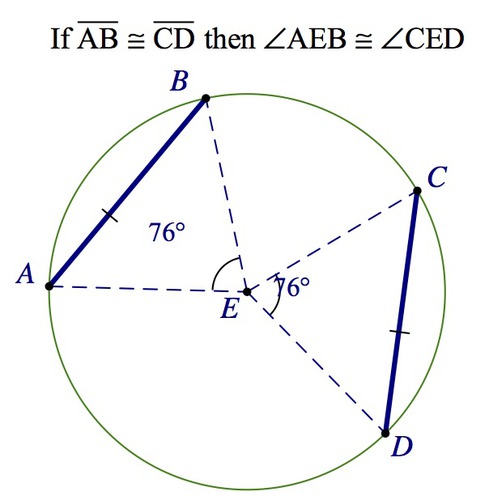
Chord Central Angles Conjecture
If two chords in a circle are congruent, then they determine two central angles that are congruent.
20
New cards
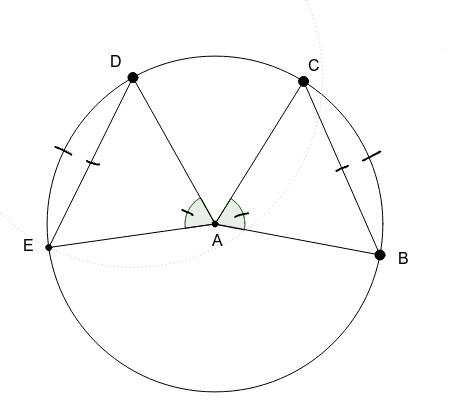
Chord Arcs Conjecture
If two chords in a circle are congruent, then their intercepted angles are congruent
21
New cards
Perpendicular to a Chord Conjecture
The perpendicular from the center of a circle to a chord is the bisector of the chord

22
New cards
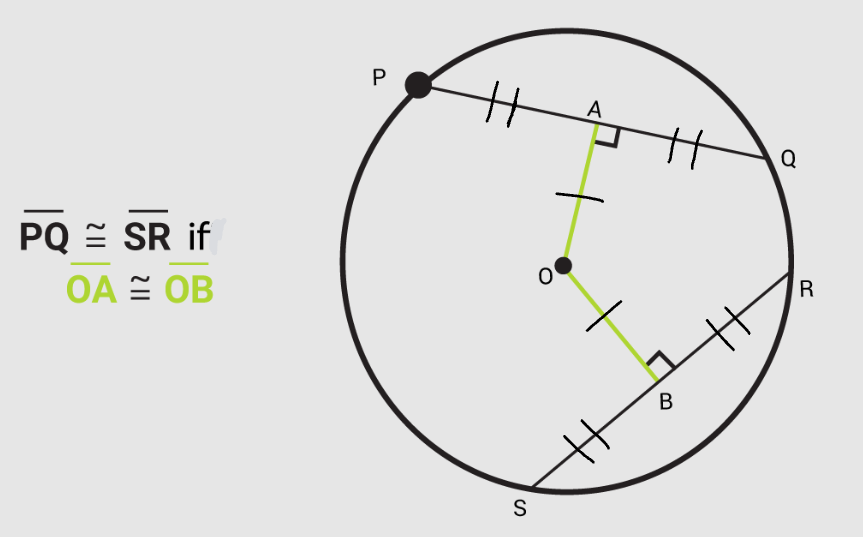
Chord Distance to Center Conjecture
Two congruent chords in a circle are equidistant from the center of the circle
23
New cards
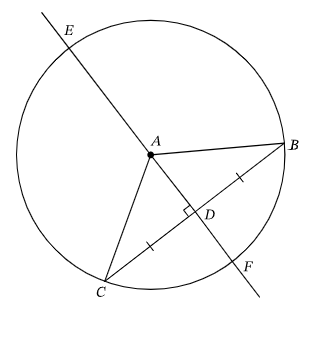
Perpendicular Bisector of a Chord Conjecture
The perpendicular bisector of a chord passes through the center of a circle
24
New cards
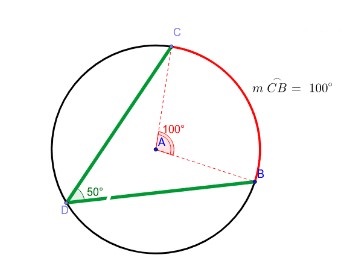
Inscribed Angle Theorem
The measure an inscribed angle is half the measure of its intercepted arc
25
New cards
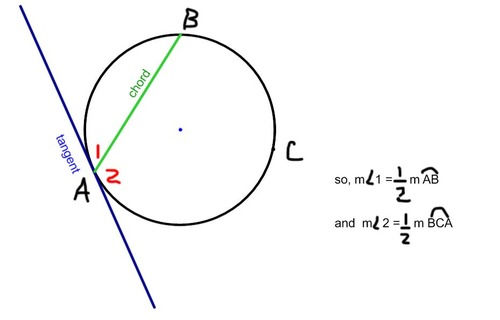
Angle Formed by a Chord and a Tangent Conjecture
The measure of an angle formed by a chord and a tangent is half the measure of its intercepted arc