week 13 - ANOVA

ANOVA

analysis of variances

I = number of group

J = observation number in WITHIN group (2nd number in the subscript)

PART 1

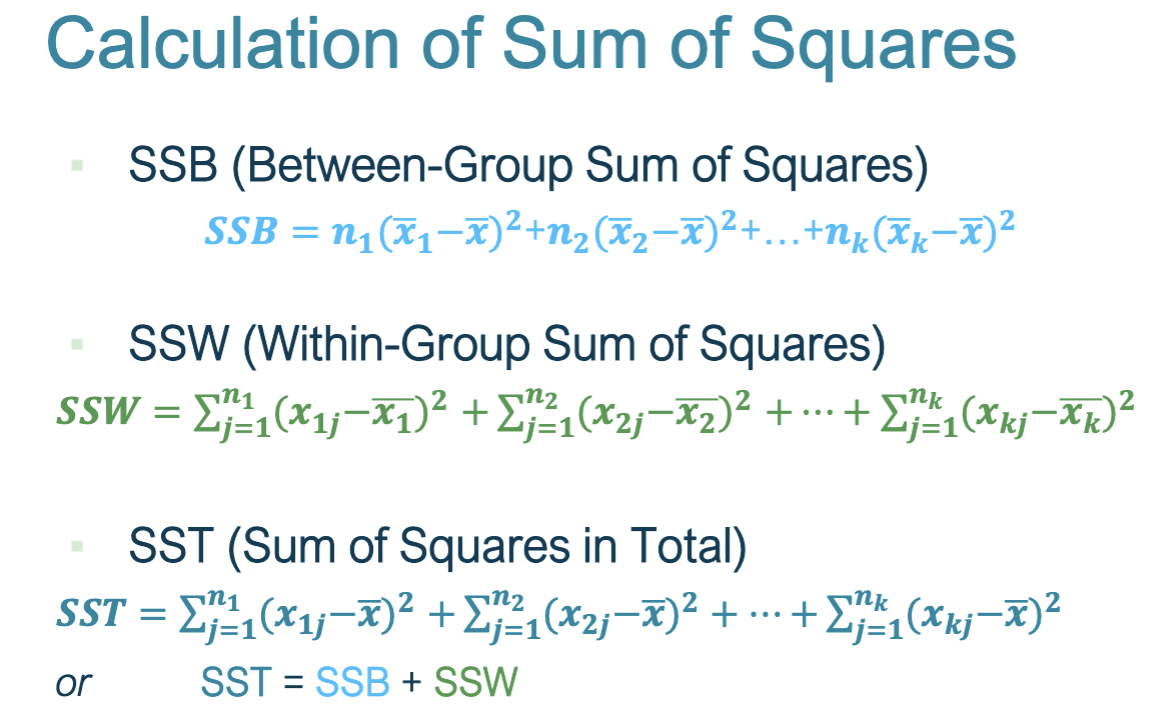
sources of VARIATION (VARIATION in and out of the groups)

1. sum of squares BETWEEN (ssb)

2. sums of squares WITHIN (ssw)

   • each observation is taken into account

=

→ sums of squares TOTAL (SST)

ssb + ssw = sst

• with the use of grand mean

PART 2

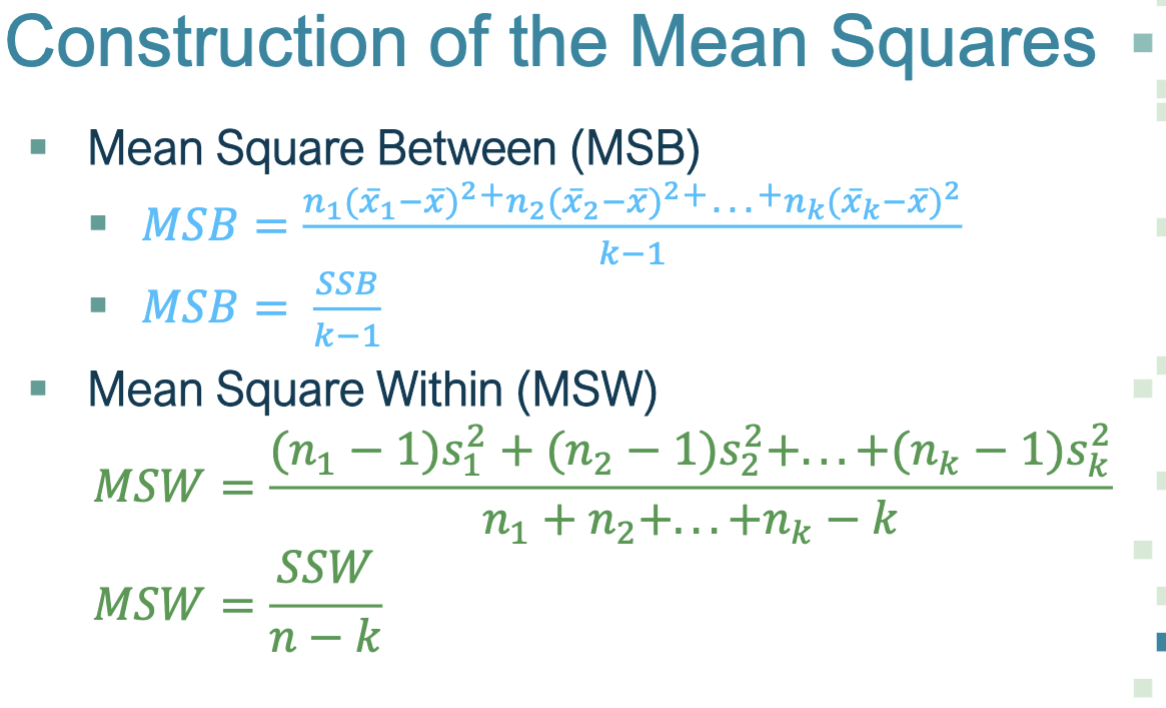
sum of squares is used for the mean of squares

1. SSB and/or SSW

2. mean square for both = MSB and MSW

   

PART 3

sum of squares and mean squares → F test statistic

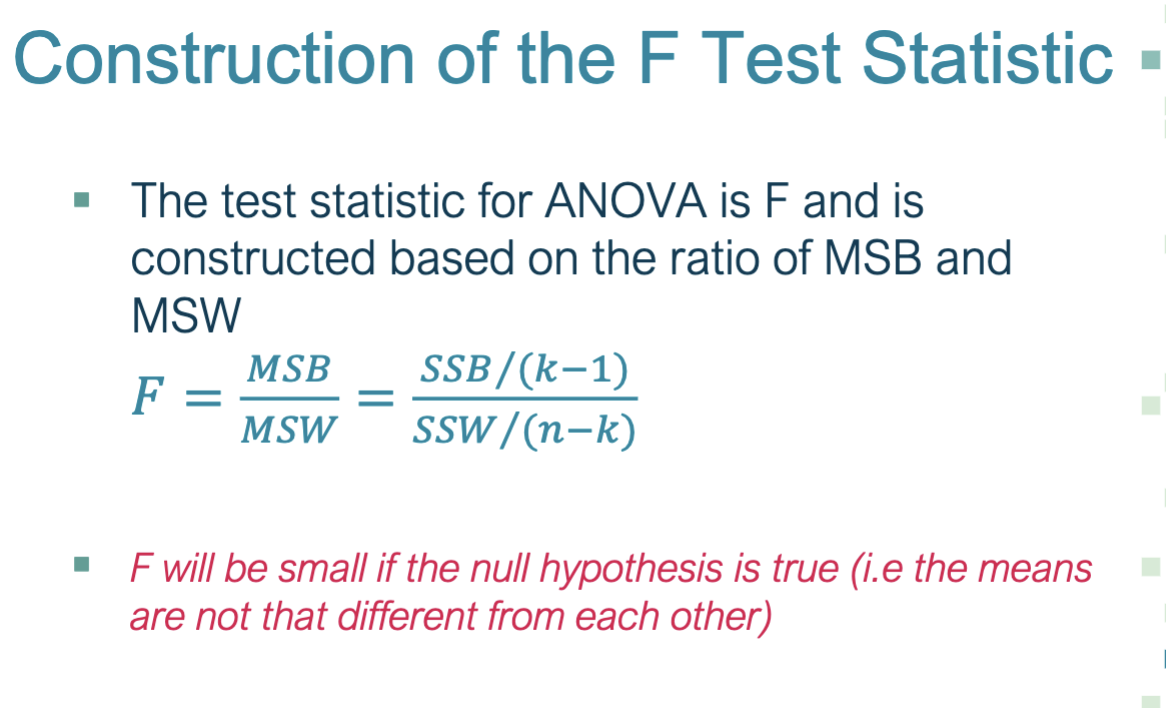
F DISTRIBUTION

one way ANOVA table

ANOVA table example

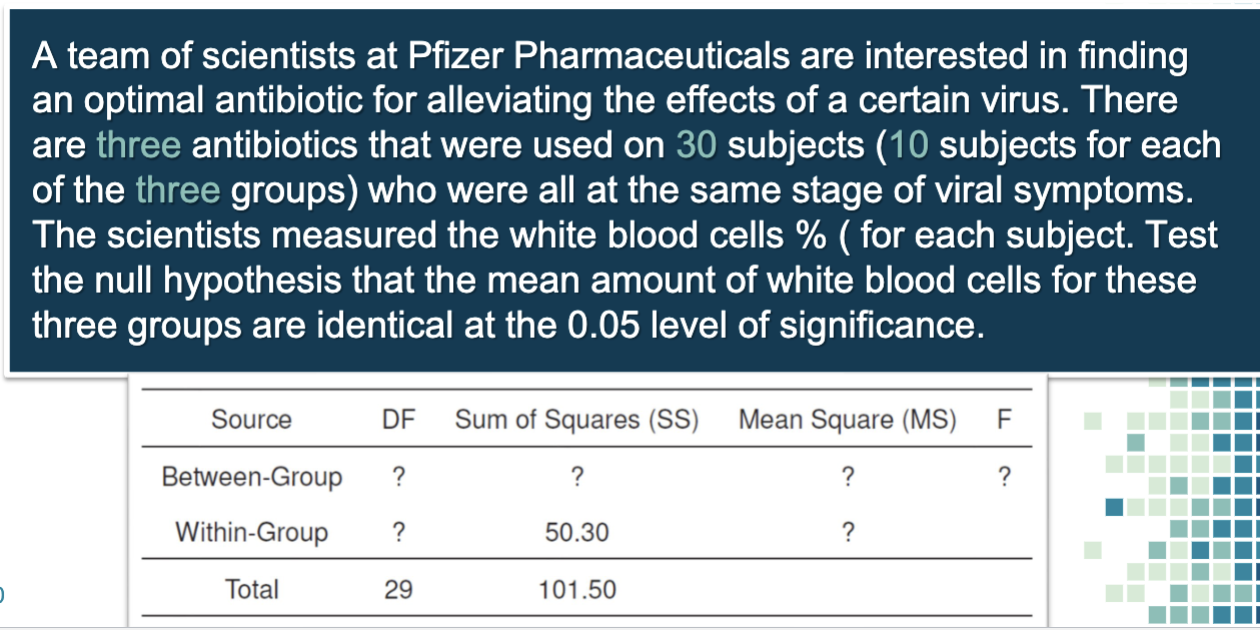
• null hypothesis: mean viral load is the same for all three groups

• alternative hypothesis: there is at least one inequality among the means null. At least one of the group mean viral loads is different

1. find the between and within groups:

2. calculate the t statistic:

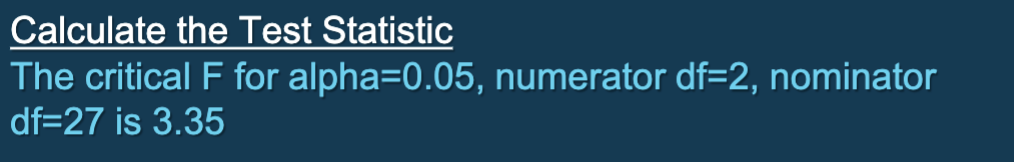
3. conclusion/interpretation: