Astrophysics - All

Define Focal Length.

A fixed property of a lens; the distance away from a screen that a lens bust be for a distant object to be focused on the screen.

Define lens power.

A measure of the curvature of a lens. A lens with more curvature has a smaller focal length.

Give the equation relating lens power and focal length.

Lens Power (Dioptre) = 1 / focal length (m)

P = 1 / f

Give the two types of lenses, and how they effect light.

Convex - converge light

Concave - diverge light

Define a real image.

An image which can be projected onto a screen, i.e. the image is focused on the opposite side of the lens to the object.

Occurs when an object is more than one focal length away from the lens.

Define a virtual image.

An image which cannot be projected onto a screen. The image is focused on the same side of lens as the object.

Occurs when an object is less than one focal length away from the lens.

Explain how to draw a ray diagram.

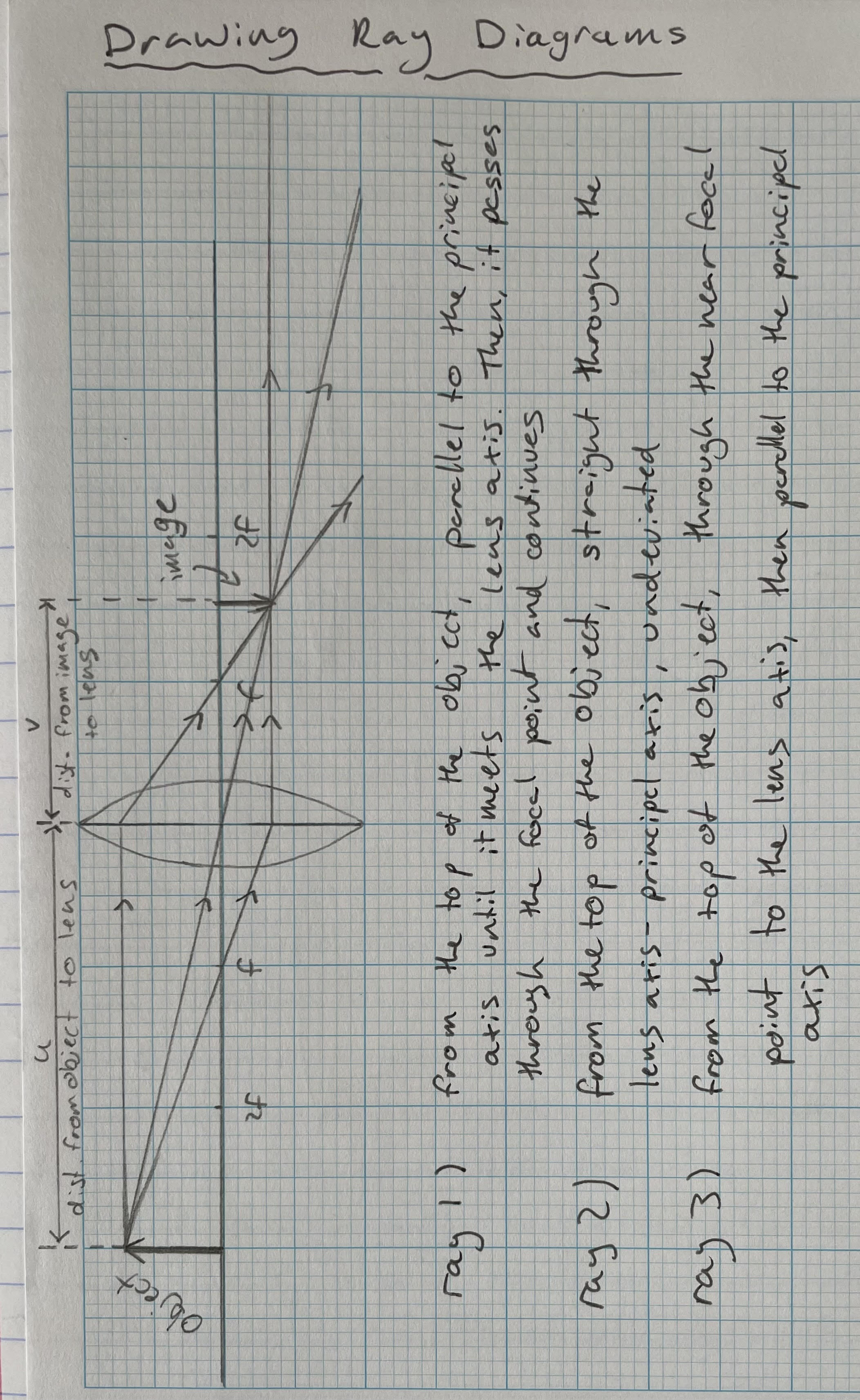
Give the lens equation.

1/focal length = 1/ distance from lens to object + 1/ distance from lens to image

1/f = 1/u + 1/v

Give the equation for magnification.

magnification = distance from lens to image / distance from lens to object

m = v / u

Explain how refracting telescopes work.

Refracting telescopes consist of two lenses. The objective lens converges light from the distant object, and focuses it onto a point in the focal plane, and the eyepiece lens is positioned so its focal plane lines up with that of the objective lens. It produces a magnified image at infinity.

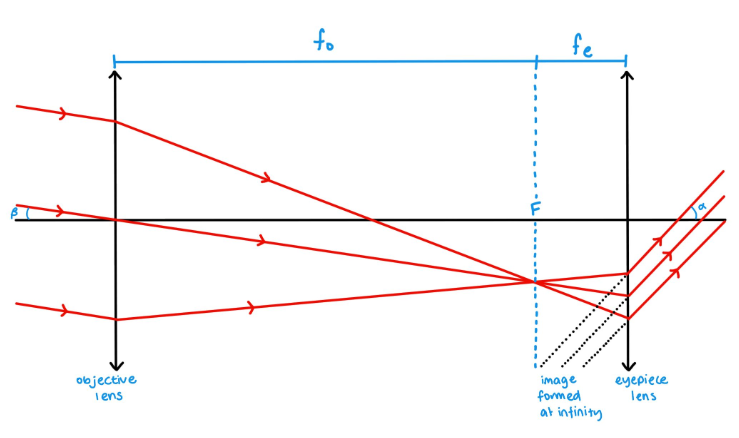
Define magnifying power.

The magnifying power of an optical telescope is the ratio of the focal lengths of the objective and eyepiece lenses.

It is also the ratio of the angles subtending the image and the object.

Give the two equations for magnifying power of an optical telescope.

magnifying power = focal length of the objective lens / focal length of the eyepiece lens

m = f_o / f_e

magnifying power = angle subtending the image / angle subtending the object

m = α / β

Give the lens equation for a telescope.

For a very distance object:

(1/u → 0)

1/f = 1/v

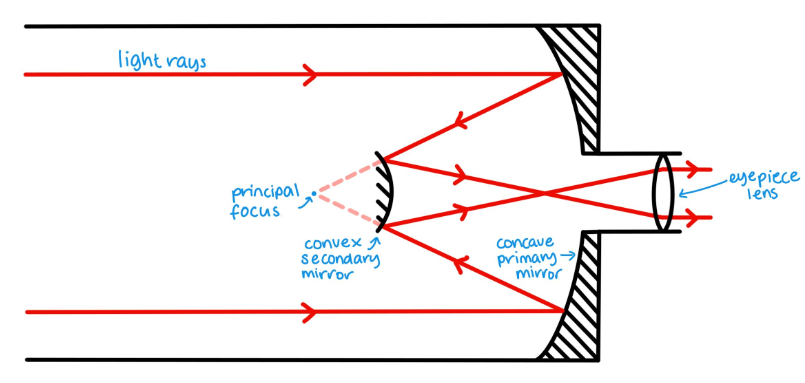
How do reflecting telescopes work?

Use mirrors (and lenses) to focus light.

Define Spherical Abberation.

When the curvature of a mirror is imperfect, so light is focused to different points.

How can spherical abberation be reduced?

Use a parabolic mirror.

Draw a diagram of a cassegrain arrangement reflecting telescope.

Explain Chromatic Abberation.

Light refracting by different amounts depending on its wavelength, causing different colours to focus in different points, causing blurring.

How can chromatic abberation be reduced?

Use a larger lens and an apperture, only allowing light to pass through the centre of the lens, or filter out all but one wavelength of light.

Explain how a CCD works.

Photons of light are incident on silicon in the CCD, causing electrons to be liberated.

These electrons are stored to be read back later.

Give some differences between the eye and a CCD.

• The eye has a low quantum efficiency (percentage of incident photons detected), whereas CCDs have a high quantum efficiency.

• CCDs can detect a much wider spectrum than just visible light like the eye.

• CCDs have a spatial resolution of 10 μm whereas the eye has a spatial resolution of 100 μm.

• CCDs require additional equipment to use and must be placed in the correct location.

• CCDs produce digital images which can be stored and shared.

Define resolution.

The ability to distinguish between two objects with low angular separation.

Explain why telescopes have a limited resolution.

When two objects are far away and their light passes through an aperture, a circular single-slit diffraction pattern occurs for each.

When the light falls on a sensor (eye or a CCD), it can overlap.

If the angular separation is too small, the central maxima superpose, appearing as a single object.

Define the Rayleigh Criteria.

The limit at which two objects can be resolved.

The point at which the central maxima of one diffraction pattern lines up with the first minima of the second.

Give the formula for angular separation of two objects a great distance from the observer.

Angular separation ≈ distance between objects / distance from objects to the observer

θ ≈ x / y

How do you calculate the maximum resolving power of a telescope?

Angular resolution = wavelength of incident light / diameter of the aperture

θ = λ / d

How are radio telescopes similar to optical telescopes?

They use a parabolic objective mirror.

How do radio telescopes differ from optical telescopes?

They use a wire mesh for the mirror, rather than an optical mirror.

Waves are directly focused onto an antenna at the focal point.

How is a signal processed in a radio telescope?

Signals are detected by the antenna, then passed through a pre-amplifier (amplifies weak signals without adding too much noise) then through a high-gain amplifier.

The signal is then passed through a tuner to filter unwanted wavelengths, then digitised into a false-colour image.

How does the resolving power of radio telescopes differ to optical telescopes?

Radio wavelengths are ~ one million times longer than visible light, so the resolution is much lower.

How can the issue of radio telescopes’ resolving power be corrected?

Multiple smaller telescopes can be combined to produce a single image, with resolutions thousands of times better than optical telescopes.

Describe two benefits of radio telescopes.

Being able to make telescopes out of fine wire mesh makes their construction cheaper and easier than optical telescopes.

The longer the wavelength, the less it is impacted by imperfections in collecting dish/mirror. Spherical aberration is much less of an issue.

How are IR and UV telescopes similar to optical telescopes?

They use very similar constructions, with similar parabolic mirrors and CCDs or photographic paper.

How are the mirrors different in IR and UV telescopes versus optical telescopes?

As spherical aberration is affected by the wavelength of light, IR (with a longer wavelength) is less affected by this aberration, so mirrrors can be less perfect.

UV, in contrast, has to be more accurate due to its shorter wavelength.

How are IR telescopes affected differently to other telescopes?

All objects, including the telescope itself, emit IR radiation, so to stop the telescopes from detecting its own IR radiation, they must be cooled to very low temperatures using liquid helium or refrigeration units.

How does the penetrative ability of X-rays make constructing telescopes difficult, and how do we get around this?

X-rays only reflect at grazing angles.

We use a series of grazing mirrors to redirect the incident X-rays into a focal point.

How do we detect X-rays in X-ray telescopes?

They can be detected using a modified GM tube, or with a fine wire mesh.

Modern telescopes use highly sensitive X-ray CCDs.

How is the resolving power of a telescope limited by the Rayleigh Criterion?

Resolving pwer depends on wavelength of incident radiation and dish / aperture diameter. For the same size of dish, a UV telescope has a higher resolving power than a radio telescope.

How is the resolving power of a telescope limited by the quality of detector?

The closeness of detector units affects resolution. This could be the dimensions of CCD pixels, or by how fine a wire mesh is.

Define collecting power.

The amount of radiation a telescope can recieve; proportional to area. A telescope with a larger dish collects more energy, so images are more intense, and fainter objects can be observed.

Give the equation for collecting power.

Collecting power ∝ (dish/aperture diameter)²

How can you use parallax to calculate distance?

Calculate an angle to a fixed point from two locations a known distance apart. The distance from you on average to that fixed point is found by the average the perpendicular distances found using trigonometry.

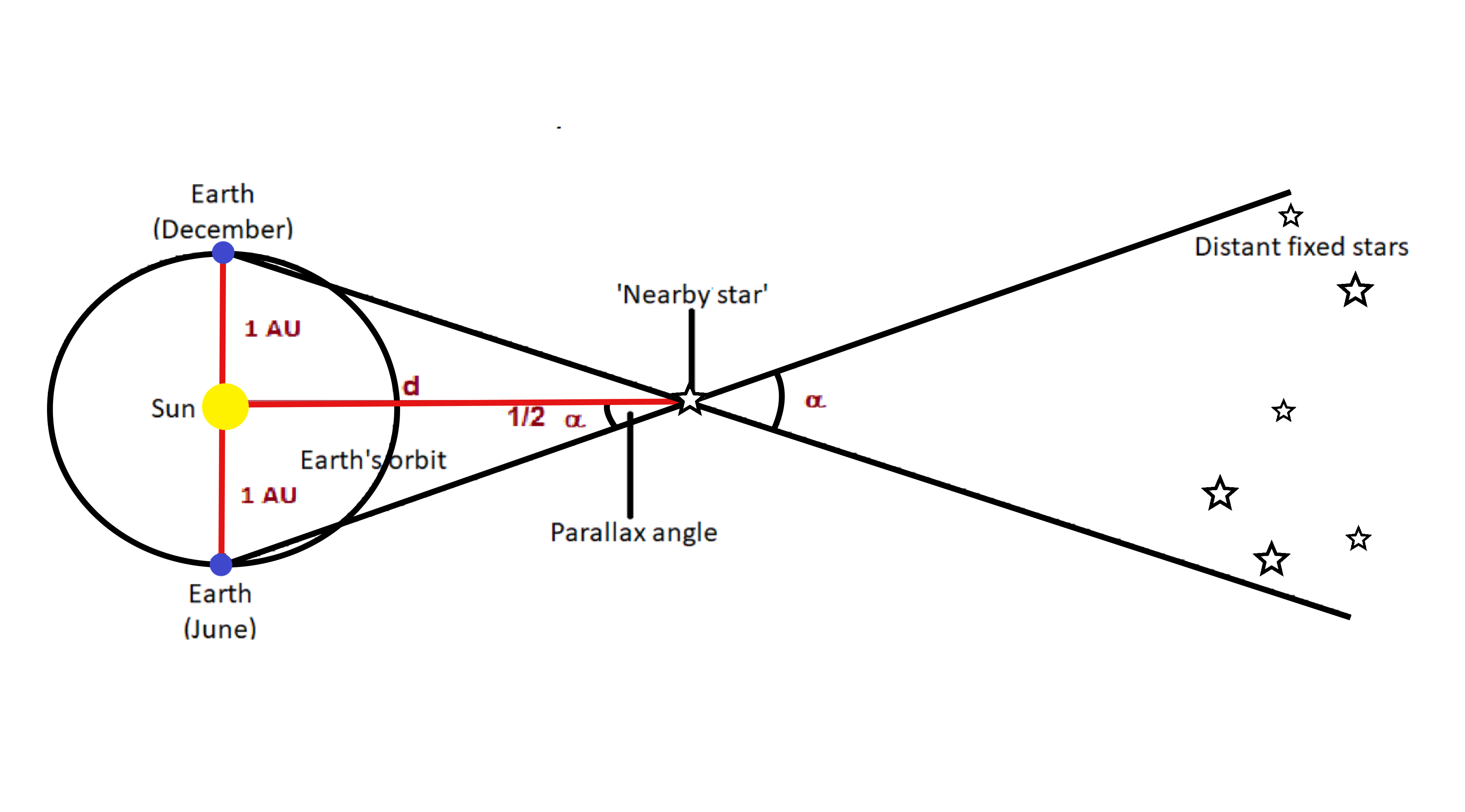
Give the equation for the distance from the Earth to a star, given a small angle of parallax.

Distance to the star ≈ radius of the earth’s orbit / angle of parallax (radians)

d ≈ r / θ

Give the definition of a parsec, and its length in meters.

A star is exactly one parsec away if the angle of parallax is one arcsecond or (1/3600)°

1pc = 3.08 ×10¹⁶ m

Define a lightyear, and its length in meters and parsecs.

The distance an EM wave can travel in one year through a vacuum.

1 ly = 9.46 ×10¹⁵ m

1 pc = 3.26 ly

Define luminosity, giving its unit.

The power output of a star in Watts.

Define light intensity.

The effective brightness of an object. The power output per unit area at the point of observation.

Define apparent magnitude.

The apparent brightness of stars when observed from Earth.

Dependent on the power output of a star and its distance from Earth.

Is magnitude linear or logarithmic?

Logarithmic

Give the definition for magnitude.

A magnitude 1 star has an intensity 100x greater than a magnutide 6 star.

Give the equation relating the intensities of two stars to their apparent magnitudes.

(Intensity of star 1 / intensity of star 2) ≈ 2.51^{(apparent magnitude of star 1 - apparent magnitude of star 2)}

I₂ / I₁ ≈ 2.51^{m₂ - m₁}

Define Absolute Magnitude.

The apparent magnitude of a star if it were 10 parsecs away.

Dependent only on power output.

Give the equation relating absolute and apparent magnitude to the distance of the star away from earth.

apparent magnitude - absolute magnitude = 5 x log (distance from earth in pc / 10)

m - M = 5 x log(d / 10)

Give the definition of a black body.

A theoretical object that absorbs and emits all wavelengths of light perfectly.

Give the curves of black bodies.

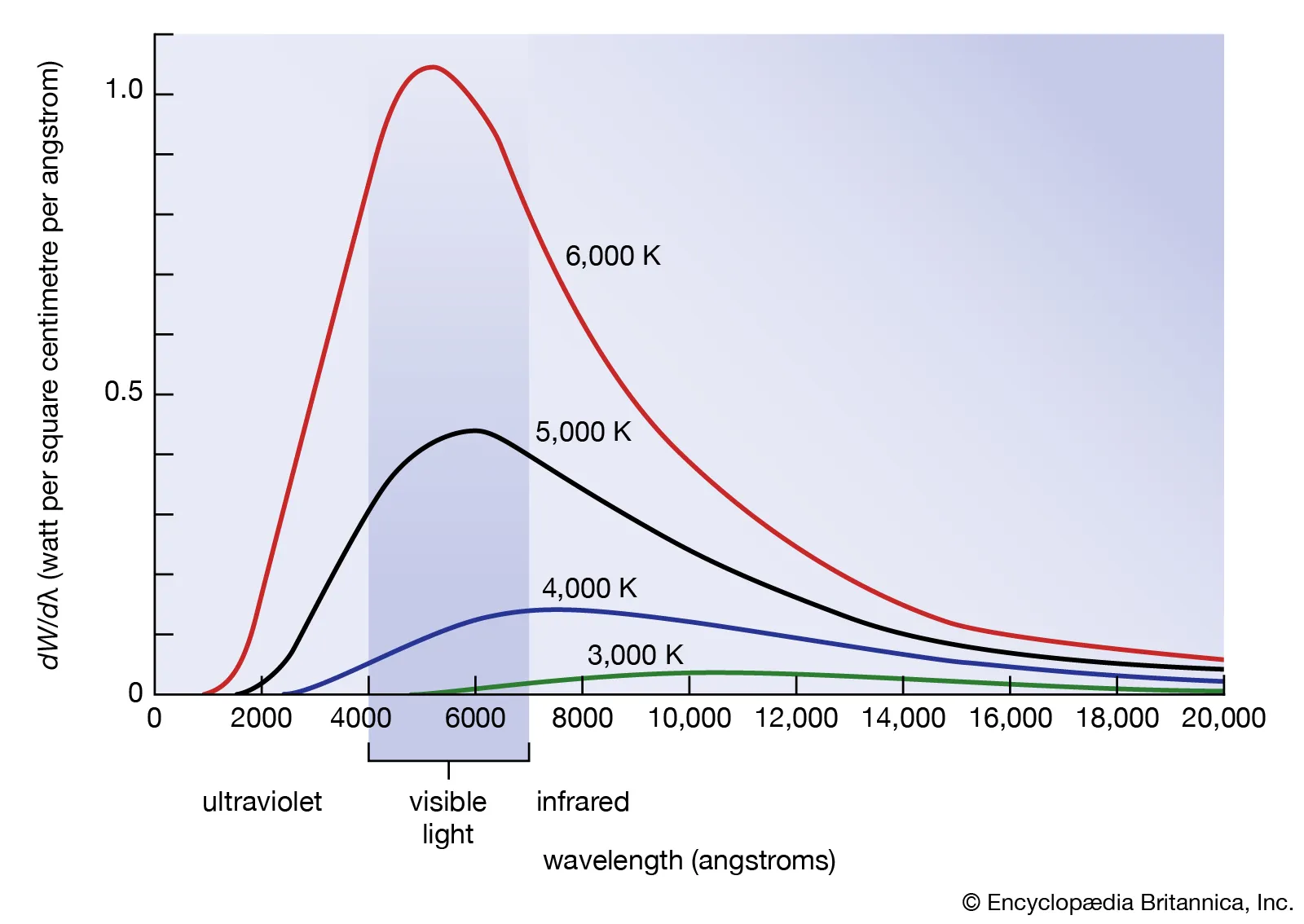
How do we use black body curves with relation to stars?

Stars behave very similarly to black bodies, so we can use black body curves to approximate the emission spectra of stars, and to estimate it’s temperature along with other properties.

Explain Wein’s Displacement Law.

The most prominent wavelength of light is related to the temperature of the star.

Give the equation for Wein’s Displacement Law.

Peak wavelength x temperature = Wein’s constant

λ_max T = 2.9 ×10^-3 Wm

Explain Stefan’s Law.

Relates the power output of a star to its size and surface temperature.

Give the equation for Stefan’s Law.

Power output = Stefan’s constant x surface area x (temperature)⁴

P = σAT⁴, where σ = 5.67 ×10^-8 W m^-2 K^-4

Give the equation for intensity in terms of power output and distance.

Intensity = power output / (4π x distance squared)

I = P / 4πd²

Explain how you can use the light output of a star to get information it.

The light has passed through cooler gas in the form of the star’s atmosphere. By using an absorbtion spectrum, and comparing the absorbtion lines against those for different elements, you can see what the star is fusing.

Fusing of different elements happens at different temperatures, so you can determine its temperature as a result.

Explain the Hydrogen Balmer absorbtion lines.

The wavelengths corresponding to the visble part of hydrogen’s line-absorbtion spectrum, caused by electrons moving between the first exitation level (n = 2) and higher energy levels.

How does the intensity of the absorbtion lines depend on temperature.

For a given absorbtion spectrum, if the temperature is too low, then not many photons have enough energy to be absorbed. If the temperature is too high, then most electrons are in too high an energy level to absorb the photons corresponding to the absorbtion spectrum.

What affects the spectral class of a star?

Only its absorbtion spectrum, and by extension temperature.

Give the seven main spectral classes, along with a mnemonic, range of surface temperatures and the colour a star appears.

Spectral Class	Mnemonic	Surface Temperature (103 K)	Colour
O	Obese	25 - 30	Blue
B	Boys	11 - 25	Blue
A	And	7.5 - 11	Blue / White
F	Fat	6-7.5	White
G	Girls	5 - 6	Yellow / White
K	Kill	3.5 - 5	Orange
M	MacDonalds	< 3.5	Red

Describe the Hertzprung-Russel Diagram.

Stars are grouped into three (or four) areas depending on their stage.

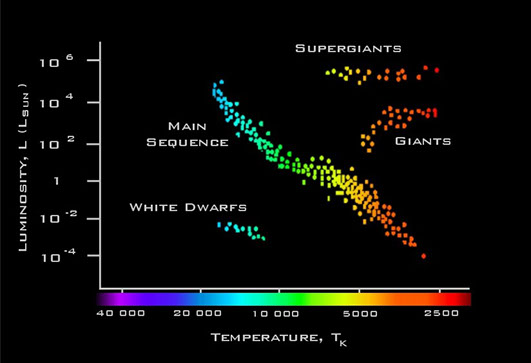
Where are main-sequence stars found on a HR diagram?

In a long diagonal band through the middle of the diagram.

They have a range of temperatures and brightnesses with strong correlation.

Define a main-sequence star.

Any star that fuses only hydrogen into helium.

Where are Red Giants and red supergiants found on a HR diagram?

In a group in the top-right of the diagram. They are generally cool but bright.

Define a red giant and red supergiant star.

Stars which fuse elements other than hydrogen into helium. Whether they are giants or supergiants depends on their mass

Where are white dwarfs found on a HR diagram?

In the bottom left. They have high temperatures but are low in brightness.

Define a white dwarf star.

Stars where fusion has stopped, and the light produced is transferring away whatever energy is left.

How are stars formed?

Clouds of dust and gas (nebulae) contract under the force of gravity into protostars (incredibly hot areas of matter, but not yet undergoing fusion). The protostars continue to contract and heat up, eventually beginning to fuse hydrogen and becoming a main-sequence star.

Explain why a main-sequence star does not expand or contract.

Fusion of Hydrogen into helium produces radiation pressure to prevent further gravitational collapse after its ignition.

What happens when the hydrogen in the core of a main-sequence star runs out?

No radiation pressure is being produced from fusing hydrogen, so the core collapses under gravity. This generates enough heat to begin fusing hydrogen in the star’s shell, releasing energy and causing the star to expand, becoming a red giant.

The helium core continues to contract intil it is hot enough for helium to fuse into carbon and oxygen, releasing a lot of energy and sending the shell even further out.

What happens when red giant stars stop fusing Helium?

No further fusion can happen, as they are too low mass. The core collapses, and the atmosphere is expelled, leaving a planetary nebula with a white dwarf at the centre (from the core).

What do white-dwarfs become when they lose all energy?

Black dwarfs

What happens to red supergiant stars when they stop fusing helium?

They have enough mass to continue fusing elements up to iron.

How does a sun-like star progress through a HR diagram?

Main-sequence (middle), to red-giant, to white dwarf.

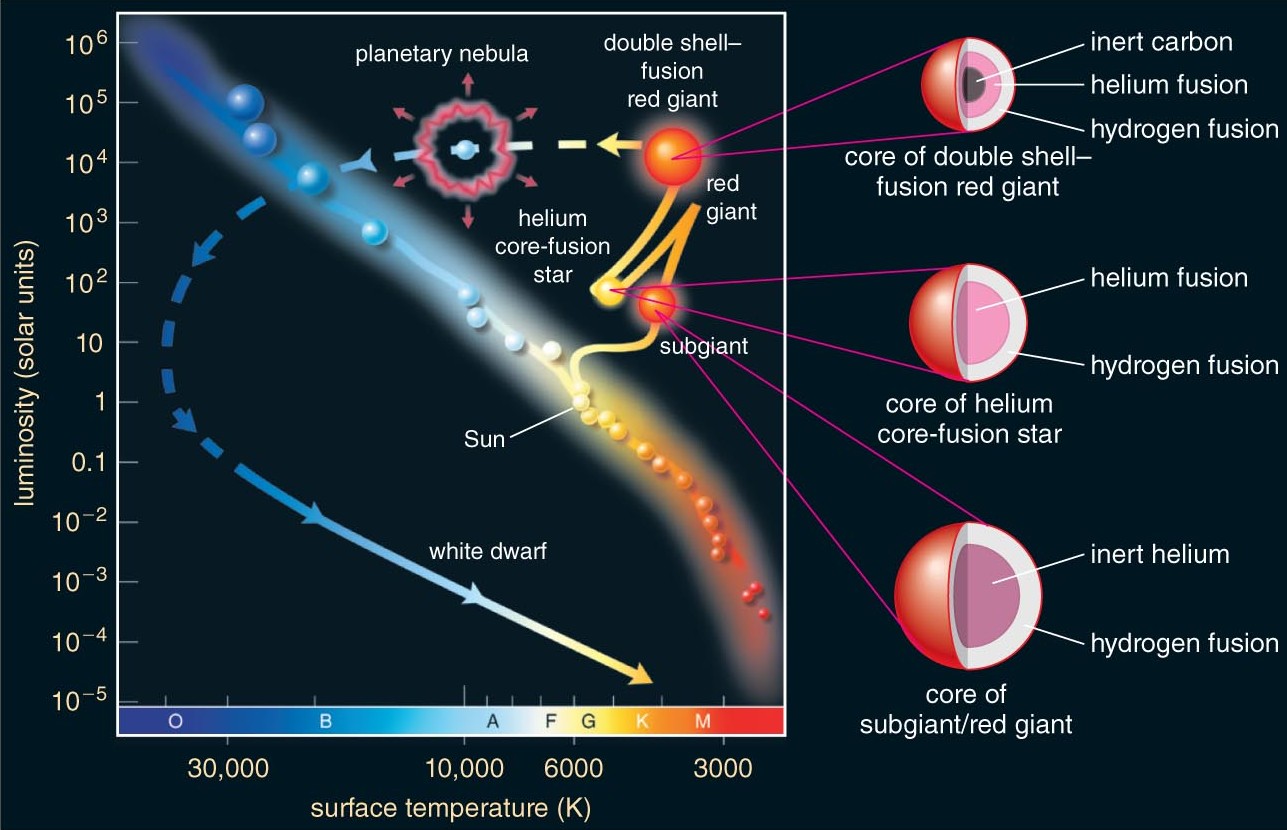
What happens when the core of a red supergiant star has fused up to iron?

The core begins to contract. As it does so, the outer layers fall in also, and rebound off the core causing a supernovae explosion.

What happens to the core of a red supergiant after a supernovae?

If it is between 1.4 - 3 solar masses, then it forms a neutron star.

If it is more than 3 solar masses, then it forms a black hole.

What happens to the brightness of the star during a supernova?

It experiences a brief and rapid increase in absolute magnitude, briefly outshining an entire galaxy, befor fading over a few days or months.

What makes a type I supernova different from a type II supernova?

Type II contain Hydrogen Balmer lines, whereas type I do not.

What makes a type Ia supernova different from a type I.

Type Ia are only formed when a white-dwarf core absorbs enough matter from a binary partner to reach 1.4 solar masses. After this, it will immediately supernova.

As they all have a similar mass, they have identical light curves, with the same peak in absolute magnitude, so they can be used as standard candles.

What is the typical density of a neutron star.

4 ×10¹⁷ kg m^-3

The density is the same as nuclear material.

What do neutron stars emit?

Radio waves from their poles.

What is a pulsar?

A neutron star emitting radio waves such that they appear as pulses of radio waves on earth.

What is the difference between the formations of black holes and neutron stars?

Mass.

A black hole is massive enough that the gravitational force on the core is so great that it collapses into an infinitely dense point at the centre.

Define the event horizon of a black hole.

The area of space around a black hole at which the escape velocity of the black hole’s gravitational field is exactly equal to the speed of light.

Inside the event horizon, not even light can escape a black hole.

Define the Schwarzschild radius.

The radius of the event horizon from the centre of a black hole.

What do astronomers lies at the centre of every galaxy?

Supermassive black holes (10⁶ solar masses).

Give the equation for the Schwarzschild radius.

Radius = 2 x Newton’s gravitational constant x mass of the black hole / speed of light squared

R_s = 2GM / c²

Define the Doppler Effect.

The apparent change in frequency of a wave due to the relative velocity of the reciever and emitter.

What is the percieved change in frequency when the reciever and emitter are moving away?

The frequency appears lower.

What is the percieved change in frequency when the reciever and emitter are moving closer?

The frequency appears higher.

When does red shift occur?

When a luminous body is moving away from earth.

When does blue shift occur?

When a luminous body is moving away from the earth.

What is the change in frequency dependent on?

The relative velocity parallel to the line between the emitter and reciever.

Define recessional velocity.

The relative velocity of an object moving away from us.