Chapter 11 Toolbox
1/12
Earn XP
Description and Tags
Name | Mastery | Learn | Test | Matching | Spaced |
|---|
No study sessions yet.
13 Terms
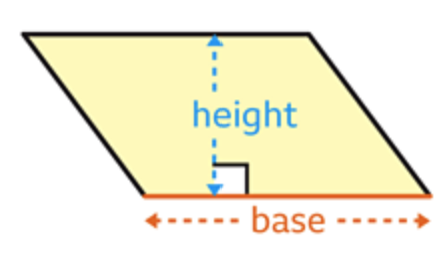
How do we find the area of a parallelogram?
Area of a parallelogram = base × height
How do we find the area of a triangle?
Area of a triangle = (base × height)/2
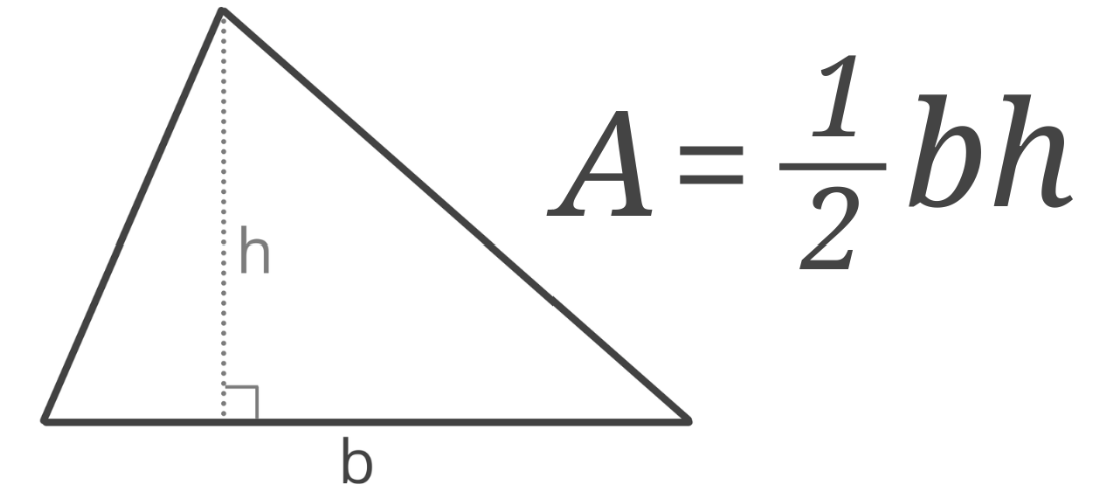
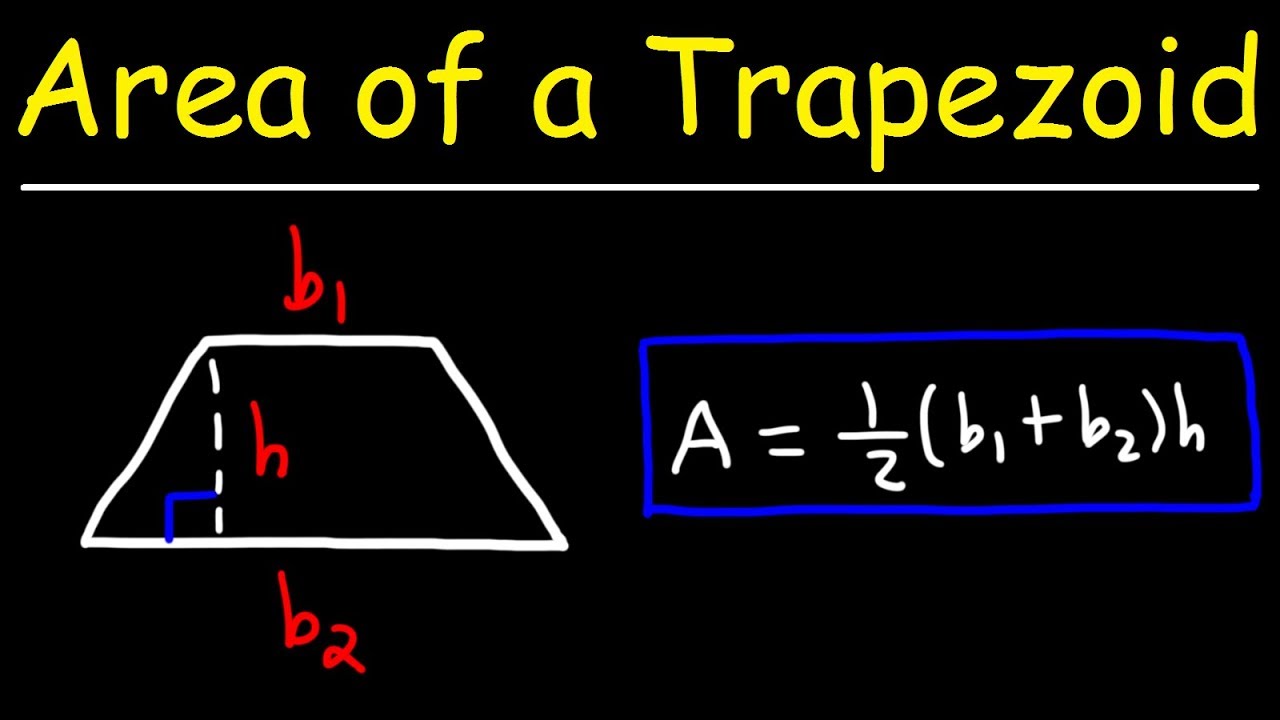
How do we find the area of a trapezoid?
Area of a trapezoid = ½ × height × (base1 + base2)
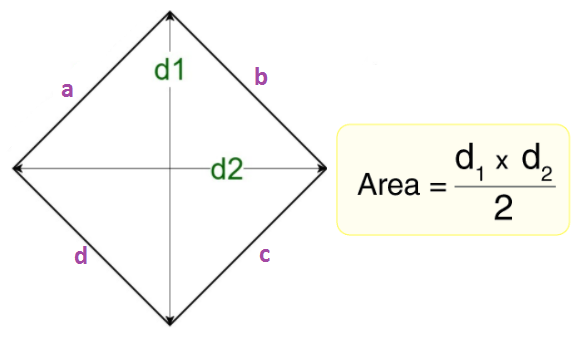
How do we find the area of a rhombus?
Area of a rhombus = ½ × (diagonal1 × diagonal2)
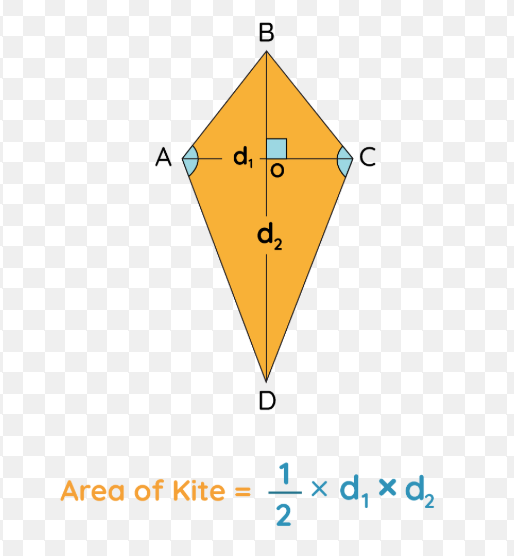
How do we find the area of a kite?
The same way we find the area of a rhombus!
Area of a kite = ½ × (diagonal1 × diagonal2)
How do we find the area of a circle?
Area of a circle = π × radius²

What is a “sector” in a circle?
A sector is a part of the circle defined by two radii and the arc between them. In other words, it is a “slice” of the circle.
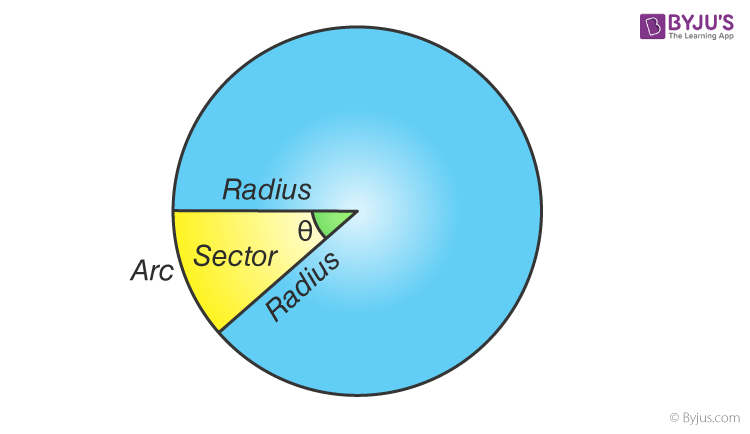
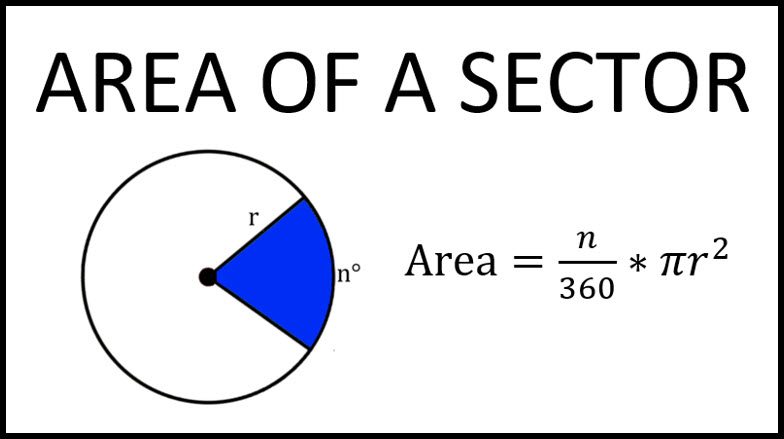
How do we find the area of a circle’s sector?
Area of a sector = πr² × (angle/360)
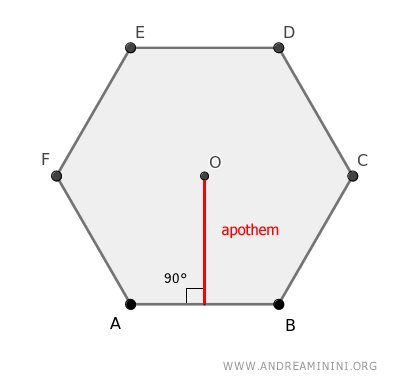
What is an apothem?
A line drawn from the center to the side of a regular polygon.
*Remember, a “regular” polygon has congruent sides and angles.
How do we find the central angle of a regular polygon?
360/n
*n = the number of sides of the polygon
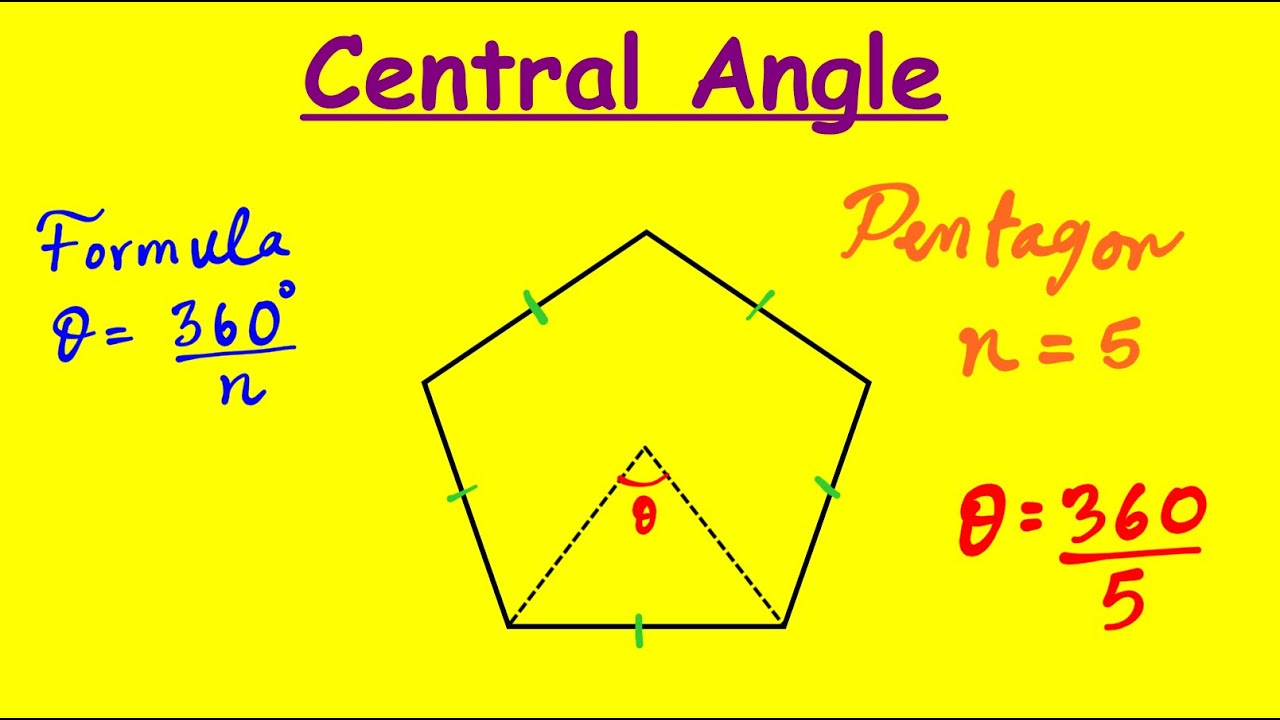
How do we calculate the area of a regular polygon?
A = ½×a×P
*a = apothem
*P = perimeter

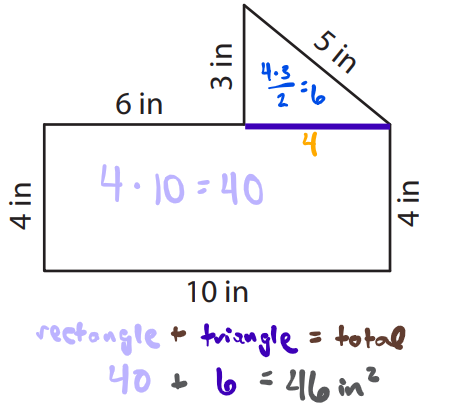
How would I approach finding the area of a figure like this?
Consider dividing the figure up into shapes you KNOW how to find the area of. For example, this shape can be divided into a rectangle and a triangle to find the area.
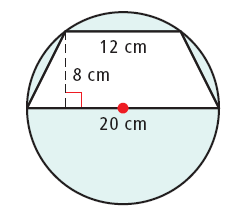
How could we find the area of the green-shaded region?
We could find the area of the circle, find the area of the trapezoid, and then subtract the trapezoid away from the circle.
