test 2 q3

polygon angle formulas

polygon angle formulas

sum of the interior angles: 180(n-2)

measure of an interior angle: [180(n-2)]÷n

measure of an exterior angle: 360/n

axiom (points on a ray)

on a ray, there is exactly one point at a given distance from the endpoint of the ray

median

a segment in a triangle from a vertex to the midpoint of the opposite side

altitude

the perpendicular segment in a triangle from a vertex to tje line that contains the opposite side

perpendicular bisector

a line or ray or segment that is perpendicular to the segment at its midpoint

theorems about perpendicular bisectors/angle bisectors

if a point lies on the perpendicular bisector of a segment, than the point is equidistant from the endpoints of the segment

if a point is equidistant from the endpoints of a segment, then the point lies on the perpendicular bisector of the segment

if a point lies on the bisector of an angle, then the point is equidistant from the sides of the angle

if a point is equidistant from the sides of the angle, then it lies on the bisector of the angle

properties of inequality

either a>b, a<b, or a=b - trichotomy

if a<b and b<c, then a<c - transitive

if a>b then a+c>b+c - addition

if a>b and c>0, then ac>bc - multiplication

if a>b and c<0, then ac<bc - multiplication

theorems about triangle inequalities

if two sides in a triangle are unequal, then the angle opposite the larger side is greater than the angle opposite the smaller side

if two angles in a triangle are unequal, then the side opposite the larger angle is greater than the side opposite the smaller angle

the sum of the lengths of any 2 sides of a triangle has to be greater than the length of the third side

corollaries about triangle inequalities

the perpendicular segment from a point to a line is the shortest segment from the point to the line

the perpendicular segment from a point to a plane is the shortest segment from the point to the plane

theorems about parallelograms

a diagonal of a parallelogram separates the parallelogram into two congruent triangles

opposite sides of a parallelogram are congruent

opposite angles of a parallelogram are congruent

the diagonals of a parallelogram bisect each other

consecutive angles of a parallelogram are supplementary

ways to prove a quadrilateral is a parallelogram

if both pairs of opposite sides of a quadrilateral are congruent, then the quadrilateral is a parallelogram

if one pair of opposite sides of a quadrilateral are both congruent and parallel, then the quadrilateral is a parallelogram

if the diagonals bisect each other, then the quadrilateral is a parallelogram

if both pairs of opposite angles in a quadrilateral are congruent, then the quadrilateral is a parallelogram

theorems about parallel lines

if 2 lines are parallel, then all the points on one line are equidistant from the other line

if 3 parallel lines cut off congruent segments on one transversal, they cut off congruent segments on every transversal

a line that contains the midpoint of one side of a triangle and is parallel to another side passes through the midpoint of the third side

the segment that joins the midpoints of two sides of a triangle is parallel to and half the measure of the third side

rectangle definitions

a rectangle is a quadrilateral with four right angles

a rectangle is a parallelogram with a right angle

rhombus definitions

a rhombus is a quadrilateral with four congruent sides

a rhombus is a parallelogram with a pair of consecutive sides congruent

square definitions

a square is a quadrilateral with four right angles and four congruent sides

a square is a rectangle with congruent adjacent sides

a square is a rhombus with four right angles

a square is a parallelogram with four congruent sides and four right angles

rectangle theorem

the diagonals of a rectangle are congruent

right triangle theorem

the midpoint of the hypotenuse of a right triangle is equidistant from the three vertices

rhombus theorems

the diagonals of a rhombus are perpendicular

each diagonal of a rhombus bisects two angles of the rhombus

the area of a rhombus = 1/2 • (m. 1st diagonal) • (m. 2nd diagonal)

trapezoid definitons

trapezoid - a quadrilateral with exactly one pair of parallel sides

isosceles trapezoid - a trapezoid with congruent legs

median of a trapezoid - the segment that joins the midpoint of the legs

trapezoid theorems

base angles of an isosceles trapezoid are congruent

the diagonals of an isosceles trapezoid are congruent

the median of a trapezoid is parallel to the bases and has a length equal to the average of the base lengths

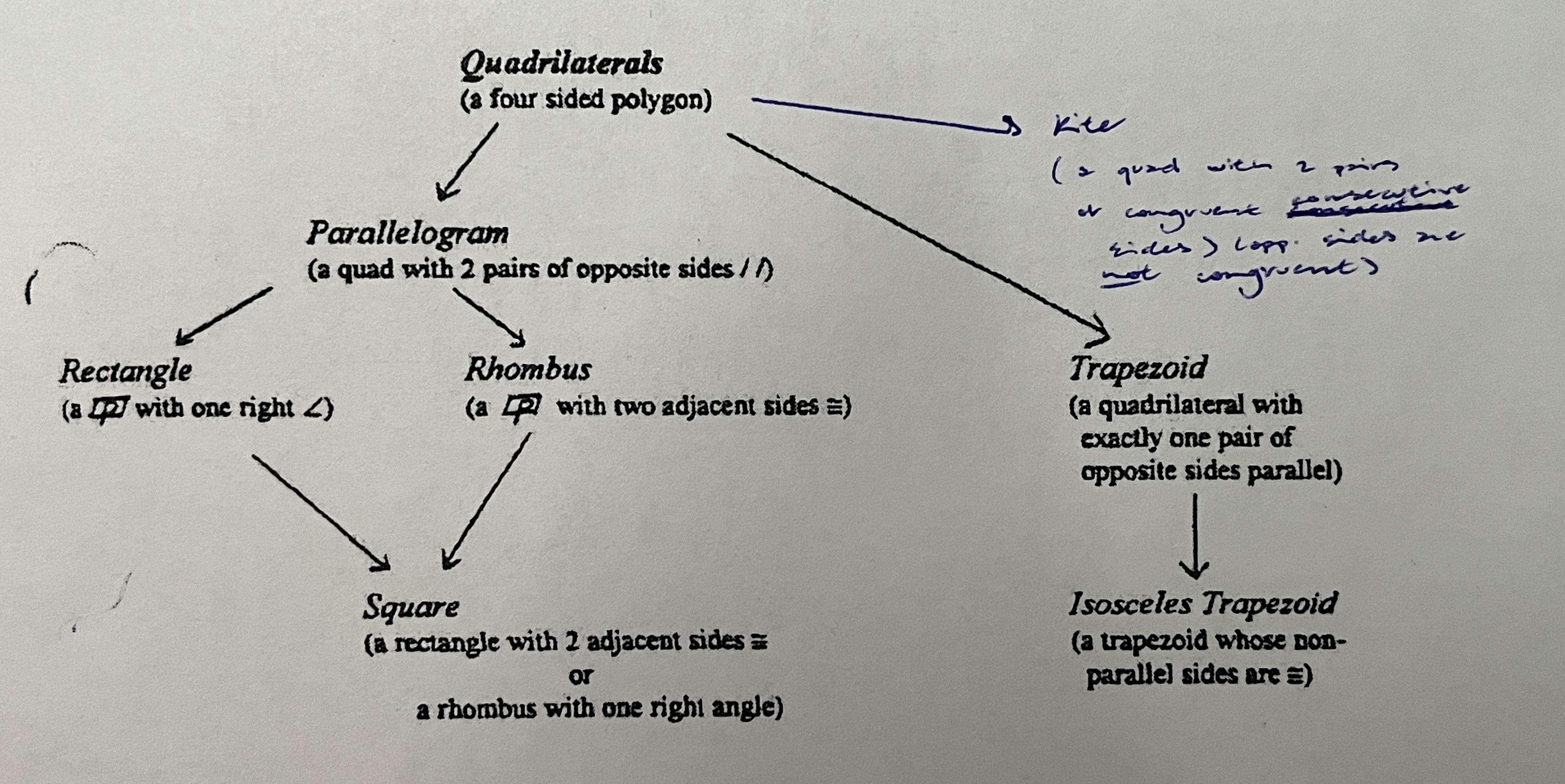
hierarchy of quadrilaterals