Midterm Review

Significant Figure Rules

estimate one sig fig past smallest division on measuring device; every non-zero digit is significant; zeros between non-zeros are significant; leftmost zeros in front of non-zeros are not significant; zeros past a number to the right of a decimal are significant; zeros before understood decimals are not significant; zeros before indicated decimal are significant; counts and exact quantities are significant

Length Units

SI- meter (m)

CGS- centimeter (cm)

BE- foot (ft)

Mass units

SI- kilogram (kg)

CGS- gram (g)

BE- slug (sl)

Time units

SI- second (s)

CGS- second (s)

BE- second (s)

Adding and Subtracting Sig Figs

retain as many places to the right of the decimal as the original number with the least places after the decimal

Multiplying and Dividing with Sig Figs

retain as many sig figs as the original number with the least

Scientific Notion

shorthand for writing really big or really small numbers; * 10^number

Scalars

quantitiy that only needs a magnitude (number with unit)

Scalars examples

time, temperatuer, mass, length, speed, distance

vectors

a quantity that needs a magnitude (number with a unit) and a direction to be complete

vectors examples

force, velocity, acceleration, displacement

resultant

R with arrow on top; result of combining two or more vectors

How do you calculate the resultant?

use a graph method by drawing the each vector and placing the tail of each next to the head of the previous; measure the angle and resultant OR vector component method to find x-component and y-component and using Pythagoras theorem to find resultant

equilibrant

R_E with arrow on top; vector equal in magnitude to, but in the opposite direction of, the resultant

How do you calculate the equilibrant?

keep same magnitude of resultant and put in opposite direction; ex. south of East turns into North of West

Kinematics

study of motion

Speed

rate of change in distance; scalar quantity; no particular direction can be assigned; d/t

Distance

length between objects or points without regard to direction

Displacement

s; distances that have a specific direction; sign indicates direction of displacement (- is left or down)

Displacement Unit

SI- m

CGS- cm

BE- ft

Velocity

v; rate of change in displacement; vector quantity; NEED to specifiy a direction

Velocity Units

SI- m/s

CGS- cm/s

BE- ft/s

Instantaneous Velocity

rate of change in displacement (or distance) at a given instant

Acceleration

rate of change in velocity; vector quantity; points in direction of the CHANGE in velocity (speeding up and slowing down)

Acceleration Units

SI- m/s²

CGS- cm/s²

BE- ft/s²

Kinematics equation for constant acceleration

Position vs. Time Graphs

The slope is equal to velocity; If linear, v is constant (i.e. no acceleration); If linear and horizontal the object is stationary (i.e. the speed is zero); If linear, horizontal, and along the x-axis, the object is stationary at the origin; If the graph is not linear, the object’s velocity is changing. (i.e. the object is accelerating)  The slope of the tangent line is equal to instantaneous velocity

Velocity vs. Time Graphs

The slope is equal to acceleration; If linear, a is constant (i.e. constant acceleration); If linear and horizontal the object is not accelerating (i.e. the speed is constant); If linear, horizontal, and along the x-axis, the object is stationary; If the graph is not linear, the object’s acceleration is varying; The slope of the tangent line is equal to instantaneous acceleration

Free Fall

object falls accelerating only because of gravity; outside forces like air resistance are neglected

Acceleration due to Gravity

Everything is accelerated towards earth at the same constant rate.  For every second of freefall, an object speeds up 9.80m/s (usually negative); AP CB uses 10m/s²

Variables for two dimension kinematics

components of variables with x and y subscripts

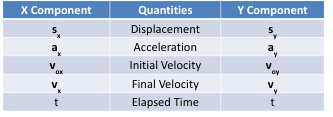
How do you use trig to resolve quantities into components?

use trig (cos and sin) to split the quantity into components; solve for opposite and adjacent

Projectiles

any object that moves through air or space and is acted only by gravity (neglect air resistance/wind); a_x = 0 m/s² and a_y = -9.80 m/s²

Horizontal Projectile

a projectile that is launched with no initial upward angle; ex: rolling an object off of a table; v_oy = 0 m/s

Horizontal Projectile mathematically manipulate

know that a_x = 0 m/s², v_oy = 0 m/s, and a_y = -9.80 m/s²; use equations to solve

Vertical Projectile

a projectile that is launched with an initial upward angle; ex: cannonball being fired; has v_ox and v_oy

Vertical Projecile mathematically manipulate

know a_x = 0 m/s² and a_y = -9.8 m/s²; usually will be given angle and initial velocity or just the initial velocity components; use equations to solve

Relative Velocity frame of reference

the velocity of an object is relative to the observer

Relative velocity 1-D situations

v_pg = v_pe + v_eg; add if going in the same direction; make one negative if going in different directions

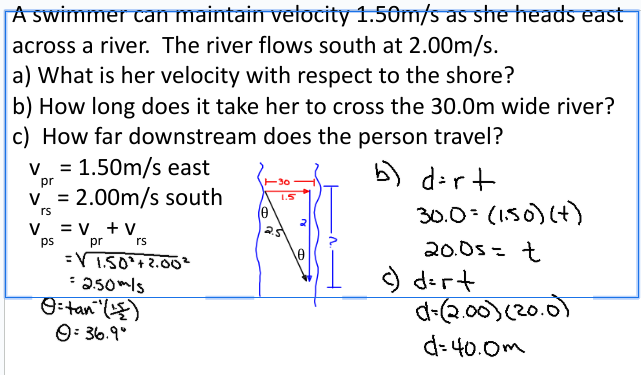
Relative Velocity 2-D situations

v_ps = v_pr + v_rs; need to use pythagorean theorem and have angles to solve using equations

Forces

pushes or pulls on an object

Contact Forces

there needs to be contacts for the force to cause motion; ex: friction, lift, air resistance

Non-Contact Forces

no contact is necessary for motion to occur; ex: gravity, magnetism

Newton’s 1st Law of Motion name

Law of Inertia

Newton’s 1st Law of Motion stated

an object in motion will remain in motion, an object at rest will remain at rest, unless acted upon by a net external force

Newton’s 1st Law of Motion net force

ΣF; vector sum of all forces

Newton’s 1st Law of Motion mass

intrinsic value that is a quantitative measure of inertia (doesn’t change based on where we are)

Newton’s 1st Law of Motion Inertia

tendency for an object to remain in its state of either motion or rest

Newton’s 2nd Law of Motion name

Law of Acceleration

Newton’s 2nd Law of Motion Stated

when a net external force, ΣF, is applied to a mass, m, it imparts acceleration a; ΣF=ma = kg*m/s² = Newton (N)

Newton’s 2nd Law of Motion units

Si- kg, m/s², Newton

CGS- gram, cm/s², dyme (dyn)

BE- slug, ft/s², lb

Newton’s 2nd Law of Motion Free Body Diagram

diagram that represents the forces acting on a single object; place a dot to represent the object, construct an xy axis with the dot as the origin, and draw and label forces using size appropriate arrows

Newton’s 3rd Law of Motion name

Law of Reciprocal Actions

Newton’s 3rd Law of Motion stated

when one body exerts a force on another body, the second body exerts a force equal in magnitude but opposite in direction to first

Newton’s 3rd Law of Motion examples

swimming

Newton’s Law of Universal Gravitation stated

every particle in the universe exerts an attractive force on every other particle based on F_g= (G*m₁m₂)/r²

Newton’s Law of Universal Gravitation equation

F_g = (G * m₁m₂)/r²; F_g- force due to gravity; G- universal gravitational constant; m₁- mass of object 1; m₂- mass of object 2; r- distance between the object’s centers

Universal Gravitational Constant

6.67 × 10^-11 Nm²/kg²

Significance of [G(m/r²)]

acceleration due to gravity; determines the gravity of a planet; is F_g = m * g when objects are close to a planet’s surface

Pound → Newton

1 lb → 4.45N

Kilogram → Newtons

kg * gravity

Normal Force (F_N)

a component of force that a surface in contact with something exerts perpendicular to itself; opposite of gravity; without it we would accelerate downwards; if resting on horizontal, non-accelerating surface, F_N = Fg

Apparent Weight

weight displayed that is different than F_g that arises from either acceleration or a non-horizontal surface; F_N = F_g + F_applied = mg + ma; ex: elevator

Friction

component of force that acts parallel to a surface and opposes motion

Static Friction definition

F_s; force that must be overcome to set an object into motion; depends on coefficient of static friction and amount of Normal Force present

Static Friction equation

F_smax = μ_s F_N

Static Friction coefficient

μ_s; dependent on surfaces in contact

Kinetic Friction definition

F_K; force that must be overcome to keep an object in motion; depends on coefficient of kinetic friction and amount of Normal Force present

Kinetic friction equation

F_k= μ_KF_N

Kinetic Friction coefficient

μ_K; dependent on surfaces in contat

Friction on an incline Fg ≠ F_N

F_N = F⊥

Fperpendicular (⊥)

= Fg cos()

Fparallel (∥)

=Fgsin()

sum of forces (ΣF) in equilibrium

ΣF = 0N; the object is either stationary or moving at a constant velocity

Acceleration in equilibrium

a = 0m/s²; the object is either stationary or moving at a constant velocity

sum of forces (ΣF) in non-equilibrium

ΣF ≠ 0N; the object is accelerating or decelerating

acceleration in non-equilibrium

a ≠ 0m/s²; the object is accelerating or decelerating

work

done when a force (F) creates a displacement (s)

work in same direction equation

W = Fs or W = ∆K

work in different directions equation

W = F_lls; W = Fcos(angle)s

work units

SI- J = N * m

CGS- erg = Dyne * cm

BE- ft * lb

work energy theorem

when work is done on something, its kinetic energy changes

work energy theorem equation

W = ΔK; derived from F = ma where a is replaced by (v²-v_o²)/2s

kinetic energy

energy of motion

kinetic energy equation

K = ½ m v²

kinetic energy unit (SI)

J

gravitational potential energy

U; energy of position (energy based on a position above a reference level)

gravitational potential energy equation

U = mgh

gravitational potential energy unit (SI)

J

gravitational potential energy is related to work done by

gravity; Wg = -ΔU becomes Wg = mgh0 - mgh

spring force

Hooke’s Law; force that opposes the stretching or compressing (restoring force)

spring force equation

F_spring= -k∆x; k is spring constant and ∆x is change in length

spring force unit

N

spring constant

k; changes based on the spring

spring constant unit

N/m

spring potential energy

equal to the work done by spring; W = F * d changes into W = ½kx * x and then to U_spring = ½ k x²

mechanical energy

E, the sum total of kinetic and potential energy

mechanical energy equation

E = U + K

conservative force

a force that does not net work if there is no displacement; ex: gravity

conservation of mechanical energy

if there is no non-conservative work done, mechanical energy is conserved; used for finding final or initial heights or speeds