Paper 2 PMT Flashcards

Hard

Can scratch or indent, and withstands being scratched.

Brittle

Breaks without plastic deformation

Ductile

Can be drawn into a wire

Malleable

Can be reshaped

Elastic

Returns to its original shape after being deformed.

Plastic

Does not return to its original shape after being deformed

Strong

Withstands large static loads without breaking

Tough

Withstands large dynamic loads without breaking

Stiff

Resists deforming by tension or compression.

difference between strong and tough materials?

Strong materials can withstand large static loads whilst tough materials can withstand large dynamic loads

What is tensile/ ultimate tensile strength?

Tensile strength is the force that is used to stretch a sample at any time while the Ultimate Tensile Strength is the force required to break it.

Calculating the volume of an object in order to find its density can be done in two main ways.

1. For regular shapes, you can measure the dimensions required and then apply a standard formula for the volume of the given shape.

2. For irregular shapes, you need to use a submersion method, where you measure the volume of water that is displaced when the object is submerged into a beaker of water.

A ball moving through a fluid will always experience a drag force. This force resists the motion f the object. The magnitude of this force on the ball can be calculated using stokes' law.

The equation for Stokes' law is : F = 6πηrv

Viscosity is a quantity that depends on the surface of the ball and the liquid that it is moving through. It is also temperature dependent.

Tensile Stress is the force applied per unit cross-section area, measured in Pa or Nm^-2

Tensile Strain is the ratio of extension to original length. It has no unit.

A brittle material fractures without showing any plastic behaviour(very little extension).

A ductile material can be stretched into long wires and stays permanently stretched.

What is the density of a material?

The density of a material is its mass per unit volume

State the equation used to calculate density

Density = mass/volume

What is the unit of density?

kg/m³

When an object is submerged in a fluid, what determines the upthrust it experiences?

The upthrust of a submerged object is equal to the weight of the fluid that it displaces.

What determines whether an object floats or sinks?

The balance between the weight and upthrust of the object. If the weight exceeds the upthrust, the object will sink.

Why will a uniform object with a density greater than that of the liquid it is submerged in, always sink?

- The upthrust of the object will be equal to the weight of the liquid displaced

- Therefore, the maximum upthrust will be equal to the density of the fluid x volume of object x gravitational field strength

- The weight of the object is equal to the density of the object x volume of object x gravitational field strength

- If the object's density is greater, the weight will always be greater than the upthrust and the object will sink

What shaped objects does Stokes' Law apply to?

Stokes' law only applies to small spherical objects.

What type of flow is required for Stokes' Law to apply?

Laminar Flow

What does Stoke's Law allow you to calculate?

The viscous drag force that a small spherical object experiences when falling at low speeds through a viscous fluid with laminar flow.

State the Stokes' Law equation

- F = 6πηrv.

- η is the viscosity of the fluid.

- r is the radius of the sphere.

- v is the speed of the sphere.

What is elastic deformation?

An object has undergone elastic deformation if it returns to its original shape once the deforming forces are removed.

What is plastic deformation?

An object has undergone plastic deformation if it no longer returns to its original shape once the deforming forces are removed. It will have permanent deformation.

Express Hooke's Law in words.

The extension of an elastic object is directly proportional to the force that is applied to it , up to its limit of proportionality.

What is the limit of proportionality?

The point beyond which the force and extension will no longer be directly proportional to each other - Hooke's Law is no longer obeyed.

What is the elastic limit?

The point beyond which the object will no longer elastically deform, and will instead deform plastically.

State the defining equation of Hooke's Law

F = kΔx

F = force applied (N)

K = stiffness constant

ΔX = extension (m)

What is mechanical stress?

The force experienced by an object per unit area.

State the equation for stress.

- Stress = F/A.

- F is the force applied.

- A is the cross-sectional area.

What is the unit of stress?

Pascal (Pa) or N/m^2

State the equation for strain

Strain = ΔL / L

ΔL is the change in length

L is the original length

What is the unit of strain?

Strain is a unitless quantity because it is the ratio of two lengths.

What does the Young Modulus of a material tell you?

A material's Young Modulus is a measure of how much force is required for a given extension, regardless of the object's dimensions.

What equation is used to calculate a material's Young Modulus?

Young Modulus = Stress/Strain

What unit is used for a material's Young Modulus?

Nm⁻⁻²

What is breaking stress?

Breaking stress is the the maximum stress that an object can withstand before fracturing.

What is the yield point?

The point beyond which the object will experience a large extension without substantial increase in the force applied.

What type of energy is stored in an object that has been stretched?

Elastic Potential Energy

State two equations used to calculate the energy stored in a spring.

E = ½ FΔx

E = ½ kΔx²

What does the gradient and area represent on a force-extension graph?

- The gradient of the linear region represents the elastic constant (k)

- The area is equal to the elastic potential energy stored in the spring

- Displacement

- Amplitude

- Wavelength

- Frequency

- Wave Speed

- The distance and direction that a particle has travelled from the equilibrium position.

- Maximum displacement of a vibrating particle.

- Shortest distance between two particles in phase.

- Number of wave cycles occurring each second.

- Distance travelled by a wave each second

- Phase difference

- Path difference

- Progressive waves

- Wavefront

- Electronvolt

- Measured in degrees or radians, the amount by which one wave lags behind another wave

- Measured in meters, the difference in the distance travelled by two waves.

- Waves whose oscillations transfer energy

- The energy gained by one electron when passing through a potential difference of 1 volt. This is equal to 1.6x10-16

- Transverse Waves

- Longitudinal Waves

- Transverse waves are waves whose oscillations are perpendicular to the direction of propagation of energy e.g. electromagnetic waves

- Longitudinal waves are waves whose oscillations are parallel to the direction of propagation of energy. They consist of compressions and rarefactions e.g. sound waves

- Glare and cameras - Polarization can be used in things such as polaroid sunglasses or in a camera to enhance the image.

- Only transverse waves can be polarized, which is where all the waves oscillate in the same plane. The discovery of polarized light helped prove that light was a transverse wave.

Radio signals - TV and radio signals are polarized by the direction of the rods on the transmitting aerial. To receive these signals well, you must ensure the receiving aerial and the waves are in the same plane.

Speed of wave

The speed of a wave is always equal to the product of its frequency and wave length.

For an EM wave, this value is always equal to c, the speed of light in a vacuum.

This can be expressed as the following equation:

v = ƒλ

In order to calculate the speed of a transverse wave on string you can use the equation:

v = √(T/µ)

where:

T= tension on the string

µ= mass per unit length of the string

Super position is where the displacements of two waves are combined as they pass each other. The total displacement at a point is equal to the sum of the individual displacements at that point.

You should know that waves:

- Constructively interfere when they are in phase with each other.

- Destructively interfere where they are in antiphase with each other (180 degrees out of phase)

Phase, Constructive and Destructive interference can be explained in terms of peaks and troughs

- When the waves are in phase, two peaks or two troughs will constructively interfere with each other, resulting in a 'double' peak or trough being created. - - When waves are in antiphase, a peak will meet a trough and result in destructive interference, which is where they cancel each other out and produce a minimum point

A stationary wave is one that stores energy instead of transferring it from one point to another.

The process of a stationary wave being formed on a string that is fixed at both ends:

1. A wave is generated at one end of the string and travels down it.

2. At the other end, this wave is reflected and travels back in the opposite direction.

3. The frequency of wave generation and the length of the string are such that the next wave generated meets this reflected wave and undergoes superposition

4. At places where the two waves are in phase, they undergo constructive interference and form a maximum point known as an antinode.

5. At places where the two waves are in antiphase, they undergo destructive interference and form a minimum point known as a node.

The fundamental frequency of a wave on a string can be found from the equation :

ƒ= 1/2l √(T/µ)

T = mg

µ = M/l

- From the equation, we can see that raising the tension or shortening the length of a given string increases the frequency/pitch

- Fundamental frequency - The oscillation of an entire object forming the lowest possible frequency for that object. For a string fixed at both ends this is where there is only a single antinode in the middle of the string.

Diffraction is the spreading out of waves when they pass through a gap or over an edge. It depend on the gap width and the wavelength of the wave.

If the gap:

- Is a lot bigger than the wave length, the diffraction is unnoticeable.

- A bit wider than the wavelength, the diffraction is noticeable.

- The same size as the wavelength, the diffraction most noticeable.

- Smaller than the wavelength, most of the waves are reflected.

Huygens' principle states that every point on a wave front is a point source to secondary wavelets, which spread out to form the next wave front.

Huygens' construction which is based on this principle, can be used to show what happens when a wave meets an obstacle and experiences diffraction, as shown below.

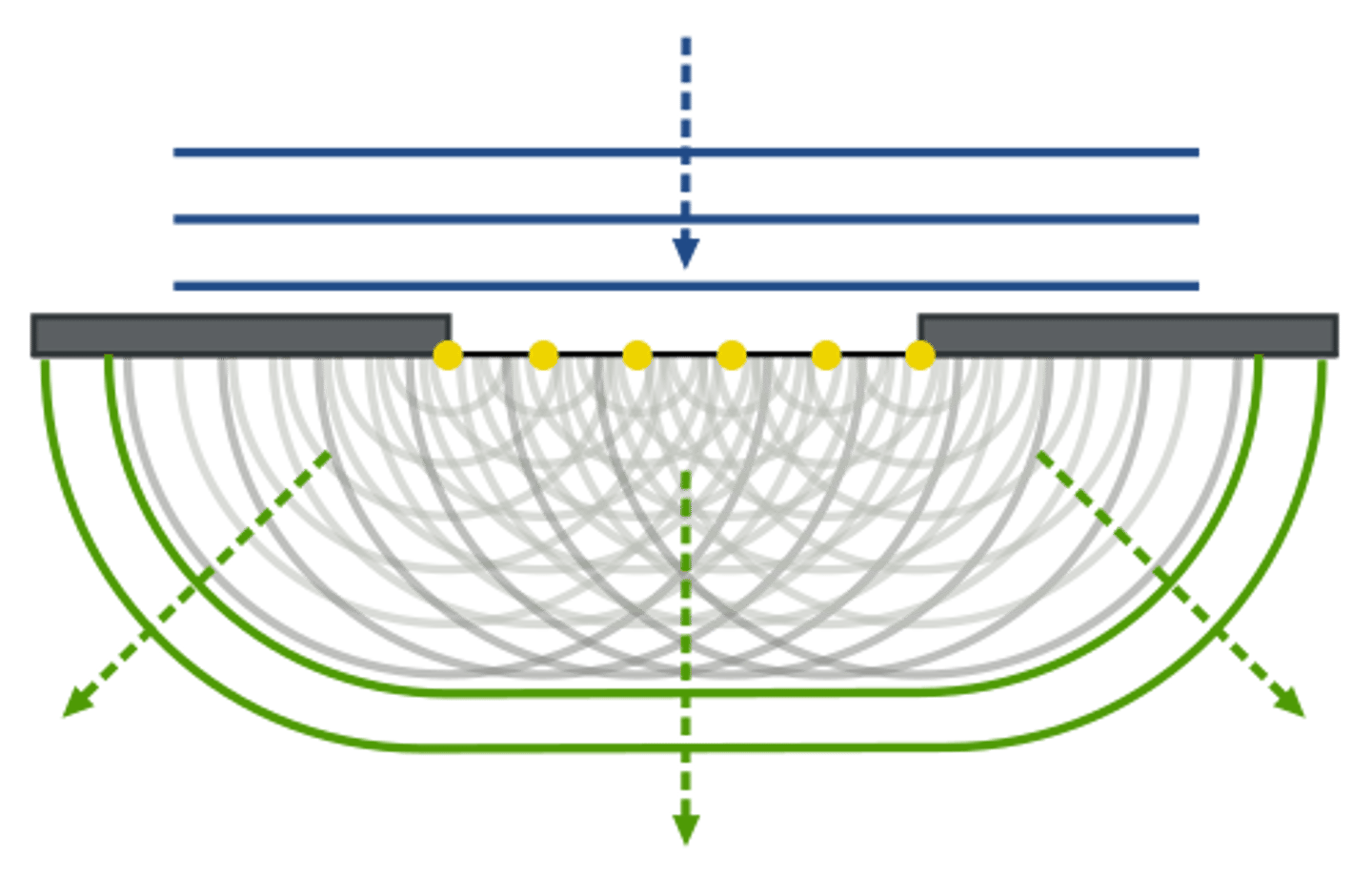
Diffraction grating-

•Diffraction can be demonstrated by shining light through a diffraction grating. You can use the following equation when using a diffraction grating:

nλ = dsinθ

•Depending on the type of light passed through a diffraction grating, the diffraction pattern will vary. Monochromatic light will form a pattern of alternating light and dark fringes, while white light will form a white central fringe and alternating bright fringes which are spectra.

- Intensity (I) is a measure of the power delivered per unit area.

I= P/A

- Diffraction is purely a wave property. Diffraction grating experiments show that light can experience diffraction, providing evidence for the wave nature of light.

• The de Broglie hypothesis states that all particles have a wave-like nature and a particle nature, and that the wavelength of any particle can be found using the following equation:

λ = h/p

• Electron diffraction provided experimental evidence for the de Broglie hypothesis as it showed that electrons, which are particles, can also undergo diffraction, which can only be experiences by waves. This provided evidence for the wave-like nature of electrons.

• When accelerated electrons passed through a crystal lattice, they interacted with the small gaps between atoms and formed a diffraction pattern

• If electrons acted only as particles, you would expect the diffraction pattern to consist of a single bright spot where the electrons passed through the gap, but this was not the case.

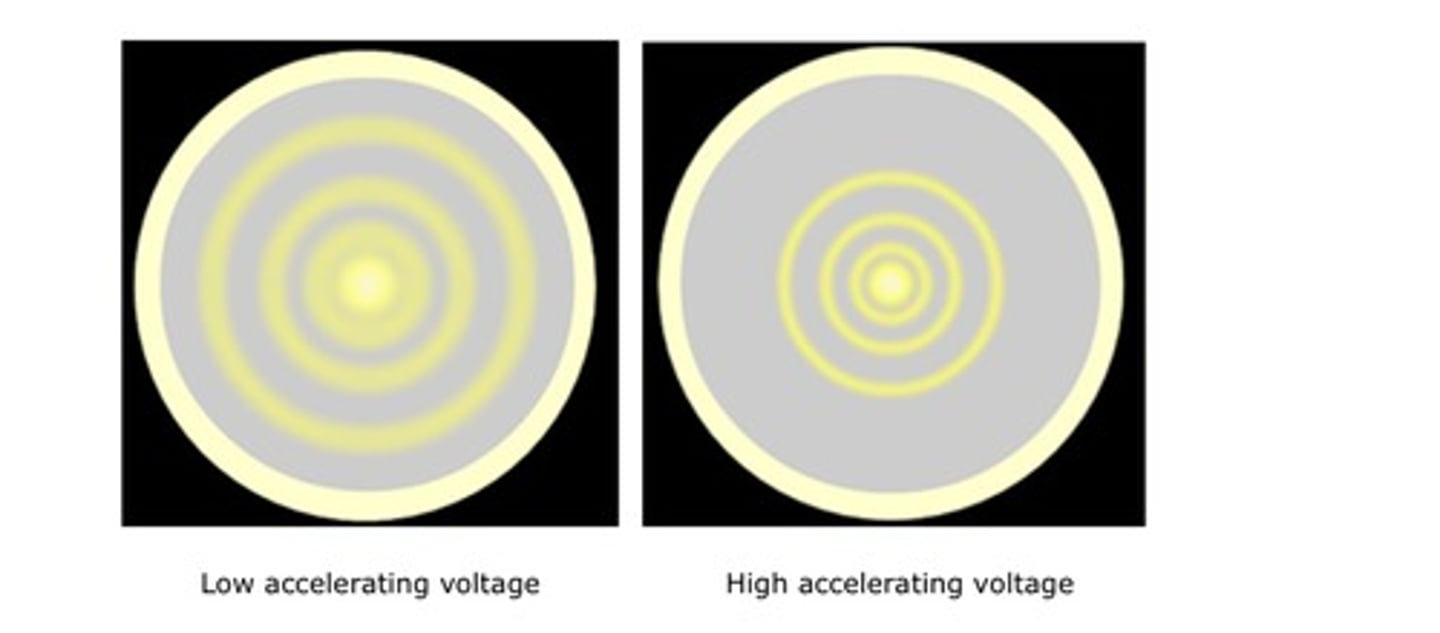
Young's Double Slit Experiment - When two double slits are illuminated, the two slits act as coherent wave sources.

Evidence for the Wave Nature of EM radiation - Diffraction and interference are purely wave properties, so this experiment showed that EM radiation has wave properties

Coherence means the waves have the same frequency with a constant phase difference. The light diffracts at the slits and the two waves superpose, forming an interference pattern. This is because a combination of a constructive and destructive interference occurs.

Refraction is when a wave changes speed when it crosses into a new medium:

•If the medium is more optically dense, the wave will slow down and bend towards the normal

θᵢ>θᵣ

•If the medium is less optically dense, the wave will speed up and bend away from the normal

θᵢ<θᵣ

• A measure of how optically dense a medium is, is the material's refraction index:

• The absolute refractive index of a material measures how much it slows down light. It is a ration

n=c/v

•The relative refractive index at the boundary between two materials is a ratio of the two materials

₁n₂ = C₁/C₂

•It is possible to calculate the refractive index from the angles of incidence and refraction, or to predict the angles of refraction for a given angle of incidence, using Snell's law:

•Snell's law states that: n₁sinθ₁=n₂sinθ₂

• Using the fact that the refractive index of air is approximately 1, you form the following equation for the critical angle (C) where one of the mediums being passed through is air:

sinC = 1/n

This can then be used to form the equation used to calculate the critical angle for a given material. The critical angle is the angle of incidence at which the refracted ray just passes along the boundary line, and beyond which the wave will be totally internally reflected.

n₁sinθ₁=n₂sinθ₂

n₁sinθ₁=n₂sin90

n₁sinθ₁=n₂x1

sinθ₁=n₂/n₁

where n₁>n₂

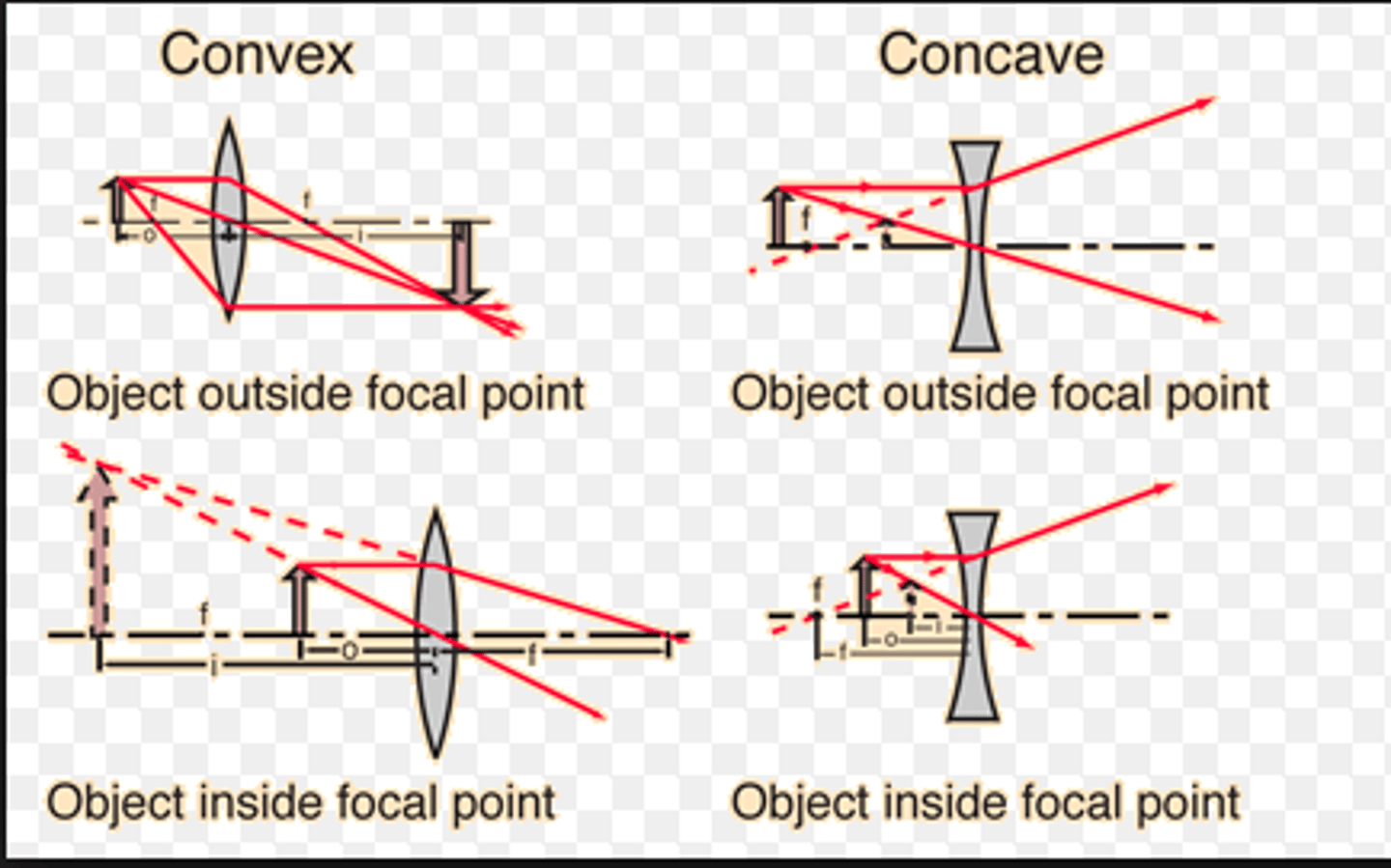
Lenses

• The focal point (F) of a lens is the point at which the rays of light converge or appear to converge.

• The focal length (f) of a lens is the distance from the center of the lens to the focal point

• Power of a Lens : P=1/ƒ

Lenses can produce two different kinds of image:

1. A real image is one in which the rays of light actually converge to produce an image that can be projected onto a screen

2. A virtual image is one in which the rays of light only appear to have converged. Virtual images cannot be projected onto a screen.

You can use ray diagrams in order to map where an image will appear after passing through a lens.

To draw a ray diagram:

1. Draw two lines from the same point of an object (e.g. the top), one which passes through the center of the lens and is left unrefracted and one which moves parallel to the principle axis and passes through the focal point (F).

2. If the image is real, it will form where the two lines meet, If it is virtual, it will appear where the two lines appear to come from - this can be found by drawing a dashed line backwards from both of the initial lines and finding the point they meet.

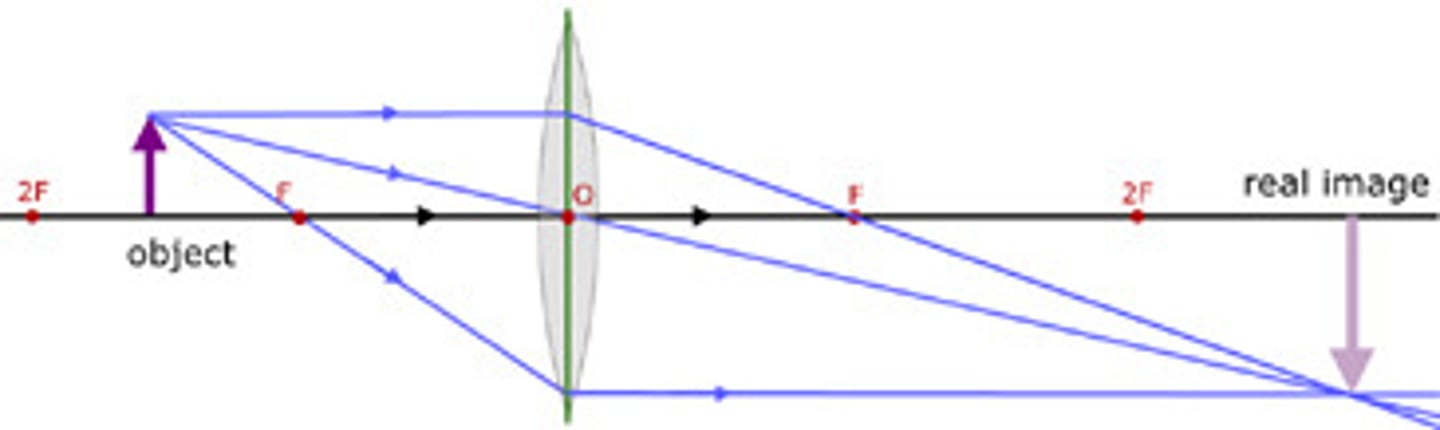
- You can calculate the total power of thin lenses used in combination using the equation P = P₁+P₂+P₃ +...

- On ray diagrams, you can measure the distance 'u' and 'v'. and use the equation (1/u)+(1/v) = 1/f

-where u = distance between object and lens axis; v = distance between the lens axis and the image; f = focal lens.

These values can also be used to calculate the magnification (m) of the lens using the equation m v/u

The photoelectric is a phenomenon that demonstrates the particle-like nature of light.

The observations made are:

- If light of a high enough frequency is shone on a metal surface, electrons are emitted

- If the frequency of the light is below the threshold frequency , no electrons will be emitted, regardless of the intensity of light

- If the intensity of light is increased, the rate of electron emission increases

- The electrons are emitted with a range of kinetic energies.

These observations led o the following conclusions:

- Light exists in discrete packets of energy known as photons, which have an energy directly proportional to their frequency.

This is described by the equation: E=hf.

- Each photon transfers all of its energy to a single electron, this is known as a one-to-one interaction.

- If the energy of the photon is higher than the work function of the metal, the electron will be emitted.

• Work function - The minimum energy required to just release an electron from the surface.

• Threshold frequency - The minimum frequency required for electrons to be emitted.

• Intensity - The number of photons per unit area.

Only the particle theory of light can correctly explain the observations, providing evidence for the particle nature of light.

This phenomenon cannot be explained by the wave model since wave theory would predict that:

• there should be no threshold frequency, since enough energy would accumulate over time

• the energy was spread across the metal's surface and so instantaneous emission wouldn't always occurs

• Increasing intensity should increase the kinetic energy that the escaping electrons have

Electrons only exist in discrete energy levels

Ionisation is when an electron is removed from an atom. Excitation is the movement of electrons up to a higher energy level; either an electron collides with the orbital electron or a photon is absorbed by it; transferring energy to it. When the electron de-excites, it moves down in energy levels and emits a photon.

This can be demonstrated by emission ad absorption spectra. In an emission spectrum you can see the frequency of photons that certain elements emit. In an absorption spectrum you can see what frequency photons certain elements absorb. These both correlate to the energy levels within its atoms

The pulse-echo technique is used with ultrasound waves (sounds waves with a frequency greater than 20 kHz) for the imagining of objects, notably for medical imaging.

Below is a brief description the pulse-echo technique:

1. Short pulse ultrasound waves are transmitted into the target (e.g the body in medical imaging).

2. As the waves move through the target, they will meet boundaries of different densities, therefore they will be reflected. The amount of reflection depends on the difference in densities of the materials; the greater this difference, the greater the reflection.

3. The reflected waves are detected as they leave the target.

4. The intensities of the reflected waves will determine the structure of the target and the time taken for these reflected waves to return will determine the position of objects in the target (using s = vt). The resolution of the image can be increased by: ● Decreasing the wa

The resolution of the image can be increased by:

- Decreasing the wavelength of the waves used

- Decreasing the duration of the pulses of waves, as this will decrease the likelihood that the waves will overlap.

What is diffraction?

Diffraction is the spreading out of a wave as it passes through a gap.

What criteria must be met for maximum diffraction to occur?

The size of the gap must be of the same magnitude as the wavelength of the wave.

What happens if the gap is much smaller than the wavelength of the wave?

The wave will be reflected.

State the diffraction grating equation

nλ = dsinθ

What does electron diffraction provide evidence for?

The wave nature of electrons. It suggests that particles can demonstrate wavelike properties

Describe the diffraction pattern produced by electrons

Concentric circles of bright and dark fringes from a central bright point

If electrons didn't have a wave nature, describe the pattern that would be produced when they pass through a slit.

The electrons would be unaffected by the gap and pass straight through. A single bright region would be formed.

What is the name given to the wavelength of a particle?

De Broglie wavelength

What two factors does the de Broglie wavelength depend on?

1. Mass

2. Velocity

State the equation used to calculate a de Broglie wavelength.

λ = h/mv

h is Plank's constant

What can 'mv' be replaced with in the de Broglie equation

p, momentum

What is the basic process of a pulse-echo technique?

- A wave pulse is emitted

- It is transmitted and reflected at the boundary between two media.

- The returning wave (echo) is detected.

- The speed and time taken are used to calculate the distance to the object.

Suggest two things that ay limit the amount of information obtained by a pulse-echo technique

1. The wavelength of the radiation

2. The duration of the pulse

What are the two models that can be used to describe electromagnetic radiation?

1. The wave model

2. The particle model

Which model does the photoelectric effect provide evidence for?

The particle model

Outline the photoelectric effect

- Light is shone on a metal plate

- If the light has a high enough frequency, electrons are emitted from the metal surface

- If the frequency is too low, no electrons are emitted

What are the particles of light used to explain the photoelectric effect called?

Photons

How do you calculate the energy of a photon?

E=hf

h is Plank's constant

f is the frequency of light

Explain how a photon can liberate an electron

One photon interacts with one electron and transfers all its energy to it. If this energy is greater than the metal's work function, the electron will have sufficient energy to be released.

What is threshold frequency?

A metals threshold frequency is the minimum frequency that a photon requires to liberate an electron from its surface.

If the intensity of light being shone on a metal increases, how does the energy of the photoelectrons change?

The energy remains unaffected. An increase in intensity means more photons per area and so more photoelectrons are emitted.

Why are photoelectrons emitted with a range of kinetic energies?

The electrons are at different depths in the metal and so require different amounts of energy to be liberated. The excess energy from a photon once an electron has been liberated, is the kinetic energy of the electron.

State the equation for the maximum kinetic energy of a photoelectron

½mv²ₘₐₓ = hf-∅

∅ is the metal's work function

What is the conversion factor between eV and J?

1eV = 1.6x10⁻¹⁹

What happens when electrons transition between energy levels?

- If electrons move to a higher energy level, radiation must be absorbed

- If electrons move to a lower energy level radiation is emitted

Why can only certain frequencies of radiation be absorbed by an atom to cause an electron transition?

The electrons can only exist in discrete energy levels. The energy of the photon absorbed must be that exact amount of energy required to cover the difference between two discrete energy levels

State the equation used to calculate intensity

I = P/A

P is the power

A is the area

What is the refractive index of a material through which light travels a speed of 'v'?

n = c/v

where c is the speed of light in a vacuum

State the equation linking the refractive index and angles at an interface between two mediums.

n₁sinθ₁ = n₂sinθ₂

What is the critical angle?

The angle of incidence for which the angle of reflection is 90⁰ and all the light passes along the boundary between the mediums. Beyond this angle all light will be reflected.

State the equation used to calculate a critical angle

sinC = 1/n

What is total internal reflection?

Total internal reflection is where all the light is reflected back at the boundary between two mediums. It occurs when light is incident at an angle greater than the critical angle.