Centripetal Force Questions

AP Physics I

A car rounds a curve while maintaining a constant speed.

With constant speed…

A car rounds a curve while maintaining a constant speed.

With constant speed…

there would still be a net force that is directed inward (relative to the curve) on the car

A mass attached to a string that is itself attached to the ceiling swings back and forth.

At the bottom of the arc…

there is no centrifugal force

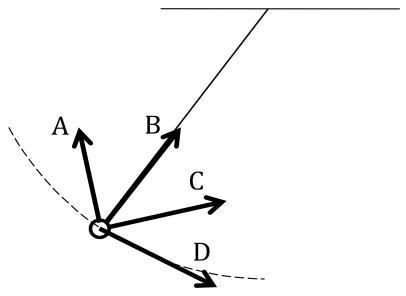
A mass attached to a string that is itself attached to the ceiling swings back and forth. If the bob is observed to be moving upward at a given instance, as shown in the image, which arrow best depicted the direction of the net force acting on the bob at that instant?

C

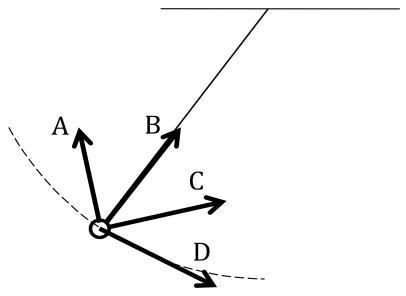
If the bob had been moving downward, the net center-seeking force would…

be the same as when the bob was moving upward along its arc

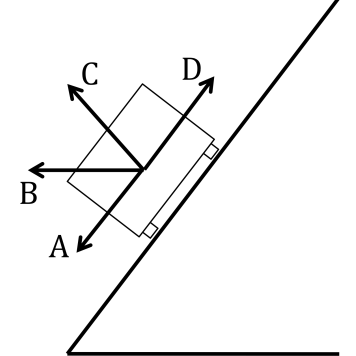
A car rounds a very steep, banked curve moving at low speed. A front-end view of the car is shown.

Which of the directions shown depicts the net centripetal force acting on the car?

B

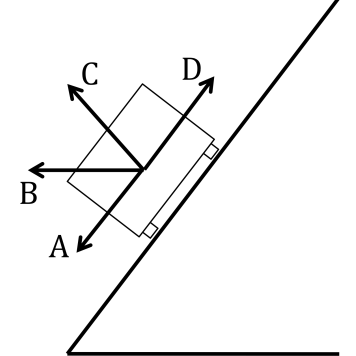
A car rounds a very steep, banked curve moving at low speed. A front-end view of the car is shown.

Which direction depicts the net force acting on the car?

B

A car rounds a very steep, banked curve moving at low speed. A front-end view of the car is shown.

The frictional force…

static and is directed up the incline

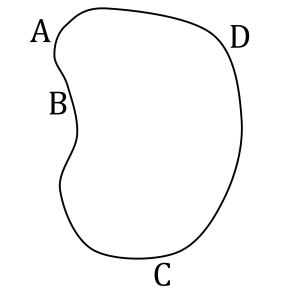
A car travels with constant speed around the track shown.

At which point will the centripetal force be the largest?

A

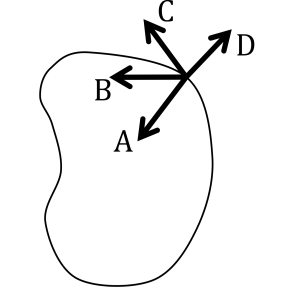
A car travels counterclockwise with constant speed around the track shown.

Which of the vectors depicts the direction of the net force acting on the car at the point shown?

A

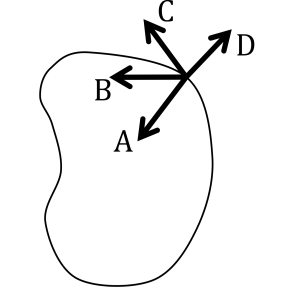
The car traveling in the image is observed to be speeding up.

Which of the vectors depicts the direction of the net force acting on the car at the point shown?

B

What statement is NOT true about centripetal forces?

They are one of the six naturally occurring forces used in conjunction with free body diagrams.

A car of mass m travels around a curve with velocity v.

What do you know about the centripetal force if the velocity triples?

The centripetal force increases by a factor of nine.

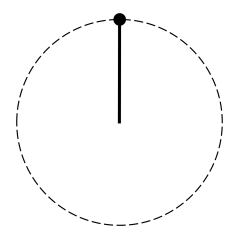
A string with a bob attached swings in the vertical (see sketch).

If it just barely makes it through the top of its arc, you know that:

The tension in the string at the top will be zero.

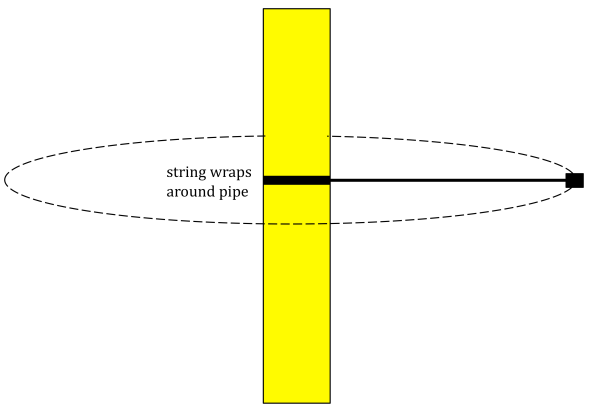
A string wraps around a fat pipe as a bob attached to the string is made to move in a circular path in the horizontal.

Assuming the velocity is somehow held constant as the radius diminishes due to the wrapping, how will the centripetal force change?

It will increase.

A car going around a curve is found to be able to take the curve with maximum speed “v”. You would like to double the maximum speed the car can take the curve.

To do this, you could:

quadruple the coefficient of friction between the car and the road;