Math - Precalculus

How to solve for x² or y^2?

Use root on both sides of equation but consider that there may be both positive and negative solutions so add ± in front of other side of equation.This technique allows for finding both possible values of x or y when working with quadratic equations.

If you're just evaluating a root you take only the positive root.

If you're solving an equation where you introduce a square root to undo squaring (like x^2 = 16), you must include ±.

What are the input/independent variable and output/dependent variable of a function?

The input/independent variable is the value that is manipulated or chosen in a function (often denoted as x), while the output/dependent variable is the value that is affected by the input (often denoted as y).

How to factor a standard quadratic equation?

To factor a standard quadratic equation of the form ax^2 + bx + c, look for two numbers that multiply to ac and add to b. Rewrite the equation using these numbers to break it into two binomial factors.

How to foil expressions?

To foil (First, Outer, Inner, Last) is a method for multiplying two binomials. Multiply the first terms, then the outer terms, inner terms, and last terms, and then combine like terms.

How to distribute in algebra?

Distributing involves multiplying a single term by each term inside a set of parentheses. For example, a(b + c) = ab + ac.

What are the steps to simplify an equation?

1. Eliminate parentheses by distributing terms. 2. Combine like terms by adding or subtracting coefficients of similar variables or constants. 3. Rearrange the equation to get all variable terms on one side and constant terms on the other side. 4. Isolate the variable by using inverse operations (addition, subtraction, multiplication, and division) to solve for the variable.

What are explicit and implicit forms of functions?

Explicit forms of functions provide a direct equation that clearly shows the dependent variable as a function of the independent variable, typically formatted as y = f(x). You can solve for y. Examples include linear equations like y = 2x + 3. Implicit forms, on the other hand, denote the relationship between variables without isolating one variable, often represented as F(x, y) = 0, indicating that both x and y must satisfy a specific condition simultaneously. This might appear in equations like x^2 + y^2 = 1, which defines a circle.

What is the difference quotient?

The difference quotient is a formula used in calculus to find the average rate of change of a function over an interval. It is expressed as f(x + h) - f(x) / h, where h is a small increment.

How to find the derivative using the difference quotient?

To find the derivative using the difference quotient, use the formula: f'(x) = lim(h→0) [f(x + h) - f(x)] / h. This computes the slope of the tangent line to the function at point x by evaluating the average rate of change as h approaches zero.

How to factor out an expression?

Factoring out involves taking a common factor from terms in an algebraic expression. For example, in the expression 6x + 9, you can factor out 3 to get 3(2x + 3).

What are the 3 steps for finding the domain of a function defined by an equation?

1. Start with the set of all real numbers. 2. If the function has a denominator (fraction), exclude any numbers for which the denominator = 0. 3. If the equation has a radical with an even index, exclude any numbers for which the expression inside the radical (the radicand) is negative.

What is interval notation?

Interval notation is a way of representing a set of numbers as an interval, defined by its endpoints. For example, (a, b) represents all numbers x such that a < x < b. Square brackets [ ] indicate inclusion of the endpoint, while parentheses ( ) indicate exclusion.

How to write the absolute value of a number?

The absolute value of a number x is written as |x| and represents the distance of x from zero on the number line, regardless of direction.

What is the symbol for union, and where is it used?

The symbol for union is ∪. It is used in set theory to combine two sets, representing all elements that are in either set. For example, A ∪ B includes all elements that are in set A, set B, or in both.

What is set-builder notation?

Set-builder notation is a concise way of specifying a set by stating the properties that its members must satisfy. It is written in the form {x | property}, meaning 'the set of all x such that the property is true for x'.

How to calculate the area under a graph?

To calculate the area under a graph, use definite integrals if the function is continuous. The area is found using the integral from a to b of f(x) dx, where f(x) is the function and [a, b] are the limits of integration.

How to calculate the area of a rectangle?

The area of a rectangle is calculated by multiplying its length by its width. The formula is A = length × width.

How to calculate the area of a triangle?

The area of a triangle is calculated using the formula A = 1/2 × base × height.

What is set notation?

Set notation is a mathematical notation for describing a set by listing its elements or stating a property that its members must satisfy, typically written in the form {x | property}.

How to determine whether a function is even, odd, or neither?

A function f(x) is even if f(-x) = f(x) for all x, odd if f(-x) = -f(x), and neither if neither condition holds. Even functions are symmetric about the y-axis, and odd functions are symmetric about the origin.

What is the sum in mathematics?

The sum is the result of adding two or more numbers or expressions together.

What is the difference in mathematics?

The difference is the result of subtracting one number or expression from another.

What is the product in mathematics?

The product is the result of multiplying two or more numbers or expressions together.

What is the quotient in mathematics?

The quotient is the result of dividing one number or expression by another.

How to divide fractions by each other?

To divide fractions, multiply the first fraction by the reciprocal of the second. For example, to divide 1/2 by 3/4: 1/2 ÷ 3/4 = 1/2 × 4/3 = 4/6 = 2/3.

What is the vertical line test theorem?

The vertical line test is a method to determine if a graph represents a function. If a vertical line crosses the graph at more than one point, the graph does not represent a function.

When can you cross multiply?

You can cross multiply when you have a proportion, meaning you have two fractions set equal to each other. For example, for a/b = c/d, you can cross multiply to get ad = bc.

What is the definition of an even function?

A function is even if f(-x) = f(x) for all x in its domain, indicating symmetry about the y-axis.

What is the definition of an odd function?

A function is odd if f(-x) = -f(x) for all x in its domain, indicating symmetry about the origin.

What is the definition of a function that is neither even nor odd?

A function is neither even nor odd if it does not satisfy the conditions for evenness or oddness, meaning f(-x) ≠ f(x) and f(-x) ≠ -f(x) for some x.

How to determine if a function is even, odd, or neither from the equation?

To determine the nature of a function, replace x with -x in the equation and simplify. If you get the original function, it's even; if you get the negative of the original, it's odd; otherwise, it's neither.

How to find if a function is increasing, decreasing, or constant in an interval?

Analyze the function's derivative. If f'(x) > 0 in an interval, the function is increasing. If f'(x) < 0, it's decreasing. If f'(x) = 0, it's constant.

What are local maxima?

Local maxima are points in a function where the value is higher than all nearby points, meaning the function changes from increasing to decreasing at that point.

Can a point be both a local and absolute maximum?

Yes, a point can be both a local and absolute maximum if it is the highest point in a function over its entire domain as well as higher than all nearby points.

What is the formula for calculating average rate of change?

The average rate of change is calculated using the formula: (Δy)/(Δx) = (f(b) - f(a)) / (b - a), where a and b are values in the domain.

What is the formula for calculating slope?

The slope (m) is calculated as m = (y2 - y1) / (x2 - x1), using two points (x1, y1) and (x2, y2) on the line.

What do you call a line between two points on a graph?

A line connecting two points on a graph is called a secant line.

What is the symbol for slope?

The symbol for slope is m.

What is point-slope form?

Point-slope form of a line is given by the equation y - y1 = m(x - x1), where m is the slope and (x1, y1) is a point on the line.

Which formula to use for a line?

You can use the slope-intercept form y = mx + b, where m is the slope and b is the y-intercept, or the point-slope form y - y1 = m(x - x1).

What is the order of operations?

The order of operations is a set of rules that determines the sequence in which calculations are performed in mathematical expressions, typically remembered by the acronym PEMDAS: Parentheses, Exponents, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).

What is the standard order for writing equations?

The standard order for writing equations is to express them in standard form, typically Ax + By = C, where A, B, and C are integers and x and y are variables.

How to do divisions on paper? (include example)

To divide on paper, use long division. For example, to divide 154 by 7: 1. How many times does 7 go into 15? It goes 2 times (2*7=14). Write 2 above the 15. 2. Subtract 14 from 15, giving 1. 3. Bring down the 4, making 14. 4. 7 goes into 14 exactly 2 times. Put 2 above the 4. Thus, 154 ÷ 7 = 22.

How to simplify -x = -y?

To simplify -x = -y, multiply both sides by -1 to obtain x = y. This shows that if both sides are negative, cancelling the negatives yields equivalent expressions.

What are derivatives?

Derivatives represent the rate of change of a function with respect to a variable and are fundamental concepts in calculus, indicating how a function's output value changes as its input value changes.

What are antiderivatives?

Antiderivatives, also known as indefinite integrals, are functions that reverse the process of differentiation, providing a function whose derivative is the original function.

What are prime numbers?

Prime numbers are natural numbers greater than 1 that have no positive divisors other than 1 and themselves, meaning they can only be divided evenly by 1 and the number itself.

What is the symbol for prime?

The symbol for prime is 'p', often used in contexts to denote prime numbers.

When to use prime?

The prime symbol (') is used in mathematics to denote the derivative of a function, to indicate a transformed variable, or to signify the following term in sequences or series.

What is the secant slope formula?

The secant slope formula measures the average rate of change between two points on a curve and is given by the formula: (f(b) - f(a)) / (b - a), where a and b are the x-coordinates of the two points.

How to solve complex fractions by splitting

Steps:

1. Rewrite as division: a/bc/d=ab÷cd\frac{a/b}{c/d} = \frac{a}{b} \div \frac{c}{d}c/da/b​=ba​÷dc​.

2. Multiply by the reciprocal: ab×dc\frac{a}{b} \times \frac{d}{c}ba​×cd​.

3. Multiply across and simplify.

Example:

Solve 56109\frac{\frac{5}{6}}{\frac{10}{9}}910​65​​:

• Rewrite: 56÷109\frac{5}{6} \div \frac{10}{9}65​÷910​

• Multiply by reciprocal: 56×910\frac{5}{6} \times \frac{9}{10}65​×109​

• Multiply across: 4560=34\frac{45}{60} = \frac{3}{4}6045​=43​

✅ Final Answer: 34\frac{3}{4}43​

How to factor out a common multiplicator?

To factor out a common multiplicator, identify the greatest common factor (GCF) of the terms in the expression, then rewrite the expression as the GCF multiplied by the remaining terms. For example, in the expression 6x^2 + 9x, the GCF is 3x, so it can be factored as 3x(2x + 3).

What is the point slop form?

The point slope form of a linear equation is used to express the equation of a line given a point on the line and its slope. It is represented as y - y_1 = m(x - x_1), where (x_1, y_1) is a point on the line and m is the slope.

What is the slop intercept form?

The slope-intercept form of a linear equation is a way to express the equation of a line using its slope and y-intercept. It is represented as y = mx + b, where m is the slope and b is the y-intercept.

Definition of accentotic

A term used in calculus to describe a curve that approaches a line but never touches it, typically referring to behavior as the variable approaches infinity.

Properties, formula and example of a linear function

A linear function is a function that can be expressed in the form f(x) = mx + b, where m is the slope and b is the y-intercept. Its properties include a constant rate of change and a graph that forms a straight line.

Properties, formula and example of the identity function

The identity function is a linear function defined as f(x) = x, where the slope is 1 and the y-intercept is 0. It maps every input value to itself, resulting in a straight line that passes through the origin.

Properties, formula and example of a constant function

A constant function is a function that always returns the same value regardless of the input, typically expressed as f(x) = c, where c is a constant. Its graph is a horizontal line, indicating that there is no change in the output as the input varies.

Properties, formula and example of a square function

A square function is a polynomial function defined as f(x) = x², where the graph is a parabola that opens upwards. Its vertex is at the origin, and it exhibits a quadratic rate of change.

Properties, formula and example of a cube function

A cube function is a polynomial function defined as f(x) = x³, where the graph is a cubic curve that passes through the origin. It exhibits a cubic rate of change and has one inflection point.

Properties, formula and example of a square root function

A square root function is defined as f(x) = √x, where the graph is a curve that starts at the origin and increases gradually. Its domain consists of all non-negative real numbers, and it exhibits a decreasing rate of change as x increases.

Properties, formula and example of a cuve root function

A cube root function is a polynomial function defined as f(x) = ∛x, where the graph is a curve that passes through the origin and extends into all quadrants. It exhibits a root rate of change and has no inflection points.

Properties, formula and example of a reciprocal function

A reciprocal function is defined as f(x) = 1/x, where the graph consists of two hyperbolas in the first and third quadrants. Its domain excludes zero, and it exhibits a decreasing rate of change as x moves away from the origin.

Properties, formula and example of an absolute value function

An absolute value function is defined as f(x) = |x|, where the graph forms a V-shape that opens upwards at the origin. Its domain consists of all real numbers, and it exhibits a constant rate of change for x values both greater than and less than zero.

What happens to the graph of y=f(x) when multiplied by a where a>1?

The graph is vertically stretched by a factor of aaa.

What happens to the graph of y=f(x) when multiplied by a where 0<a<10 ?

The graph is vertically compressed by a factor of

𝑎.

What happens to the graph of y = f(x) when multiplied by a where a<0?

The graph is vertically stretched or compressed by a factor of ∣𝑎∣ and reflected across the x-axis.

What does multiplying a function by a constant a do to the graph?

It results in vertical stretching or compression depending on aaa, and if a is negative, it also reflects across the x-axis.

What does the graph of y=−f(x) represent?

It is a vertical reflection ofu=f(x) across the x-axis.

What does the graph of y=f(−x) represent?

It is a horizontal reflection of y=f(x) across the y-axis.

How do you reflect a function across the x-axis?

Multiply the output of f(x) by -1, resulting in y=−f(x).

How do you reflect a function across the y-axis?

Multiply the input of f(x) by -1, resulting in y=f(−x).

How does y=-f(x) behave compared to f(-x)

y=-f(x) reflexts over the x axis while f(-x) reflexts over the y axis

How to find the y-value of a transformed function using a given point.

Find the transformed x, if not vertical change, then original y-value can replace x. Then solve for the new y-value.

What is a polynomial function?

A polynomial function is a mathematical expression consisting of variables and coefficients, involving only addition, subtraction, multiplication, and non-negative integer exponents. It is typically written in the form:

P(x)=anxn+an−1xn−1+⋯+a1x+a0

where an,an−1,…,a0​ are constants, and nn is a non-negative integer representing the degree of the polynomial.

Can a polynomial function have a negative of non-integer exponent?

No, functions with those would not be polynomial functions.

What are monomial functions?

A monomial function is a polynomial function with only one term. It has the form:

f(x)=axn

where a is a coefficient, x is a variable, and n is a non-negative integer representing the degree of the monomial. Examples include 3x23x2 or −5x−5x.

How to find the 0s of a polynomial function?

To find the zeros of a polynomial function using factoring, first set the function equal to zero.

Given a polynomial f(x), solve f(x) = 0.

Next, factor the polynomial by breaking it down into simpler expressions, if possible.

For example, if f(x) = x² - 5x + 6, it factors as (x - 2)(x - 3) = 0.

Then, set each factor equal to zero and solve for x. In this case, x - 2 = 0 gives x = 2, and x - 3 = 0 gives x = 3.

Finally, list the solutions, which are the zeros of the function.

What is the degree of a polynomial function?

The degree is the highest power of x after the funciton is fully simplified (may need to foil).

If r is a real zero of a multiplicity, what does even or uneven mean both numerically and graphically?

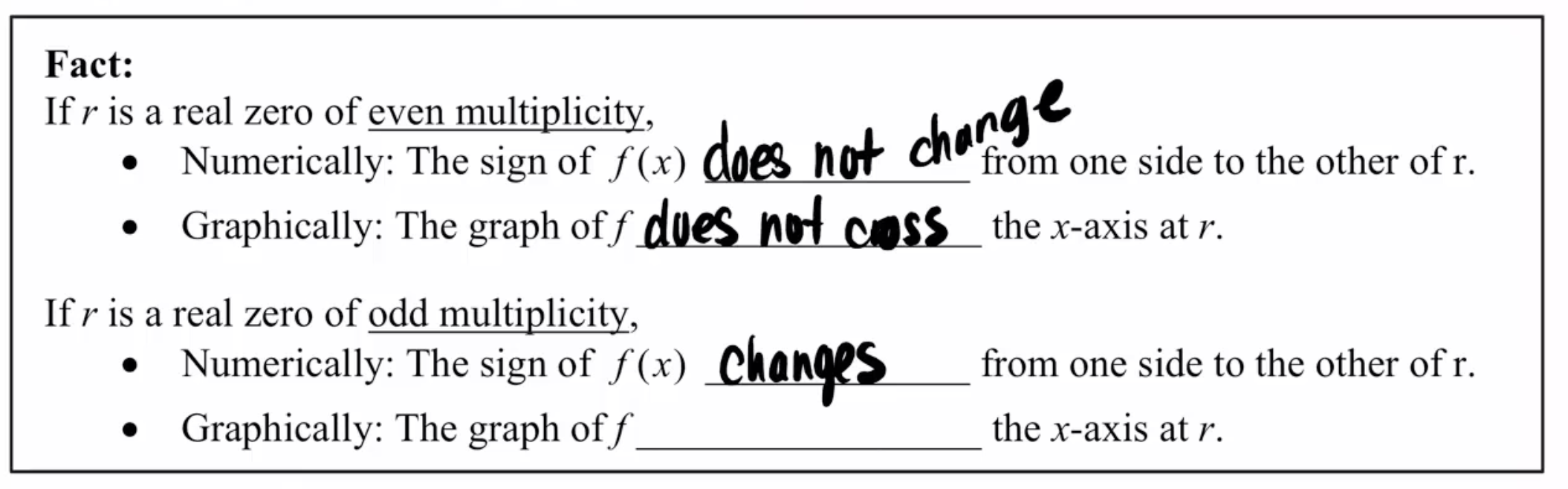
How are turning points of a polomial function related to it's exponents?

The number of turning points can only be up to n-1, n being is largest radicant.

Can you deduce a polynomial functions exponent from the number of turning points?

Kind of. If the graph of a polynomial functions has n-1 turning points, then the degree of f is at least n.

What is end behavior?

End behavior describes how a function behaves as x approaches positive or negative infinity, meaning what happens to f of x at extreme values of x.

For polynomial functions, end behavior is primarily determined by the leading term, which is the term with the highest degree, the degree being even or odd, and the leading coefficient being positive or negative.

For even degree polynomials like x squared or x to the fourth, if the leading coefficient is positive, both ends go up. If the leading coefficient is negative, both ends go down. For example, in f of x equals x to the fourth, as x approaches both positive and negative infinity, f of x approaches positive infinity.

For odd degree polynomials like x cubed or x to the fifth, if the leading coefficient is positive, the left end goes down while the right end goes up. If the leading coefficient is negative, the left end goes up while the right end goes down. For example, in f of x equals negative x cubed, as x approaches positive infinity, f of x approaches negative infinity, and as x approaches negative infinity, f of x approaches positive infinity.

Are exponents of like terms multiplied or added?

They are added.

• For example, (x2)×(x3)=x2+3=x5=

  • The degrees (2 and 3) are added to get the new degree (5).