MCHE3310 Final MCQ

Polyethylene (PE)

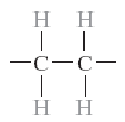
Poly(vinyl chloride) (PVC)

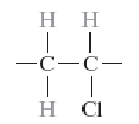
Polytetrafluoroethylene (PTFE)

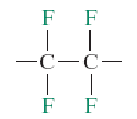
Polypropylene (PP)

Poly(methyl methacrylate) (PMMA)

Polystyrene (PS)

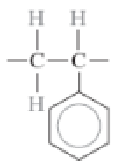
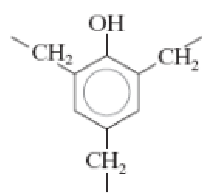
Phenol-formaldehyde (Bakelite)

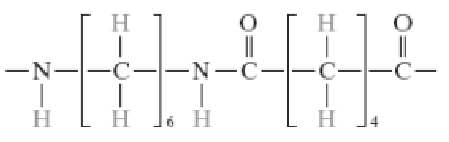
Poly(hexamethylene adipamide) (nylon 6,6)

Poly(ethylene terephthalate) (PET, a polyester)

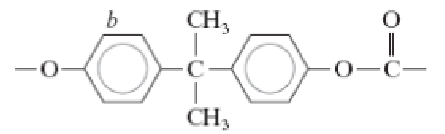
Polycarbonate (PC)

Linear

Linear

Branched

Branched

Cross-Linked

Cross-Linked

Network

Network

Random

Alternating

Block

Graft

What are polymers?

Molecules made of many repeat units, often hydrocarbons, such as polyethylene, poly(vinyl chloride), and polypropylene.

“Poly“ meaning

many

“mer“ meaning

repeat unit

What are some examples of natural polymers?

Wood, rubber, cotton, wool, leather, and silk.

Oldest known uses of polymers

Rubber balls used by Incas, and Noah used pitch (a natural polymer) for the ark

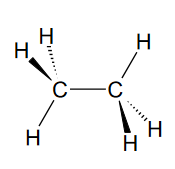
What are saturated hydrocarbons?

Molecules where each carbon is singly bonded to four other atoms, such as in ethane (C2H6).

What is the primary composition of most polymers?

Most polymers are hydrocarbons, composed primarily of hydrogen (H) and carbon (C).

What is the difference between double and triple bonds in hydrocarbons? (Unsaturated hydrocarbons)

These bonds are less stable than single bonds and can form new bonds.

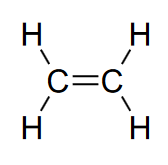
Double bonds (unsaturated hydrocarbons)

Double bonds are found in ethylene (C2H4)

Triple bonds (unsaturated hydrocarbons)

Triple bonds are in acetylene (C2H2).

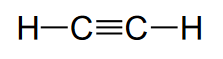
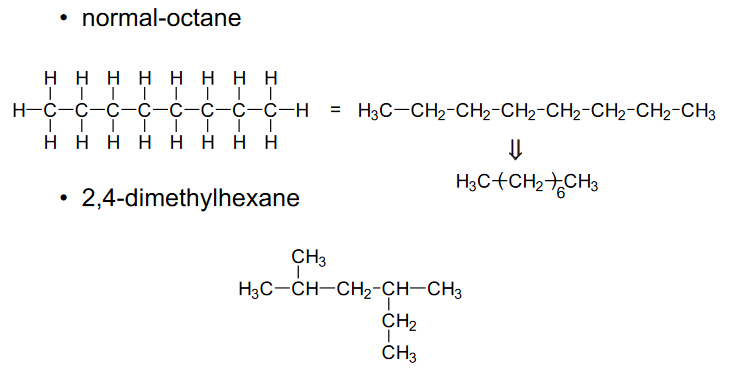
Isomerism in polymers

Two compounds have the same chemical formula but different structures, such as (C₈H₁₈) normal-octane and 2,4-dimethylhexane

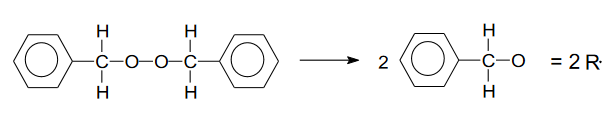
Free radical polymerization

Involves an initiator (e.g., benzoyl peroxide) creating free radicals that initiate chain reactions with monomers like ethylene, forming long polymer chains.

How is polyethylene structured?

Long-chain hydrocarbon, where each carbon atom is bonded to two hydrogen atoms. It is similar to paraffin wax but has a much longer chain.

Molecular weight, M

The mass of a mole of chains. Polymers contain chains of varying lengths, leading to a distribution of molecular weights.

Number-average molecular weight, M_n

The total weight of the polymer divided by the total number of molecules

Degree of polymerization, DP

The average number of repeat units per chain, calculated by dividing the number-average molecular weight by the molecular weight of the repeat unit.

Molecular Shape (or Conformation)

Chain bending and twisting are possible by rotation of carbon atoms around their chain bonds.

Note: not necessary to break chain bonds to alter molecular shape

Stereoisomerism (configurations)

Occurs when molecules are mirror images of each other, but they cannot be superimposed without breaking a bond

Tacticity

The spatial arrangement of R units along the polymer chain. It can be isotactic (same side), syndiotactic (alternating sides), or atactic (random arrangement).

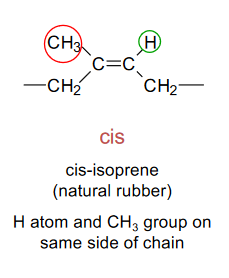
Cis-isomerism

The H atom and CH3 group are on the same side of the chain.

Trans-isomerism

The H atom and CH3 group are on opposite sides of the chain.

Polymer Crystallinity

The ordered atomic arrangements of molecular chains. Polymers are rarely 100% crystalline due to difficulty aligning all regions of the chains. Ex. polyethylene unit cell

Crystalline regions in polymers

Thin platelets with chain folds at faces, chain folded structure

Why are polymers rarely 100% crystalline? (Degree of crystallinity/%crystallinity)

It is difficult for all regions of all polymer chains to align perfectly, preventing polymers from being fully crystalline.

Heat treating

Causes crystalline regions to grow and increases %crystallinity

Polymer single crystals

Form under slow, controlled growth rates, consisting of multilayered chain-folded layers.

Spherulite in Polymers

Form in some polymers due to rapid growth and consist of alternating crystallites and amorphous regions

How does cross-polarized light reveal spherulites in polymers?

Shows a Maltese cross pattern when viewed under cross-polarized light, revealing their semicrystalline structure.

Composites in Nature

Bones (collagen (strong & soft) and mineral apatite (hard & brittle)) and wood (cellulose fiber (strong & flexible) and lignin (stiffer))

Composite Materials

Materials consisting of two or more chemically distinct constituents, artificially-made, on a macroscale, and having a distinct interface separating them.

Primary motivation for using composites

To obtain materials with an unusual combination of properties, especially mechanical properties like strength, stiffness, toughness, and fatigue resistance, which cannot be met by conventional materials.

Phases in a composite material

Matrix (continuous) and Dispersed/reinforcement (surrounded by matrix)

Purpose of Matrix phase

To transfer stress to dispersed phase and protect dispersed phase from environment

Elastic Modulus, E

Higher in Dispersed phase (reinforcement) than Matrix

Types of Matrix phase

MMC (Metal matrix composite), CMC (Ceramic matrix composite), PMC (Polymer matrix composite)

Purpose of Dispersed phase (reinforcement) in MMC

Increases yield strength (σy), tensile strength (TS), and creep resistance

Purpose of Dispersed phase (reinforcement) in CMC

Increases fracture toughness (K_IC)

Purpose of Dispersed phase (reinforcement) in PMC

Increases elastic modulus (E), yield strength (σy), tensile strength (TS), and creep resistance.

Types of dispersed phase (reinforcement)

Particle, fiber, and structural

Particle-reinforced composites

are isotropic

Fiber-reinforce composites

are basically anisotropic

Structural composites

can be isotropic or anisotropic based on configurations

Large particle composites

• particle-matrix interaction cannot be treated on the atomic or molecular

  level;

• continuum mechanics is used;

• particles restrain movement of matrix;

• particles bear a portion of load

• the bonding between the particles and matrix should be strong.

Dispersion-strengthed composites

• particle diameters: 10 – 100 nm;

• particle-matrix interaction occurs on the atomic or molecular level;

• Particles hinder or impede the motion of dislocations to increase the composites’ yield and tensile strength and hardness.

Examples of particle-reinforced composites

Spheroidite steel (ferrite matrix with cementite particles), WC/Co cemented carbide, automobile tire rubber (rubber matrix with carbon black particles), and concrete (cement matrix with sand and gravel).

‘Rule of mixture' in particle-reinforced composites

The elastic modulus (Ec) of a particle-reinforced composite falls between the upper limit [Ec = VmEm + VpEp] and the lower limit [Ec = 1 / (Vm/Em + Vp/Ep)]

Why are fiber-reinforced composites important?

They provide significant strength improvement to the composite because fibers are strong in tension, and the matrix holds fibers in place and transfers the load to them.

Fiber types

Whiskers, Fibers, Wires

Whiskers

Thin single crystals - large length to diameter ratios

• graphite, silicon nitride, silicon carbide

• high crystal perfection – extremely strong, strongest known

• very expensive and difficult to disperse

Fibers

• polycrystalline or amorphous

• generally polymers or ceramics

• Ex: alumina, aramid, E-glass, boron, UHMWPE

Wires

Metals - steel, molybdenum, tungsten

What is the critical length of fibers in composites?

The critical length (lc) is lc = d*fiber tensile strength / (2 fiber-matrix bond strength), where d is the fiber diameter.

What is the effect of fiber orientation on the properties of fiber-reinforced composites?

Aligned fibers provide higher reinforcement efficiency, while random orientations reduce reinforcement effectiveness.

Longitudinal modulus for continuous fiber-reinforced composites

[Ec = Ef*Vf + Em*Vm], where Ef and Em are the elastic moduli of the fibers and matrix, and Vf and Vm are their volume fractions.

Transverse modulus for continuous fiber-reinforced composites

[1/Ect = Vf/Ef + Vm/Em], under isostress conditions.

Isostress

The stress on the material causes equal stress on both fibers and the matrix.

Isostrain

The stress on the material causes the uniform strain on both fibers and matrix.

What is the role of the efficiency factor (K) in discontinuous fiber-reinforced composites?

The efficiency factor (K) accounts for fiber orientation. For aligned (Parallel) fibers K = 1, for aligned fiber (perpendicular) for random 2D fibers K = 3/8, and for random 3D fibers K = 1/5.

Efficiency factor (K) for aligned (parallel)

K = 1

Efficiency factor (K) for aligned (perpendicular)

K = 0

Efficiency factor (K) for random 2D (2D isotropy)

K = 3/8

Efficiency factor (K) for random 3D (3D isotropy)

K = 1/5

Pultrusion in the fabrication of fiber-reinforced polymer composites

Involves pulling continuous fibers through a resin tank, impregnating them with resin, then passing them through a die that preforms and cures the composite.

What is filament winding in the fabrication of fiber-reinforced polymer composites?

Filament winding involves winding continuous fibers, impregnated with resin, onto a mandrel to form hollow cylindrical shapes, followed by curing.

Types of Laminates (structural composites)

Unidirectional, cross-ply, angle-ply, multidirectional

What are the types of structural composites?

Laminates (stacked fiber-reinforced sheets) and sandwich panels (with a thick, lightweight core and strong face sheets)

What are the benefits of sandwich panels in composites?

Low density and high bending stiffness, making them suitable for lightweight, stiff structures

CMC benefits

Increased toughness

PMC benefits

Increased E (Elastic modulus)/ρ (density)

MMC benefits

Increased creep resistance