Chem 1103 Exam 3
0.0(0)
0.0(0)
Card Sorting
1/166
There's no tags or description
Looks like no tags are added yet.
Study Analytics
Name | Mastery | Learn | Test | Matching | Spaced |
|---|
No study sessions yet.
167 Terms
1
New cards
Previous Chapter Info
2
New cards
Prefixes (conversion)
* mega (M)- 10^6
* kilo (k)- 10^3
* deka (da)- 10
* deci (d)- 10^-1
* centi (c)- 10^-2
* milli (m)- 10^-3
* micro (the weird u symbol)- 10^-6
* nano (n)- 10^-9
* pico (p)- 10^-12
* kilo (k)- 10^3
* deka (da)- 10
* deci (d)- 10^-1
* centi (c)- 10^-2
* milli (m)- 10^-3
* micro (the weird u symbol)- 10^-6
* nano (n)- 10^-9
* pico (p)- 10^-12
3
New cards
Acetate
C₂H₃O₂⁻
4
New cards
Carbonate
CO₃²⁻
5
New cards
Hydrogen Carbonate (aka bicarbonate)
HCO₃⁻
6
New cards
\
Hydroxide
Hydroxide
OH⁻
7
New cards
Nitrate
NO₃⁻
8
New cards
Nitrite
NO₂⁻
9
New cards
Chromate
CrO₄²⁻
10
New cards
Dichromate
Cr₂O₇²⁻
11
New cards
Phosphate
PO₄³⁻
12
New cards
Hydrogen Phosphate
HPO₄²⁻
13
New cards
Dihydrogen Phosphate
H2PO4-
14
New cards
Ammonium
NH₄⁺
15
New cards
Hydronium
H3O+
16
New cards
Hypochlorite
ClO⁻
17
New cards
Chlorite
ClO₂⁻
18
New cards
Chlorate
ClO₃⁻
19
New cards
Perchlorate
ClO₄⁻
20
New cards
Permanganate
MnO₄⁻
21
New cards
Sulfate
SO₄²⁻
22
New cards
\
Sulfite
Sulfite
SO₃²⁻
23
New cards
hydrogen sulfite (aka bisulfite)
HSO₃⁻
24
New cards
\
hydrogen sulfate (aka bisulfate)
hydrogen sulfate (aka bisulfate)
HSO₄⁻
25
New cards
Peroxide
O₂²⁻
\
\
26
New cards
Cyanide
CN⁻
27
New cards
Chapter 7-11
28
New cards
Visible light
is a type of __**electromagnetic radiation**__
29
New cards
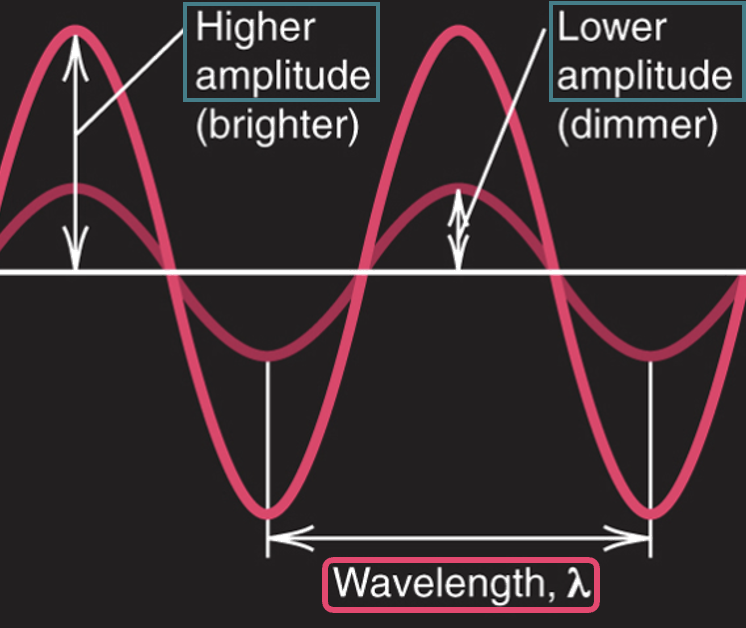
Wave properties of electromagnetic radiation
* **frequency** (ν, *nu*)
* cycles per second (1 / s)
* **wavelength** (λ, *lambda*)
* the distance a wave travels in one cycle; the distance between adjacent wave peaks
* **amplitude**
* the height of a wave crest or depth of a trough
* cycles per second (1 / s)
* **wavelength** (λ, *lambda*)
* the distance a wave travels in one cycle; the distance between adjacent wave peaks
* **amplitude**
* the height of a wave crest or depth of a trough
30
New cards
**Speed of light**
3\.00x10^8 m/s
31
New cards
amplitude and wavelength
* no relationship between the two
32
New cards
Frequency and wavelength
* __**inverse relationship**__ between the frequency of a wave and its wavelength
* For waves traveling at the same speed, the shorter the wavelength, the more frequently they pass
* For waves traveling at the same speed, the shorter the wavelength, the more frequently they pass
33
New cards
frequency/ wavelength formula
**v = c/λ**
34
New cards
energy and wavelength
Inversely proportional
35
New cards
electromagnetic spectrum (from Low wavelength/ high energy to high wavelength/low energy)
* Gamma Rays
* X-Rays
* Ultra-violet
* Visible range
* infrared
* microwaves
* radio
* X-Rays
* Ultra-violet
* Visible range
* infrared
* microwaves
* radio
36
New cards
Visible Range
* 400-750 nm
37
New cards
color
* “White” light is a mixture of __**ALL**__ the colors of visible light
* wavelength of colors decreasing order: ROY G BIV
* color= when object absorbs some of wavelengths of white light but reflects others
* wavelength of colors decreasing order: ROY G BIV
* color= when object absorbs some of wavelengths of white light but reflects others
38
New cards
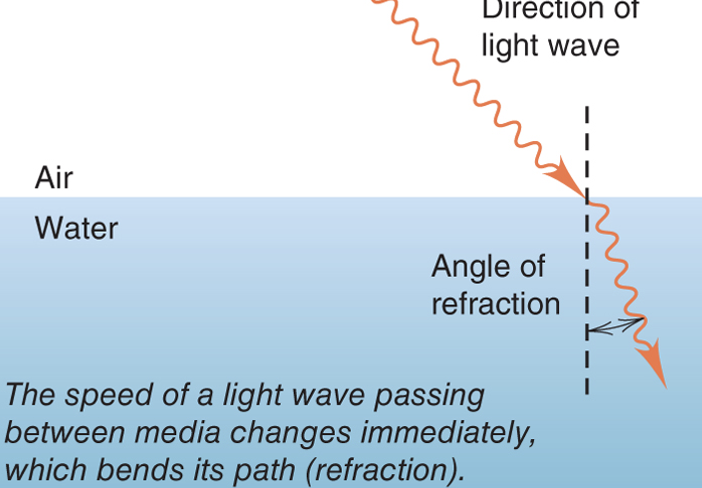
Refraction
* When a light wave passes from one medium into another, the speed of the wave changes
* Particles of matter do not undergo refraction
* Particles of matter do not undergo refraction
39
New cards
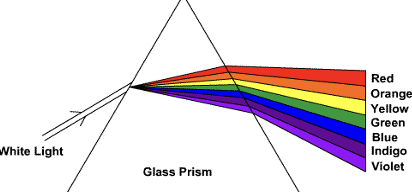
Dispersion
* White light separates into its component colors when it passes through a prism
* Each incoming wave is refracted at a slightly different angle
* Each incoming wave is refracted at a slightly different angle
40
New cards
Interference
* interaction between waves
41
New cards
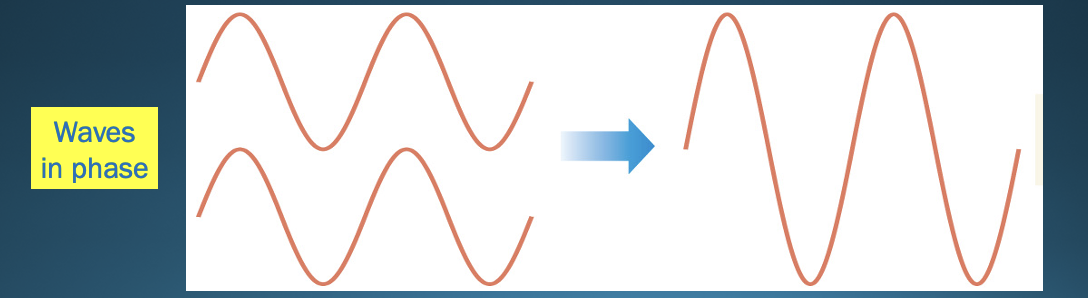
Constructive interference
* waves interact so they add to make a larger wave
* IN phase
* IN phase
42
New cards
Destructive interference
* The waves interact so they cancel each other
* OUT of phase
* OUT of phase
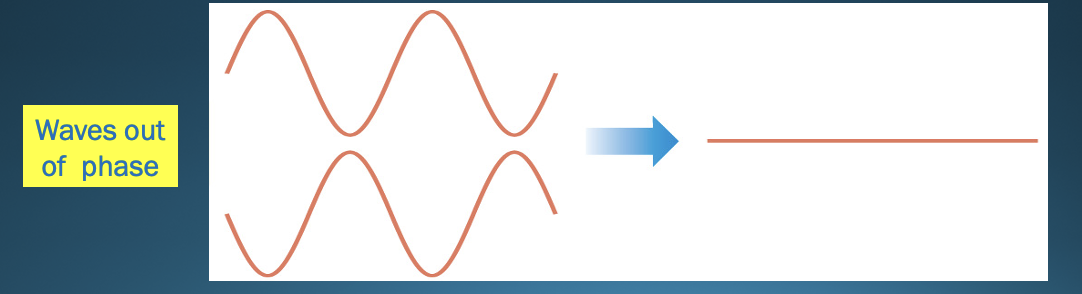
43
New cards
Diffraction
* NOT refraction
* When traveling waves encounter an obstacle or opening in a barrier, they “*move*” through or around it
* Particles do not diffract
* either go thru slit or dont
* When traveling waves encounter an obstacle or opening in a barrier, they “*move*” through or around it
* Particles do not diffract
* either go thru slit or dont

44
New cards
Blackbody radiation
* energy radiated by any object or system that absorbs all incident radiation
* Black Body Radiation illustrates that **temperature** is related to **energy**
* Black Body Radiation illustrates that **temperature** is related to **energy**
45
New cards
Quantum Theory
* color/ intensity of emitted light changes as the temperature changes
* **COLOR** is related to *n* and λ
* THUS **energy** has to be related to frequency and wavelength somehow
* made by Max Plank
* determined that a hot, glowing object could emit (or absorb) only ***certain*** quantities of energy
* **COLOR** is related to *n* and λ
* THUS **energy** has to be related to frequency and wavelength somehow
* made by Max Plank
* determined that a hot, glowing object could emit (or absorb) only ***certain*** quantities of energy
46
New cards
Energy and Frequency Formula
* E=*n*h*v*
* *E = energy of the radiation*
* *n* = quantum number; a positive integer (1, 2, 3…)
* v = frequency
* h= Planks Constant (6.626x10^-34)
* *E = energy of the radiation*
* *n* = quantum number; a positive integer (1, 2, 3…)
* v = frequency
* h= Planks Constant (6.626x10^-34)
47
New cards
Planks Constant
6/626x10^-34
48
New cards
The Quantum Theory of Energy
* Any object can emit or absorb ONLY __**certain**__ quantities of energy
* energy is quantized
* occurs in fixed quantities rather than continuous
* Each fixed quantity of energy is called a quantum
* atom changes energy “state” by emitting or absorbing one or more quanta of energy
* energy is quantized
* occurs in fixed quantities rather than continuous
* Each fixed quantity of energy is called a quantum
* atom changes energy “state” by emitting or absorbing one or more quanta of energy
49
New cards
Energy Changes
* Δ*E =* Δ*n*h*v*
* *E = energy of the radiation*
* *n* = quantum number; a positive integer (1, 2, 3…)
* v = frequency
* h= Planks Constant (6/626x10^-34)
* *E = energy of the radiation*
* *n* = quantum number; a positive integer (1, 2, 3…)
* v = frequency
* h= Planks Constant (6/626x10^-34)
50
New cards
Energy Formulas
E = h*v* = hc /λ
* E= hc/ λ
* E= hv
* V= c/ λ
* E= hc/ λ
* E= hv
* V= c/ λ
51
New cards
52
New cards
Threshold Freq. in Wave Model & Real World
Wave model:
* intensity is responsible for observed E and e- will break off when it has absorbed enough light of any color
Real world
* the e- only breaks free when it is hit w certain color of light (certain v), regardless of brightness
* intensity is responsible for observed E and e- will break off when it has absorbed enough light of any color
Real world
* the e- only breaks free when it is hit w certain color of light (certain v), regardless of brightness
53
New cards
Time Lag in Wave Model & Real World
Wave Model
* if the light is dim, less E is absorbed, so the e- should have to spend more time absorbing before it can break free
Real World
* current begins to flow immediatley when it is hit w appropriate color of light, again, regardless of brightness
* if the light is dim, less E is absorbed, so the e- should have to spend more time absorbing before it can break free
Real World
* current begins to flow immediatley when it is hit w appropriate color of light, again, regardless of brightness
54
New cards
Photon Theory
Threshold Frequency:
* intensity represents the number of photons, not the E.
* E is related to v, so an e- must absorb a photon of a certain minimum color to break free
Time Lag
* photon either has enough energy to free e- in one hit or it doesnt; the e- cannot store energy until it has enough
* intensity represents the number of photons, not the E.
* E is related to v, so an e- must absorb a photon of a certain minimum color to break free
Time Lag
* photon either has enough energy to free e- in one hit or it doesnt; the e- cannot store energy until it has enough
55
New cards
Line spectrum
* series of fine lines at specific frequencies separated by “black spaces”
* Each atom of a particular element has its own unique line spectra (aka emission spectra)
* Each atom of a particular element has its own unique line spectra (aka emission spectra)
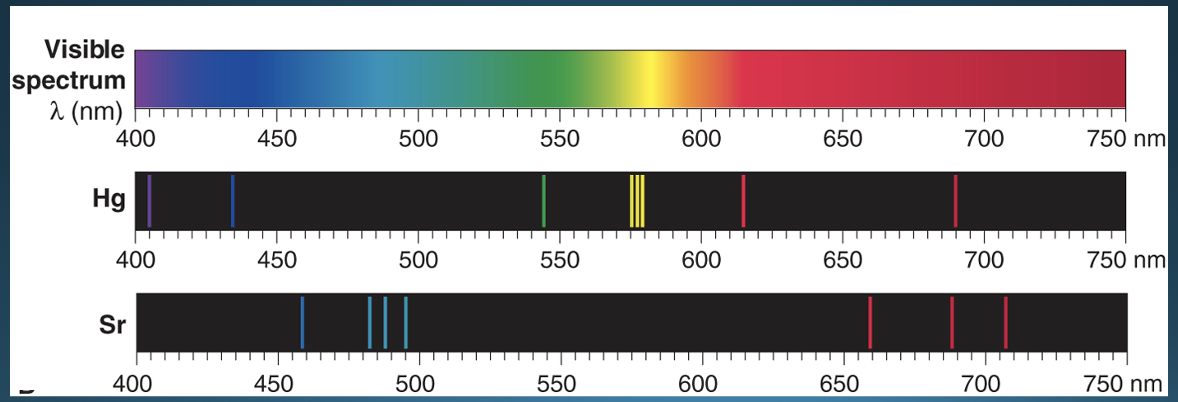
56
New cards
Bohr’s Model of the Hydrogen Atom
* made of ORBITS not orbitals!
1. The H atom has only certain energy levels, stationary states
1. The higher the energy level, the farther the orbit is from the nucleus
2. The atom does not radiate energy while in one of its stationary states
3. The atom changes to another stationary state __**ONLY**__ by absorbing or emitting a photon
1. The energy of the photon (hn) equals the difference between the energies of the two energy states
1. The H atom has only certain energy levels, stationary states
1. The higher the energy level, the farther the orbit is from the nucleus
2. The atom does not radiate energy while in one of its stationary states
3. The atom changes to another stationary state __**ONLY**__ by absorbing or emitting a photon
1. The energy of the photon (hn) equals the difference between the energies of the two energy states
57
New cards
quantum numbers and electron orbit
* n (quantum) positive integer that reps radius of e orbit
* lower the n value, the smaller the radius of the orbit, and the lower the energy level
* When the electron is in an orbit closer to the nucleus (lower n), more energy is required to move it out of that orbit than when it is in an orbit farther from the nucleus (higher *n*)
* lower the n value, the smaller the radius of the orbit, and the lower the energy level
* When the electron is in an orbit closer to the nucleus (lower n), more energy is required to move it out of that orbit than when it is in an orbit farther from the nucleus (higher *n*)

58
New cards
Ground state
* When the electron is in the first orbit (n=1), closest to nucleus, H atom is in its lowest (1st) energy level
59
New cards
excited state
* if electron in any orbit further from nucleus, atom in excited state
* second orbit (n=2) = first excited state, third orbit (n=3) = second excited state etc etc
* second orbit (n=2) = first excited state, third orbit (n=3) = second excited state etc etc
60
New cards
Absorption & Bohr Model
If a H atom absorbs a photon whose energy equals the difference between lower and higher energy levels, the electron moves to the outer (higher energy) orbit
61
New cards
Emission & Bohr model
If a H atom in a higher energy level (electron in a farther orbit) returns to a lower energy level (electron in a closer orbit), the atom emits a photon whose energy equals the difference between the two levels
62
New cards
Quantum staircase
The energy difference between two consecutive orbits decreases as *n* increases
* absorption & emission = inversely related
* absorption & emission = inversely related

63
New cards
Rydberg’s equation: NRG transition problem (constant provided)
* used to to solve for the wavelength of a spectral line or energy-level transitions
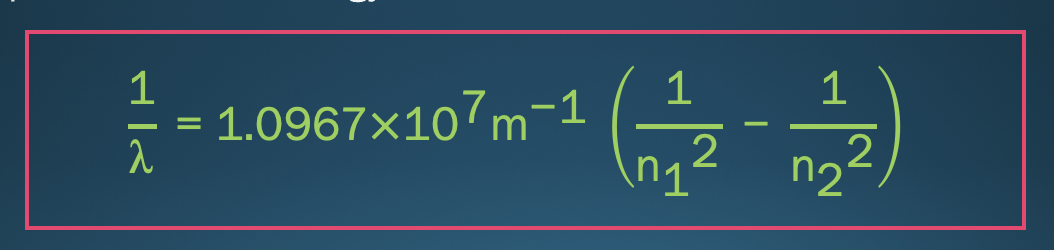
64
New cards
Limitations of Bohr’s Model
* ONLY works for Hydrogen
* fails completely when you introduce more than one electron to the system
* MAJOR FLAW: assumes *electrons move in fixed, defined orbits*
* fails completely when you introduce more than one electron to the system
* MAJOR FLAW: assumes *electrons move in fixed, defined orbits*
65
New cards
Emission Spectrum
* Occurs when atoms in an excited state *emit* photons as they return to a lower energy state
* Some elements produce an intense spectral line that is evidence of their presence
* **Flame tests –** performed by placing a granule of an ionic compound or a drop of its solution in a flame
* Some elements produce an intense spectral line that is evidence of their presence
* **Flame tests –** performed by placing a granule of an ionic compound or a drop of its solution in a flame
66
New cards
Absorption Spectrum
* “opposite” of an emission spectra
* Produced when atoms ***absorb*** photons of certain wavelengths and become excited
* Sodium’s absorption spectrum shows dark lines at the same wavelengths as the yellow-orange lines in sodium's emission spectrum
* Produced when atoms ***absorb*** photons of certain wavelengths and become excited
* Sodium’s absorption spectrum shows dark lines at the same wavelengths as the yellow-orange lines in sodium's emission spectrum

67
New cards
**Theory of Relativity**
**matter and energy are alternate forms of the same entity**
68
New cards
de Broglie Wavelength equation
* an equation for the wavelength of any particle of mass m moving at speed u (substituted for c)
* Matter behaves as though it moves in waves
* An object’s wavelength is **inversely** proportional to it’s mass and speed
* Matter behaves as though it moves in waves
* An object’s wavelength is **inversely** proportional to it’s mass and speed

69
New cards
Heisenberg’s Uncertainty Principle
* impossible to know, simultaneously, the position ***and*** momentum of an particle
70
New cards
locations of electrons
* dont know exact position of an electron, but can determine where it *probably* might be
* Solving the wave function gives the ***probability density***, a measure of the ***probability*** of finding an electron of a particular energy in a particular region of the atom
* Solving the wave function gives the ***probability density***, a measure of the ***probability*** of finding an electron of a particular energy in a particular region of the atom
71
New cards
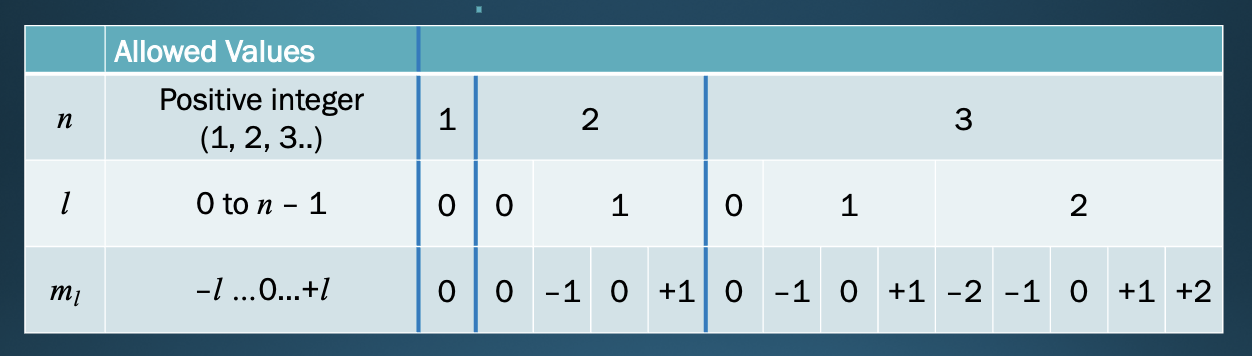
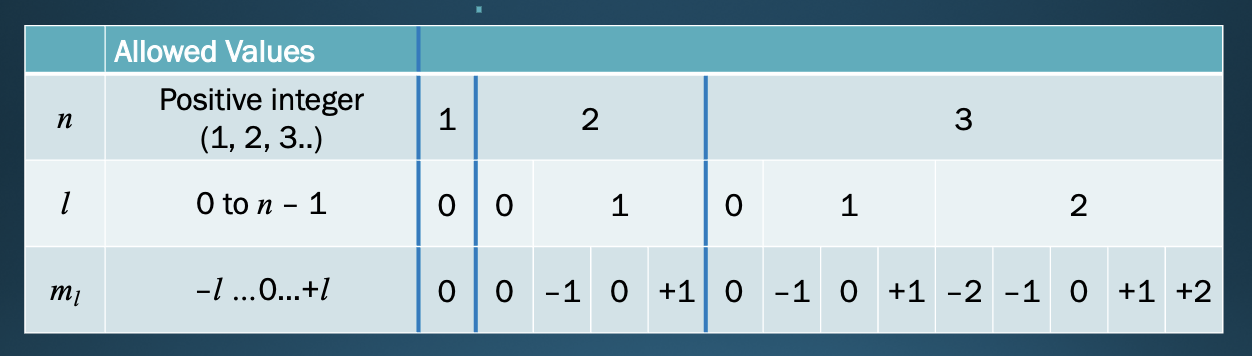
Quantum numbers and Atomic Orbitals
* atomic orbital specified by 4 quantum numbers:
* Principal quantum number
* Angular momentum quantum number
* Magnetic quantum number
* spin quantum number
* Principal quantum number
* Angular momentum quantum number
* Magnetic quantum number
* spin quantum number
72
New cards
Principal quantum number
* n
* positive whole # (1, 2, 3…)
* Indicates the relative *distance from the nucleus (tells u how far u r from nucleus)*
* Specifies the energy level
* orbital energy (size)
\
* positive whole # (1, 2, 3…)
* Indicates the relative *distance from the nucleus (tells u how far u r from nucleus)*
* Specifies the energy level
* orbital energy (size)
\
73
New cards
Angular momentum quantum number
* (*l*)
* integer from 0 to ***n*** **– 1**
* shape of the orbital
* S,P,D,F
* integer from 0 to ***n*** **– 1**
* shape of the orbital
* S,P,D,F
74
New cards
Magnetic quantum number
* (*ml*)
* integer from –*l* to +*l*
* Describes the 3D orientation of the orbital in the space around the nucleus (what orientation L is in n state)
* integer from –*l* to +*l*
* Describes the 3D orientation of the orbital in the space around the nucleus (what orientation L is in n state)
75
New cards
Spin Quantum Number
* Ms
* +1/2 or -1/2
* direction of e- spin
* +1/2 or -1/2
* direction of e- spin
76
New cards
how to get orbitals from quantum numbers

77
New cards
Energy Levels
* The levels (given by n) are divided into sublevels (or subshells), given by the *l* value
* *l* = 0 is an *s* sublevel
* *l* = 1 is an *p* sublevel
* *l* = 2 is an *d* sublevel
* *l* = 3 is an *f* sublevel
* *l* = 0 is an *s* sublevel
* *l* = 1 is an *p* sublevel
* *l* = 2 is an *d* sublevel
* *l* = 3 is an *f* sublevel
78
New cards
S Orbital shape
* spherical shape w/ nucleus in center
* has only ONE *ml* value
* has only ONE *ml* value
79
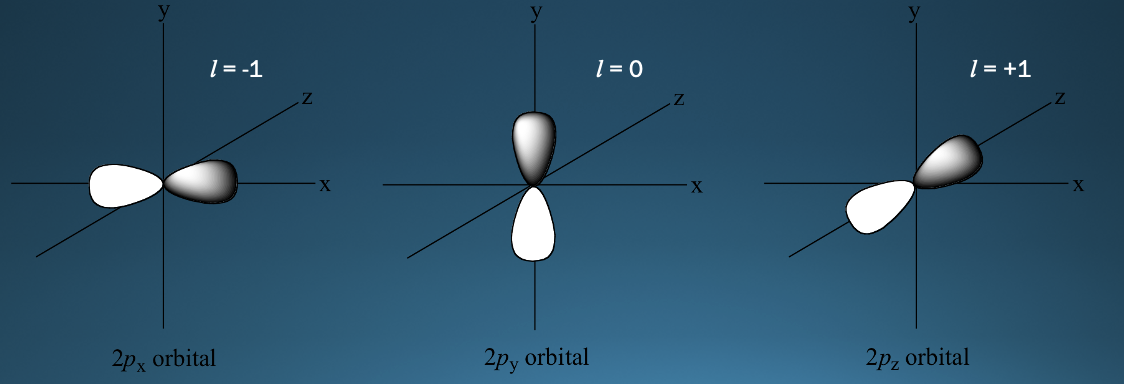
New cards
P Orbitals shape
* have two regions (lobes) of high probability of finding an electron, one on either side of the nucleus
80
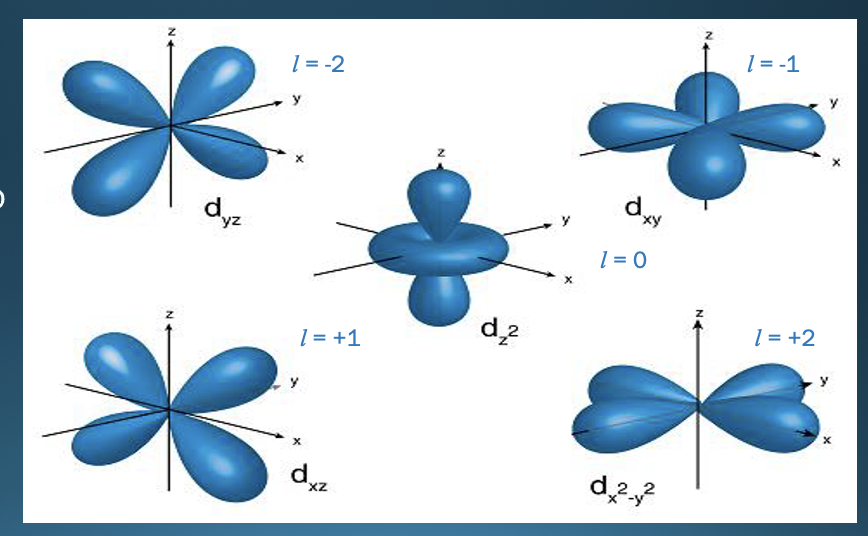
New cards
D Orbitals shape
81
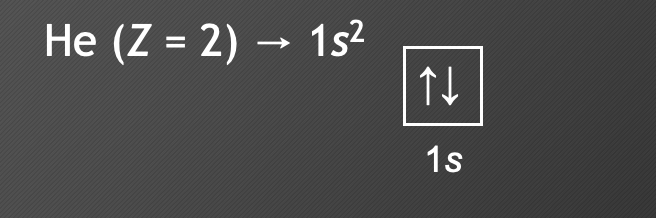
New cards
Pauli Exclusion Principle
* each orbital may contain a maximum of 2 electrons, which must have opposite spins
82
New cards
aufbau principle
* electrons are always placed in the lowest energy sublevel available

83
New cards
**Hund’s rule**
* when orbitals of equal energy are available, the lowest energy electron configuration has the **maximum number of unpaired electrons** with parallel spins
84
New cards
S orbital electrons
* (*l* = 0)
* max number of e—s = 2
* *ml* = 0, so there is only one atomic orbital
* max number of e—s = 2
* *ml* = 0, so there is only one atomic orbital
85
New cards
P orbital electrons
* (*l* = 1)
* max number of e—s = 6
* *ml* = -1, 0, +1 → three atomic orbitals
* max number of e—s = 6
* *ml* = -1, 0, +1 → three atomic orbitals
86
New cards
d orbital electrons
* (*l* = 2)
* max number of e—s = 10
* *ml* = -2, -1, 0, +1, +2 → five atomic orbitals
* max number of e—s = 10
* *ml* = -2, -1, 0, +1, +2 → five atomic orbitals
87
New cards
f orbital electrons
* (*l* = 3)
* max number of e—s = 14
* *ml* = -3, -2, -1, 0, +1, +2, +3 → seven atomic orbitals
* max number of e—s = 14
* *ml* = -3, -2, -1, 0, +1, +2, +3 → seven atomic orbitals
88
New cards
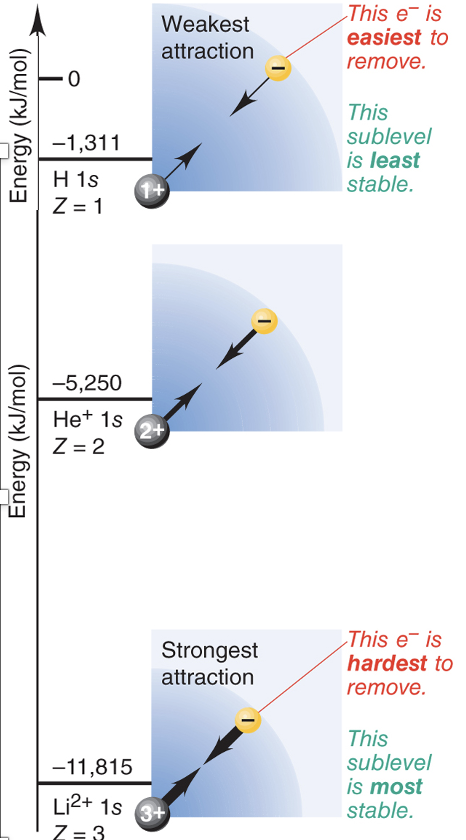
Nuclear Charge (*Z*)
* A higher nuclear charge (more protons) increases nucleus-electron attractions, lowering the sublevel energy and stabilizes the atom (lower E = good!)
89
New cards
Shielding
* each electron “feels” presence of others so each electron __**shields**__ the others from the nuclear charge (charge of the nucleus)
* Essentially, each e— is blocking some of the nucleus’s attraction from other nearby e—
* Essentially, each e— is blocking some of the nucleus’s attraction from other nearby e—
90
New cards
**effective nuclear charge (*****Z***eff)
* “full” nuclear charge is reduced to an __**effective nuclear charge (*****Z***____eff)__, the nuclear charge an electron __*actually*__ experiences
91
New cards
Penetration
* increases nuclear attraction and decreases shielding
* The better an outer electron is at penetrating through the electron cloud of inner electrons, the more attraction it will have for the nucleus
* The better an outer electron is at penetrating through the electron cloud of inner electrons, the more attraction it will have for the nucleus
92
New cards
stability of sublevels
s < p < d < f
93
New cards
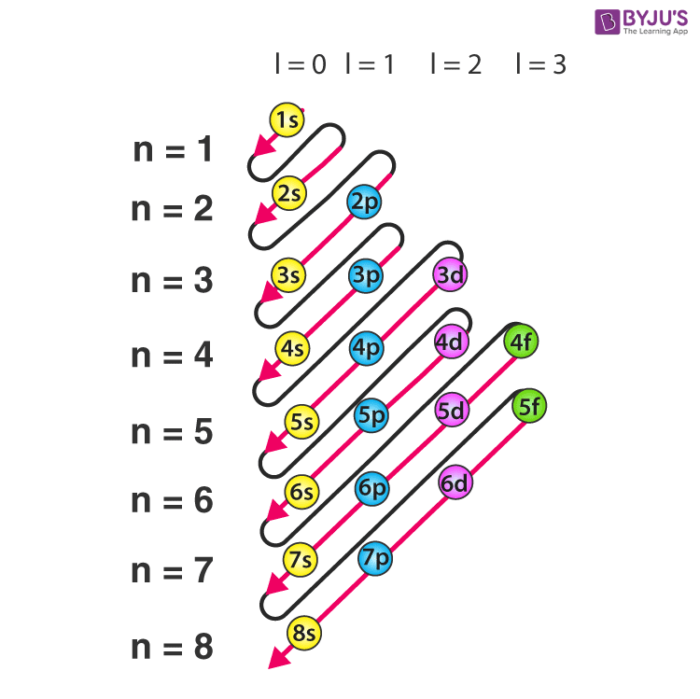
e configuration
94
New cards
Half-filled exceptions!
* **Cr** (Z=24) → \[Ar\] 4s2 3d4 → __[Ar] 4s13d5__
* Mo → __5s1 4d5__
* Cu (Z=29) → \[Ar\] 4s1 3d10
* Ag→ 5s1 d10
* Au→ 6s1 4f15 5d10
* Mo → __5s1 4d5__
* Cu (Z=29) → \[Ar\] 4s1 3d10
* Ag→ 5s1 d10
* Au→ 6s1 4f15 5d10
95
New cards
writing e config→ types of electrons
1. full is based off atomic #
2. condensed is valence electrons
3. inner electrons are the electrons which get replaced by a noble gas
96
New cards
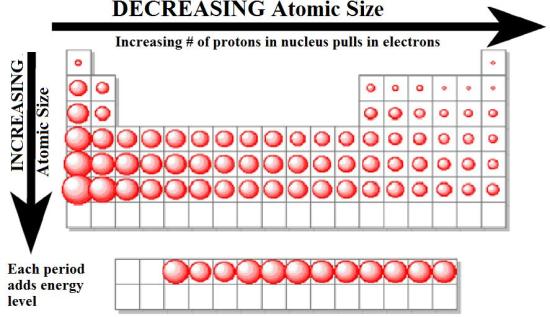
atomic size
\*transition metalls increase down but dont really change ACROSS
97
New cards
ionization energy
* energy required to remove e

98
New cards
exceptions to ionization energy trend
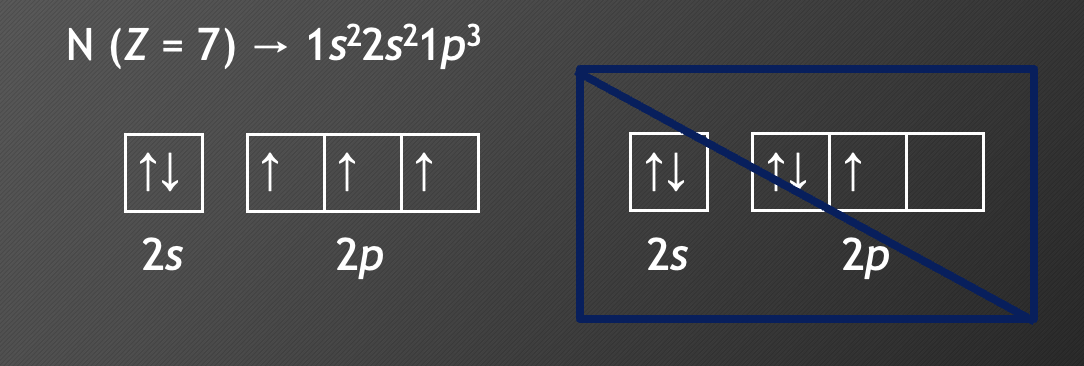
* nitrogen: 1s2 2s2 2p3
* stable half-filled structure
* Taking an e— from N would make it **less stable** (higher energy)
* Oxygen: 1s2 2s2 2p4
* one e— beyond stable
* Taking an e— from oxygen would make the atom **more stable** (lower energy)
* It is easier to remove an e— from O (creating stability) than it is to remove one from N (destroying stability)
* True for Be/B, N/O, Mg/Al, P/S, Ca/Ga, As/Se
* stable half-filled structure
* Taking an e— from N would make it **less stable** (higher energy)
* Oxygen: 1s2 2s2 2p4
* one e— beyond stable
* Taking an e— from oxygen would make the atom **more stable** (lower energy)
* It is easier to remove an e— from O (creating stability) than it is to remove one from N (destroying stability)
* True for Be/B, N/O, Mg/Al, P/S, Ca/Ga, As/Se
99
New cards
Successive Ionization Energies
* For a given element, IE1, IE2, and so on, increase because each electron is pulled away from a species with a higher positive charge
* This increase includes an enormous jump __***after***__ the __**last valence electron**__ has been removed because *much* more energy is needed to remove an inner (core) electron
* This increase includes an enormous jump __***after***__ the __**last valence electron**__ has been removed because *much* more energy is needed to remove an inner (core) electron

100
New cards
ID an element from its IEs
* identify the largest increase in IE
* occurs after last Ve is removed
* that increase identifies Ve
* period X (will be given) element which has that # valence electron
* occurs after last Ve is removed
* that increase identifies Ve
* period X (will be given) element which has that # valence electron
