Chapter 9: Games and Strategic Behavior

player/strategy/payoff

basic elements of a game

the ____, the ____ available to each …., and the ____ each … receives for each possible combination of …

game

basic elements of a __

the players, the strategies available to each player, and the payoffs each player receives for each possible combination of strategies

dominant

____ strategy

one that yields a higher payoff no matter what the other players in a game choose

dominated

____ strategy

any other strategy available to a player who has a dominant strategy

Nash Equalibrium

_____ _______

any combination of strategy choices in which each player’s choice is his or her best choice, given the other players’ choices

A/B

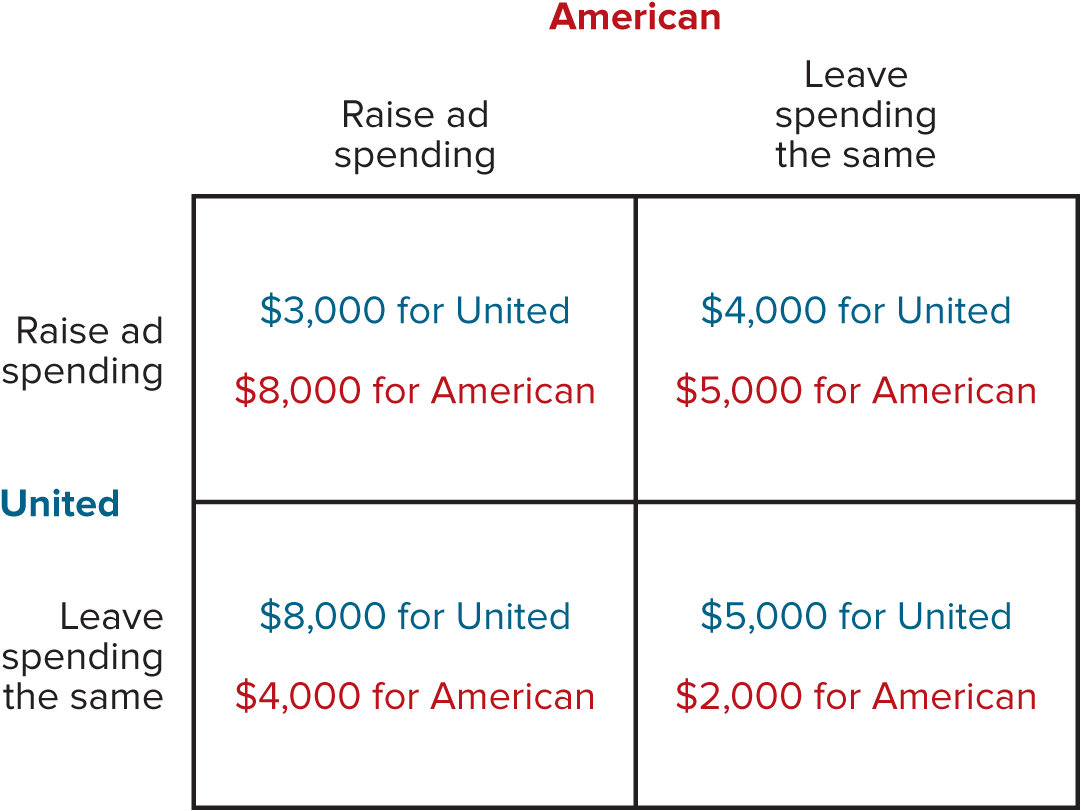
Consider the table on the right. For American, the dominant strategy is ________, for united, dominant strategy is ________

A. raise ad spending

B. leave spending the same

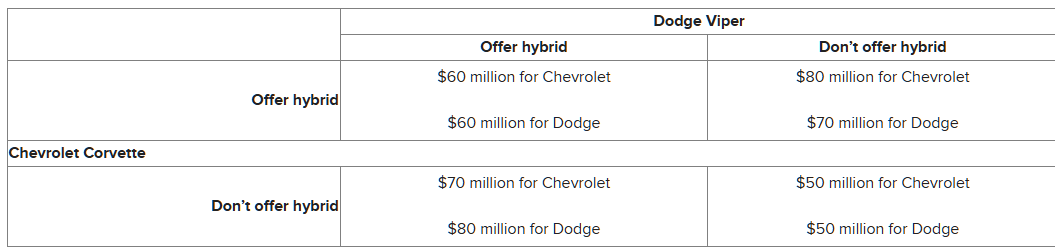
D

A. only Dodge has a dominant strategy

B. both Dodge and Chevrolet have a dominant strategy

C. only Chevrolet has a dominant strategy

D. neither Dodge nor Chevrolet have a dominant strategy

prisoner’s dilemma

_________ ________

a game in which each player has a dominant strategy, and when each plays it, the resulting payoffs are smaller than if each had played a dominated strategy

invest/not invest/is not

GM has a dominant strategy to ____ (invest/not invest), and Chrysler has a dominant strategy to ____ (invest/not invest). In following their dominant strategy, each does BETTER than if they had followed their dominated strategy (10>5). So, this game ____ (is/is not) prisoner's dilemma.

cartel

_____

a coalition of firms that agree to restrict output for the purpose of earning an economic profit

strong

A firm in a cartel agreement has a ___(strong/weak) incentive to undercut the prices of the other cartel members. This behavior can lead to a breakdown of the cartel as firms prioritize individual profit over collective agreements.

repeated

____ prisoner’s dilemma

a standard prisoner’s dilemma that confronts the same players repeatedly

tit-for-tat

A strategy for the repeated prisoner's dilemma in which players cooperate on the first move, then mimic their partner's last move on each successive move is known as a ____ strategy.

C

Shouting at parties

A. may make everyone worse off, and is therefore irrational.

B. may make everyone worse off, and is not a dominant strategy for each individual.

C. may make everyone worse off, but is a dominant strategy for each individual.

decision tree

A ____ ____ (or game tree) is a diagram that describes the possible moves in a game in sequence and lists the payoffs that correspond to each possible combination of moves.

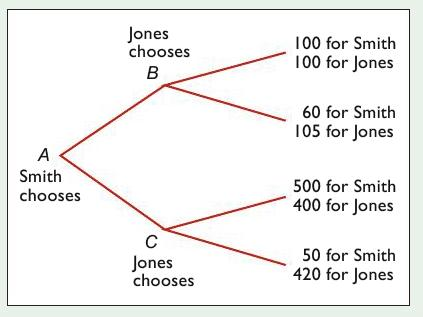
A

In repeated prisoner's dilemmas, tit-for-tat strategies have been shown to be effective at limiting defection in

A. computer simulations but not real-world cartel agreements.

B. real-world cartel agreements, but not computer simulations.

C. both

D. neither

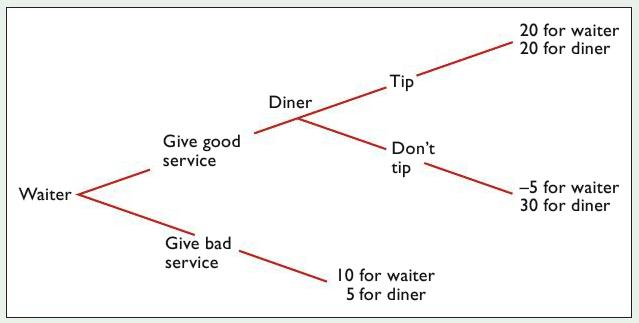
D

Consider the decision tree to the right. What is each player's equilibrium payoff?

Multiple choice question.

A. -5 for the waiter and 30 for the diner

B. 20 for the waiter and 20 for the diner

C. 20 for the waiter and 30 for the diner

D. 10 for the waiter and 5 for the diner

confess/0

Blackadder and Baldrick are rational, self-interested criminals imprisoned in separate cells in a dark medieval dungeon. They face the prisoner’s dilemma displayed in the matrix.

 

		Blackadder
		Confess	Deny
Baldrick	Confess	5 years for each	0 years for Baldrick20 for Blackadder
	Deny	20 years for Baldrick0 for Blackadder	1 year for each

 

Assume that Blackadder is willing to pay $1,000 for each year by which he can reduce his sentence below 20 years. A corrupt jailer tells Blackadder that before he decides whether to confess or deny the crime, she can tell him Baldrick’s decision.

 

since baldrick is rational and self interested, he would act on his best payoff, which means he will always choose the dominant strategy _____ (confess/deny)

therefore, given the decision of baldrick is already known, then blackadder has only one choice. therefore the value is _________

no/yes

The owner of a thriving business wants to open a new office in a distant city. If they can hire someone who will manage the new office honestly, they can afford to pay that person a weekly salary of $2,000 ($1,000 more than the manager would be able to earn elsewhere) and still earn an economic profit of $800. The owner’s concern is that they will not be able to monitor the manager’s behavior and that the manager would therefore be in a position to embezzle money from the business. The owner knows that if the remote office is managed dishonestly, the manager can earn $4,000, which results in an economic loss of $100 per week. (Hint: Construct a decision tree to help you answer the questions below.)

(yes/no) If the owner believes that all managers are narrowly self-interested income maximizers, will they open the new office?

(yes/no) Suppose the owner knows that a managerial candidate is a genuinely honest person who condemns dishonest behavior and who would be willing to pay up to $6,000 to avoid the guilt they would feel if they were dishonest. Will the owner open the remote office?

C/2/No/B/B

Consider the following “dating game,” which has two players, A and B, and two strategies, to buy a movie ticket or a baseball ticket. The payoffs, given in points, are shown in the matrix below. Note that the highest payoffs occur when both A and B attend the same event.

 

		B
		Buy movie ticket	Buy baseball ticket
A	Buy movie ticket	2 for A3 for B	0 for A0 for B
	Buy baseball ticket	1 for A1 for B	3 for A2 for B

 

Assume that players A and B buy their tickets separately and simultaneously. Each must decide what to do knowing the available choices and payoffs but not what the other has actually chosen. Each player believes the other to be rational and self-interested.

 

1. Does either player have a dominant strategy?

    

   multiple choice 1

   • Player A alone has a dominant strategy.

   • Player B alone has a dominant strategy.

   • Neither player has a dominant strategy.

   • Both players have a dominant strategy.

    

2. How many potential equilibria are there? (Hint: To see whether a given combination of strategies is an equilibrium, ask whether either player could get a higher payoff by changing his or her strategy.)

    

    

3. Is this game a prisoner’s dilemma?

    

     (Click to select)   Yes   No 

    

4. Suppose player A gets to buy his or her ticket first. Player B does not observe A’s choice but knows that A chose first. Player A knows that player B knows he or she chose first. What is the equilibrium outcome?

    

   multiple choice 3

   • Both players A and B buy movie tickets.

   • Both players A and B buy baseball tickets.

   • Player A buys a movie ticket and player B buys a baseball ticket.

   • Player A buys a baseball ticket and player B buys a movie ticket.

   (hint: which strategy gives A best payoff, given B will choose the strategy that maximize B’s payoff reacting to A’s)

    

5. Suppose the situation is similar to part d, except that player B chooses first. What is the equilibrium outcome?

    

   multiple choice 4

   • Both players A and B buy baseball tickets.

   • Both players A and B buy movie tickets.

   • Player A buys a movie ticket and player B buys a baseball ticket.

   • Player A buys a baseball ticket and player B buys a movie ticket.
     

0/10/A

Imagine yourself sitting in your car in a campus parking lot that is currently full, waiting for someone to pull out so that you can park your car. Somebody pulls out, but at the same moment a driver who has just arrived overtakes you in an obvious attempt to park in the vacated spot before you can. Suppose this driver would be willing to pay up to $10 to park in that spot and up to $30 to avoid getting into an argument with you. (That is, the benefit of parking is $10 and the cost of an argument is $30.) At the same time he guesses, accurately, that you too would be willing to pay up to $30 to avoid a confrontation and up to $10 to park in the vacant spot.

 

1. Model this situation as a two-stage decision tree in which his bid to take the space is the opening move and your strategies are (1) to protest and (2) not to protest. If you protest (initiate an argument), the rules of the game specify that he has to let you take the space. Show the payoffs at the end of each branch of the tree.

    

   Instructions: If you are entering any negative numbers be sure to include a negative sign (−) in front of those numbers.

    

                                           $10 for you
                                           $0 for other driver

    

   If the other driver leaves a spot for you he avoids confrontation. If the other driver attempts to steal your spot then you can either protest or defer.

   $-20 for you $-30 for other driver   $0 for you $10 for other driver

2. What is the equilibrium outcome?

    

   You: $

    

   Other driver: $

    

3. What would be the advantage of being able to communicate credibly to the other driver that your failure to protest would be a significant psychological cost to you (for example, maybe a cost of $25)?

    

   The other driver will believe that you will

    

   multiple choice

   • protest if he attempts to steal your spot, so he'll leave the spot for you.

   • defer if he attempts to steal your spot, so he'll leave the spot for you.
     

C

The accompanying payoff matrix shows the payoffs (in millions of dollars) for two firms, A and B, for two different strategies, investing in new capital or not investing in new capital.

 

		Firm B
		Invest	Not Invest
Firm A	Invest	32 for A	70 for A
		20 for B	11 for B
	Not Invest	5 for A	10 for A
		51 for B	50 for B

 

An industry spy from firm A comes to firm B and offers to pay firm B in exchange for firm B's certain and enforceable promise to not invest. What is the most that firm A will be willing to pay firm B to not invest?

Multiple Choice

• $35 million

• $51 million

• $38 million

• $32 million

0

Refer to the accompanying figure. In this game, how many dominant strategies does Player B have?


note: there is either 1 dominant strategy or none

B

Lee buys first

A.Both Jordan and Lee will buy a ticket to the comedy.

B Both Jordan and Lee will buy a ticket to the documentary

C Jordan will buy a ticket to the comedy and Lee will buy a ticket to the documentary.

D Jordan will buy a ticket to the documentary and Lee will buy a ticket to the comedy.