1st Law of Thermodynamics

Thermodynamic system

Part of the physical universe with a specified boundary for observation

Open system

Can exchange heat and matter

Closed system

Can only exchange heat

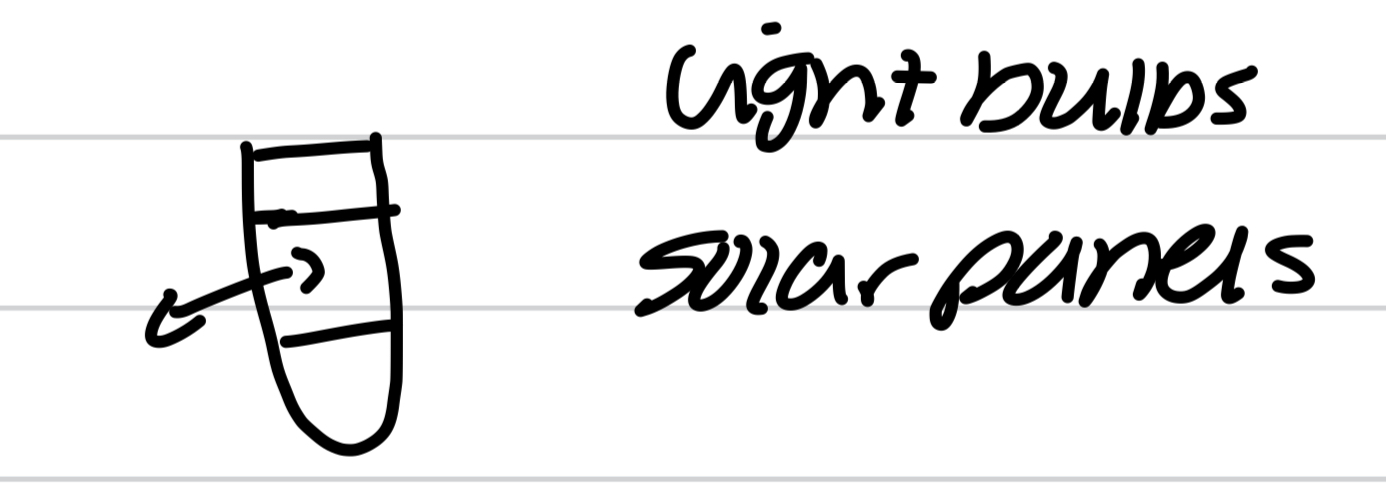
Isolated system

Can’t exchange heat or matter

Isothermal change

Temperature of the system stays the same as the surroundings

Only closed system

Diathermal wall

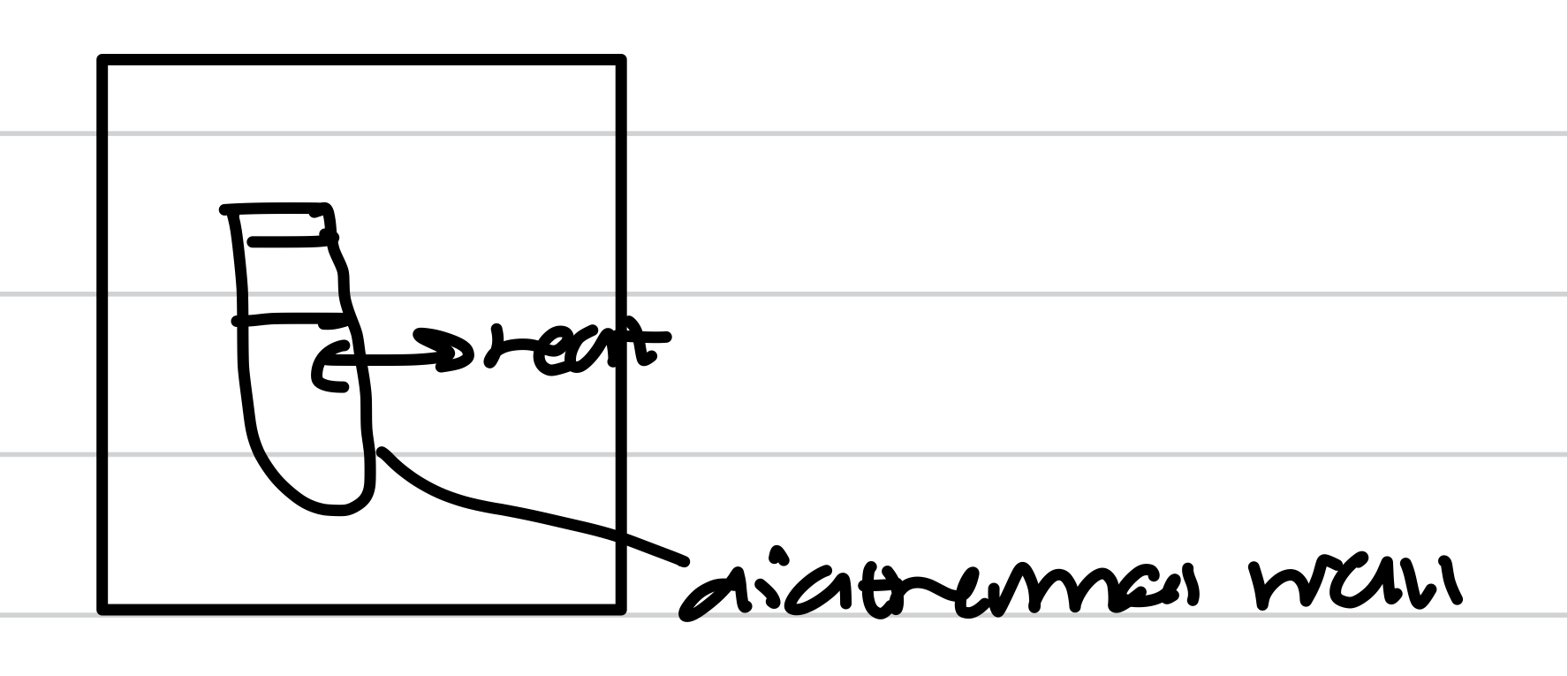
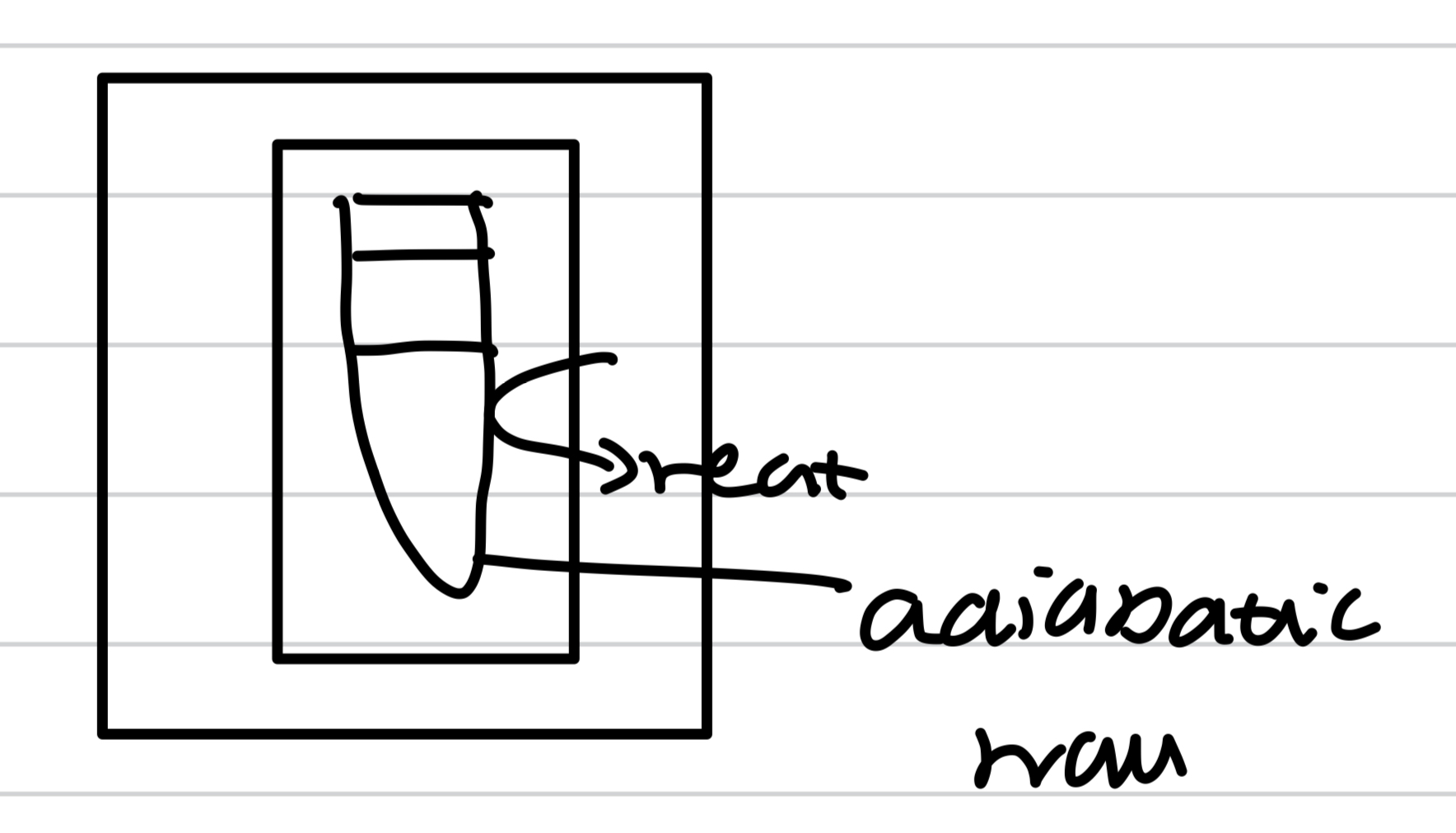
Adiabatic change

No heat exchange between the system and the surroundings

Only isolated system

Adiabatic wall

Earth

Closed system

Exchanging light and heat with the surroundings

Only have very minor exchange of matter

First Law of Thermodynamics

The law relates the internal energy, U, of a system to the amount of heat added to the system and the amount of work done on the system

Work

Work is done when a force is applied to an object and that causes that object to move a distance

Work = distance x opposing force

Opposing force

Mass x -gravity

Total internal energy, U

U of an isolated system is constant

ΔU = q+w

change in internal energy = heat added + work

Conservation of energy

The total internal energy of an isolated system is constant; energy can be transformed from one form to another but can be neither created or destroyed

Energy, E with units of Joules, J

Interconvert energy

Kinetic energy

Chemical energy

Nuclear energy

Heat, work and light

Applies to:

• atoms and molecules

• chemical bonds

• photons and electrons

• biology, engineering, Earth science

Work and heat

Energy can be exchanged between systems/surrounds as work, w or heat, q

U, w, q are key to thermodynamics

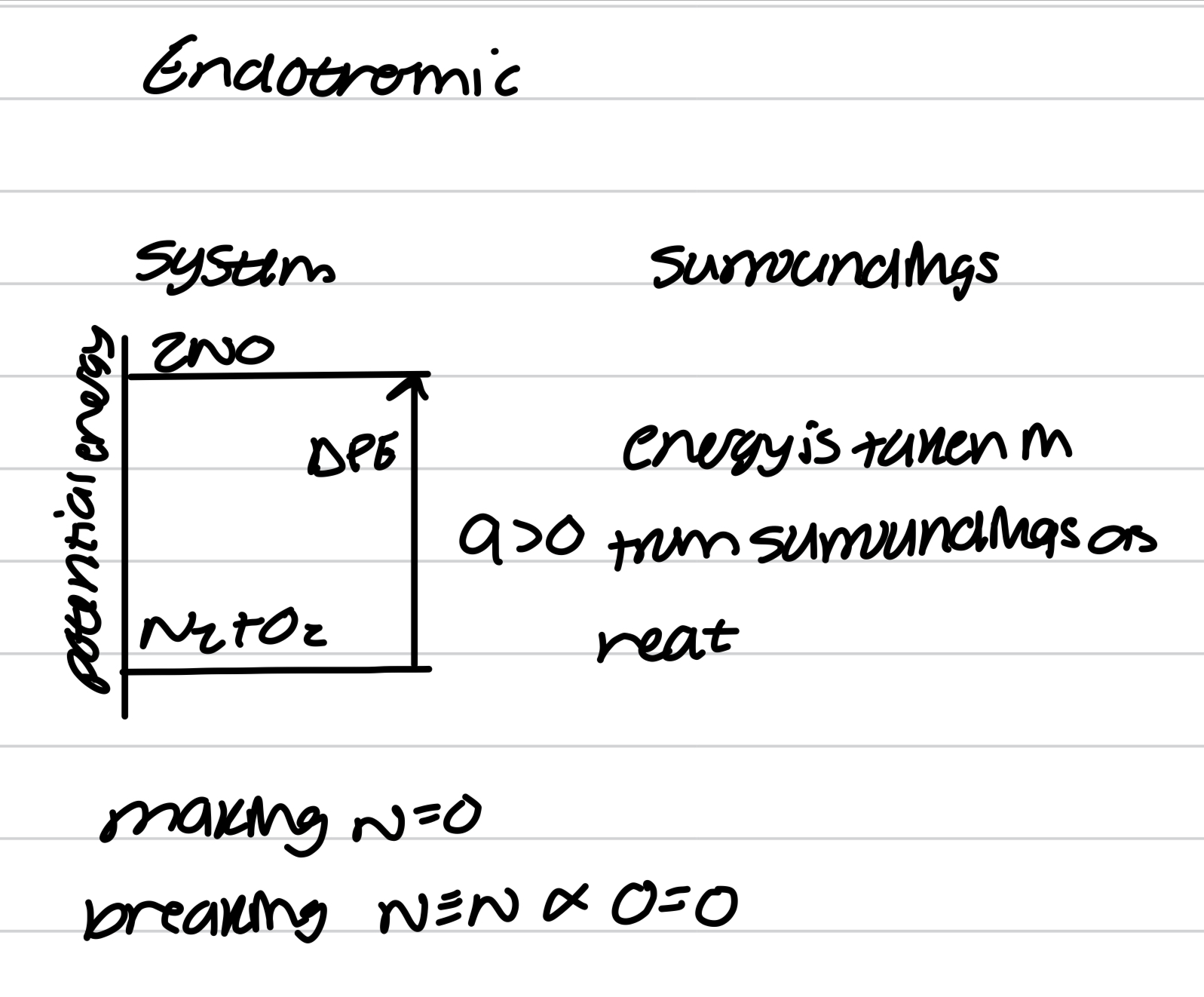
Exothermic exchange of heat

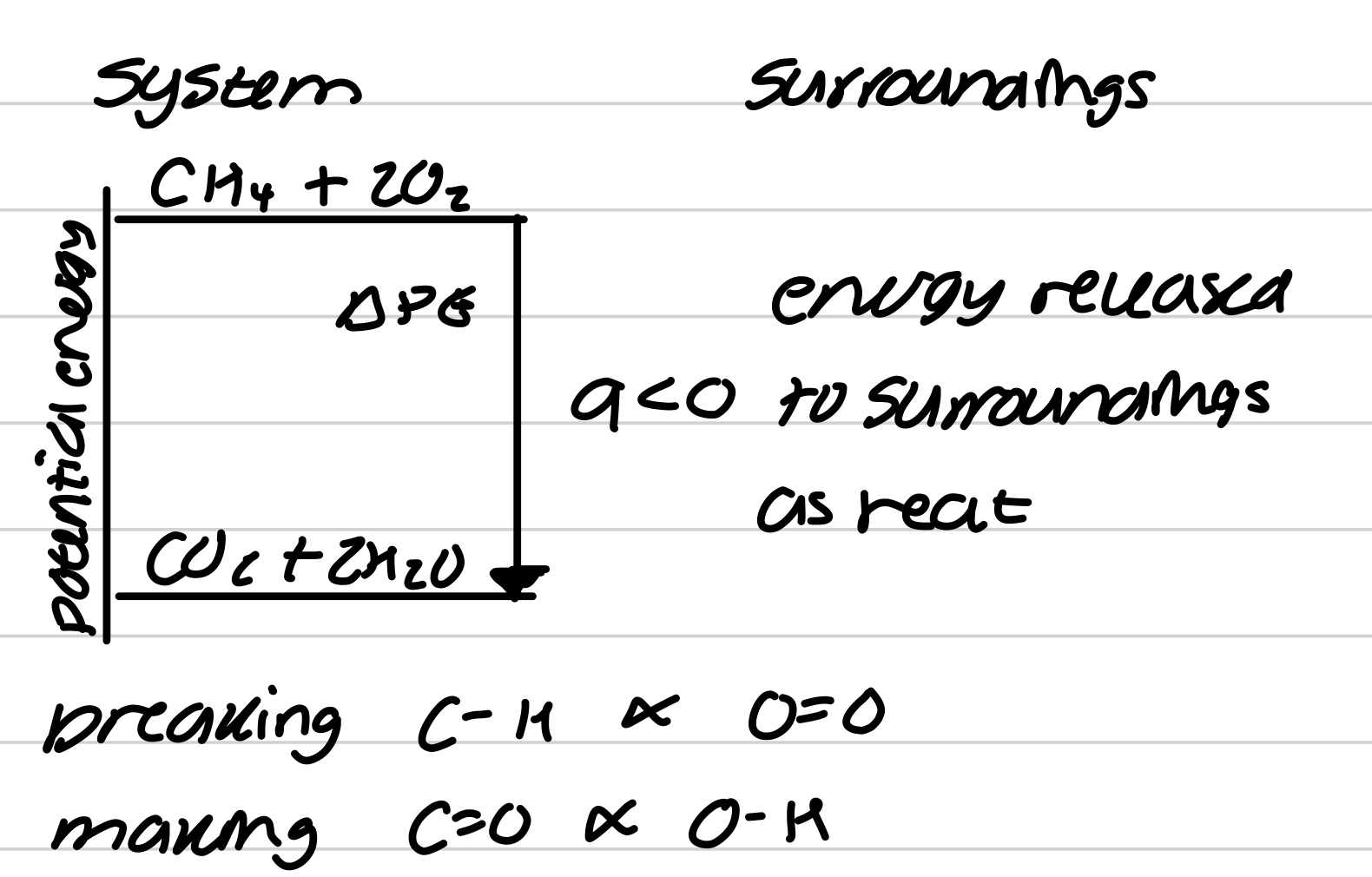
Endothermic exchange of heat

Work and heat in terms of molecular motion

Molecules in uniform motion, energy is transferred to surroundings as work

Molecules in chaotic motion, energy is transferred to surroundings as heat

Energy is the capacity to do work

In an open system, the CO2 would push the air out of the way so still doing work on the surroundings

Work is done by the system on the surroundings because the gas pushes the weight upwards as it expands. The weight represents the pressure of the atmosphere → w<0

w>0 when gases are being consumed → the surroundings are doing work on the system

Expansion work

A gas expands and does work against external pressure, Pex

Opposing force = Pex x area

Work = force(F) x distance(s)(height,h)

= (Pex x A) x h

= Pex x ΔV

When w<0 system loses energy → w=-PexΔV

At constant pressure and temperature

Pex=Pgas

pΔV= -nRT → ideal gas equation

w=-nRT → only when we have perfect reversibility and theoretical max work obtained

Δn = (nfinal - ninitial) is the change in the number of moles of gas

Reversible expansion

Constant P+T conditions rarely realistic → complex maths

Maximum work obtained is when expansion reversible otherwise energy lost (wasted) as external heat, sound, etc

Process can be reversed with minimum effort

Key assumptions for reversible expansion

No temperature or pressure gradients - constant

System at equilibrium throughout

Internal energy

A measure of all of the energy reserves of a system

Um = molar internal energy → energy of 1 mol Jmol-1

ΔU = change in internal energy

Enthalpy changes

Measured at constant pressure in open systems

Standard states and standard conditions

ΔUθ/AU° = standard conditions

Gas - pure gas at 1 bar (1×10^5 Pa)

Liquid - pure liquid at 1 bar

Solid - pure solid at 1 bar

Solution 1moldm-3 concentration

Temperature unless stated but 298.15K

Enthalpy changes of state

ΔvapHθ - liquid to gas → -ΔconHθ

ΔconHθ - gas to liquid

ΔfusHθ - solid to liquid

Reference state

Is the most stable state at standard temperature and pressure

Enthalpy change of formation, ΔfHθ

When 1 mole of substance is formed from elements in their reference states at stated temperature

What does dissociate mean

Separates to infinite distance

Bond dissociation energy

BDE

Enthalpy change when a specific bond in a compound dissociates

Bond energy/enthalpy

BE

Mean bond energy

Mean of the BDE values for similar bonds within a specific compound

Tabulated Average Bond Energies

ABE

Apply yo a type of bond across a range of related compounds

Estimations but some values are annotated with a → true BDE values as there exists only one compound containing the bond

Enthalpy changes of reaction, ΔrHθ

Enthalpy change when molar quantities of the reactants as stated in the end react together under standard conditions with all substances in standard states

Pay attention to:

• temp

• states

• stoichiometry

State functions

Depends only on the current state of the system as defined by the temperature and pressure

Any change in the value of state function depends only on initial and final conditions, not route taken

Hess’s Law

The standard enthalpy change for a reaction is the sum of the standard enthalpy changes to the reactions into which can be divided

Cas use:

• bond enthalpies

• reaction enthalpies

• separate particles

• any other reference info

• standard enthalpy of formation

ΔrHθ equation

ΣvΔfHθ(products) - ΣvΔfHθ(reactants)

The Zeroth law

If 2 systems are each in thermal equilibrium with a third, they are in thermal equilibrium with each other → allows us to do experiments

Only absolute temps

Uses K

Bomb calorimetry

Used to measure temp changes at constant volume

No work can be done at constant V so w=0 and ΔU=q

Isolated system → adiabatic

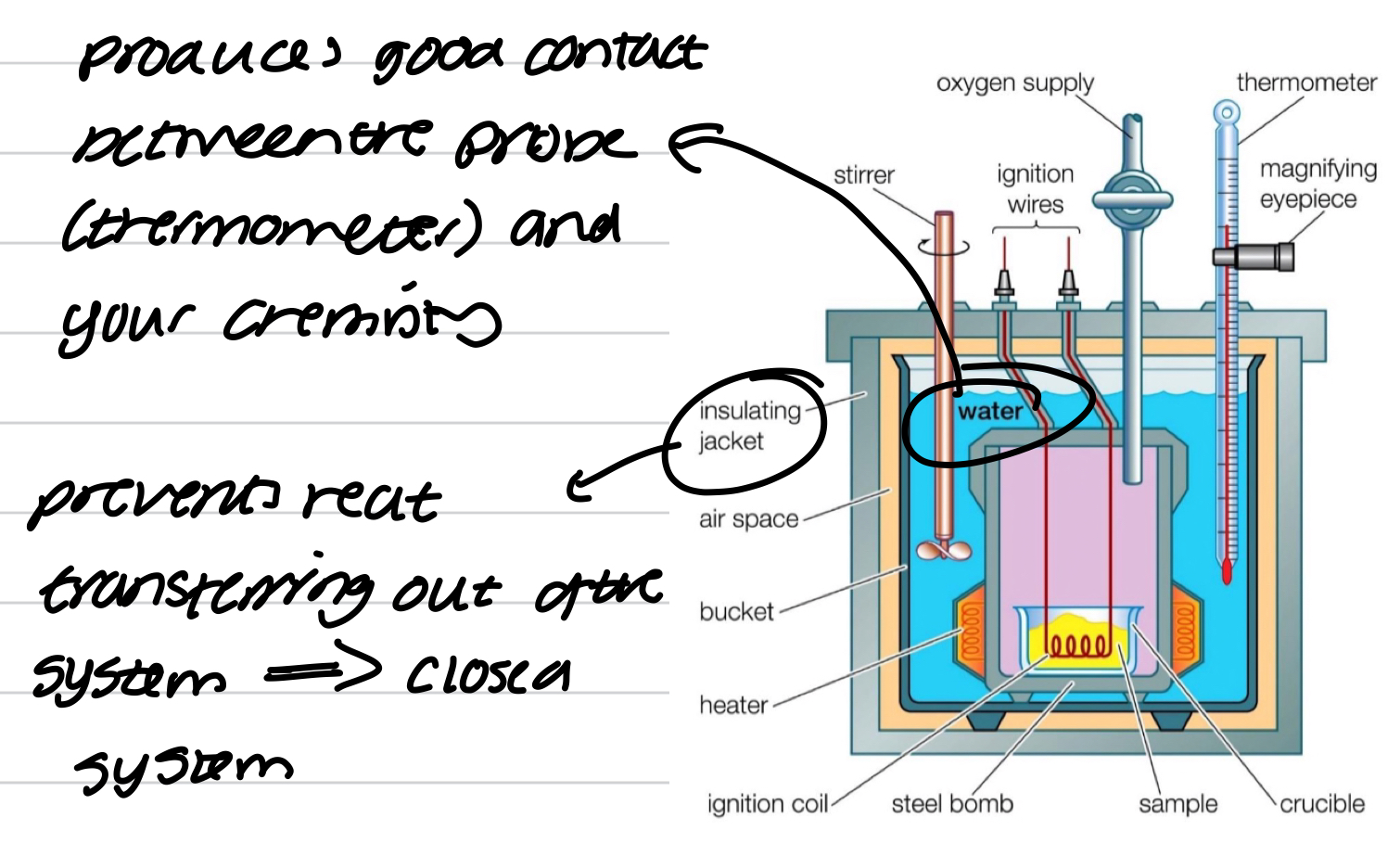
Method for bomb calorimeter

1. measure heat (temp) evolved by RXN

2. Use electricity to get to the same temp change again → power

Heat exchange in open systems

Internal energy changes as the energy is rearranged

Energy transferred between the system and surroundings as heat and/or work

ΔU = q + w

ΔU = q + pΔV

Experiments to measure enthalpy change

Everyday chemical changes occur in open systems that have constant pressure, not constant volume

Open calorimeter, q=ΔH

Enthalpy change

Heat transferred at constant pressure

ΔU = ΔH + w

ΔU = ΔH - pΔV

ΔH = ΔU + ΔnRT

Δn = moles of gas in products - moles of gas in reactants

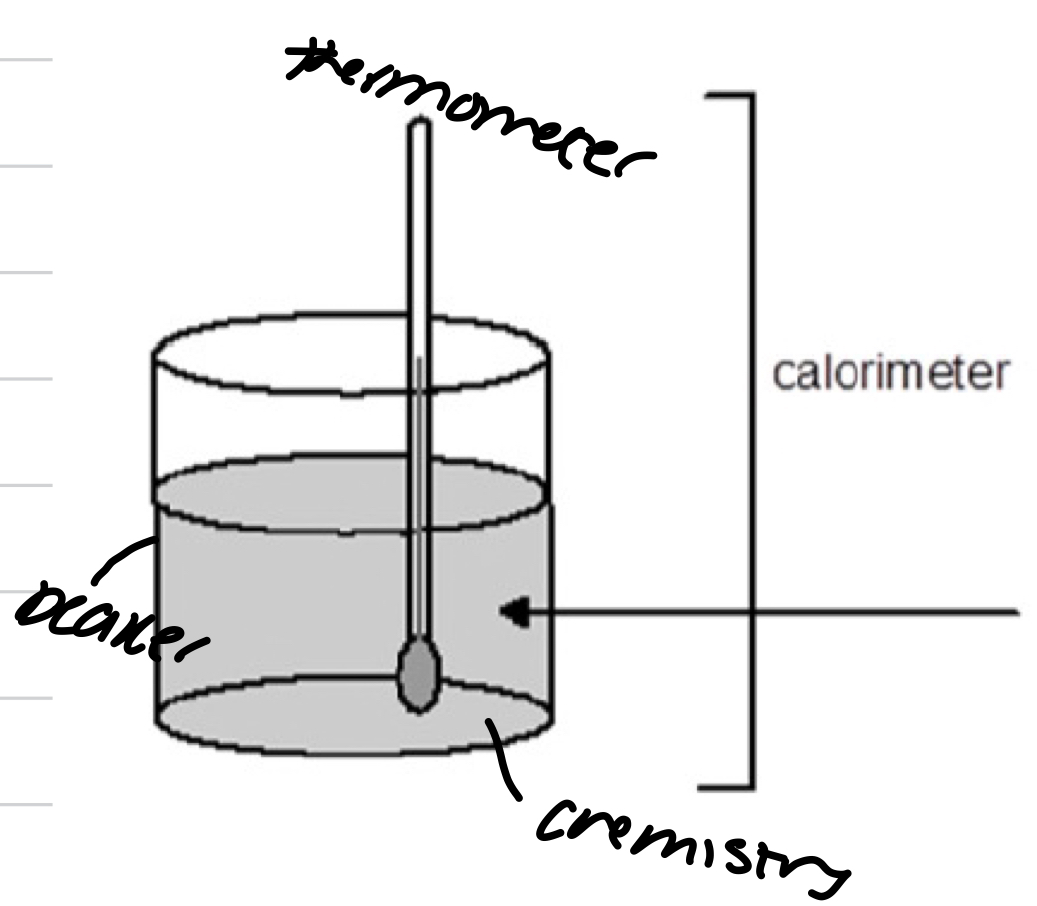
Experiment 1

Open system

Need to take into account for heat leakage

Need to consider heat capacity

RXN takes place in solution and is part of the surroundings

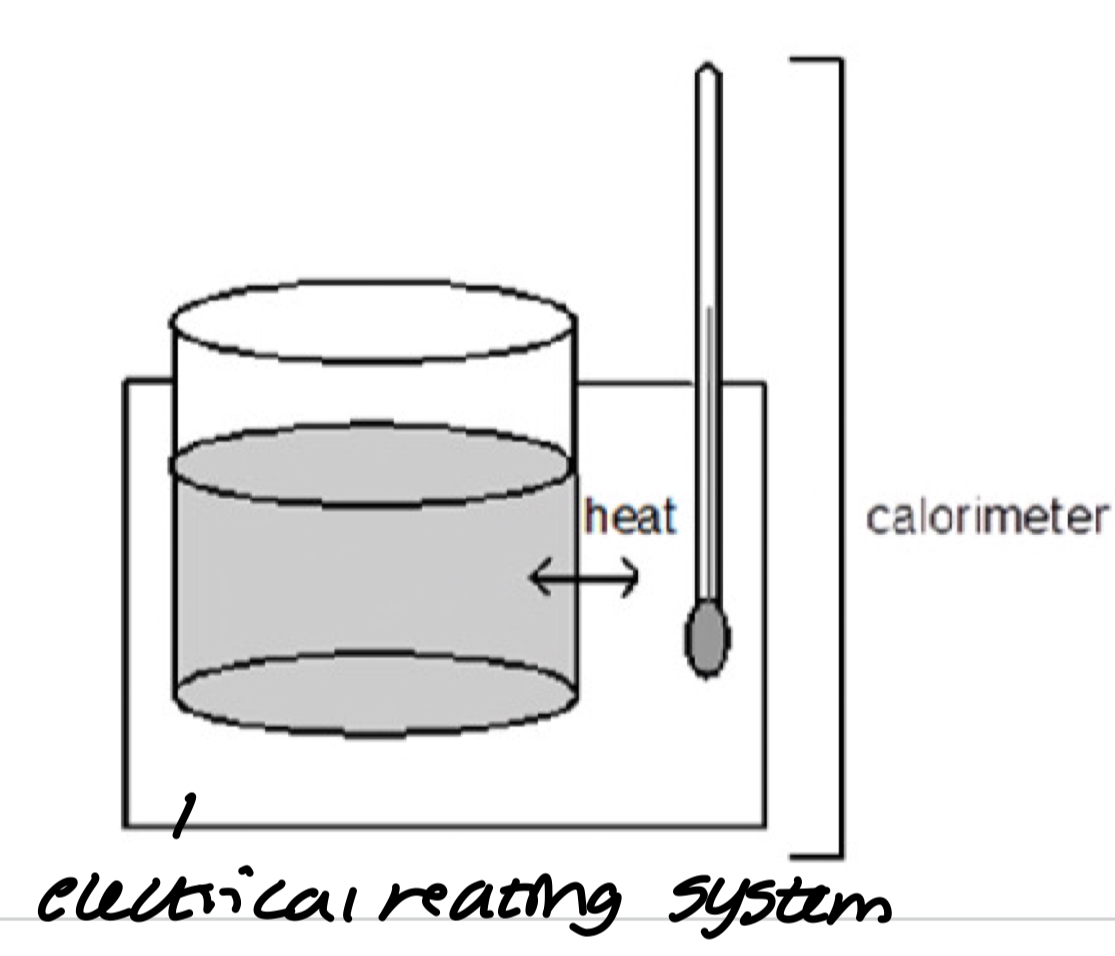
Experiment 2

Improvement on 1

Using electrical heating system to measure the heat capacity of the calorimeter

1. measure heat rise from the chemistry

2. replicate change in temp using electrical energy ΔH=ΔEelec

Experiment 3

Accurate research lab-solution

1. Use a bomb calorimeter to measure ΔU - constant volume

2. convert ΔU to ΔH using ΔH = ΔU + ΔnRT

Explosions with work and enthalpy

Rapid and extreme increase in volume accompanied by energy release

Usually characterised by temperature increase and generation of gases

Explosive effect comes from:

• production of gases

• Expansion of gases on heating

Standard enthalpy of combustion

The value of ΔrH⦵ for complete combustion of one mole of fuel is the standard enthalpy change of combustion ΔcH⦵

Everyday uses of fuels

Heating - 16% of CO2 emissions

Transport - 21% of CO2 emissions

Electricity generation - lighting

Waste disposal - incineration

Methane and coal

Coal mainly carbon: C(s) + O2 (g) → CO2(g)

Natural gas mainly methane: CH4(g) + 2O2 (g) → CO2(g) + 2H2O(l)

To generate power, work must be done → need large ΔnRT → to turn turbines and generate electricity

Use external combustion engine/steam engine to turn water into gas

This assumes all the heat from the RXN is being used to turn water into steam

Fuel metrics

Compare fuels in quantitative way

Economic → price, weight, volume

Environmental → CO2, NOx, O3, particulates

Carbon load of fuels

Energy per unit mass

|ΔcH|/RMM in MJkg-1

By volume: energy density and mass per unit volume in MJm-3

Focus on biofuels

Produced via contempory biological processes eg agriculture or anaerobic digestion

Potentially carbon neutral

Bioethanol added to petrol → reduces carbon load

Larger alcohols show promise as petrol replacements → similar combustion characteristics

Biodiesel used for lorries and heavy vehicles

As O2% increases, ΔcH decreases

Biofuels are already part oxidised

Biofuel issues

Land-use/food vs fuel/ soil erosion/loss of biodiversity

Poor fuel metrics

New chemistry/new engineering

Cryogenic fuels

High energy density

Typically H2 → liquified and stored at low temp sub 33K

Good fuel metrics

Realistic energy densities = taken into account

Inconvenient:

• storage

• hazardous

• need O2 supply

Hypergolic fuels

A two-component propellant combination that spontaneously ignites on mixing

Hypergolic fuel disadvantages

Highly corrosive and toxic

Lots more chemistry to research and understand

Hypergolic fuel advantages

No need for air → good for space

Liquid at 290K → easy storage

Near-instant conversion of chemical energy to heat and work

No need for ignition control

Excellent fuel metrics

Heat capacity

Heat supplied/temp change

For single substance, specific heat capacity, Cs = heat capacity per unit mass JK-1g-1

q=m x Cs x ΔT → m is mass of substance

Tells us what is happening to the energy, in molecular terms, when you heat a substance

Water specific heat capacity

Large specific heat capacity

Many everyday applications

Climate

Central heating

Industrial cooling

Molar Heat Capacity

Cm, heat capacity per mole JK-1mol-1

q = n x Cm x ΔT where n is moles of the substance

For gases, two values of Cm:

• molar heat capacity at constant pressure Cp, JK-1mol-1

• molar heat capacity at constant volume, Cv, JK-1mol-1

H and U changing with temp

Enthalpy and internal energy both increase with temperature

ΔH (increase of enthalpy for ΔT) =CpΔT

ΔU (increase of internal energy for ΔT) = CvΔT

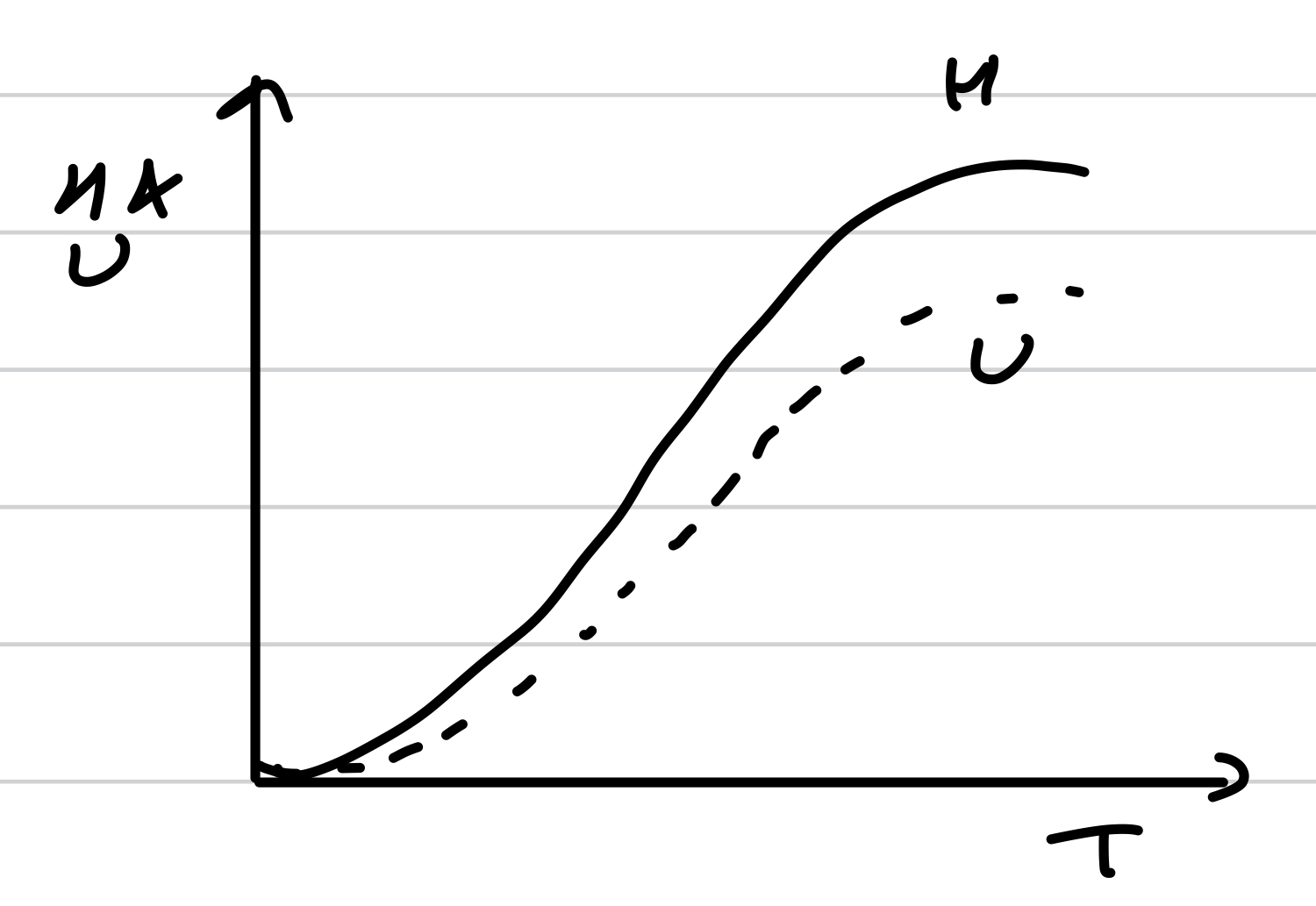
Relation between Cp and Cv for a perfect gas

Hm = Um + pV

Hm = Um + RT

ΔHm = ΔUm + RΔT

ΔHm/ΔT = ΔUm/ΔT + R

Cp = Cv + R

when n=1

Enthalpy change of solution, ΔsolnH⦵

Heat exchanged at constant pressure upon changing to aqueous phase

Change ΔH with temperature

Enthalpy increases with T

H2 = H1 + nCpΔT

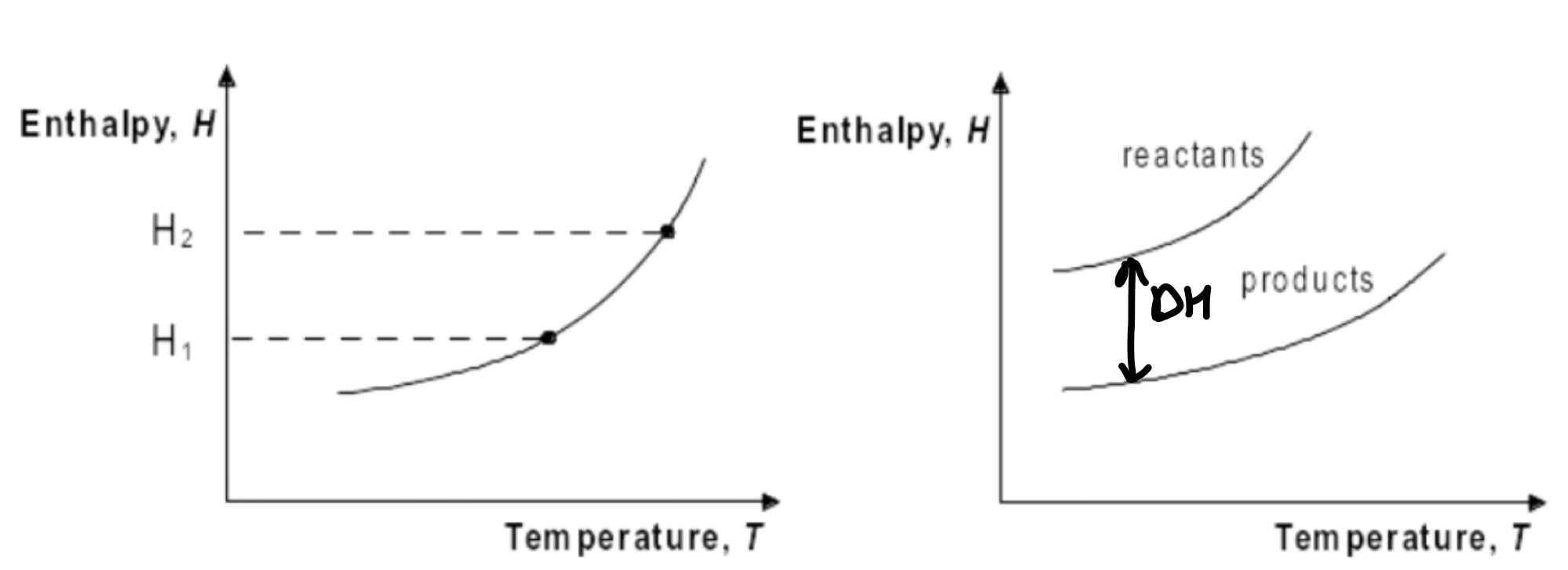
Kirchkoff’s Law

Using to calculate change ΔH with temperature

ΔrHT2 = ΔrHT1 + ΔrCpΔT at constant pressure

ΔrCp = ΣvCp(products) - ΣvCp(reactants) if you cross a phase boundary, will need to adjust the values

This assumes Cp itself doesn’t change with temperature