BF Ch11 capital budgeting and risk

the IRR and NPV analysis of an investment porject should reflect …

the riskuness of the project

the Ka = WACC should be hustified for project risk

→ risk adjustment discount rate (RADR) = k*a = WACC*

why should we adjust for project risk and use K*a or RADR

if we use Ka (instead of K*a) for all investment projects we will

• reject low-risk projects that should be accepted

• accept high-risk projects that should be rejected

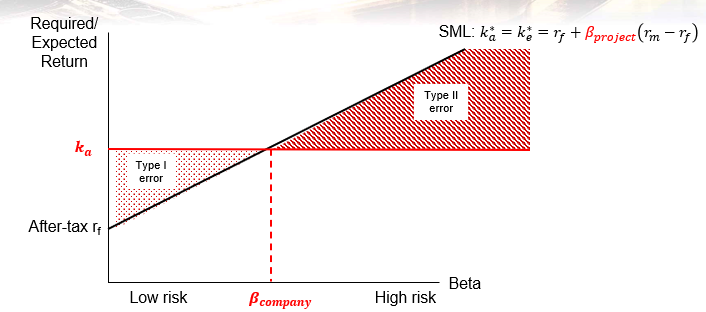
low project risk : project risk < company risk

K*a < Ka

cost improvement projects

typical prokect risk : project risk = company risk

K*a = Ka

expansion of existing business

high porject risk : project risk > company risk

K*a > Ka

speculative ventures

how do we calculate the approprate BETA project ?

can be observed as the company beta of a company for which the existing buisness is similar to the investment project

• reflects : operational risk + financial risk of the observed company

unleveranged project Beta

removes the financial aspect of the Beta (of the observed leveranged beta)

leveranged project Beta

add the financial risk of the investing company

IRR and NPV analysis only adjust for (what type of risk)

market risk via the beta

total risk

specific risk (the risk of firm specific events)

+ maret risk (the sensivity to general economic events)

when does specific risk matters

• for investors that do not or cannot diversify, like owners of small firms or employees

• when firm failure (due to dramatic firm-specific events) reuslts in negative social effects, like bank failure, infrastructure failure or healthcare failure

techniques that consider TOTAL risk

NPV-payback approach

simulation approach

scenario analysis

sensitivity analysis

certainty equivallent

NPV-Payback approach

a project must have a positive NPV and a payback period of less than a critical number of years to be acceptable

• the longer the payback period the higher the probability of a firm-specific project failure

simulation approach

• estimate probability distribution of each input variable (price, number of units sold, unit production costs, unit selling cost, annual depreciation)

• combine input variables into a mathematical model to compute the NPV of the project

• select at random a value of each input, based upon the probability distribution (step 1)

• repeat step 3 and 4 many times to arrive at the following : the project expected NPV (mean), the standard deviation of the NPV

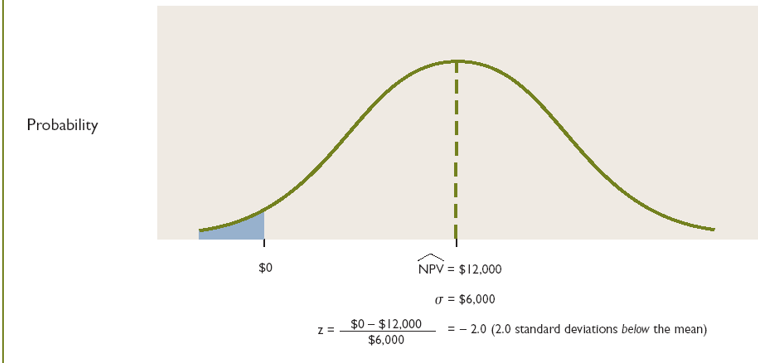
scenario analysis

considers the impact of simultaneous changes in the input variables on the acceptability of an investment project

define the different scenarios based on the sumultaneous values of the input variables : an optimistic, a pessimistic and a most likely scenario

estimate the probability of each scenario

compute the NPV under each scenario

compute the expected NPV

compute the standard deviation

compute the z-score and associated probability of failure

sensivity analysis

involves systematically changing each input variable separately to identify which input variables have the most impact on the NPV → identifying the KEY VARIABLES

putting additional efforts in the estimation and or improvement of the key variables

wat doen we bij verschillende risiconiveaus

• certainty equivalent approach (aanpassing cashflow)

• risk adjustment discoynr rate (RADR) (wijziging risicovoet)

  → beta (un) leveraged

at =

certainty equivalent factor

tussen 0 en 1

hoe hoger at hoe zekerder de cash flow

cashflows verder in de toekomst hebben een lagere at → wegen dus minder zwaar door op de NPV

als een onderneming een risicovol project uitvoert, gaan de investeerders hun …. aanpassen

vereist rendement Ke

B leveraged

operationeel risico + financieel risico