IB SL Physics all units

making degrees smaller

• 1º = 60’ (minutes of arc)

• 1’ = 60’’ (seconds of arc)

• 1º = 3600’’

parallax

• the apparent shift of a near star compared to the distant stars over a period of time

• a star will look like it has moved backwards and forwards throughout the year

• really it is Earth that has moved which changes the perspective from which we look

• other stars will look like they haven’t moved because they are so far away

angle of parallax and finding it

• angle of parallax is measured in seconds of arc, when it is 1’ the distance is one parsec

• further away = smaller angle of parallax

• distance between earth’s position in June and in December is 2R, p = parallax angle

• the distance to the star is Tanp = R/d therefore d=R/tanp

• using small angle approximation:

• tanp = p therefore d=R/p (when the angle is measured in radians)

finding distance in parsecs

when these distances are too approximate

d(parsec) = 1/p (arc-second)

angles smaller the 1/100 arc seconds are too small to measure accurately so distances farther away than about 100 parsecs are too approximate to be useful

luminosity

stars are assumed to radiate like black bodies (emissivity =1), equation is P=eσAT^4

luminosity = power of a star

L=σAT^4

• power

• efficiency

• σ — constant

• area — can be written as area of a circle (stars are spheres but we see circles)

  • L=σπR^2T^4

• temperature

combining brightness and luminosity equations

surface are of a sphere = 4πR^2

brightness is the power per unit area received at a distance from the source

b=L/4πR^2

luminosity cannot be directly measured but it can be calculated from brightness and distance found using parallax

combining the two formulae (not in data booklet)

b=σAT^4/4πd^2

• d = distance from us to star

stellar spectra and Wien’s law

stellar spectra — below 400nm = ultraviolet radiation at a hotter temperature

Wien’s law — λmaxT = 2.9x10^-3 mK

• because the product is a constant λ and T are inversely proportional

the smaller the maximum wavelength, the greater the temperature

classifying stars based on temperature

stars are separated into classes based on their temperature

• hot to cold = O, B, A, F, G, K, M

• our sun is class G

• the hottest stars are blue and the coldest are red

Hertzsprung-Russell (HR) diagram

• stars age following the main sequence from top left to bottom right

• temperature is measured from highest to lowest — pay attention to the axes

• L star/L☉ is used for the y axis, ☉ represents the sun, ratio of Lstar to L☉

• y axis is exponential

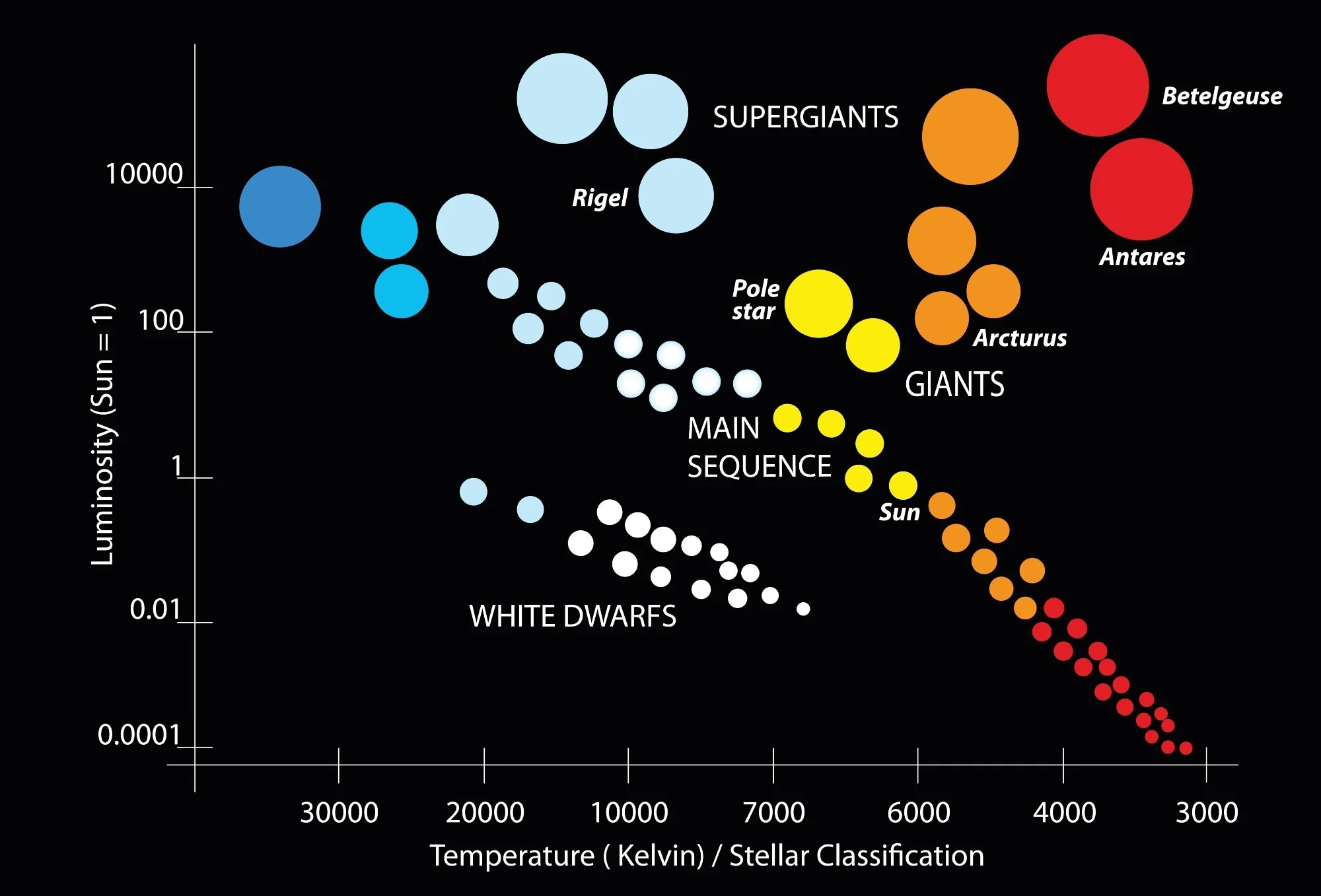
brightness and luminosity of the super giants, relative to the sun

why there aren’t super giants in the bottom left of the HR diagram

• super giants have to be incredibly big to be brighter than the sun given the equations for brightness and luminosity

• no super giants in the bottom left corner because the temperature is too high (relative to the luminosity), we don’t see objects of that temperature in the universe

linking luminosity and mass for main sequence stars

for stars in the main sequence the luminosity and mass (M) of the star can be linked

L=σAT^4

A=4πR^2

L∝R^2

L∝M^3.5

• 3.5 comes from the exponent for the main sequence gradient

• the gradient is not negative because the x axis goes from high to low

impact of a difference in mass on luminosity

• a slight difference in the mass causes a huge difference in luminosity

  • a star with a mass 10 times that of the sun will have a luminosity of 10^3.5 times the luminosity of the sun which is about 3200

• stars have different densities

• more luminous stars on the main sequence have greater mass and shorter lives than less luminous stars on the main sequence

single star

A luminous sphere of plasma held together by its own gravity.

binary star

two stars orbiting a common centre

black hole

A singularity in space-time.

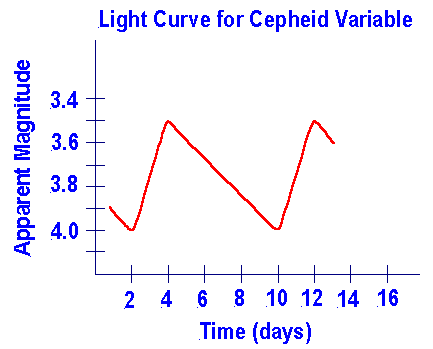
cepheid variable

A star with a period of varying luminosity. The luminosity can be determined from the period and along with the apparent brightness can be used to determine the distance of the star from Earth.

cluster of galaxies

Two or more galaxies that are close enough to each other to affect each other through gravitation.

constellation

A pattern of stars visible from Earth that are not gravitationally bounded.

dark matter

Matter in galaxies that are too cold to radiate. Its existence is inferred from theoretical physics rather than direct visual contact.

galaxies

stars, gas, and dust held together by gravitational forces.

main sequence star

A normal star that is undergoing nuclear fusion of hydrogen into helium.

neutron stars

A very dense star, consisting only of uncharged neutrons

nebula

A cloud of dust, hydrogen, helium and other ionized gases.

planet

 A celestial body that orbits a star.

planetary system

Gravitationally bounded non-stellar objects in orbit around a star or star system.

planetary nebula

The ejected envelope of a red giant star.

stellar clusters

A group of stars gravitationally bounded together.

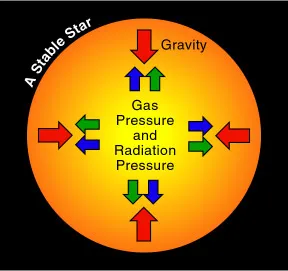
the stability of a star

• depends on the equilibrium of opposing forces

• forces = gravitational, gas and radiation pressure

• equilibrium gained through nuclear fusion which keeps the star hot

cepheid variables graphed

apparent magnitude of luminosity vs time

stellar evolution for less than 1.4 solar masses (solar mass of the sun = 1)

• star stops fusing at about oxygen as the amount of energy released decreases

• planetary nebula occurs (big explosion)

• outer layers of the star blow away (gravity does not keep them together)

• the core (leftover) becomes colder and colder, it contracts more and more (gravity)

• electrons behave as a gas in the core and the pressure they generate stops the core from contracting

• it becomes a white dwarf, cools and becomes invisible

Chandrasekhar limit

The largest mass a white dwarf can have is about 1.4 solar masses.

• electron degeneracy prevents further collapse of the core provided that its mass is less than about 1.4 solar masses

• the star will become a stable white dwarf then a black dwarf

• dwarf = no fusion = dead star

Oppenheimer-Volkoff limit

the largest mass a neutron star can have is approximately 2-3 solar masses

• when the mass of the star is 1.4-2.5 solar masses it is a neutron star

• the core will collapse further (due to weight) until electrons are driven into protons forming neutrons

• neutron pressure prevents the star from collapsing further which makes it a neutron star

• neutron stars are heavy because there is no electromagnetic repulsion

when the Oppenheimer-Volkoff limit is exceeded the star becomes a black hole

Hubble’s law

describes the speed at which celestial bodies move away from each other at the present time and changes because the expansion of the universe if accelerating.

v=Hd

• v = velocity

• H = Hubble parameter

• d = distance

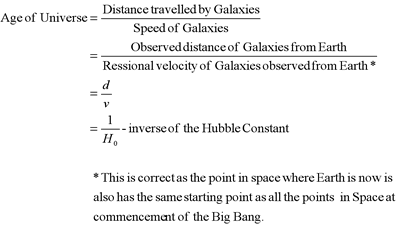
using Hubble’s law to find the age of the universe

• using the relationship between speed, distance and time

• the age of the universe is the inverse of the Hubble constant

the cosmic scale factor (R)

a function of time which represents the relative expansion of the universe.

This may be represented by

where d(t) is the proper distance at time t, d0 is the distance at time t0, and a(t) is the cosmic scale factor.

Astrophysicists would out the cosmic scale factor using Einstein’s theory of general relativity laws.

Doppler effect in galaxies

• light has a longer wavelength than when it was originally emitted

• red shift (z) = unitless measure of the speed of an object as a proportion of the speed of light

• change in wavelength due to velocity

• galaxies rotate so light is doppler shifted

• the radiation from the side approaching the earth is blue shifted and the radiation moving away from the earth is red shifted

velocity of recession

Hubble constant

velocity of recession = Hubble constant x distance

Hubble constant has units of k ms^-1 Mpc^-1

V=H₀d

z=H₀d/c

d=cz/H₀

uniform circular motion

• circular motion at a constant speed

• changing angular velocity and angular acceleration

• acceleration is always directed toward the centre of the circular path

• period is the time taken to complete one circle

centripetal force

• causes the centripetal acceleration

• towards the centre of the circle

• F=Mv²/r

• does no work, is a net force

centripetal acceleration

• gives rise to circular motion

• directed toward the centre of the circle

• perpendicular to instantaneous velocity

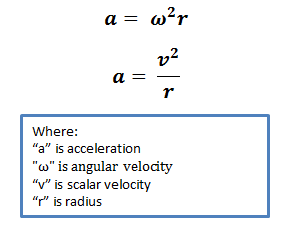
gravitational field strength

• g=F/m

• 9.81ms^-2 on earth

oscillations

any vibration that goes back and forth without an overall resultant displacement

describing oscillations — displacement (x)

the distance the particle has moved from its rest position (equilibrium)

describing oscillations — amplitude (A)

the maximum distance that a particle moves from its rest position (this is a scalar!)

describing oscillations — frequency (f)

the number of oscillations per second or the number of waves to pass a point every second (also a scalar because of time), f=1/T

describing oscillations — period (T)

the time it takes for an oscillating particle to complete an oscillation or the time for a wave to move through one complete wavelength

graphing oscillations

• on a sine wave, movement would continue infinitely without friction

• sine waves come from circular motion looking at vertical or horizontal displacement from a point on the circumference of the circle

• time on the x axis, displacement on the y axis

• the length between repeating parts of the sine wave is the time period, direction matters in this eg. from halfway going up the time period would need to be measured until it hits the line going up again (not the first point where it would be going in the other direction)

• amplitude on the wave is symmetrical on the horizontal access and the space between the extremes and the x axis

relating displacement, velocity and acceleration in SHM

• velocity is the derivative of displacement

• acceleration is the derivative of velocity, the derivative of the derivative of displacement

• displacement cannot be represented as a derivative but it is the anti derivative of velocity (the integral)

• displacement lags T/4 behind velocity, 3T/4 ahead (out of phase by…)

• velocity lags T/4 behind acceleration, 3T/4 ahead

• displacement lags T/2 behind acceleration (can go either way as it is in the middle)

conditions for SHM

• There is a fixed cyclical path.

• There is a central equilibrium point.

• The motion repeats at equal time periods (periodic).

• Displacement, velocity, and acceleration change continuously.

• There is a restoring force directed toward the equilibrium point.

definition of SHM

• motion arising from acceleration of an object that is proportional to its displacement from a fixed equilibrium point (rest position) and directed toward that point.

• motion arising from a linear restoring force directed to a fixed equilibrium point

when is there a phase shift? what does it mean?

Two sinusoidal curves of equal period have a phase shift that translates one curve relative to the other in time, expressed as a fraction of a cycle, or as an angle in radians or degrees

travelling wave

a disturbance that propagates (transmits) energy without transferring physical material (a medium)

mechanical wave

A mechanical wave propagates its disturbance through a medium by an oscillating movement of the particles in the medium

transverse wave

particles of the medium oscillate perpendicular to the direction of the wave and propagation of energy.

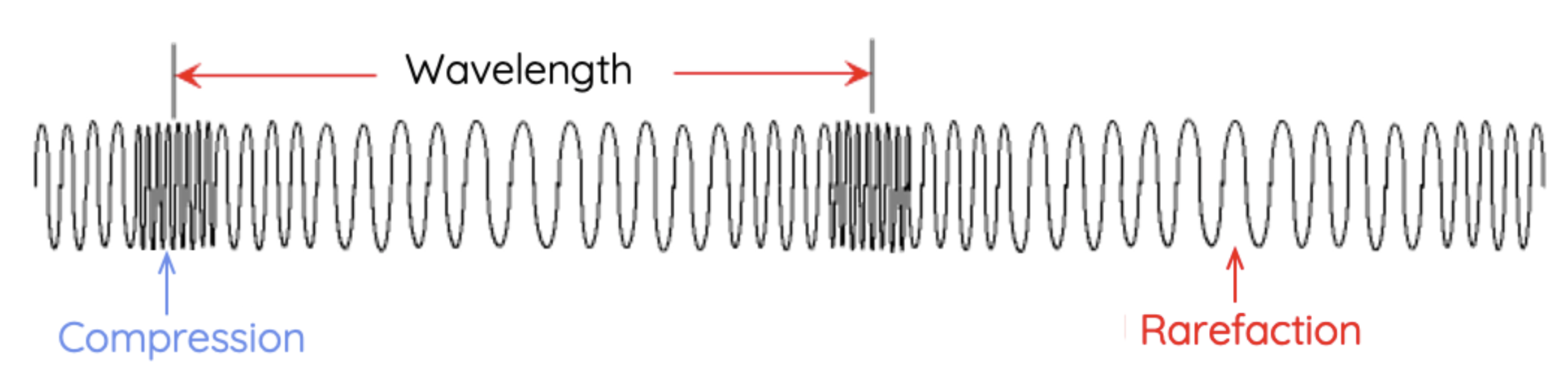
longitudinal wave

the particles of the medium oscillate parallel to the direction of the wave, the wave and oscillations follow the same direction

electromagnetic wave

propagates as a transverse wave through a medium or a vacuum by oscillations of coupled, time-varying electric and magnetic fields

how is wave length measured?

• wavelength (λ) is measured in metres as is amplitude (A)

• wavelength is peak to peak while amplitude is vertical from the x axis to the peak

definition of a ray

• the line showing the direction in which a wave transfers energy

  • direction of propagation, the two are parallel

  • perpendicular to the wave front

definition of a wave front

• the surface that travels with a wave and is perpendicular to the direction in which the wave travels (the ray)

  • the lines that are perpendicular to the ray

  • parallel to the direction of propagation

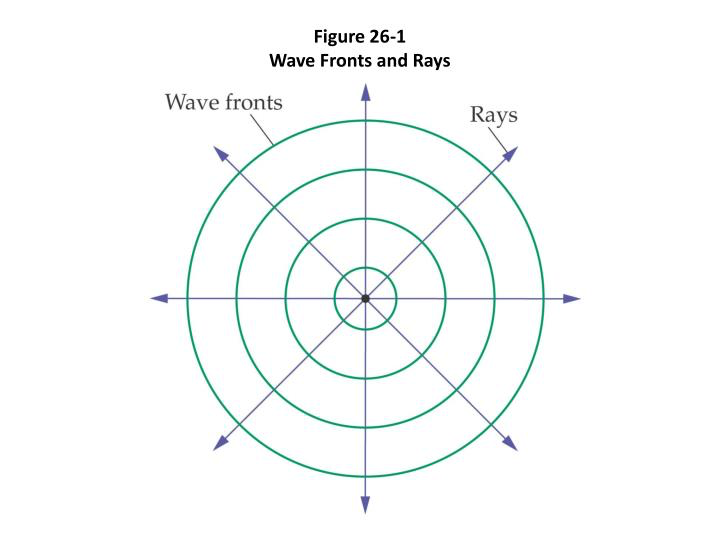
wave fronts and rays from a point sources

• this diagram shows a point source so makes waves that propagate away from the point, making the wave fronts circles

• the rays are parallel to the tangent of the circle — if you zoomed in enough the curve would look flat

• the rays can be perpendicular to the wave front as the tangent will always be a straight line

superposition

• the resultant displacement at any point is the sum of the seperate displacements due to the two waves

  • the way we add waves, like dealing with vectors

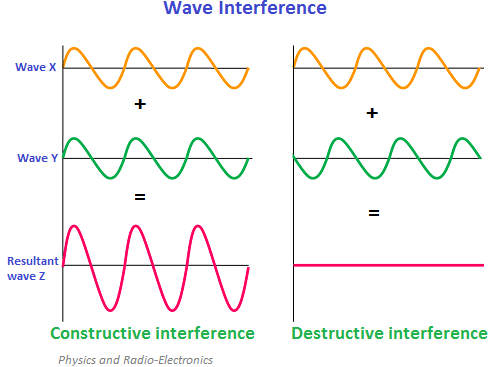
constructive interference

constructive interference is when the waves are in phase so the amplitudes add together to produce a stronger signal

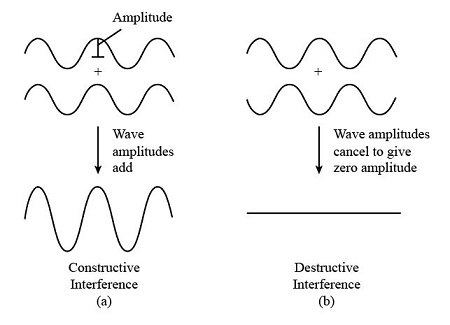
destructive interference

destructive interference is when the waves are out of phase so the amplitudes cancel each other out

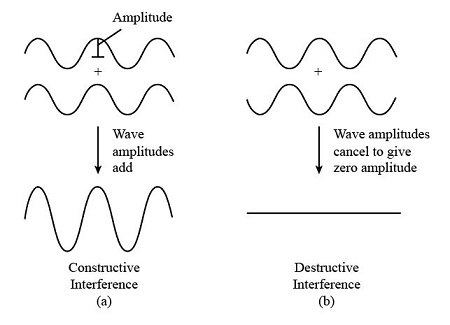
conditions for interference

• only the amplitude can change if the waves have the same frequency, speed and wave length

• the waves only cancel out completely is the amplitudes are the same

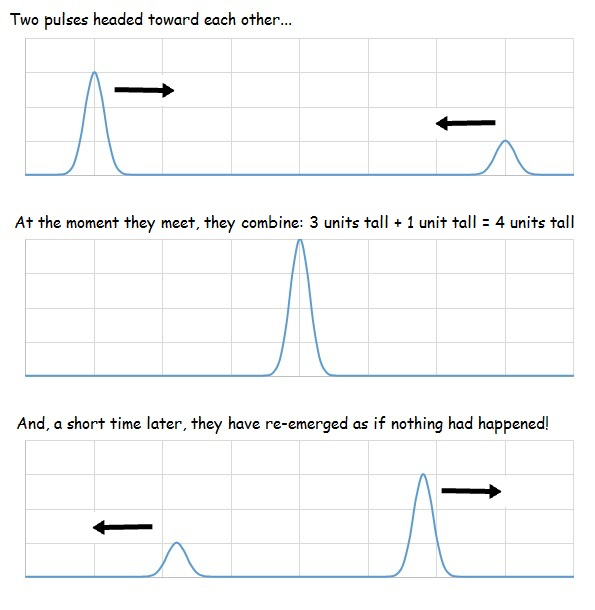
• when two pulses meet they instantaneously become one and then continue as before having crossed over, this behaviour is different to particle collisions as the pulses do not join or rebound

intensity

• intensity — the rate of energy transmitted (power) per unit area at right angles to the wave velocity

  • is proportional to the amplitude squared so doubling the amplitude will quadruple the intensity

  • measured in watts per square metre (Wm^-2)

• energy is also proportional to the amplitude squared

polarisation of light

• a polarised lens contains many long chains of molecules

• after going through a polariser the amplitude of oscillations is diminished

critical angle

the smallest angle at which there is total internal reflection, the refractive angle is 90º

optical density

• high to low optical density = away from the normal

• low to high optical density = towards the normal

• optical density has nothing to do with actual density, instead it is related to the refractive index

• the refractive index of water is about 1.33

• hot air and cold air have different refractive indexes

sine of a critical engle

the sine of the critical angle is n2/n1 so long as n2<n1 since the sine of any angle must be between -1 and 1

condition for total internal reflection

if the incident angle is greater than the critical angle there is total internal reflection such that the light goes like a mirror on the normal

how pulses collide

• principle of superposition applies

• when they overlap they add together

• once they pass through each other they continue as if nothing happened

wave interference

• waves of different frequencies can be added

• any waves that are the same type (eg. both sound) can be added

phase of waves

• sine waves can be shown using a reference circle so can be referred to as an angle, this is the phase

completely out of phase (anti-phase)

• the phase difference between the crests and valleys is pi which is referred to as completely out of phase

• if waves that are completely out of phase meet the resultant displacement is zero

• on a circle this is two points that are opposite each other showing the diameter of the circle

• a phase difference of pi is also the distance of wave length/2

path difference leading to interference

• a whole number path difference results in a phase difference of 0º so constructive interference occurs

• if the path difference is half a wave length away from being a whole number (eg. 121/2(λ)) the phase difference is pi so destructive interference occurs

coherent waves

• coherent waves have a constant phase difference which can be anything so long as its constant

• coherent waves also have the same frequency however not all waves with the same frequency will be coherent

interference with coherent waves

• two coherent sources of light will produce interference

• when the path difference is an integer number of wave lengths (whole number) the interference will be constructive

destructive interference of coherent waves

• a path difference of half a wave length will create maximum destructive interference

Huygen’s principle

• Huygen’s principle — every point in a wave front is a point source of the wave

• point source — a place in space which is an origin of waves

wave length definition

• the distance between the wavefronts is the wavelength

• the wavefronts are put at the peaks of the wave

• the troughs are half way between the wavefronts assuming the waves are in SHM (are sinusoidal)

what is the energy in a wave proportional to?

the energy in a wave is proportional to the square of the wave’s amplitude

is the rate of energy transfer by waves constant?

• straight waves in a uniform medium do not change wavelength or speed or direction or shape so the rate of energy transfer is also constant

• for circular waves the amount of energy is constant along the wavefronts however it is distributed evenly so for the circumference of the wave front there is less energy the further from the point source it is measured, this is why amplitude decreases with distance for a circular wave

which waves can be polarised?

transverse waves

how is light polarised?

• by going through a polariser

  • with an analyser perpendicular to the polariser no light is observed at the end

  • light must pass through thin molecular chains

• reflecting light from a smooth surface

  • all light reflected from a smooth non-metallic surface is partially polarised

  • at a particular angle of incident (Brewster’s angle) on smooth insulators the light will be fully polarised

  • this is how polarising sunglasses work

Malus’ law

the relationship between intensity and the incident angle is not linear — the intensity of the unpolarised light entering the polariser must be twice the intensity of the polarised light leaving the polariser

small angle approximation (in radians!)

• sinθ=0

• cosθ=1

• tanθ=0

standing wave definition

A standing wave (or stationary wave) is a wave in a medium in which each point on the wave axis has an associated constant amplitude – all points move coherently – and there is no transport of energy. 

nodes on standing waves

The locations of zero amplitude are called nodes, and the locations of maximum amplitude are called anti-nodes. Adjacent nodes are separated by λ/2, where λ is the wavelength.

resonance

the situation in which an external driver oscillating at a system's natural frequency transfers energy into the system, which in this case is the standing wave.

electric charge (q)

• current (I) x time (t)

• measured in Coulombs (C)

• 1 Coulomb is the amount of charge carried by 1 Ampere in 1 second (derived unit)

current

• measured in amperes, fundamental unit

potential difference (V)

• work per unit charge, V=W/q

• measured in Volts (V)

• 1 Volt is 1 Joule of work done on or by 1 Coulomb of charge

Coulomb’s law

• The forces on two charges are equal in magnitude and opposite in direction

• F ∝q1q2 → also F∝q1 and F∝q2

• F∝r^-2 → F∝q1q2/r²

• constant → F=k q1q2/r²

• k = 1/4πε0 = permittivity of free space

the elementary charge (e)

• every electron and proton has a charge of the same magnitude → 1.6×10^-19 C

• positive for protons, negative for electrons

drawing electric field lines

• lines don’t cross

• begin on positive charges, end on negative charges

• direction of the field is the direction of the force on a positive charge

• more lines = stronger field

• perpendicular to the surface of conductors

• no field inside a conductor

uniform electric field

• same strength and direction throughout the field

• parallel plates and even distance between field lines

• electric fields = vectors

electric field strength

• the force per unit charge exerted on a stationary positive charge at a point

• E=Fe/q so measured in NC^-1

volts

• work done per unit charge

• JC^-1

show that 1 NC^-1 = 1 Vm^-1

N/C = kg m /C s² (as F=ma)
1 Volt = 1 J/C (as V=W/q)
1N/C = 1V/m = kg m /C s²

behaviour of a charge in a uniform electric field

mathematically same equations for Fg and Fe so charge is also subject to projectile motion following a parabolic path