Physics I: Final Review

A review of conceptual questions from Physics I Lecture. It includes pre-lecture questions, PowerPoint questions, and questions from the book.

Kinematics (1D)

Which of these is not considered a variable in kinematic equations?

k

Kinematics (1D)

What are the units for acceleration?

meters per second squared (m/s²)

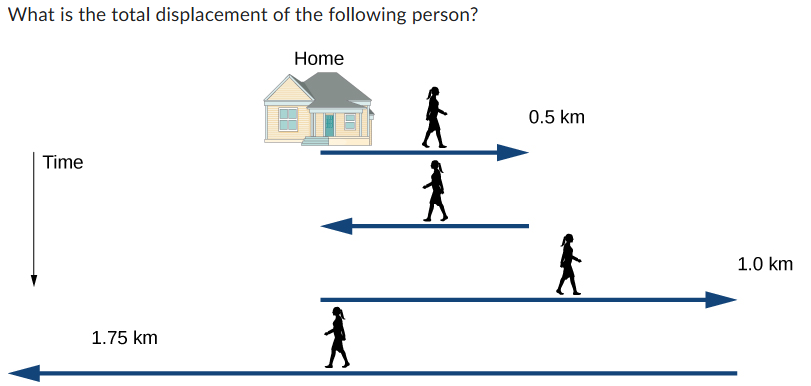
Kinematics (1D)

0.75 km

Kinematics (1D)

1 and 3

Kinematics (2D)

At the peak of a projectile trajectory, the acceleration is…

pointed downwards

Kinematics (2D)

Which variable is critical to determine in 2D motion (to link the x and y directions)?

time

Kinematics (2D)

What is the path of 2D motion?

Parabolic path

Kinematics (2D)

Velocity in the x-direction during projectile motion…

is constant

Kinematics (2D)

A ball is thrown at 9.2 m/s at an angle of 35 degrees above the horizontal. What is its final x velocity?

7.54 m/s

Newton (1st and 2nd Law)

Which of the forces listed below is considered a fundamental force?

gravity

Newton (1st and 2nd Law)

What is the unit of force?

Newton (N)

Newton (1st and 2nd Laws)

What is Newton’s first law?

Inertia

Newton (1st and 2nd Law)

If a 2 kg object is provided with 4 N of force, how much acceleration would it experience?

2 m/s²

Newton (1st and 2nd Law)

What is Newton’s 2nd law?

F = ma

Newton (3rd Law)

Which feels a greater force during a crash? A car or a truck?

Neither Vehicle

Newton (3rd Law)

Bob, from account temps, is working in a factory. He is attempting to lift a 460 N palette of boxes that are resting on the ground by using a rope attached to a pulley. Bob pulls downward on the rope with 320 N of force. How much force is now exerted by the ground on the palette?

140 N

Newton (2D)

In which direction does centripetal force point?

towards the center of the path

Newton (2D)

How do physicists refer to centrifugal force?

fictitious force

Newton (2D)

If you were spinning a ball on a string over your head and the string were to break, which path would the ball take?

continue in a straight line in the direction it was traveling at the moment of the break

Conservation Laws (Work)

Consider the 'whirly gig device' from class (the ball on the string moving in a circle). The work done on the ball by tension is…

zero, as tension is always perpendicular to the motion

Conservation Laws (Work)

A box (10 kg) is dragged across a floor 5 m by a rope that has a tension of 50 N. If the rope makes an angle with the floor, at which of these angles to the horizontal would provide the most work?

0 degrees

Conservation Laws (Work)

What are the units of work?

Joules (J)

Conservation Laws (Momentum)

What do we call a change in momentum?

Impulse

Conservation Laws (Momentum)

Object A has a mass that is 2x that of object B but both have the same momentum. Which object has the larger velocity?

Object B

Conservation Laws (Momentum)

Which of these types of collisions conserve momentum?

All of them

Conservation Laws (Momentum)

What are the units of momentum?

Kilogram meter per second (kg·m/s)

Rotation

What direction does torque have relative to the force applied and the lever arm?

perpendicular

Rotation

What are the units of Torque?

Newton meter (Nm)

Rotation

What is Newton’s 2nd law of rotating objects?

T = Iα

Rotation

In the image below, the girl in the pink shirt and the boy in the grey striped shirt are two different distances from the center. Which one experiences the greater angular speed during rotation?

neither

Gravity

How many laws did Kepler derive from the data given to him?

3

Gravity

What relationship did Kepler discover in his 3rd Law?

p² = a³

Gravity

What shape did Kepler assign to all planetary motion?

Ellipses

Gravity

What is ‘a’ in Kepler’s equation (1st Law)?

semi-major axis

Gravity

Who made the observations that Kepler used to make his laws?

Tycho Brahe

SHM

At what point does a block oscillating on a spring have maximum kinetic energy?

At the equilibrium position

SHM

What do we call the maximum displacement for an object experiencing SHM?

Amplitude

SHM

Typically we consider a SHM without friction. If we include friction and allow the object to slow down, we refer to this as a…

damped harmonic oscillator

The Scope and Scale of Physics

What is Physics?

Physics is the science concerned with describing the interactions of energy, matter, space, and time to uncover the fundamental mechanisms that underlie every phenomenon.

The Scope and Scale of Physics

If two different theories describe experimental observations equally well, can one be said to be more valid than the other (assuming both use accepted rules of logic)?

No, neither of these two theories is more valid than the other. Experimentation is the ultimate decider. If experimental evidence does not suggest one theory over the other, then both are equally valid. A given physicist might prefer one theory over another on the grounds that one seems more simple, more natural, or more beautiful than the other, but that physicist would quickly acknowledge that he or she cannot say the other theory is invalid. Rather, he or she would be honest about the fact that more experimental evidence is needed to determine which theory is a better description of nature.

The Scope and Scale of Physics

Certain criteria must be satisfied if a measurement or observation is to be believed. Will the criteria necessarily be as strict for an expected result as for an unexpected result?

Probably not. As the saying goes, “Extraordinary claims require extraordinary evidence.” In general, expected results may rely on less rigorous criteria since they align with established theories, while unexpected results may demand more stringent examination to validate them.

Units and Standards

Identify some advantages of metric units.

Conversions between units require factors of 10 only, which simplifies calculations. Also, the same basic units can be scaled up or down using metric prefixes to sizes appropriate for the problem at hand.

Units and Standards

What is the difference between a base unit and a derived unit? (b) What is the difference between a base quantity and a derived quantity? (c) What is the difference between a base quantity and a base unit?

a. Base units are defined by a particular process of measuring a base quantity whereas derived units are defined as algebraic combinations of base units. b. A base quantity is chosen by convention and practical considerations. Derived quantities are expressed as algebraic combinations of base quantities. c. A base unit is a standard for expressing the measurement of a base quantity within a particular system of units. So, a measurement of a base quantity could be expressed in terms of a base unit in any system of units using the same base quantities. For example, length is a base quantity in both SI and the English system, but the meter is a base unit in the SI system only.