Library of Functions

f(x)=[x]

f(x)=[x]

Greatest Integer

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y∈Z}

Range (Int.): N/A

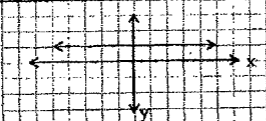
f(x)=c

Constant

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y=c}

Range (Int.): N/A

f(x)=x

Identity

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y∈R}

Range (Int.): (-∞,∞)

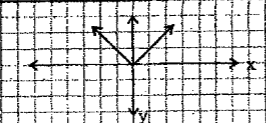
f(x)=|x|

Absolute Value

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y≥0}

Range (Int.): [0,∞)

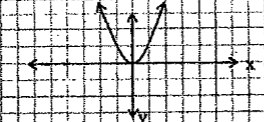
f(x)=x²

Quadratic

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y≥0}

Range (Int.): [0,∞)

f(x)=√x

Square Root

Domain (Set): {x|x≥0}

Domain (Int.): [0,∞)

Range (Set): {y|y≥0}

Range (Int.): [0,∞)

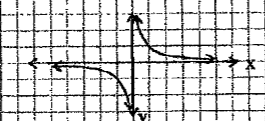
f(x)=1/x

Reciprocal

Domain (Set): {x|x∈R} and x≠0 or {x|x≠0}

Domain (Int.): (−∞,0) ∪ (0,∞)

Range (Set): {y|y∈R} and y≠0 or {y|y≠0}

Range (Int.): (−∞,0) ∪ (0,∞)

f(x)=x³

Cubic

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y∈R}

Range (Int.): (-∞,∞)

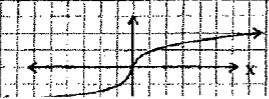
f(x)=√x (cube root)

Cube Root

Domain (Set): {x|x∈R}

Domain (Int.): (-∞,∞)

Range (Set): {y|y∈R}

Range (Int.): (-∞,∞)