CH165

what does thermodynamics tell us/not tell us

tells us about heat, work and temperature and their relation to energy, entropy and the physical properties of matter and radiation

doesn’t tell us about timescale or speed of processes or what the pathways are

what variables characterise an equilibrium

• temperature

• pressure

• volume

• amount of materials (moles)

what is an open system

can exchange matter and energy/heat

what is a closed system

can’t exchange matter but can exchange energy/heat

what is an isolated system

can’t exchange matter or energy/heat

what are extensive properties and give examples

a property that changes if a different amount of substance is examined

• mass

• volume

• energy

• entropy

  • heat capacity

what are intensive properties and give examples

property is independent of the amount of substance

• density

• temperature

• specific heat capacity (per kg)

• molar heat capacity (per mole)

equation for pressure

p = F/A

• p = pressure

• F = force

• A = area

what are the 4 units of pressure and their conversions

Pa

• Pa = Nm^-2

atm

• 1 atm = 101,325 Pa

• depends on weather/altitude

bar

• 1 bar = 100,000 Pa (x10⁵)

• 1 bar = pressure in standard state

Torr

• Torr = mmHg

• 1 Torr = 1/760 atm

conversion between ^oC and K

0 ^oC = 273.15 K

what is the state equation for temperature, volume, moles and pressure

pV = nRT

how are p & V related when T & n are fixed

p ∝ 1/V

how are T & V related when p & n are fixed

T ∝ V

how are p & T related when V & n are fixed

p ∝ T

how are n & V related when T & p are fixed

n ∝ V

equation for Boltzmann constant k_B

k_B = R / N_A

• k_B = Boltzmann constant

• R = 8.314 JK^-1mol^-1

• N_A = Avogadro number

describe ideal gas and when it is a good model

ideal gases:

• all collisions are perfectly elastic

• no interaction between particles

• point like particles

good model when gas is low pressure and high temperature

equation; pV/nRT = 1

how do real gases differ from ideal gases

• attraction between particles, making compression easier

• particles repel each other when pushed too close together

• particles have finite volume

• at low temp, gases condensate and form liquid

• equation becomes pV/nRT = Z

  • Z = compression factor

what does the compression factor suggest

• when Z ≈ 1, closer to an ideal gas

• when Z < 1, particles attract each other

• when Z > 1, particles repel each other

what is kinetic theory

a classical model describing the microscopic behaviour of gas particles and explaining macroscopic properties

• point like particles

• random movement

• no interactions between particles except perfectly elastic collisions

kinetic energy equation

E_k = ½ mv²

equation showing pressure exerted by particles colliding to the wall of a container

pV = 2/3NE_k = 2/3N(1/2mv²)

• N = number of particles

equation derived from ideal gas law to connect microscopic properties to temperature

E_k = 3/2k_BT

what can you calculate with kinetic theory

• transport properties and diffusion in gases

• frequency of particle collisions

• distance travelled between collisions

• reaction rates in gases

what happens when there is a mixture of ideal gases

• total pressure = sum of contributions from each gas

• partial pressure = pressure of that gas if it alone occupied the entire volume of the original mixture at the same temperature

• p_i = p_totalx_i

internal energy definition

the sum of all forms of microscopic energy in the system

work definition

the energy transfer from the system to the surroundings, by a mechanism through which the system can spontaneously exert macroscopic forces on its surroundings

examples of thermodynamic work

• electrical work

• gravitational work

• magnetic

what is volumetric work and what is the equation

when pushing a piston, the gas compresses inside the piston

work is done against a force to move an object by distance dx

reversible work definition

no clear direction of the process, as the piston is in equilibrium in each step and at each step, work is done against a different force

irreversible work definition

clear direction of the process, the work is done against a constant external pressure

irreversible volume change equation

reversible volume change equation

at constant T, can change the volume of a piston reversible

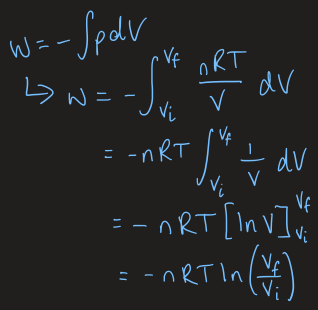
endothermic definition

heat flows into the system

• Q > 0

• temperature of system increases

• temperature of surrounding decreases

exothermic definition

heat flows out of the system

• Q < 0

• temperature of system decreases

• temperature of the surrounding increases

what is the first law of thermodynamics

the energy of a closed system is constant, unless it is changed by transfer of heat or performance of work

equations of W, Q, U when a system is isolated

internal energy cannot change

• W = 0

• Q = 0

• ΔU = 0

equations when no work can be done by a system as it is at constant volume/cannot do electrical work

any change in temperature so Q completely describes the change in internal energy

• dV = 0

• W = 0

• ΔU = Q

equations relating to internal energy when V is constant

no work can be done by a system so any change in T and Q completely describes the change in internal energy

• dU = dQ

heat capacity definition

a systems ability to absorb heat as heat

• increase in T is different for different materials

(differential) equation to show heat capacity

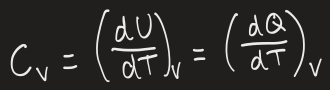
simplified equation when heat capacity is in a narrow T range so doesn’t depend on it

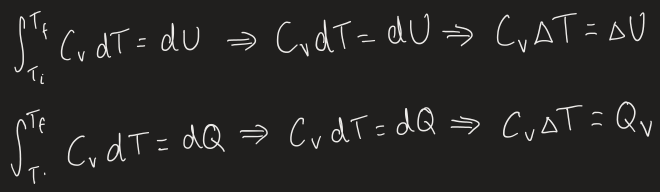
units and symbol for heat capacity

C_V

JK^-1

units and symbol for molar heat capacity

C_V,m = C_V/n

JK^-1mol^-1

units and symbol for specific heat capacity

c_V = C_V/m

JK^-1kg^-1

equation for constant volume heat capacity for ideal gases

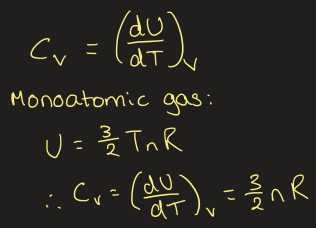
what is the molar heat capacity of the ideal gas

equation for constant volume heat capacity for diatomic ideal gas

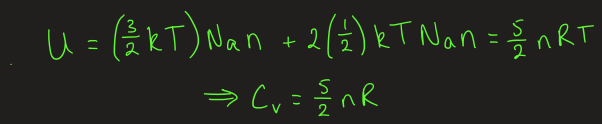
molar C_V of diatomic ideal gas

what happens when heat is applied to a substance at constant volume

heat supplied to the system will increase the internal energy of the system by the same amount

• ΔU = Q

what happens when heat is applied to a substance with constant pressure

heat supplied to the system will increase the internal energy of the system, but some of it will be used to change the volume

• ΔU < Q

enthalpy definition

a change in enthalpy is equal to the heat energy supplied to a system under constant pressure

enthalpy equations

ΔH = Q_p

→ H = U + pV

what happens if work is done to the system as it heats (and equations)

the internal energy gained will be greater than the amount of heat

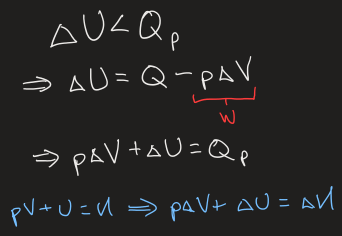
what happens when heat is released/absorbed during a process

if heat is released, ΔH_system < 0

if heat is absorbed, ΔH_system > 0

standard state definition

the most stable form of a substance at 1 bar pressure and a specified temperature

in terms of enthalpy, what is defined as the zero point

standard enthalpy of formation of pure elements in their standard states

what is Hess’s law

the standard reaction enthalpy Δ_rH is the sum of the individual reactions into which the overall reaction can be separated

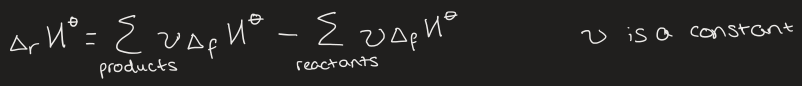
specific heat capacity defintion and equation

heat capacity for a material of specific mass

c_p = C_p/m

diagrams + equations for heat capacity as internal energy vs temperature and enthalpy vs temperature

equation for heat capacity at different temperatures

heat capacity equation if assuming heat capacity is independent of temperature

ΔH = C_pΔT

heat capacity equation if assuming it is dependent on temperature

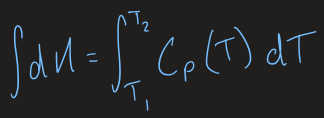
what happens in an adiabatic process and when can it be assumed to be true

no heat or mass is transferred between the system and its surroundings

true for fully isolated systems or when a process is so fast that we can assume that no heat could have been transferred in or out of the system

what is an isobaric process and what equations relate to it

a process occurring at constant pressure

• dp = 0

• W = -pΔV

• dU = dQ + dW

• c_p = ΔH/ΔT

what is an isochoric process and what are the relating equations

a process occurring at constant volume

• dV = 0

• W = 0

• dU = dQ

• c_V = ΔV / ΔT

what is an isothermal process and what are the relating equations

a process occuring at constant temperature

• dT = 0

• W = -∫p dV

• dU = dQ + dW

what are the equations relating to adiabatic processes

• dQ = 0

• W = ΔV

• dU = dW

• c = 0

equation for entropy

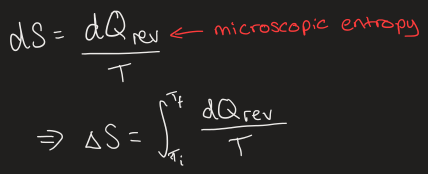
what causes entropy change

more disorder = larger entropy

• solid < liquid < gas

increased chemical complexity = larger entropy

• NaCl < MgCl2 < AlCl3

softer metal = larger entropy

• diamond < graphite < lead

equation for universal entropy

ΔS_univ = ΔS_surrounding + ΔS_system

• ΔS_univ > 0

what happens to entropy as T → 0 K

entropy change tends towards 0

• any pure substance in a perfect crystalline structure, at the absolute 0, has 0 entropy

what is Helmholtz energy equation and what is the condition

constant volume

ΔA = ΔU - TΔS

• A is Helmholtz free energy

what is Gibbs energy equation and what is the condition

constant pressure

ΔG = ΔH - TΔS

what does ΔG tell you

if ΔG < 0 then the process is favourable and will occur spontaneously

• if ΔG_B < ΔG_A then B is more favourable

what is an endergonic process

non-spontaneous process where ΔG > 0

what is an exergonic process

spontaneous process where ΔG < 0

draw a diagram to predict spontaneity using ΔS and ΔH

equations for calculating the standard Gibbs energy of the reaction

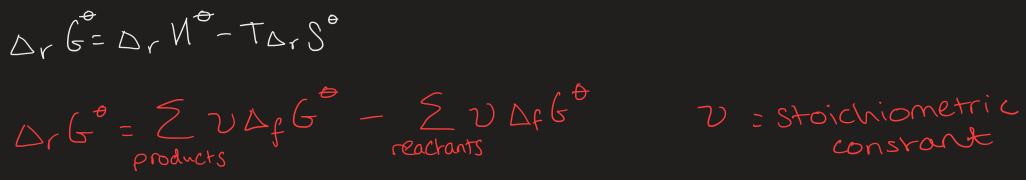
equations for calculating the standard Gibbs energy of formation

natural variables and equation for state function U

S, V

dU = TdS - pdV

natural variables and equation for state function S

U, V

not possible to form equation

natural variables and equation for state function H

S, p

dH = TdS + Vdp

natural variables and equation for state function G

p, T

dG = Vdp - SdT

natural variables and equation for state function A

V, T

dA = -pdV - SdT

what do each of these letters mean:

1. T

2. V

3. p

4. n

5. x

6. U

7. W

8. Q

9. H

10. S

11. G

12. F

13. A

14. K

15. R

16. c

17. μ

1. temperature

2. volume

3. pressure

4. amount of matter

5. mole fraction

6. internal energy

7. work

8. heat

9. enthalpy

10. entropy

11. Gibbs energy

12. force

13. Helmholtz energy

14. equilibrium constant

15. universal gas constant

16. heat capacity

17. chemical potential

phase definition

a form of matter that is microscopically uniform throughout in both chemical composition and physical state

vaporisation equations

1. enthalpy change

2. entropy change

1. Δ_vapH = H_gas - H_liquid > 0

2. Δ_vapS = S_gas - S_liquid > 0

condensation equations

1. enthalpy change

2. entropy change

1. Δ_condH = H_liquid - H_gas < 0

2. Δ_condS = S_liquid - S_gas < 0

effect of temperature at vaporisation

1. at bp

2. at low T

3. at high T

1. Δ_vapG = Δ_vapH - T_bΔ_vapS = 0

   • so Δ_vapH = T_bΔ_vapS

   • so T_b = Δ_vapH / Δ_vapS

2. G_L < G_g

   • liquid is more favourable

   • condensation occurs

3. G_L > G_g

   • gas is more favourable

   • vapourisation occurs

what is Trouton’s rule

for many liquids the entropy of vaporisation is almost the same, around 85 J/K

effect of pressure on vapourisation

higher pressure = smaller volume of gas so less freedom to move = smaller entropy do gas less favourable

what is Gibbs free energy equation for an ideal gas if pressure is changed

equation for Gibbs energy change compared to standard state

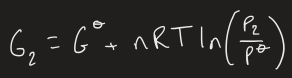
boiling curve definition

the group of p-T points, where liquid and gas are in equilibrium so they coexist

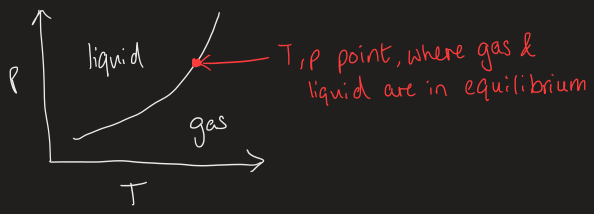
what happens to the phase when you increase pressure/temperature

as you increase pressure, the pressure will stop and remain constant as liquid forms so there is less gas

as temperature increases, the increase in heat stays constant but change in T = 0 as all heat is supplied to phase transition

equations used to find value of vapour pressure at a given temperature

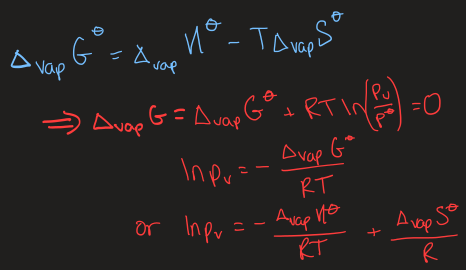
the Clausius-Clapeyron equation

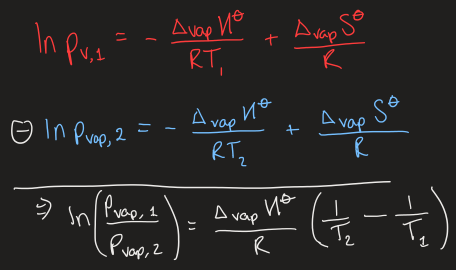
at fixed volume, how can you tell whether a substance is liquid or gas

if p < p_vap then substance is a gas

what happens when you add more gas to a fixed volume container

it will immediately condense