AP Chemistry Unit 4 Gases

Gases

Gases

Substances that fill any container, are easily compressed, and mix completely with any gas.

How to measure the barometer

The mercury stops flowing out of the tube when the pressure of the column of the mercury is equal to the pressure of the air on the mercury dish. This makes the mercury look like it is standing on the surface of mercury.

By knowing the sea level measurement of normal atm read at 760 mmHg on the tube’s increments, one can find the atm by dividing the recorded measurement of the mercury column’s height by 760.

Atmospheric Pressure using a barometer

The pressure exerted by the weight of air, measured in mmHg or torr, with 1 atm = 760 mmHg.

Manometer

An instrument used to measure pressure by comparing the height of mercury levels.

Boyle’s Law

The principle stating that P1V1 = P2V2, showing the inverse relationship between pressure and volume at low pressures.

Ideal Gas

A theoretical gas that perfectly follows Boyle’s Law under all conditions.

Charles’s Law

The law stating that the volume of a gas is directly proportional to its temperature, represented by V1/T1 = V2/T2.

Absolute Zero

The theoretical temperature (0 K) at which a gas would have zero volume, which cannot be reached.

Avogadro’s Law

The principle that states V1/N1 = V2/N2, indicating that volume is directly proportional to the number of moles of gas at constant temperature and pressure.

Ideal Gas Law

The equation PV = nRT that describes the behavior of an ideal gas under various conditions.

R Constant

The ideal gas constant in the equation PV = nRT, valued at 0.0821 L*atm/K*mol.

Molar Volume

The volume occupied by one mole of an ideal gas at STP, which is 22.42 L.

STP

Standard Temperature and Pressure, defined as 0 degrees Celsius and 1 atm.

Stoichiometry

A method used to calculate the amounts of reactants and products in a chemical reaction, which can involve molar volume at STP.

Dalton’s Law of Partial Pressures

The principle that the total pressure of a gas mixture equals the sum of the partial pressures of its components.

Mole Fraction

The ratio of the number of moles of a component to the total number of moles in a mixture.

Vapor Pressure

The pressure exerted by water vapor when it is in equilibrium with its liquid form.

Kinetic Molecular Theory (KMT)

A theory explaining the behavior of ideal gases based on four postulates regarding particle motion and interactions.

KMT Postulates

1) Negligible volume of particles, 2) Constant motion of gas particles, 3) No attraction or repulsion between particles, 4) Average kinetic energy proportional to temperature.

Real Gas Behavior

The deviation from ideal gas behavior due to finite particle volume and intermolecular forces.

KMT and Boyle’s Law

Explains that decreasing volume increases pressure due to more frequent particle collisions.

KMT and Pressure/Temperature

States that increasing temperature increases particle speed, leading to higher pressure.

KMT and Charles’s Law

Indicates that to maintain constant pressure, volume must increase with temperature.

KMT and Avogadro’s Law

Suggests that increasing the number of gas particles at constant temperature and volume raises pressure.

If most of the mercury is close to the closed end of a manometer, how would you find the gas pressure when the atmospheric pressure is given?

Gas pressure = atmospheric pressure -h

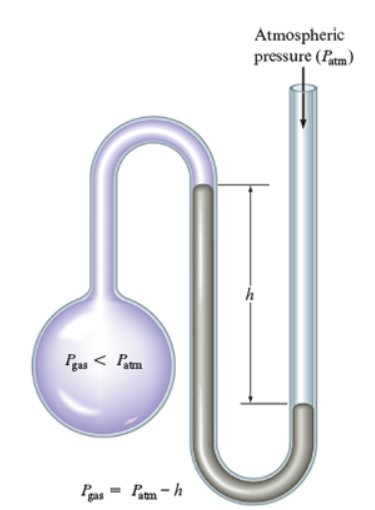
If most of the mercury is close to the open end of a manometer, how would you find the gas pressure when the atmospheric pressure is given?

Gas pressure = atmospheric pressure + h

The graph of Boyle’s Law gives a hyperbola/not linear function because

as the pressure decreases from the y-axis, the volume increases in the x-axis.

Charles’s Law graph shows that the volume of a gas is directly proportional to? Creates what type of graph?

Charles’s Law graph shows that the volume of a gas is directly proportional to the temperature, extrapolates to zero at 0 K, and creates a linear graph with temperature directly affecting the volume. (Temp x-axis, y-axis is volume)

Charles’s Law is?

The equation is V₁/T₁=V₂/T₂

Boyle’s Law is

P₁V₁=P₂V₂.

Kelvin is used instead of Celsius because? Solved by?

there can’t be a negative volume. Add 273 to celsisus.

Avogadro’s Law is represented by

V₁/N₁=V₂/N₂

V₁/N₁=V₂/N₂ states that a gas at constant temperature and pressure,

the volume is directly proportional to the number of moles of gas.

Combined Gas law/ideal gas law is?

PV = nRT

The R in the ideal gas law equation is a constant with a value of

0.0821 L*atm/K*mol.

The ideal gas law is an empirical equation or an equation that finds the

hypothetical value. no real gas is ideal.

The molar volume of an ideal gas (0 degrees Celcius and 1 atm) is

22.42 L/mol

One can use the molar volume for gas stoichiometry. This process can be listed as followed:

1. Balance the equation

2. Find the mols of the reagents that need to be included in the stoichiometry problem.

3. Find the limiting reactant if there are two reactants.

4. Use the mol ratio.

5. Use the molar volume to convert the mols to volume.

It is important to note that molar volume can only be used when

the conditions of the problem are at STP.

If the problem is not at STP, molar volume conversion of 22.4 L/mol can not be used, and so

then the ideal gas law must be used to compute the volume.

Deriving density from PV=nRT?

1. n, the variable that represents te number of moles in a gas is expressed by:
- Grams of gas/molar mass = mass/molar mass = m/molar mass.
2. When substituting the calculation of the molar mass of the gas, you can find the measured density.
- P = nRT/V = (m/molar mass)RT/V = m(RT)/V(molar mass).
- m/V is equal to the density, or d.
3. Knowing m/V equals to d, it can be rewritten as
- P=dRT/molar mass
- molar mass = dRT/P

Dalton’s law of partial pressures is expressed as

P_TOTAL = P₁+P₂+…

When gas is collected over water, a vapor pressure of water occurs. This is because the water molecules escape the surface of the liquid and collect in the space above the liquid. They also return back to the liquid. When the rate of escaping the liquid equals the rate of return, the number of water molecules stay constant = vapor pressure of water. (kind of like the barometer experiment with the mercury staying still)

Due to water vapor, when solving stoichiometry problems, the water vapor pressure must be

added to the total pressure.

Kinetic Molecular Theory (KMT) explains the properties of IDEAL gas. There are four postulates of the KMT.

1. The volume of the individual particles can be assumed to be negligible because they are so small.
2. The gas particle are in constant motion, and the collisions of the walls and particles are what causes the gas to exert pressure. These collisions are elastic, meaning no kinetic energy is lost.
3. The gas particles are assumed to neither attract nor repel each other.
4. The average kinetic energy is directly proportional to the temperature.

KMT based on Boyle’s Law: It makes sense because a decrease in volume (container) would

increase the gas particles to hit each other and the walls thus increasing pressure.

KMT based on pressure and temperature: it makes sense because when the temperature increases

the speed of the particles increases. This makes the particles hit the wall and each other with greater force and frequency, leading to increase of pressure.

KMT based on Charles’s Law: Charles’s Law predicts that in constant pressure, the volume and temperature both must increase. This is true because

temperature increases pressure, so in order to have a constant pressure, the volume must increase as well to compensate.

KMT based on Avogadro’s Law: this makes sense because more gas particles at the same temperature and volume will cause

the pressure to increase.

Mole fraction equation

mol of substance/total moles * the total pressure = the partial pressure

The root mean square velocity equation is

sqrt(3RT/M)

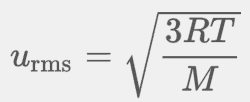
The SI unit in the root mean square equation is? This is represented bY?

A joule is a kilogram meter squared per second squared (kg*m²/s²)

In a root mean square velocity equation, R is now? M is now? New units now?

This means that in the root mean square velocity equation, the M is in kilograms, R is now 8.3145 not 0.0821, and the units is m/s

While the root mean square velocity answer “U_rms” gives the average meters per second of a gas, not all molecules

have this velocity. When a gas molecules collide, they exchange kinetic energy meaning the one hit moves faster than the one who hits.

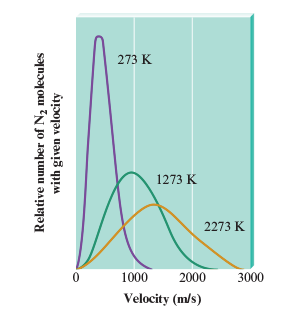
In the Boltzmann curve the peak of a curve reflects the

most probable velocity that can be found in the various particles.

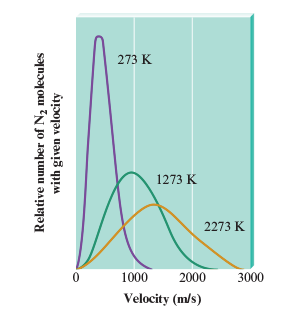
Temperature increase average kinetic enregy or average velocity?

Av. Kinetic Energy. There are places in the average velocity-temperature graph (Boltzmann) where some molecules have lower velocities compared to others at different temperatures.

Effusion

gas passes through a tiny hole into an empty chamber.

rate of effusion

the speed of which the gas transfer into said chamber.

The effusion rate for a gas depends directly on the

average velocity of its particles, which can be calculated using the root mean square velocity equation. The faster the gas particles, the more likely it will pass through the hole.

Graham’s Law states that the

ratio of the rate of effusion for gases 1 and 2 is inversely proportional to the square roots of the masses of the gas particles when it has constant temperature and pressure.

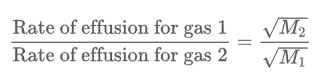
In Graham’s Law, the faster light gas is?

Gas 1

In Graham’s Law, the slower, heavier gas is?

Gas 2

Diffusion is when

gases are mixed.

rate of diffusion

rate of the gases mixing

Graham’s Law can not be used with diffusion because

so many collisions occur when gases mix, like with O2 and N2 (air), that the theoretical value is inconsistent.

Ideal Gas Conditions are?

A real gas can only exhibit behavior close to ideal behavior at low pressures and high temperatures.

The two postulates that are incorrect in the KMT postulates is

theorized gases are negilibile, or no volume, meaning it has the same volume as the container. However, real gas consists of atoms/molecules have finite volumes.

There is also intermolecular forces between gas molecules. Because of these attraction, it causes the particles to hit the wall less often as some of its time is taken to being attracted to other molecules. This leads to a lower pressure than expected in the absence of interactions.

In the van der Waals equation, what corrects what?

the (V-nb) corrects the volume to become finite, and the Pobs +a(n/V)² corrects the attraction.

A low value for a in the van der Waals equation means?

weak intermolecular force.

Because of van der Waals findings, finite volume is less important when? More important when?

the finite volume is less important when it is in a large container, which means low pressure, but if it is at a small container, the finite volume needs to be accounted for as there is high pressure.

In relation to van der Waals findings, if there is a high temperature?

the particles don’t attract as quickly.