straight line graphs

what can you do when 2 equations are intersecting?

• set them equal to each other

• substitution

when do you substitute when 2 lines are intersecting?

when the equation doesn’t have y as the subject

gradient of parallel lines are …

The same

gradient of perpendicular lines are …

negative reciprocals

what is the product of the gradients of 2 perpendicular lines?

-1

when finding the negative reciprocal of the gradient, what do you have to ensure?

that y x 1

how do you prove a quadrilateral is a rectangle?

2 pairs of parallel sides

how do you prove a quadrilateral is a trapezium?

1 pair of parallel sides

how do you prove a quadrilateral is a parallelogram?

2 parallel sides

…. what’s a trapezium …..

you should be ashamed of yourself

what’s a parallelogram …

sigh

how do you find the distance between 2 points?

pythagoras

how do you answer this? (Example 14, 5.3)

what does congruent mean?

of the same length

how do you find the point of intersection?

1. make sure y is the subject

2. set equal to each other / substitute

3. rearrange for x. this gives u the x coord at the p.o.i

4. substitute ur x value into one of the equations for the y coord

what is the shortest distance between parallel lines?

the perpendicular distance between them

how do you answer this? 5g 6d

how do you answer this 5g 7 also ask teacher abt it

how do you prove a triangle is a scalene?

the length of all 3 sides are different

what is a scalene triangle?

a triangle with each side being of a different length

how do you answer this? 5g 8 (b)

what is an equilateral triangle?

a triangle where each side is the same length

how do you prove a triangle is an equilateral?

all sides (the distance between points) are the same length

what is an isosceles triangle?

a triangle where 2 sides are the same length

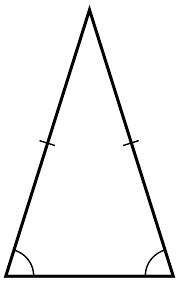
how do you show a triangle is an isosceles?

2 sides (the distance between points) are the same length

when are 2 quantities in direct proportion?

• increase at the same rate

• straight line

• through the origin

  

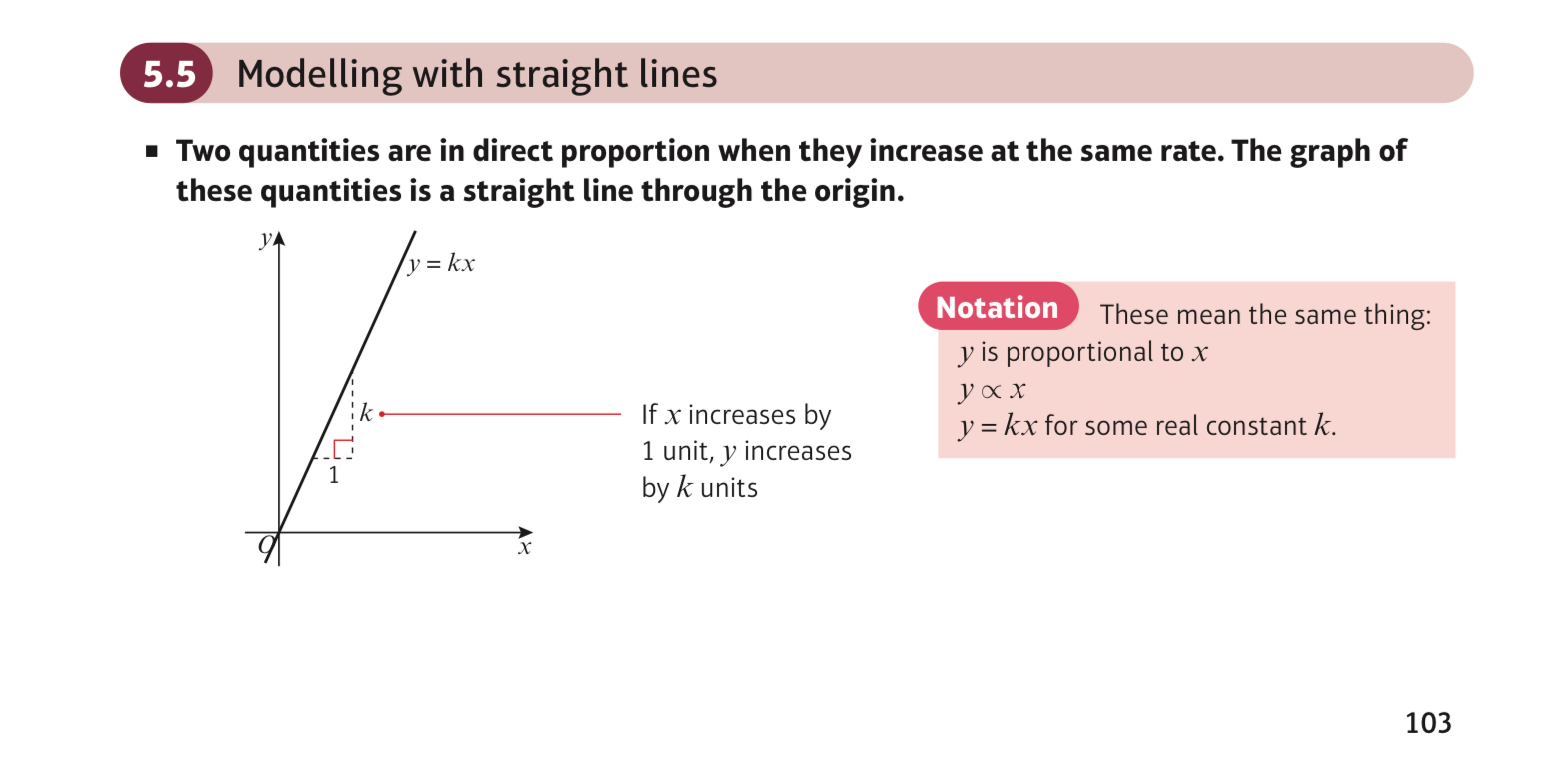
does a direct proportion graph go through the origin or not?

yes, it does

why is the y-int of a direct proportion graph 0?

because it goes through the origin

why is a direct proportion graph straight?

because they are increasing at the same rate

what is the equation of a direct proportion graph?

y = kx

why is the equation of a direct proportion graph y = kx?

• y ∝ x

• y = kx

• y-int = 0 because it goes through the origin

what does the gradient of a direct proportion mean in straight line modelling?

if x increases by 1 unit, y increases by k units

HERE

what is a linear model?

a model showing the relationship of 2 variables that aren’t directly proportional

what is the difference between a linear model and a direct proportional model?

• direct proportion model goes through the origin, y = kx

• linear model may not go through the origin, y = kx + c

what is the equation for a linear model?

y = kx + c

for a linear model, do all values need to lie on the line?

no, but they must be close to the line

sometimes a linear model is not appropriate for modelling. what determines this?

the proximity of the values to the line - the further away, the less appropriate a linear model is

what is the gradient in a linear model? (example answer)

the amount the [y variable] increases by in [units] when the [x variable] increases by 1 [unit]

when explaining a linear model, what must you remember to include?

to include the units of measurement (e.g., the amount the [variable] increases in [units])

what does it mean in linear modelling when the gradient is negative?

the y variable is decreasing when the x variable increases by 1 unit

what does it mean in linear modelling when the gradient is positive?

the y variable in units is increasing when the x variable increases by 1 unit

what is the y-intercept in linear modelling?

the initial y value

when asked to see if a linear model is appropriate, what should you include?

a conclusion statement, saying that ‘the points do / don’t form a straight line, therefore a linear model is / isn’t suitable’

when asked to find the equation for a linear model, what should you include?

what the values represent (e.g., the x variable represents the time)

what should you do throughout all of modelling?

add a conclusion explaining what everything represents

in linear modelling, what does the x value increase by in the gradient?

always 1 unit, regardless of the scale

what is a common assumption we make in linear modelling?

that the y value will increase by the same amount each 1 x variable unit

in linear modelling, how do you find the value when the y value = x value? exercise 5h 6d

algebra here

what does this mean; ‘find the equation in terms of y and x only’?

not including any constants or unknown values

how do you solve this? mixed exercise 12 b

when asked to prove if 2 lines are perpendicular, what do you do?

compare their gradients (don’t do anything about magnitude since it’s not gradient) and prove they are negative reciprocals of each other by multiplying them together to get -1

how to find;

• gradient

• distance

here

mixed exercise 17 a