Differential Equations Final Exam

Final

linear Independence

y1 and y2 are linearly independent if the Wronkskian does not equal zero.

Fundamental Set of solutions

A collection of solutions to a differential equation that are linearly independent and can be used to express the general solution. Y1 and Y2 are known.

General Solutions

Contains all possible solutions

Repeated roots and Order Reduction 3 possibilities.

2nd order, linear, homogenous ODE w/ constant coefficients.

1) Two real and distinct roots

2) one real repeated root (usually multiply by t)

3) Two complex conjugate roots. (trig)

Repeated Root Theorem

If ODE has a characteristic eqn that has a repeated root (k), then general solution y=c1e^kt+c2te^kt

Order Reduction (not constant coefficients).

v(t)= integral(1/y1²*alpha)

Complex Roots

2nd order linear, homogenous, quadradic formular yield complex roots. z=alpha +i*beta (always come in conjugate pairs).

Gen Sol: y(t)=c1e^alpha*tcos(beta*t) +c2e^alpha*t sin(beta*t)

*real valued and continuous)

Non-Homogenous Eqns

1) solve Homogenous version to find y1 and y2

2) Find particular solution (undetermined coefficients, variation of parameters).

3) Write general solution to NH problem with yp

4) Use I.C to solve for c1 and C2

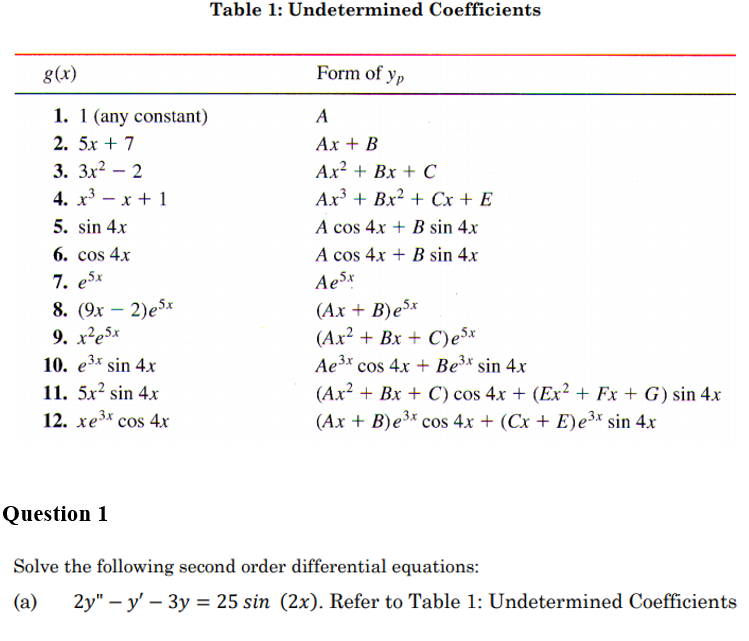
Undetermined Coefficients

Particular solution, by guessing.

ypt will be the product of all the guesses (does not wrk with quotients t^-1)

GUESS MUST BE LINEAR INDEPENDENT

If already apart of solution, multiply by t (order reduction) to ensure linear independence.

Variation of Parameters

Works for all particular solutions for all 2nd order linear non-homogenous ODES.

yp= u1y1+u2y2

u1= int(-y2*g/w)

u2=int(y1*g/w)

Mechanical systems and simple harmonic motion

2nd order linear ODE with constant Coefficients

mx”=dx’+kx=g(t)

x= horizontal displacement (dep variable)

t=time (ind.

m=mass

d= dampening factor

k= spring constant

Unforced vibrations with no dampening (simple)

mx”+kx=0

char eqn: typically complex roots, r=0±sqrt(k/m)=Beta

Yh(t)= c1cos(sqrt(k/m)*t)+c2sin(sqrt(k/m)*t))

x(t)=Acos(wo*t-theta)

A:Amplitude sqrt(c1²+c2²) (meters)

theta: phase shift tan=c2/c1 if negative, subtract or add by pi.

w0(t)=beta*t=sqrt(k/m)*t

T: period (2pi/sqrtk/m) 2pi/w0

Unforced Damped Vibrations

mx”+dx’+kx=0

Case 1: 2 distinct roots, both negative d²-rmk>0 (overdamped comes to rest over time)

case 2: Complex roots, d²-4mk<0, Underdamped, goes to rest after oscillations.

Case 3: d²-4mk=0, repeated root. Critically damped (system goes to rest the quickest)

Forces Vibrations

mx”+dx’+kx=g(t)

if d>0, Xht will have one of the forms underdamped, critically, or overdamped.

Xh(t) as limit goes to infinity will always go to zero. Any long term behavior will mi

d=0, xht is simple harmonic motion with natural frequency, as t goes to infinity will oscillate forever.

Forcing term is periodic

case 1: frequencies match! forcing term frequency matches natural frequency of system, (TRUE RENOSANCE!) oscillations will grow larger and larger in amplitude until system destroys itself

case 2: Frequencies are different, Beats! The system oscillates, but to a neat looking wave form. The more equal the frequencies, the more pronounced the beats will be.

Laplace Transform

k(s,t) kernal of tranform

F(t)=integral (e^-st*f(t))dt

Domain: what values as the limit converges.

TO be laplace must

1: f(t) is piece wise continuous (contains every point on interval [0,inf), with finite discontinuities).

2: of exponential order e^a*t, f(t)<Me^at, Find an alpha that makes this equal to zero. f(t)/e^at

Properties of Laplace

LT is a linear transformation (can have a constant multiplied in or out)

Use table of transformations

THM: LT of derivative, Ly’=sLy-y0

Transform Property: Le^alphat*f(t)=F(s-a) for s>a +alpha

Inverse Laplace Theorem

Very often need algebra for this: completing the square, partial fraction decomposition. If degree numerator> degree denom. perform polynomial long division.

THM: