Gravity, Orbits, Centripetal Force, and Kepler's Laws

A planet is 3 AU from the sun. The force is 21555 N. What is the force at 6 AU?

5388.75

What is the relationship between Distance and Force?

Inverse square

What is the relationship between Mass and Force?

Direct

If the sun suddenly disappeared, what would happen to the movement of Earth?

It would continue to go in the direction it was headed instead of staying in orbit.

If the sun’s mass was tripled what would happen to the gravitational force (Start with 10 N of force)? How would this change the Earth’s orbit?

The force would be 30 N. The Earth would move closer to the sun and it would move faster.

If the distance between the sun and the Earth was tripled what would happen to the gravitational force? The starting force is 10 N.  How would this change the Earth’s orbit?

The force would be 1.11 N. The Earth would move slower and it would be farther away.

Planet A lies at 4 AU (Astronomical Units) from the Sun. Planet B lies at 12 AU from the Sun.  How much less gravitational force does Planet B receive than Planet A? (Distance vs. Force)

1/9 less

What is Kepler’s first law? What does it show?

The Law of Ellipsis. It shows that all planets orbit in the shape of an ellipsis.

What is Kepler’s Second law?  How does speed change from the perihelion to the aphelion?

Planets travel equal areas over equal time. They move faster at perihelion and slower at aphelion.

What if there were a planet that went around the sun 5 times as fast as that of earth (Earth’s orbital period is 1 year). Compute the orbital size as compared to earth. Use the following equation: p² = a³ 

0.342 AU

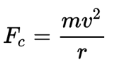
The force of friction between a 4,000 kg car and the road is 15,000 N. If you are driving in a car on a circular racetrack with a radius of 3,000m. Use the above equation to solve for v. What is the fastest speed at which you could drive around the track without skidding out?

106.1 m/s

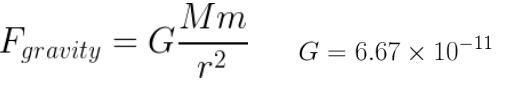
A planet with a mass of 8 x 10²⁸ kg orbits a sun with a mass of 4 x 10³⁵ kg at a distance of 4.2 x 10¹⁴ m. What is the velocity of this planet?

112.25 m/s