Geometry 1
5.0(1)
Card Sorting
1/31
There's no tags or description
Looks like no tags are added yet.
Study Analytics
Name | Mastery | Learn | Test | Matching | Spaced |
|---|
No study sessions yet.
32 Terms
1
New cards
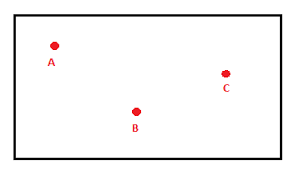
A location with no size or shape
Point
2
New cards
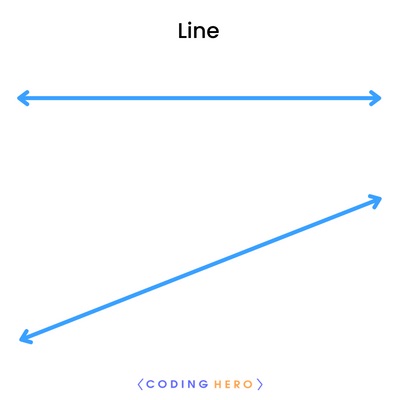
Made up of points with no thickness or width
Line
3
New cards
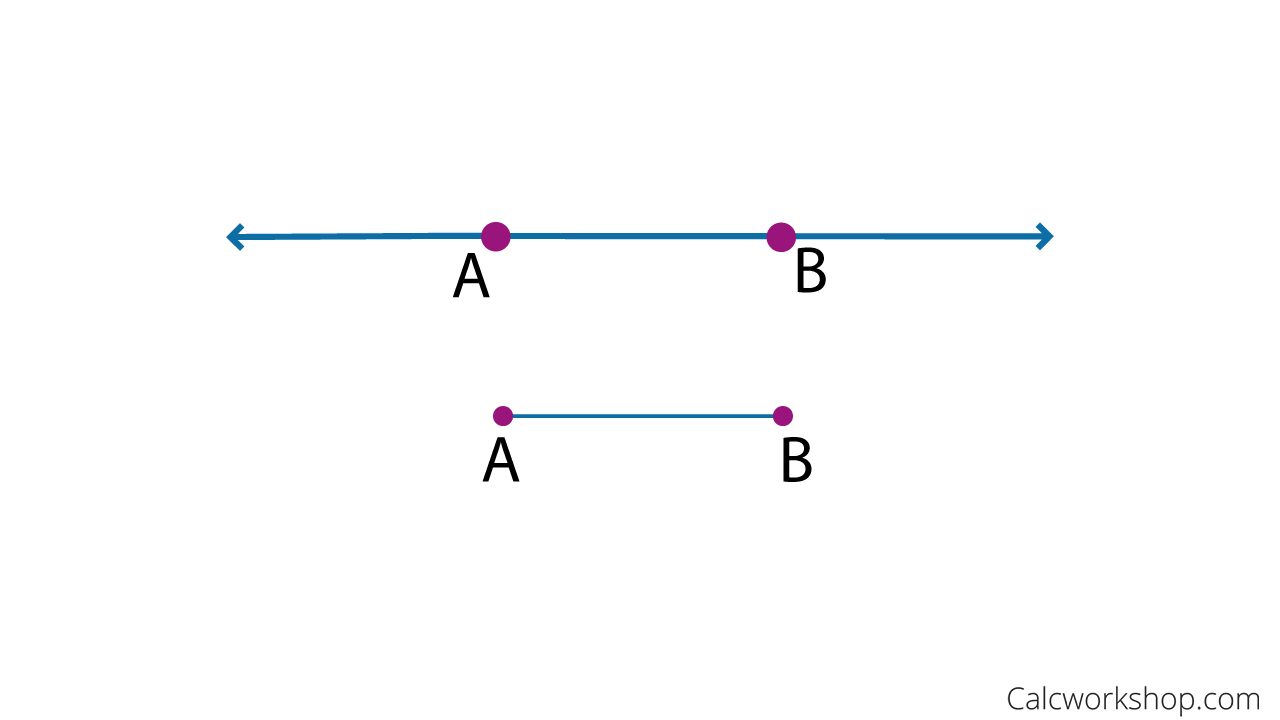
A measurable part of a line consisting of two endpoints
Line segment
4
New cards
\
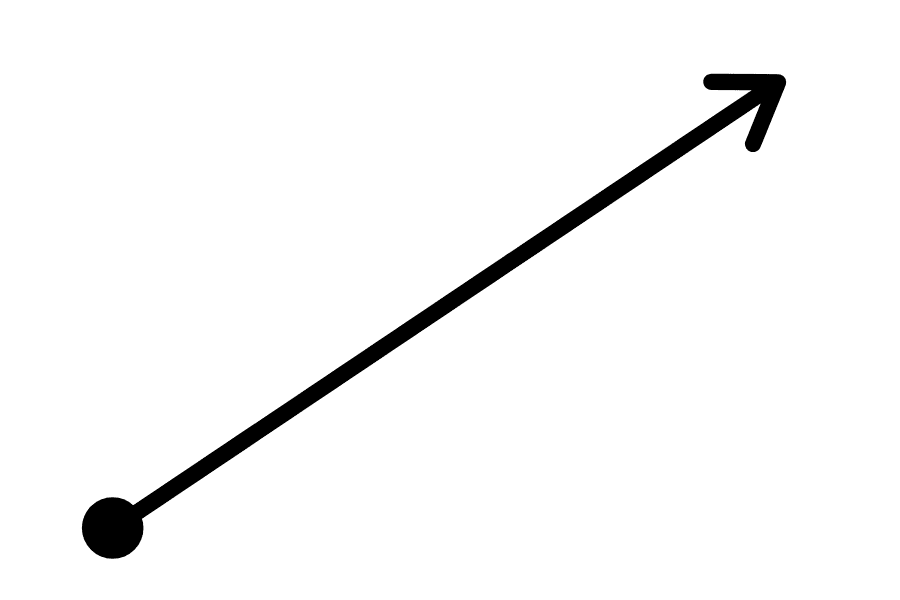
**A line** that extends indefinitely in one direction
**A line** that extends indefinitely in one direction
Ray
5
New cards
A **flat surface** made up of points and extends indefinitely in a direction
Plane

6
New cards
Points that lie on the same line
* Non-Collinear:points that don’t lie on the same line
* Non-Collinear:points that don’t lie on the same line
Collinear

7
New cards
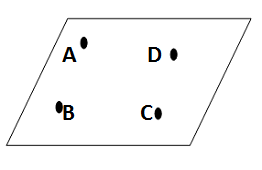
Points that lie on the same plane
* Non-Coplanar:points that don’t lie on the same plane
* Non-Coplanar:points that don’t lie on the same plane
Coplanar
8
New cards
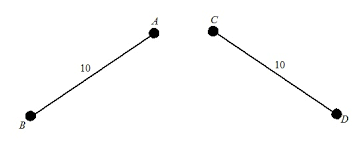
If two segments have the same length, then they are congruent
Congruent segments
9
New cards
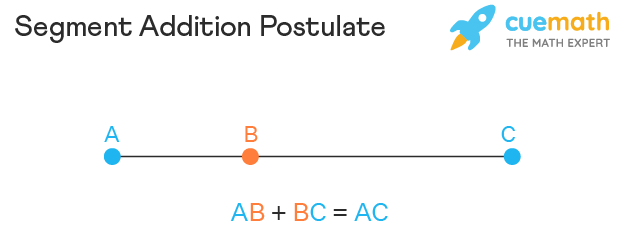
If A,B and C are collinear points and B is between A and C then AB+BC=AC
Segment addition postulate
10
New cards
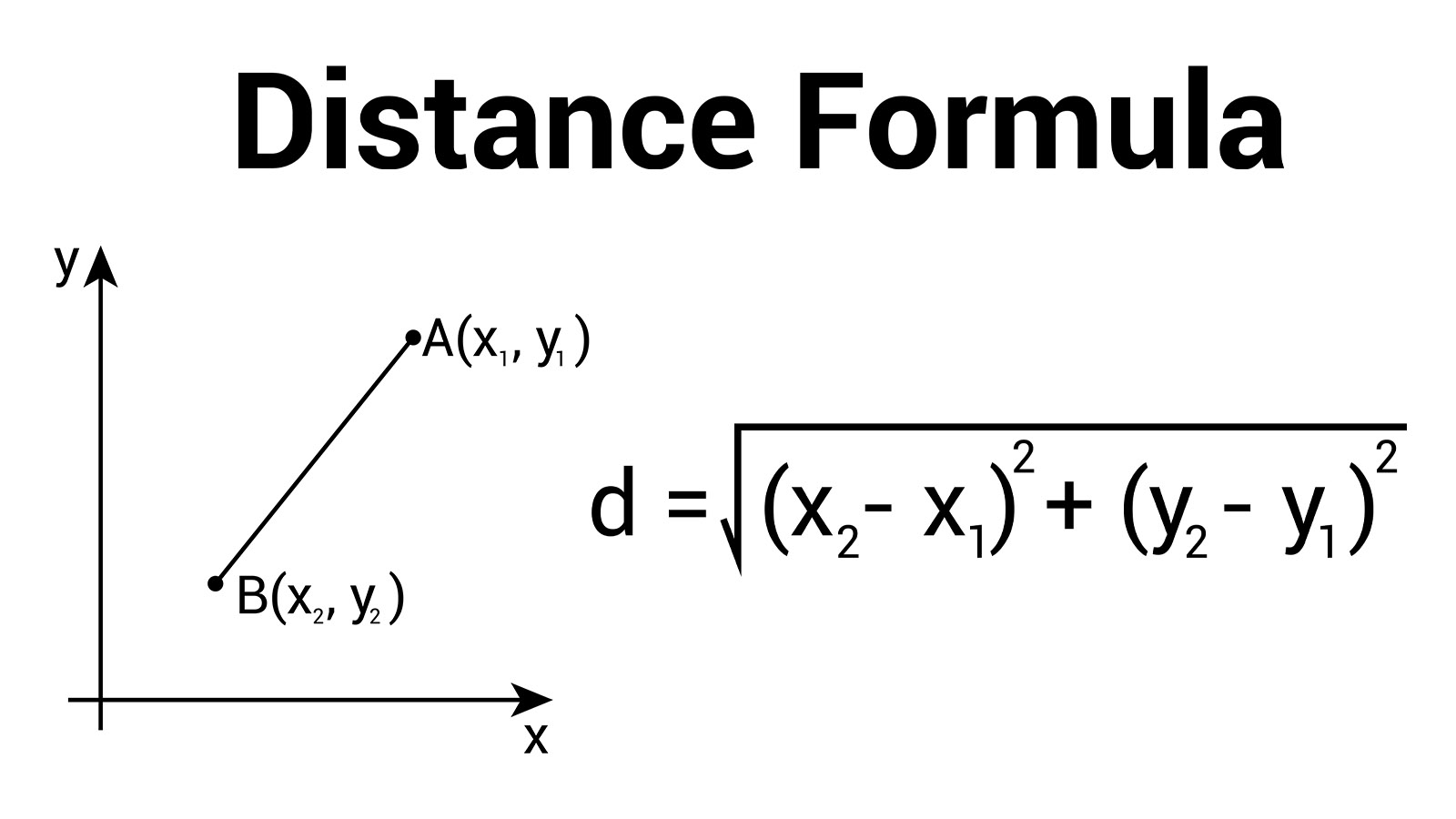
The formula used to find the distance between two points on a coordinate plane
Distance formula
11
New cards
The formula used to find the midpoint between two endpoints
Midpoint formula

12
New cards
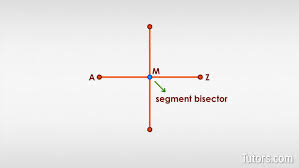
A segment, line, plane that can intersect a segment at its midpoint
Segment bisector
13
New cards
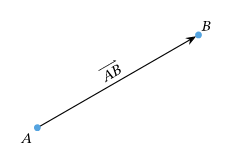
A line segment with associated direction
Directed line segment
14
New cards
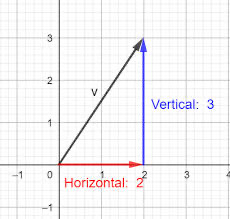
The “run” of a directed line segment
Horizontal component
15
New cards
The “rise” of a directed line segment
Vertical component
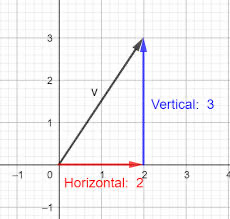
16
New cards
Two lines that intersect at a 90° angle (right angle)
Perpendicular
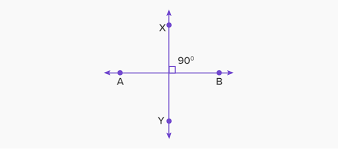
17
New cards
A line, segment, or ray perpendicular to a segment at its midpoint
Perpendicular bisector
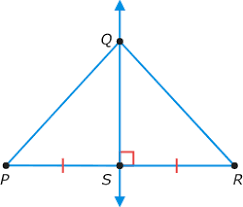
18
New cards
Two lines that never intersect
Parallel lines
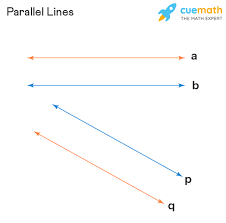
19
New cards
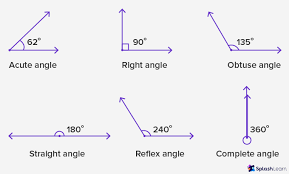
The intersection of two right at an endpoint
Angle
20
New cards
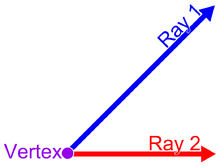
The common endpoint of an angle (where two rays/sides, intersect)
Vertex
21
New cards
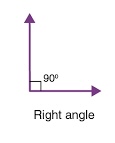
An angle with a degree measure of 90°
Right angle
22
New cards
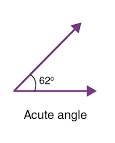
An angle with a degree measure less than 90°
Acute angle
23
New cards
An angle with a degree measure greater than 90°
Obtuse angle

24
New cards
An angle with a degree measure of 180°
Straight angle

25
New cards
Two angles that have the same measure are congruent
Congruent angles

26
New cards
Two angles that share a common side and vertex “next to” each other
Adjacent angles

27
New cards
Angle addition postulate

28
New cards
A line or ray that divides an angle into two equal parts
Angle bisector
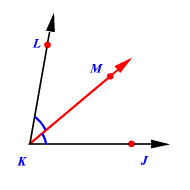
29
New cards
Two angles directly across from each other on intersecting
Vertical angles
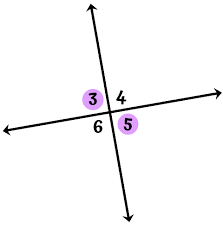
30
New cards
Two angles with measures that sum to 90°
* **DO NOT** have to be adjacent
* **DO NOT** have to be adjacent
Complementary angles

31
New cards
Two angles with measures that sum to 180°
* **DO NOT** have to be adjacent
* **DO NOT** have to be adjacent
Supplementary angle

32
New cards
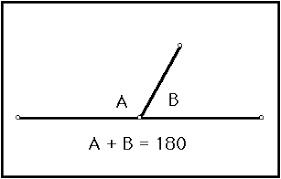
Adjacent angles that are supplementary. Combine, they form a straight line
Linear pair