Unit 2: Introduction to Chemistry

Standard notation (St. N)

Scientific notation (Sc. N)

• Scientific notation expresses very large or extremely small numbers as a coefficient raised to a power of 10.

• The exponent for 10 can be a positive number (large numbers) or a negative number (small numbers).

→ Ex: 123,456,000 is standard notation.
1.23456000 × 10⁸ is scientific notation.

Conversion factor (CF)

A fractional expression relating or connecting two different units or prefixes.

• A fraction with a value = 1

• CF are used to change from one unit to another

• Always include units in every step

Unit conversion

U = I x CF (x CF x CF… etc.)

• Identify the Unknown amount and units

• Identify the Initial amount and units

• List the CF(s) that will relate or connect the I units to the U units.

• Solve (the units of I must cancel with units of the bottom of the CF)

Multiple CF’s

Always create a plan:

• Ex: L → gal → $ or mL → L → kL

Each “→” represents a CF.

• Can be computed in separate steps or all in one step.

SI units

• The International System of Units (SI) is the modern form of the metric system.

• Devised around the convenience of 10.

• World’s most widely used system.

• Base (SI) units: a base unit of measurement, all other units are multiples of the base unit.

Metric prefixes

• mega (M) = 10⁶

• kilo (k) = 10³

• deci (d) = 10^-1

• centi (c) = 10^-2

• milli (m) = 10^-3

• micro (µ) = 10^-6

• nano (n) = 10^-9

• pico (p) = 10^-12

Converting Squared & Cubed Units

Convert 2.4 × 10⁶ cm³ in m³?

2.4 × 10⁶ cm^{3 ×} 10⁶ m³ / 1cm³ = 2.4 m

Converting Derived Units

Derived Units are made by combining 2 or more other units.

→ Ex: Velocity (speed) is a derived unit made up of distance (km) and time (h).

Density

• A derived unit consisting of mass (m) and volume (V).

• Formula: d = m / V

• The density of water is ~1.0 g/mL

• Density depends on how tightly packed your particles are.

• More tightly packed = more dense.

• 1cm³ = 1mL

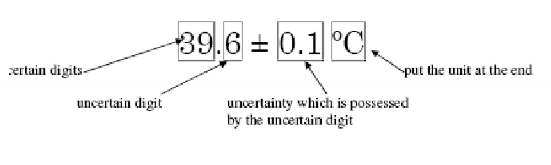
Significant figures (Sig Figs / SF)

Significant figures/digits include the digits that you are certain about.

• All non-zero digits are significant

  → Ex: 123 has 3 SFs.

• Trailing zeros with no decimal point after them are NOT considered significant.

  → Ex: 100 has 1 SF.

• Trailing zeros after a decimal point are assumed to be significant. They show the precision of the measuring device.

  → Ex: 1.20 has 3 SFs.

• Leading zeros are not significant.

  → Ex: 0.03 has 1 SF.

• All zeros between non-zero digits are significant.

  → Ex: 5002 has 4 SFs.

• Exact numbers (like conversion factors) have an infinite number of significant figures.

  → Ex: “37 people” has ∞ SF.

Calculations with Significant Figures

• Multiplying and diving: The answer should have the same number of SF as the LEAST precise measurement.

• Adding and subtracting: Line up the decimal place and round to the LEAST precise decimal place.

• REMEMBER!

  • Don’t forget BEDMAS, determine SF in each step but do not round early.

  • SF applies to measured values, not defined values (like conversion factors).

Rouding rules

• If your answer ends in a number that is greater than 5, increase the preceding digit by 1.

  → Ex: 2.346 can be rounded to 2.35.

• If your answer ends with a number that is less than 5, leave the preceding number unchanged.

  → Ex: 5.73 can be rounded to 5.7.

• If your answer ends with 5

  • Increase the preceding number by 1 if it is odd.

    → Ex: 18.35 can be rounded to 18.4.

  • Leave the preceding number unchanged it it is even.

    → Ex: 18.25 can be rounded to 18.2.

Precise vs. Accurate

• Accurate = measure that is close to the correct or accepted value.

• Precise = reproducible measurement, more significant digits / more decimal places.

• Assume the CORRECT measurement of a pencil is 27.3200 cm.

  • 27.3 is ACCURATE but not very PRECISE.

  • 28.466 is PRECISE but not very ACCURATE.

  • 27.3201 is both ACCURATE and PRECISE.

  • 35.2 is neither ACCURATE nor PRECISE.

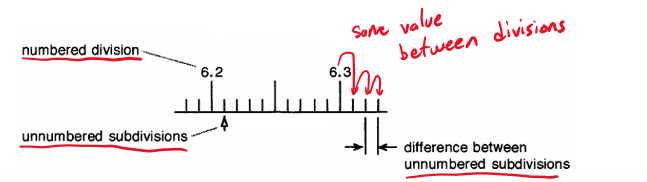
Uncertainty

• To find uncertainty value for a measurement, take the difference between the smallest increment/ division and divide by 10. The uncertainty is always in the last digit (the one that was estimated).

• This gives us a RANGE for the measurement.