Instrumental Analysis Exam 3 CHEM 3334

Peak Generation

Repetitive distribution depends on the relative phase movement

Peak Broadening

Nonequilibrium distribution depends on the relative phase movement

Peak Broadening can be induced by molecular diffusion

Can be expressed in terms of flux:

Flux (mol/m² * s) —> J=-D_M (dC/dx)

D_M= molecular diffusion coefficient

dC/dx = concentration gradient

x= distance

If diffusion exhibits normal/random distribution then:

C_(mol/m³)= (mol/sqrt(4piD_Mt)) * e^-x2/(4Dmt)

Plate Theory of Chromatography

sigma² /x = 2Dm /ux = H

Plate Theory Definitions

H is the plate height (or height equivalent to a theoretical plate) hetp

Variable N

The number of theoretical plates is also a measure of column efficiency: the greater the number, the narrower the bandwidth, the better the resolution.

Equation for number of theoretical plates:

N_t=L/H

L= is the column length

H= is the plate height

The longer the column, the greater N

The smaller the plate height, the greater N

Substituting H= sigm²/x

N_t= L * x/sigma²=L² /sigma²

Considering a certain analyte that provided a peak with a given t_r

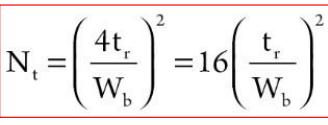
W_h (full width at the half maximum W_FWHH) can be measured more accurately than W_b

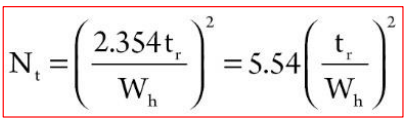
Q. How can we decide on the number of theoretical plates? How can we decide how many plates we need to get a good separation?

It is crucial to be able to estimate the resolution necessary to solve the problem, extent of separation between two adjacent solutes

Resultion

Extent of separation between two adjacent bands/peaks/solutes

Resolution Equaiton

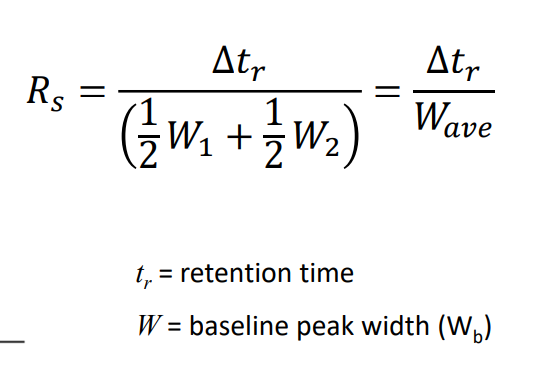
Resolution when there is only one peak

R_s = t_r/W_50%

W_50% can be the width of the peak at 50% height, the full width at half height, or the full width at half maximum (all the same damn thing)

Resolution is proportional to the square root of the number of theoretical plates!

Rate theory of chromatography

band spread is the result of cumulative random processes

• variance is additive

Processes can be divided into 2 categories

• on column (processes occur during separation)

• off-column (pre/post column) either at injection port or post detector

Rate theory of Chromatography central equation

H_T=H_E+H_D+H_S

E= eddy diffusion/multiple flow paths

D=Longitudinal (molecular) diffusion

S= equilibration between phases (mass transfer)

Eddy Diffusion

Relates to the different paths that analyte might take through the stationary phase support particles

Eddy diffusion caveats

• assumes that the column is not effected by mobile phase flow rate

• more possible paths lead to greater peak broadening

• minimized by small, uniformly packed SP particles

• However, smaller particles leads to tighter packing, so greater pressures are needed to push the mobile phase through column

Longitudinal Diffusion (thermodynamic and kinetic)

sigma²=B/u_x

B=constant f (column stationary phase, etc_

u_x= flow rate

Longitudinal Diffusion

this is a natural diffusion from a region of high concentration (center of band) to a low concentration (beyond the band). It occurs regardless of flow rate. A higher flow rate means less time in column, hence a lower band broadening

Mass transfer (kinetic)

There occurs equilibration between phases: the solute in the stationary phase lags behind the rest of the solute zone as it travels down the column.

Competition of mass transferring between phases and mass transport along with mobile phase. High flow rate causes analyte molecules in th eMP to move away from those partitioned into SP, increasing broadening.

Mass transfer (kinetic) equation

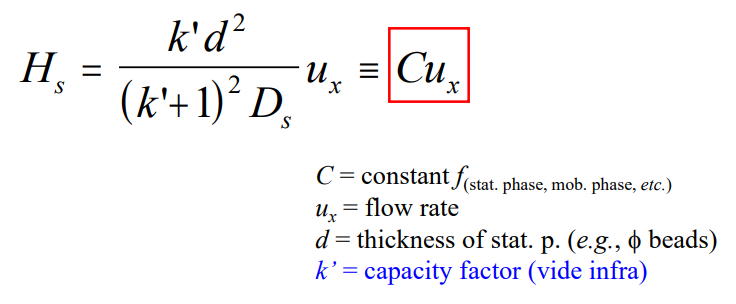
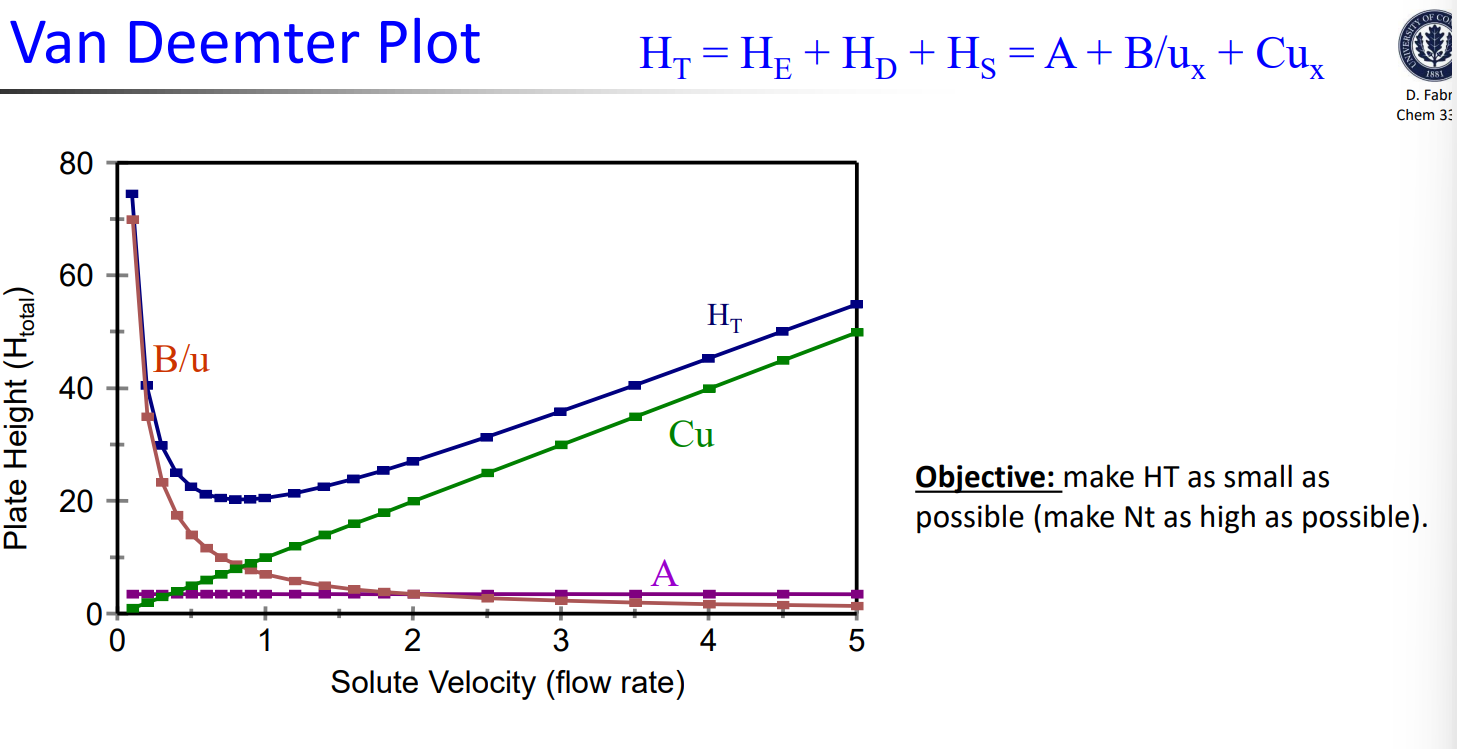
Van Deetmer Equation****

H_T=A+B/u_x+Cu_x

A=eddy diffusion

B/u_x= longitudinal (molecular) diffusion

Cu_x= equilibration between phases (mass transfer)

Van Deetmer Plot example:

Retention time

Time needed after injection for component to elute

Adjusted retention time

t_r’=t_r=t_m

t_m=void/dead volume

Relative retention

alpha=t_r’ (2)/t_r’ (1)=K₂/K₁

K1 and K2 are partition coefficients for solute 1 and 2

Partition coefficient

K=C_s/C_m

C_s is the concentration of solute in stationary phase

C_m is the concentration of solute in mobile phase

Retention (capacity) factor (k’)

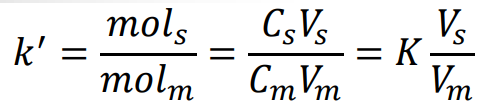
Alternative Retention (capacity) factor (k’)

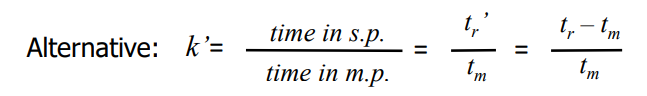
Retention Volume

volume of mobile phase needed to elute solute

V_r=t_r*F_mp=V_m + K*V_s

V_r’=V_r-V_mK*V_s

F_mp=flow rate

V_m=mobile phase volume

V_s=stationary phase volume

Exam problem 1:

answer:

Different types of chromatography

• Absorption chromatography

• partition chromatography

• ion exchange chromatography

• Size exclusion

• Affinity Chromatography

Absorption chromatograhpy

Solid stationary phase and a liquid or gas mobile phase

solutes absorb onto surface of stationary phase (GSC, LSC, TLC)

Partition Chromatography

Liquid/polymeric stationary phase and a gas/liquid mobile phase. Solutes partition onto stationary phase (GC, GLC, HPLC)

Ion exchange chromatography

Stationary phase is ion exchange resin; liquid (aqueous) mobile phase. Separation based on electrostatic (charge) interactions

Size exclusion chromatography

stationary phase is porous gel; liquid mobile phase. Separation is based on size so, larger molecules are excluded from pores of gel, travel faster through system.

SHAPE IS MORE IMPORTANT THAN SIZE/MW

Affinity Chromatography

used predominantly for separation/isolation of biochemicals/proteins. There is a liquid mobile phase. The stationary phase consists of specific proteins/compounds covalently linked to solid phase. (for example antigen/antibody; enzyme/substrate-cofactor)

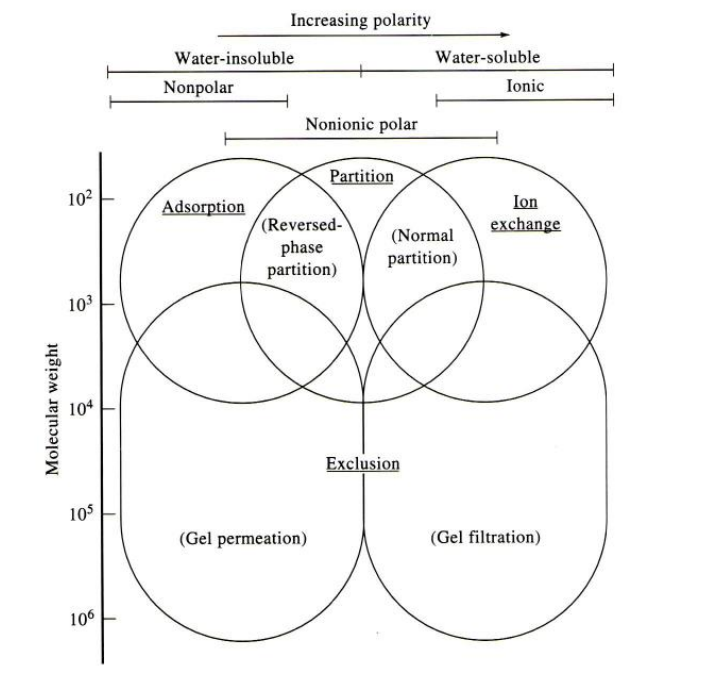
Chromatography selection chart

separation based on physical and/or chemical features such as size and polarity.

Chromatography workflow:

1. choose stationary phase (theoretical plates/resolution)

2. choose a mobile phase (theoretical plates/resolution)

3. decide how much to inject (capacity factor)

4. dial a flow rate (van deetmer equation)

5. run

6. evaluate results

7. adjust conditions

8. repeat

Gas Chromatography Increasing resolution

increase N by increasing the partitioning surface

Gas Chromatography (increasing selectivity)

increase deltaK by adjusting the chemistry

GC detectors

• Flame ionization detector

• thermal conductivity detector

• differential amplifier for TCD (twin sample and reference cells)

• Electron capture detector

Flame ionization detector (FID)

detects organic compounds by burning the effluent in a hydrogen air flame producing ions. the resulting current is electrodes is proportional to the number of carbon atoms. Highly sensitive to hydrocarbons but insensitive to water and permanent gases.

Thermal Conductivity detector

measures changes in thermal conductivity of the carrier gas by teh presence of analyte molecules. it is universal and non-destructive, but less sensitive than other detectors.

Differential amplifier for TCD

Enhances TCD sensitivity and stability by comparing the thermal conductivity of the column effluent (sample cell) to that of pure carrier gas (reference cell), using a differential amplifier to detect imbalances.

Electron Capture Detector

Detects electronegative compounds (like halogens) by measuring the decrease in current caused by analyte molecules capturing electrons from a radioactive beta-emitter. Very sensitive and selective to halogen compounds.

Atomic emission detector (AED)

uses a microwave induced plasma to excite atoms from eluted components. Provides both quantitative and qualitative information and can simultaneously detect multiple elements with high sensitivity

Supercritical fluid chromatography (SFC)

mobile phase is a supercritical fluid, substance with temperature and pressure above the critical point. Can have a solid or liquid stationary phase. Chromatographic characteristics intermediate between GC and LC. A supercritical fluid can dissolve samples soluble in liquids (not really volatile)

Reverse Phase Chromatography

Uses:

• hydrophobic stationary phases

• polar to non-polar gradients

As the gradient goes from polar to non-polar, hydrophobicity rules the order of elution

Size Exclusion chromatography (SEC)

V_e=V_o+K_avV_g

in order of variables, elution volume, void volume occupied by mobile phase, partition coefficient between gel and surrounding mobile phase, gel volume

V_t= total volume (void+gel)

Radius of gyration R_gyr=k(MW)^a

a=0.1 for rods, 0.5 for flexible coils, 0.3 for spheres

GC vs LC

GC, mobile phase: carrier gas; Stationary phase solid or liquid. key parameters: temperature and polarity. limited choice of carrier gases, adjust temperature and flow rate only. perform a temperature gradient.

LC, mobile phase: liquid; Stationary phase: solid or liquid. key parameters: polarity and reactivity. broader choice of separation solvents/systems. Adjust pressure, flow rate and temperature. Perform gradients of organic content, salt pH, etc…

Both (stationary phase): packed and capillary columns used to increase SA. the longer the column the greater the number of theoretical plates.

Spectroscopy

studies the interaction between electromagnetic radiation, energy, and matter

Wave Properties:

• characterized by v, lambda, nu, I = A sin (pi nu t + phi), E = h nu, P, etc

• additive nature, destructive/constructive

• diffraction when crossing a slit or pinhole

• refraction and reflection when crossing boundary between media

  • transmission absorption, scattering, polarization when crossing a specific medium

Particle properties

The photoelectric effect

Wavelength equation

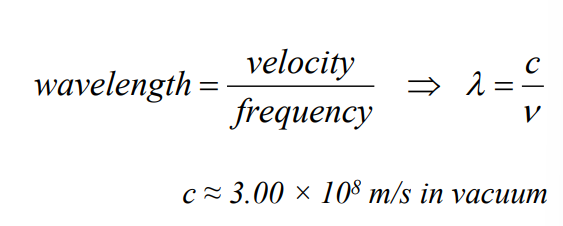
coherent radiation

the phases of two or more waves representing the radiation differ by a known constant that does not change over time (RF oscillators, microwave sources, optic lasers)

Incoherent radiation

the phase differences between two or more waves are unknown or random (tungsten bulb)

Radiation refraction

radiation passes at an angle through interface between two transparent media

Radiation reflection

when radiation crosses interface between media with different n. The reflected fraction increases with delta n. for a right angle beam:

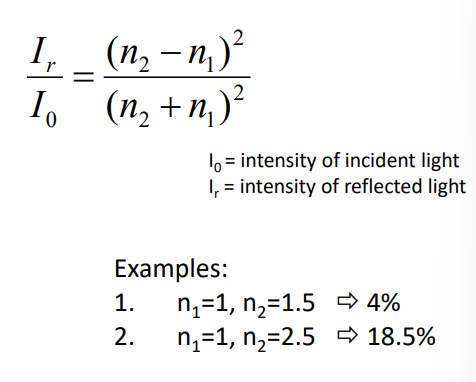
Radiation scattering

due to re-emission of light after interaction. Absolute destruction for small molecules, partial interference for larger ones.

Rayleigh Scattering

molecules «lambda —> which is the reason why the sky is blue due to greater scattering of short wavelengths

Large molecule scattering

used to determine size and shape of molecules

Raman Scattering

Used to determine size and shape of molecules

Radiation Polarization

removal of all but one of the possible vibrational planes

atomic absorption

atoms in gas phase

only electronic

Molecular Absorption

molecules

electronic, vibrational and rotational data

Non-radiative relaxation

energy loss in small steps, collisions

Radiative relaxation

fluorescence relaxation: t < 10^-5 s

phosphorescence relaxation: t > 10^-5 s

Emission

Emitted P_e

P_e=kc

atomic emission

Luminescence

P₁

P₁=kc

Atomic and molecular fluorescence, phosphorescence, and chemiluminescence

Scattering

P_sc=kc

Raman scattering, turbidimetry, and particle sizing

Absorption

Incident, P₀ and transmitted, P

-logP/P₀=kc

Absorption process

determines the part of the incident power that is transmitted (not absorbed as a function of frequency/wavelength

Emission happens in two steps

1. excitation: stimulus energy is applied in the form of light, heat, electrical energy, particles, chemical reaction

2. Emission: electromagnetic radiation is returned

Chemoluminescence

excitation by thermal, electrical or chemical stimulus (non-radiative)

Photoluminescence

excitation by radiation: fluorescence and phosphorescence

Fluorescence

prompt re-emission

Phosphorescence

delayed re-emission

Elastic Scattering

Inelastic scattering

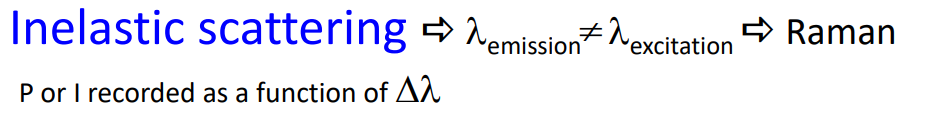
Emission Spectra

when excited electrons relax back, their excess energy is released as photons

Bands: electronic and vibrational levels (small molecules)

Lines: only electronic (atoms, ions)

Discrete lines

indicate an atom

unresolved lines

indicate a molecule

Continuum

is produced when solids are heated up: blackbody radiation

Blackbody Spectrum

BB radiation is emitted when a solid is heated to incandescence

• produced by thermal excitation and relaxation of many vibrational (and rotational) levels

• temperature dependent

• different materials exhibit different ranges

Can be used as a light source with very broad emission ranges

Blackbody absorption happens when

0% of radiation is transmitted or reflected and 100% is absorbed

Absorption quantitative aspect

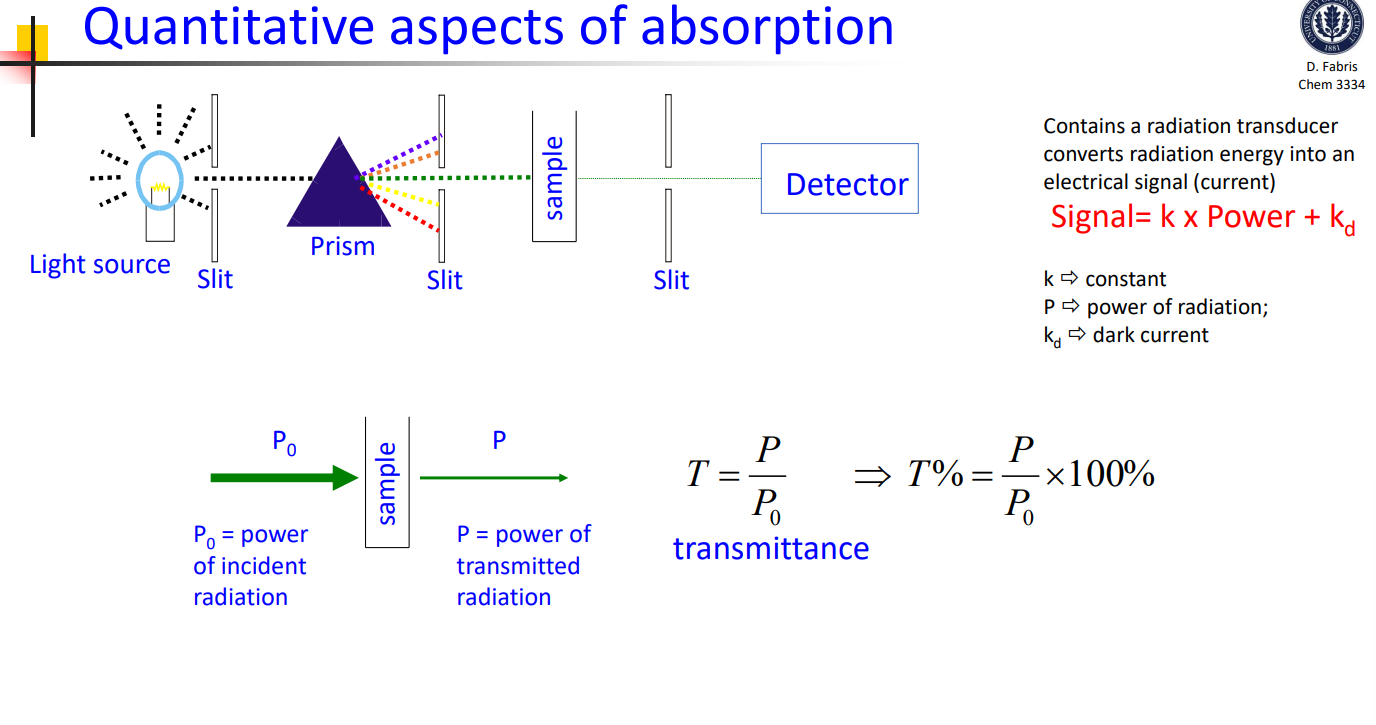
Transmittance

P/P₀

Absorbance

=logP₀/P=log1/T=-logT

No light absorbed

P=P₀

_T=1

_A=0

All light absorbed

P=0

T=0

A= infinity

90% of light absorbed

P=10%

T=10%/100%=0.1

A=-log(0.1)=1

Lambert’s law

T=P/P₀=10^-kb

Beer’s Law

T=P/P₀=10^-k’c

Beer-Lambert’s Law

-log T =log P₀/P=abc=A

B-L law in terms of transmittance

A=2-logT%

Scatchard plot for the det of equilibrium constants