Psych 2220 Exam 3

Effect Size

The extent of difference between means.
(e.g., observed mean & population mean)
• Alt: The extent to which participants' mean exceeded the value expected by chance (H0)

What factors influence power

Change alpha level
Increase sample size N.
Increase effect size (Cohen's d):
Switch to a one-tailed test (only if you're certain about the direction of difference!)

pooling variance for independent samples t test

ssquareda/N + ssquaredb/N

confidence interval formula one sample t test

Mupper= M+tcrit*Sm
Mlower=M-tcrit*Sm

Mlower<----M---->Mupper

confidence interval formula paired samples t test

Mupper=Md+tcrit*Sm
Mlower=Md-tcrit*Sm

Mlower<----M---->Mupper

effect size formula one sample t-test

dhat=M-mu/s

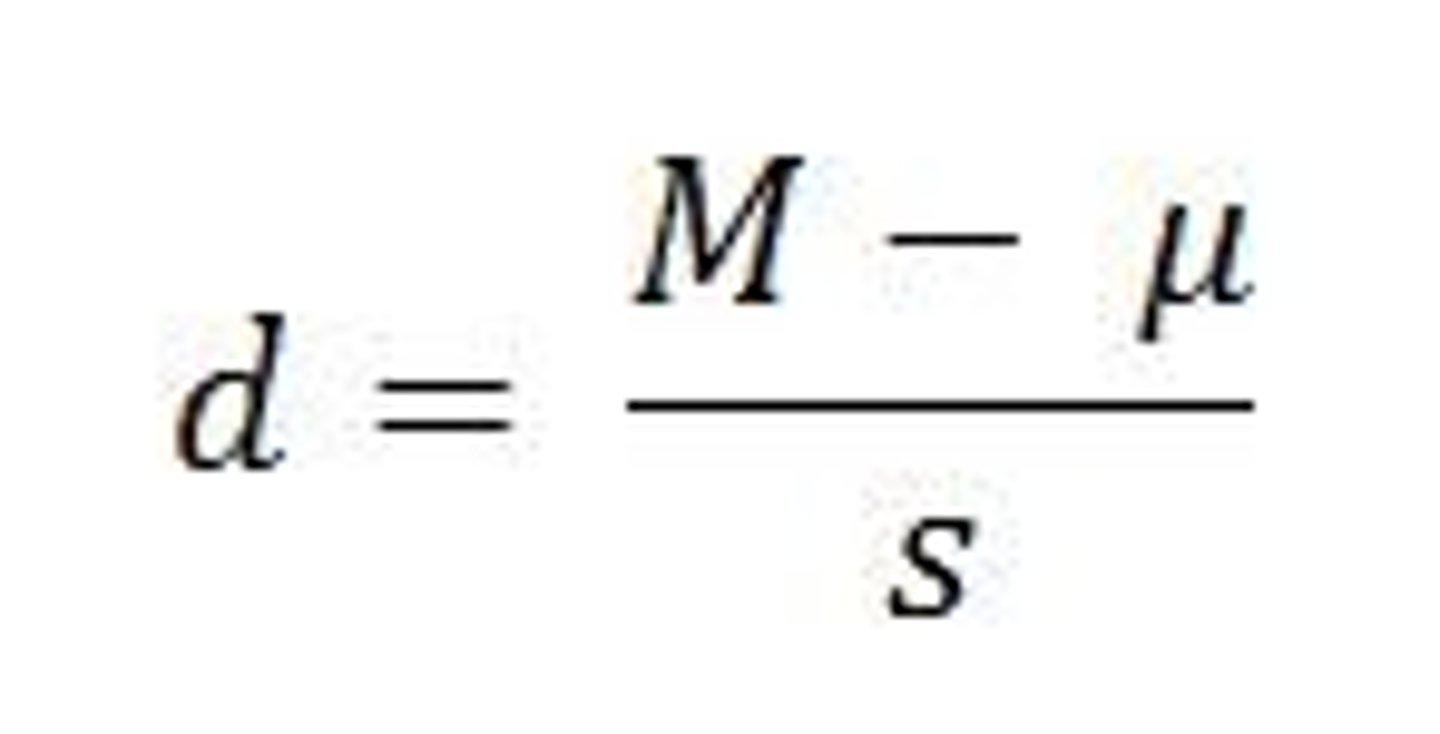
effect size formula paired samples t test

dhat=(M1-M2)-0/Sd

effect size formula independent samples t test

dhat=M1-M2/Spooled

Type 1 Error

false positive

Type 2 Error

false negative

independent samples t test

compares an observed sample mean (MA) to another observed sample mean (MB). If the samples are actually independent, then the scores vary independently of each other.
These are "unrelated" samples or non-paired samples.

paired samples t test

It represents a single observed mean
difference between two related observations, in terms of its distance from the center of the sampling distribution of the mean difference, as estimated by the observed sample standard deviation of differences sD

one sample t statistic

a test statistic that
expresses the distance between a sample mean M
and some suspected population mean μ, scaled to an
inferentially valuable indication of variability: standard
error of the mean (sM).

ANOVA

"ANalysis Of VAriance"

pooled variance

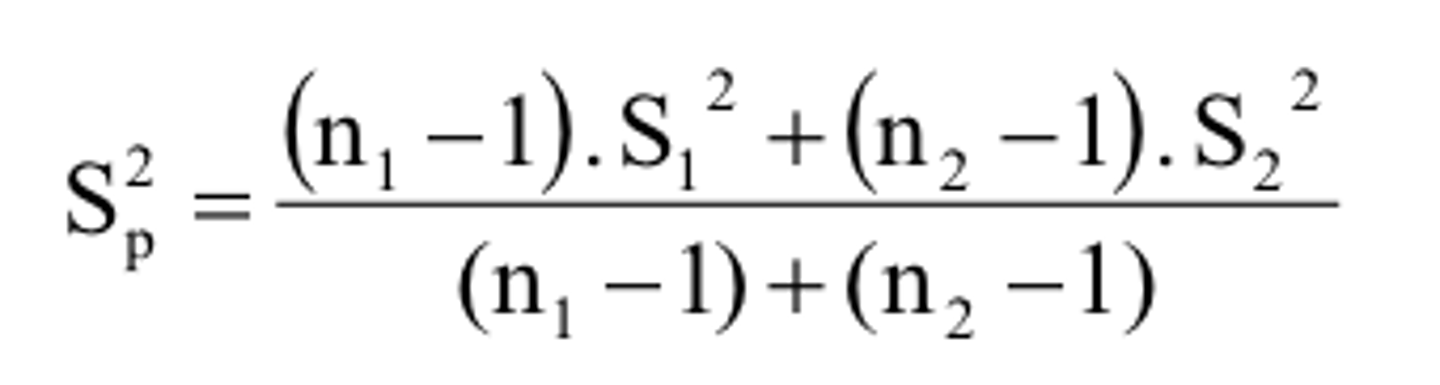
Formula for one sample t test

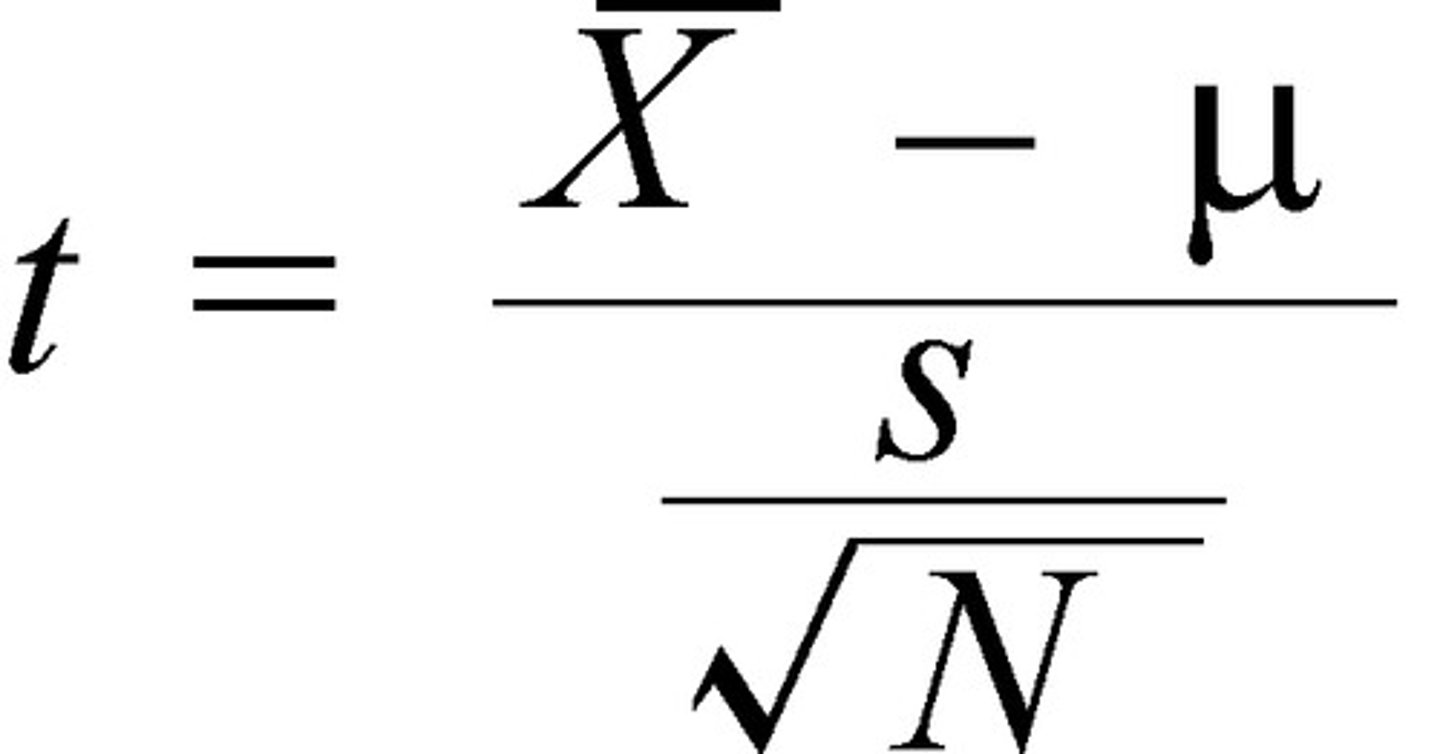
Formula for paired samples t test

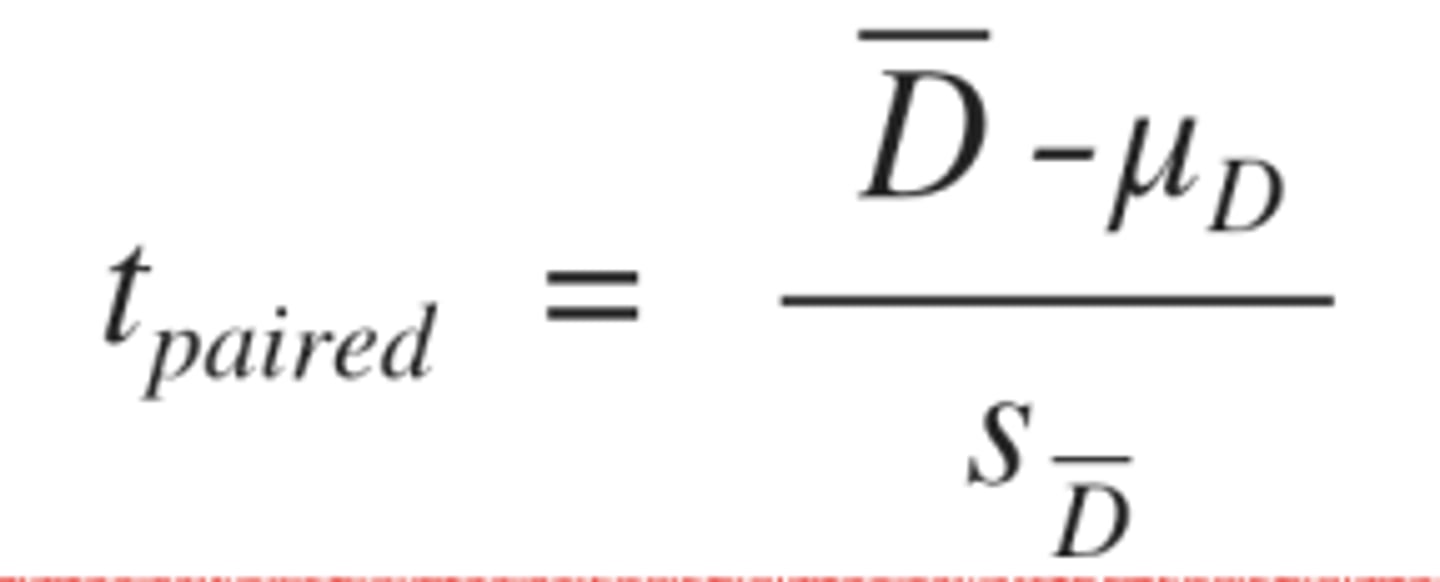
Formula for independent samples t test

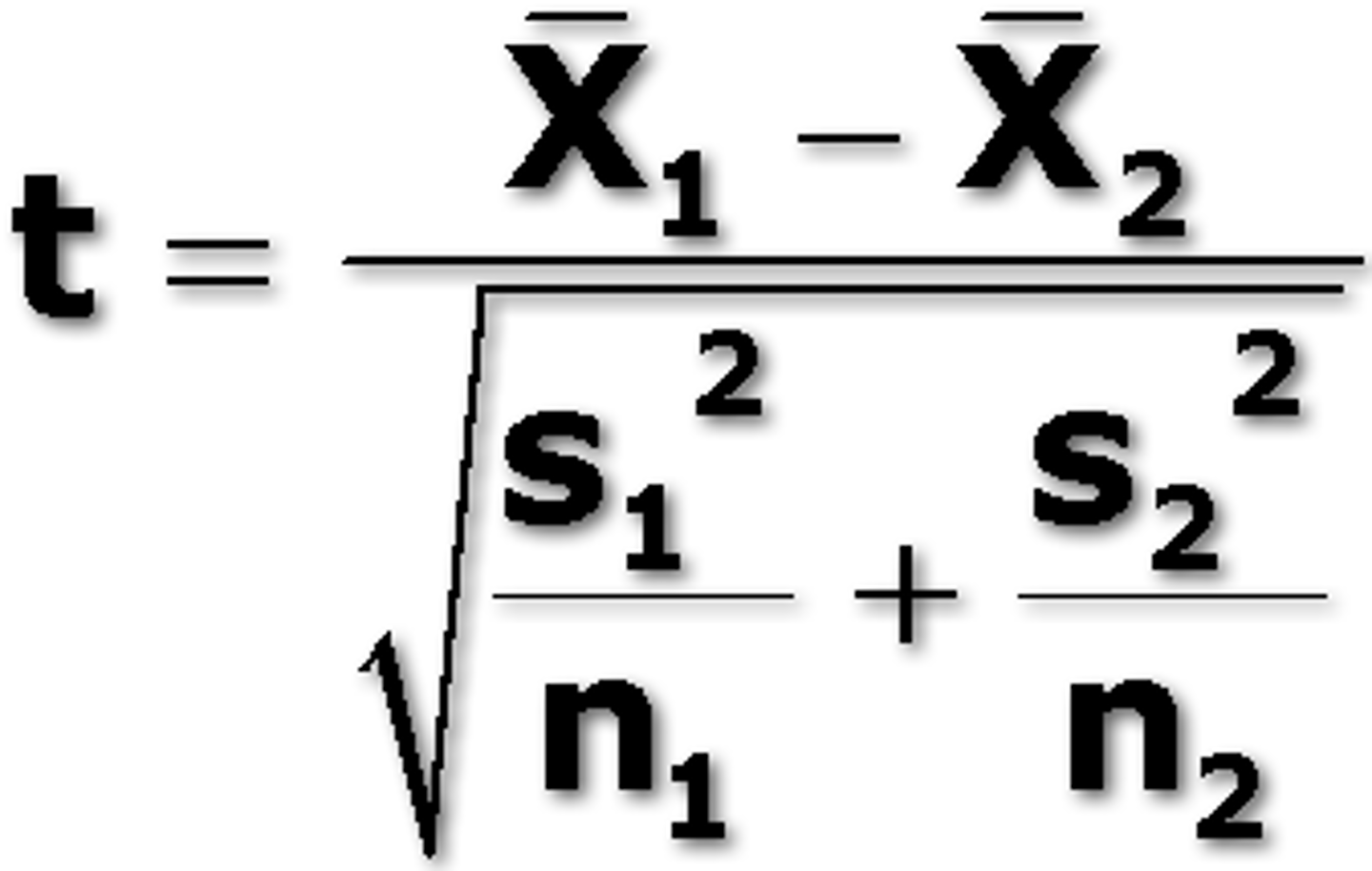
standard deviation, s,

We don't know σ, so we need to estimate it somehow.
We'll use the sample's __________ as our
best point estimate of σ.

one sample t test

The hypothesis test we perform to
determine whether we should reject a null hypothesis
about μ.
Follows the same approach as the z test

degrees of freedom for one sample t test

df: N - 1

degrees of freedom for independent samples t test

dftotal = (n1 - 1) + (n2 - 1) = n1 + n2 - 2

Hypothesis' examples for one sample t tests

Do psychology majors' GPAs differ from the OSU average?
• Are students favorable toward the new OSU president?
(Significantly more positive than a hypothesized population
average that reflects "neutral" or "no opinion")

one sample t test

It represents a single observed mean
in terms of its distance from the center of the sampling
distribution of the mean, as estimated by the observed
sample standard deviation s.

paired samples t test

a design in which two
observations covary (i.e., are related to each other),
and the difference between observations is the focal
dependent measure

examples of paired samples t tests

Most common: Same people, measured twice (two conditions or
different times)
• Less common but equally valid: Different people bound by a clear one-to-one relationship (spouses, siblings, interaction partners, etc.), or even unrelated people matched on some extra variable that correlates with the DV.
• Measure depression, then give CBT, then measure depression.
• Measure parent's intent to quit smoking BEFORE and AFTER an educational intervention presenting their child's biochemical SHS exposure data to the parent.

order effects

Taking one observation can affect later
observations recorded from same individuals.

example of independent samples t test

Suppose you're interested in testing a claim that psychology majors perform logic problems better than chemistry majors.

independent samples

(two) sets of observations that
cannot be assumed to share variance on an individual
level. That is, the observations are unrelated across
groups; a test of mean
differences between two unrelated groups of scores.

one sample t test

Compare a sample's mean score to a population

paired samples t test

Compare a mean difference to the population of differences.

independent samples t test

Compare a difference between two sample means to the population of differences between means.

F statistics

compare the observed variance based on a set of means to the expected variance of all the individually sampled observations.

F statistic Formula

F=Ssquared observed/Ssquared expected

standard error

s/squareroot(N)