Final Exam Study Chapters 1

This assigns to each element of one set (domain) to one element of a second set (called the range)

What is a function?

In a function, these are all the x values

The domain of the function.

In a function, these are all the y values

The range of the function.

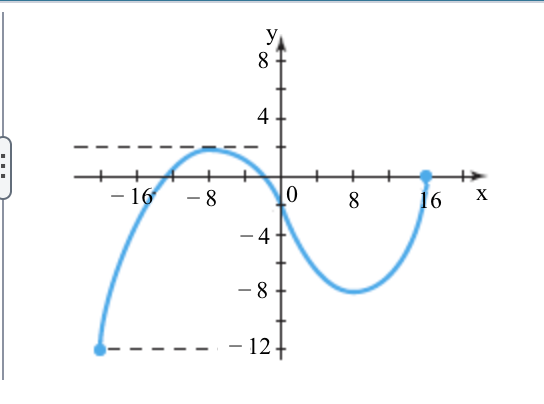
Domain of function?

[-20,16]: Left → Right

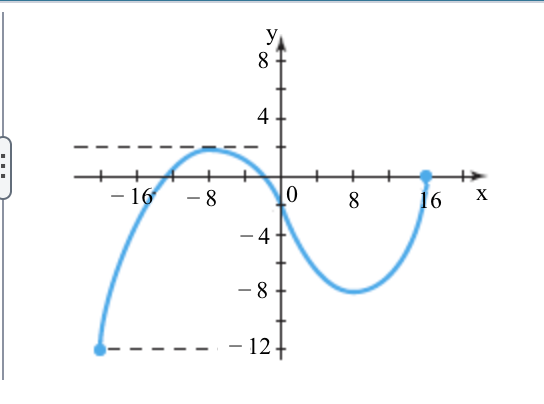
Range of function?

[-12,2]: Bottom → Up

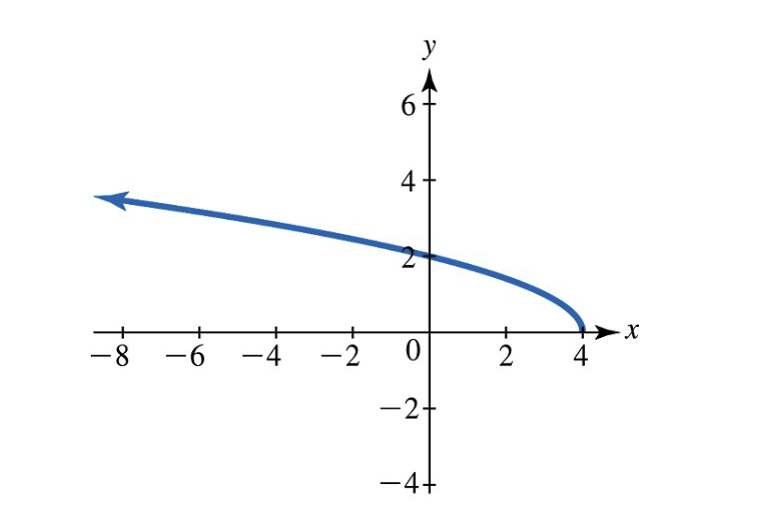
Domain of function?

(- infinity, 4]

Left→Right

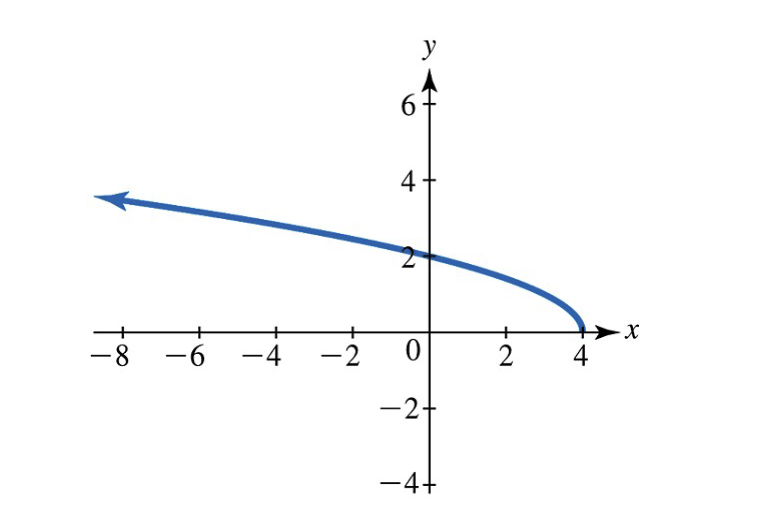
Range of function?

[0, infinity)

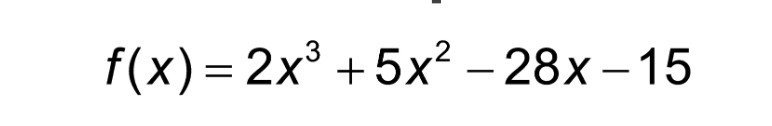
find f(-2)

2(-2)³+5(-2)²-28(-2)-15 = 45

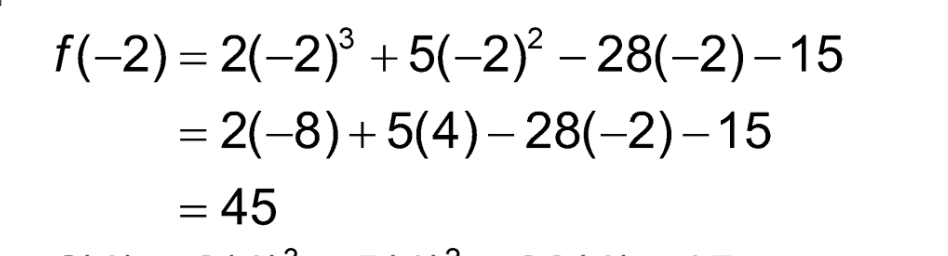
find f(4)

• Plugging 4 in for x

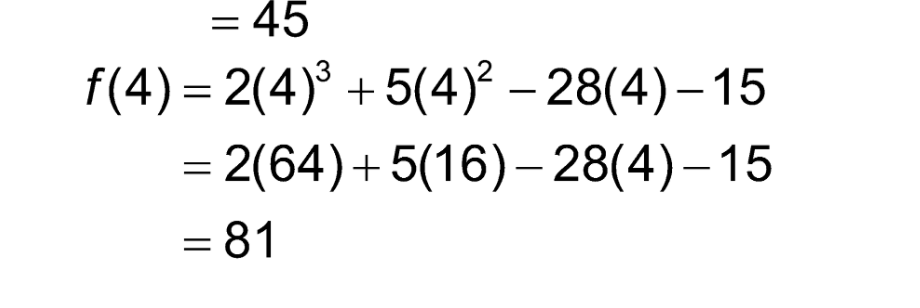
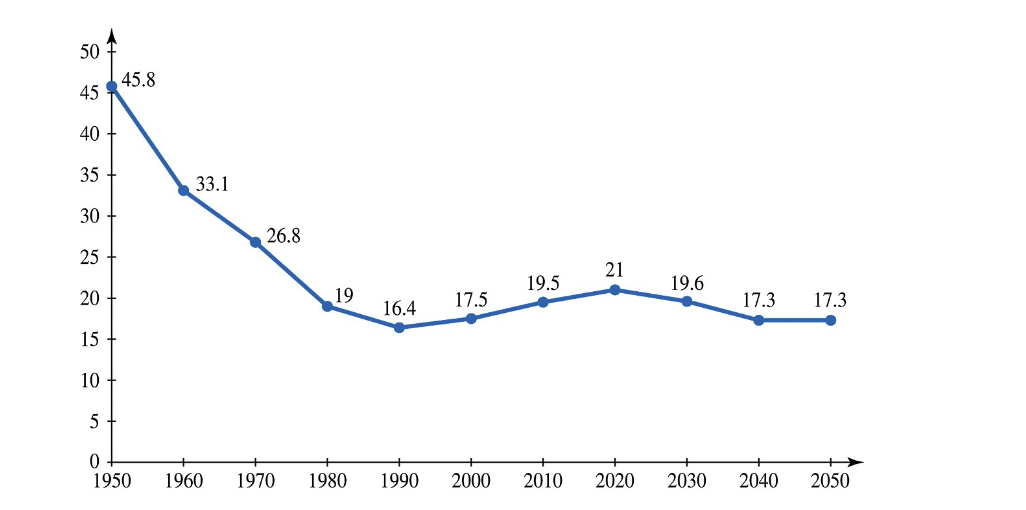
Find and interpret g(2020)

g(2020)=21

The function V(t)= 3.77t² +24.4t+105.6, where t equals the number of years after 2010

• What value of t represents 2025?

The value of t representing 2025 is 15, as 2025 is 15 years after 2010. 2010+15= 2025.

The function V(t)= 3.77t² +24.4t+105.6, where t equals the number of years after 2010

• What is the value of the projected medical 3D printing market in 2025?

The projected medical 3D printing market in 2025 is represented by V(15) using the function.

• 3.77(15)²+24.4(15)+105.6= $1320

The function V(t)= 3.77t² +24.4t+105.6, where t equals the number of years after 2010

• Find V(17)

represents the projected medical 3D printing market in 2027, calculated as V(17) = 3.77(17)² + 24.4(17) + 105.6.= 1610.

What makes a complete graph?

Shows the basic shape of the graph (points where it crosses the axes and where graph turns)

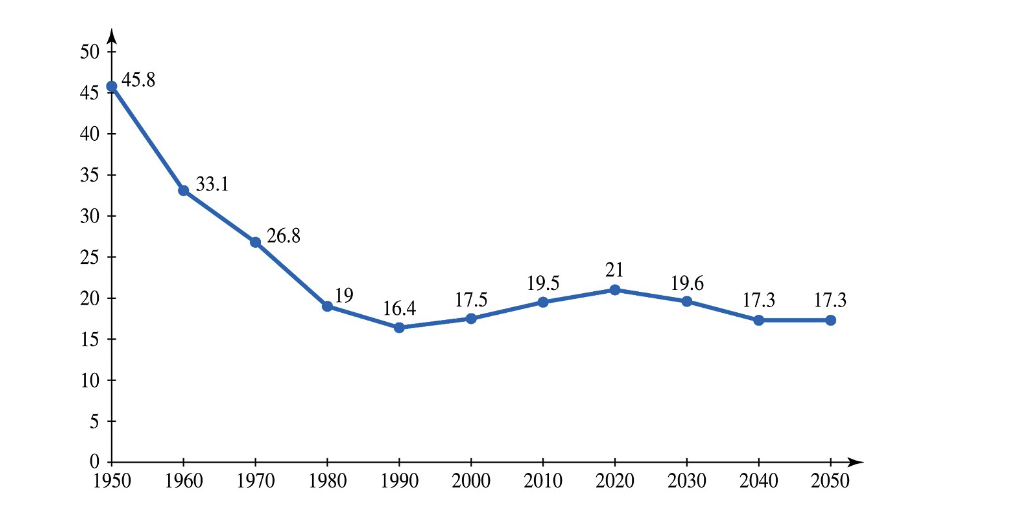
What is the input t if the output is g(t)=19.6 million?

t=2030

This is how individuals test if a graph is a function

What is the vertical line test? If a vertical line intersects the graph at more than one point, it is not a function.

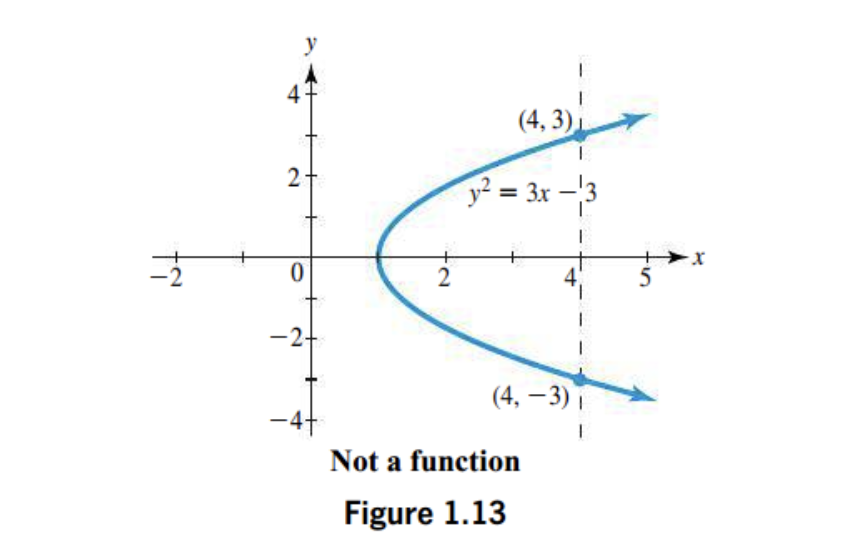
Is this a function?

No, fails the vertical line test

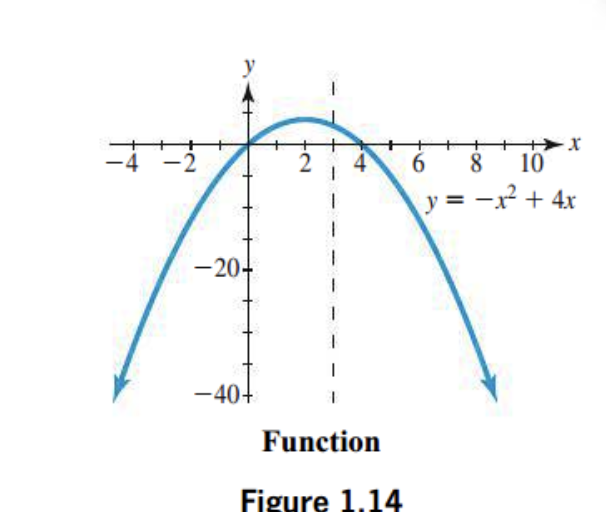
Is this a function?

Yes, passes the vertical line test

Find the domain of: x+2/x-3

Set the denominator equal to zero

• x-3=0

• x=3

• Domain: All real numbers expect 3

This is a function in the form (f)x “y”= ax+b

What is a linear function?

This is how you find the x-intercept

Set y to zero

This is how you find the y-intercept

Set x to zero

2x-3y=6, find x-intercept.

3y=0

• 2x=6

• x=3

• (3,0)

2x-3y=6, find the y-intercept.

2x=0

• -3y=6

• y=-2

• (0,-2)

This is how you find the slope of a line

What is rise/run?

This is how you find the slope between two points

y2-y1/x2-x1

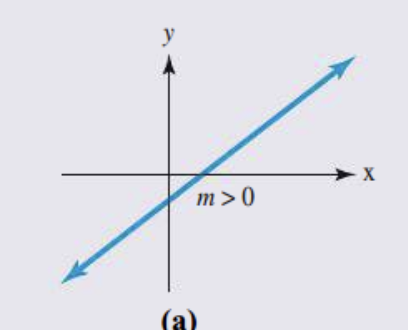
What is this slope?

Rising from left-right: Slope is Positive

What is this slope?

Falling from left-right: Slope is Negative

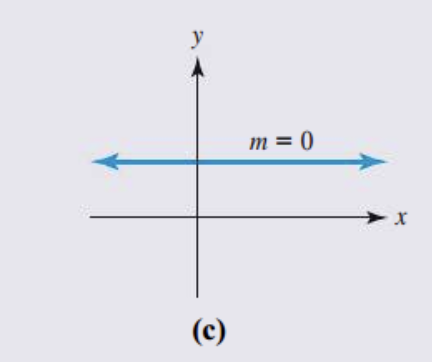
What is this slope?

No change between left-right: Slope is Zero.

What is this slope?

Undefined: Slope is Undefined

This is the equation for the slope of a line

What is y=mx+b

• mx: Slope

• b: Y-intercept

Slope and y-intercept of: y=7x-12

• Slope: 7

• Y-intercept: -12

This is this equation of a line with a slope “m” the passes a known point (x1,y1)

What is the Pointe-Slope form

• y - y1 = m(x - x1).

The is the form of a vertical line

What is x = a ?

This is the form of a horizontal line

What is y = b ?

Equation for Average Rate of Change

f(b)-f(a)/b-a

• You want to square f(b) and f(a)

Find the average rate change for x=-7 and 7

7²-(-7)²/7+7= 49 - 49 / 14 = 0

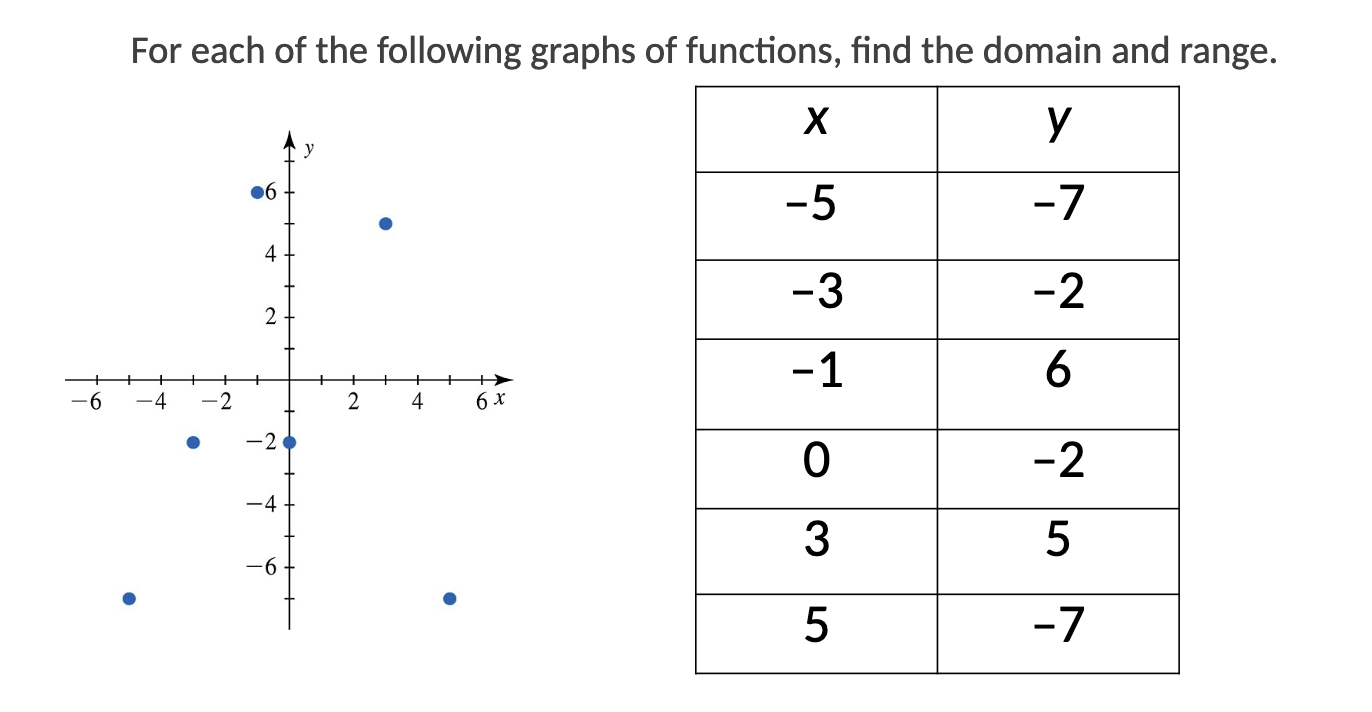
What is the Domain?

{-5,-3,-1,0,3,5}

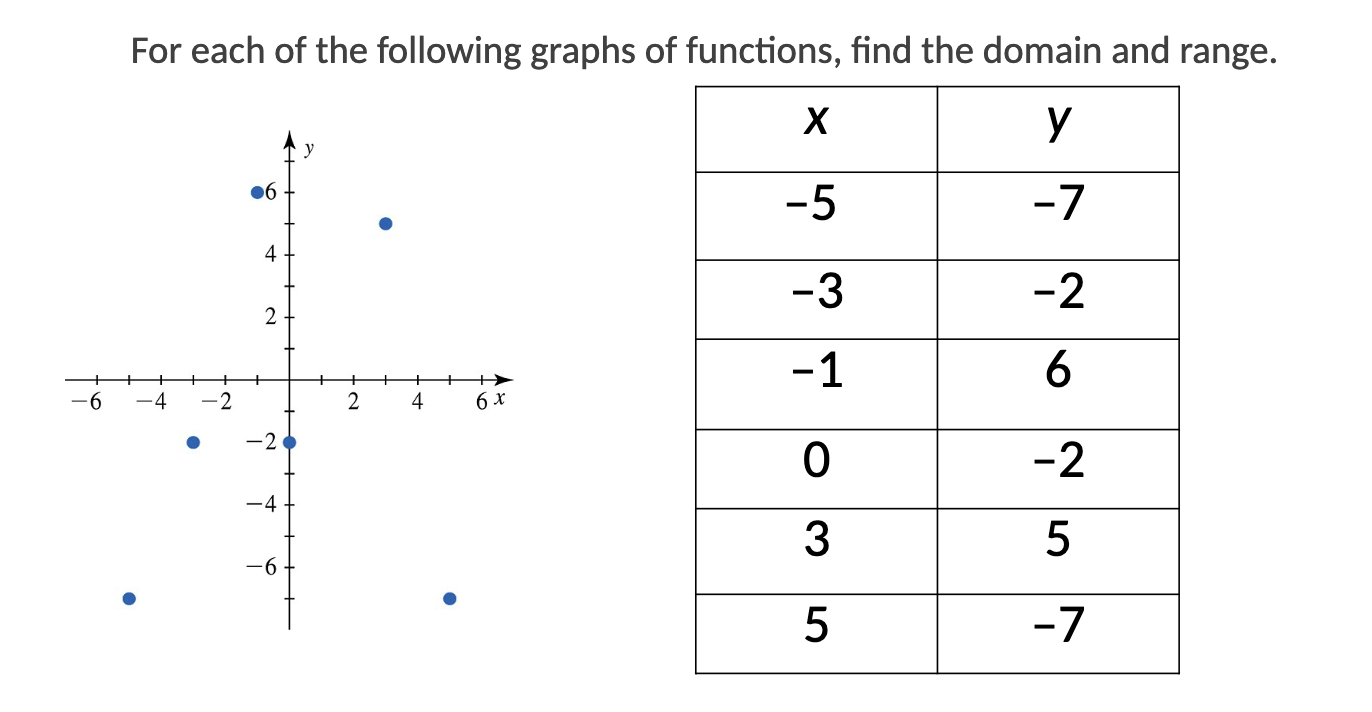
What is the Range?

{-7,-2,6,5}

Find the slope of the line passing through the points (-3,2) and (5,-4)

The slope is calculated as (y2 - y1) / (x2 - x1), so it equals (-4 - 2) / (5 - (-3)) = -6 / 8 = -3/4.

Write an equation for the line that passes through the point (-1,5) and has the slope ¾

• y-5=3/4(x-(-1)

• y-5=3/4x+3/4

• y=3/4x+23/4

Write an equation of the line, passing through (-1,5) and (2,4)

1) find the slope:

(y2 - y1) / (x2 - x1) = (4 - 5) / (2 - (-1)) = -1 / 3

2)Using point-slope form, the equation is y - 5 = -1/3(x + 1).

If lines are parallel

The slopes are the same

Write the equation of the line through (4,5) and parallel to the line: 3x+2y=-1

1) find the slope, solve for y

• 3x+2y=1

• 2y=-3x-1

• divide by 2 on both sides

• y=-3/2x-1/2

2) Plug into point slope

• y-5=-3/2(x-4)

Write the equation of the line through (4,5) and perpendicular to the line: 3x+2y=-1

1) Find the slope

• 2y=-3x-1

• Divide by 2: y=-3/2x-1/2

The perpendicular line has the opposite, reciprocal slope: 2/3x2) Use point-slope form: y - 5 = 2/3(x - 4)

For parallel line: slopes are

the same

For perpendicular lines: slopes are

opposite reciprocals

This is the equation for a linear with one variable

ax+b=0

The is the equation for a linear with two variables

ax+by=c

Solve for x: 2x-3/4=x/3 +1

To isolate x, first eliminate the fractions by multiplying through by 12, then simplify to find the value of x.

!make sure you find the LCD!

This is any number “a” for which is also called the x-intercept and solution

What are the zeros of a function or equation.