AP STATS AP TEST REVIEW 1, 2, 3 +& 4

Stratified Sample

Separates sample by homogenous (same) groups called strata. Like race, gender, income or age.

Cluster Sampling

splits population into similar parts or clusters, then select a few random clusters, then take a census of each.

Multistage Sampling

multiple types of samples combined

Voluntary Response Bias

-volunteers who wanted to participate in the survey.

Systematic sample

Start from a randomly selected person. Then start surveying/collecting data from every n^th person

Under coverage

Subgroups are completely left out or underrepresented.

Non response Bias

option to respond but choose not to

Response Bias

Question is worded or phrased in such a way that influences the response.

Convenience Sampling

sample consists of participants that are nearest and easiest to sample.

population

the entire group of indivi­duals we want inform­ation about

census

a complete count of the popula­tion; when you gather general inform­ation about the entire population

sample

a part of the population we actually examine in order to gather inform­ation

sampling design

the method used to choose the sample from the population

sampling frame

is a list or source that contains all the units (individuals, households, etc.) from which a sample will be selected. 

simple random sample

is a sampling method in which every member of the population has an equal chance of being selected.

stratified random sample

is a sampling method that divides the population into distinct subgroups (strata) based on shared characteristics and then randomly selects samples from each stratum, ensuring representation across the different subgroups.

systematic random sample

randomly select a number between one and n and survey every nth person after that.

cluster random sample

The population is divided into naturally occurring groups (clusters) that are often geographically based (e.g., schools, neighborhoods, hospitals). and then randomly selects entire clusters to sample, often used for convenience or cost-effectiveness.

multistage sample

is a sampling method that combines different sampling techniques, such as stratified and cluster sampling, at various stages to obtain a more representative sample from a larger population.

describe an srs

put the names/­numbers of all ___ on slips of paper and place in a hat. Mix and randomly draw ___ slips of paper without replac­ement. Survey the corres­ponding people.

describe a systematic random sample.

number all ___ and place ___ numbers in a hat. Mix and randomly select one number and survey the corres­ponding person. Survey every nth person on the list after that.

describe a cluster random design

number all clusters and put the numbers into a hat Mix and randomly select a number from the hat. Survey everyone in that cluster.

stratified random sample

sort everyone into strata then number ___ in all the stratum. place the numbers in a hat and draw __ numbers. survey the corres­ponding people in that specific stratum. repeat the process for all strata

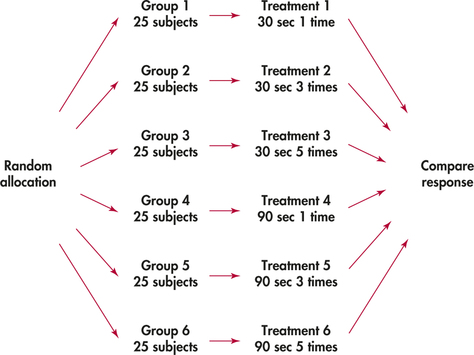
Completely randomized design

experi­mental units are assigned completely at random to treatments.

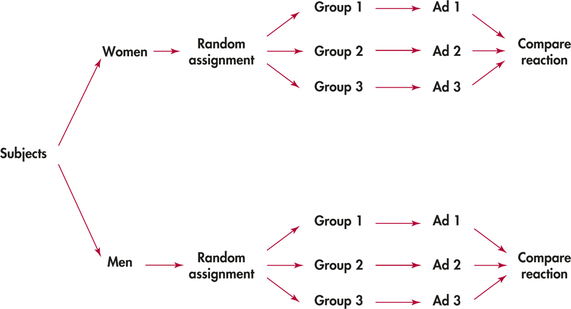
randomized block design

experi­mental units are blocked in homoge­neous groups and then randomly assigned to treatmentswithin each block to control for variability.

matched pairs design

a special type of block design; match up experi­mental unit according to similar charac­ter­istics and randomly assign one to treatment A and the other get treatment B randomly.

observational study

a study where researchers observe subjects without manipulating anything; they collect data on variables of interest.

Experiment

actively impose a randomly assigned treatment in order to observe the response

experimental unit

the single individual to which the different treatments are randomly assigned

factor/ explanatory variable

what we test or what we change

Level

the specific values or settings of a factor in an experiment.

response variable

what you measure or record at the end of the experiment

treatment

a specific experi­mental condition applied to the units

Control group

a group that is used to compare the factor against; can be placebo

placebo

a "­dum­my" treatment that can have no physical effect; not required in every experiment

blinding

method used so that units or evaluators do not know which treatment units are getting

double blinding

neither the units nor the evaluators know which treatment a subject recieved.

confounding variable

a third variable that potent­ially affects both the factor and the response variable.

Randomized block design

completely randomized design

Simpsons Paradox

-A conjecture can be reversed when several different groups of data are combined to form a single group. The effect of another variable is masked when the groups are combined.

Correlation coefficient

-a quantitative value of the strength of a linear relationship.

“r”

-ranges from -1 to +1

-no unit of measurement

-strongly affected by outliers.

Coefficient of Determination

-r²

gives the proportion of variation in y (response) that is explained by the relationship of (x, y) or x, the explanatory variable.

-between 0—>1 or 0-100%

-if r²=100%, then all the variation in the response variable is explained by the variation in x or the explanatory variable.

Skewed left

Mean<median

Skewed right

Mean>median

Fences + Outliers

Less than Q1-1.5(IQR)

Greater than Q3+1.5(IQR)

Conditional Relative Frequency

-The relative frequency for a specific part of the table. For example, the cell frequencies in a row divided by the total for that row or the cell frequencies in a column divided by the total for that column.

frequency=

counts

relative frequency=

%

mean of a sample

x̄

mean of population

μ

5 number summary helps

understand variability

Variability

- describes how scattered or clustered data points are within a dataset. 

-Range, Interquartile Range (IQR), standard deviation, and variance are all measures of

-A dataset with high variability has data points widely scattered, while a dataset with low variability has data points clustered tightly together. 

Quantitative Variable

-takes on numerical values for a measured or counted quantity

Discrete Variable

-takes on a finite of countable number of values

Continuous Variables

-takes on uncountable or infinite values with no gaps such as heights and weights of students.

Sensitive to extreme values?

-mean, range, variance, and st dev

not sensitive to extreme values?

median and IQR

Variance

• quantifies the average squared distance of each data point from the mean (average) of the dataset. 

• Why it's important:

  A higher variance indicates a greater spread in the data, while a lower variance suggests data points are clustered closer to the mean.

Dotplot

advantages-shows every value and it is easy to see the shape of the distribution

disadvantages-hard to plot large data sets.

(4.2) Law of Large Numbers?

Simulated probabilities seem to get closer to the true probability as the number of trials increases.

(4.3) The probability of an event is between what two numbers?

The probability of any event is a number between 0 and 1, inclusive.

(4.3) Probability of an Event Formula?

(4.3) Probability of the Complement of an Event Formula?

E’ (aka E^C or not E) = 1 - P(E)

(4.4) What is a mutually exclusive event?

Two events are mutually exclusive (disjoint) if they cannot occur at the same time. If two events are mutually exclusive, then the probability of their intersection is 0; in other words if two events are mutually exclusive, P(A and B) = 0.

(4.4) What is Joint Probability?

The joint probability is the probability of the intersection of two events; P(A and B).

(4.5) What is conditional probability?

Conditional probability is the probability that an event happens given that another event is known to have already happened.

(4.5) General multiplication rule for two events A and B?

Note that it can be manipulated to give the conditional probability formula as well; P(B | A) = P (A ⋂ B) / P (A).

(4.6) What are the conditions for two events to be independent?

Two events A and B are independent iff

• P(A | B) = P(A) and P(B | A) = P(B)

• P(A and B) = P(A) ⋅ P(B)

(4.6) How do you calculate the probability of the union of two events?

The probability of the union of two events can be found by the formula:

• P(A or B) = P(A) + P(B) - P(A and B)

Using proper notation it is:

• P(A ⋃ B) = P(A) + P(B) - P(A ⋂ B)

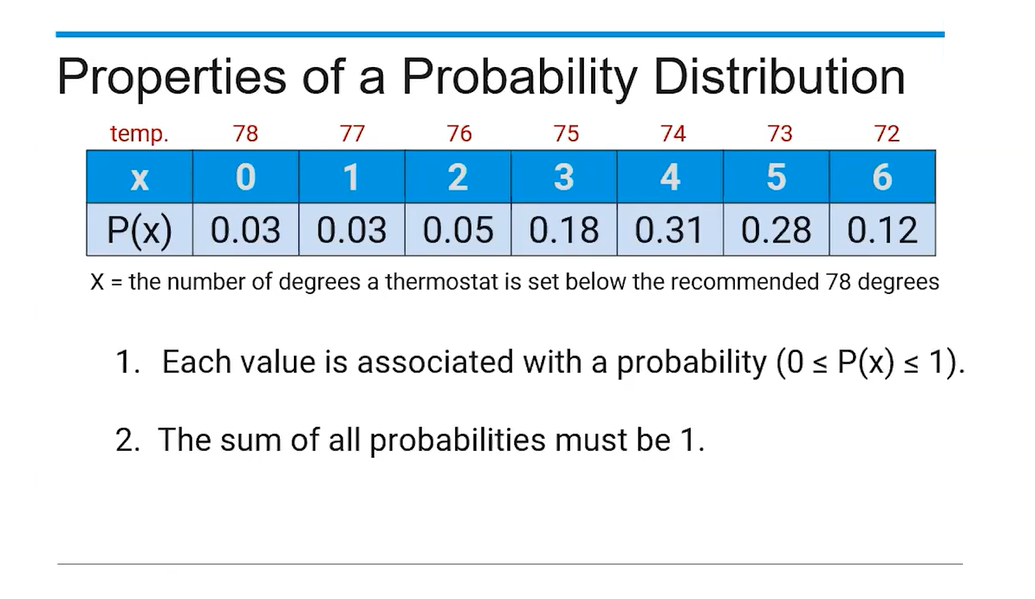
(4.7) Properties of a probability distribution?

(4.8) Mean (Expected value formula)?

(4.8) Standard Deviation Formula (σ_x)?

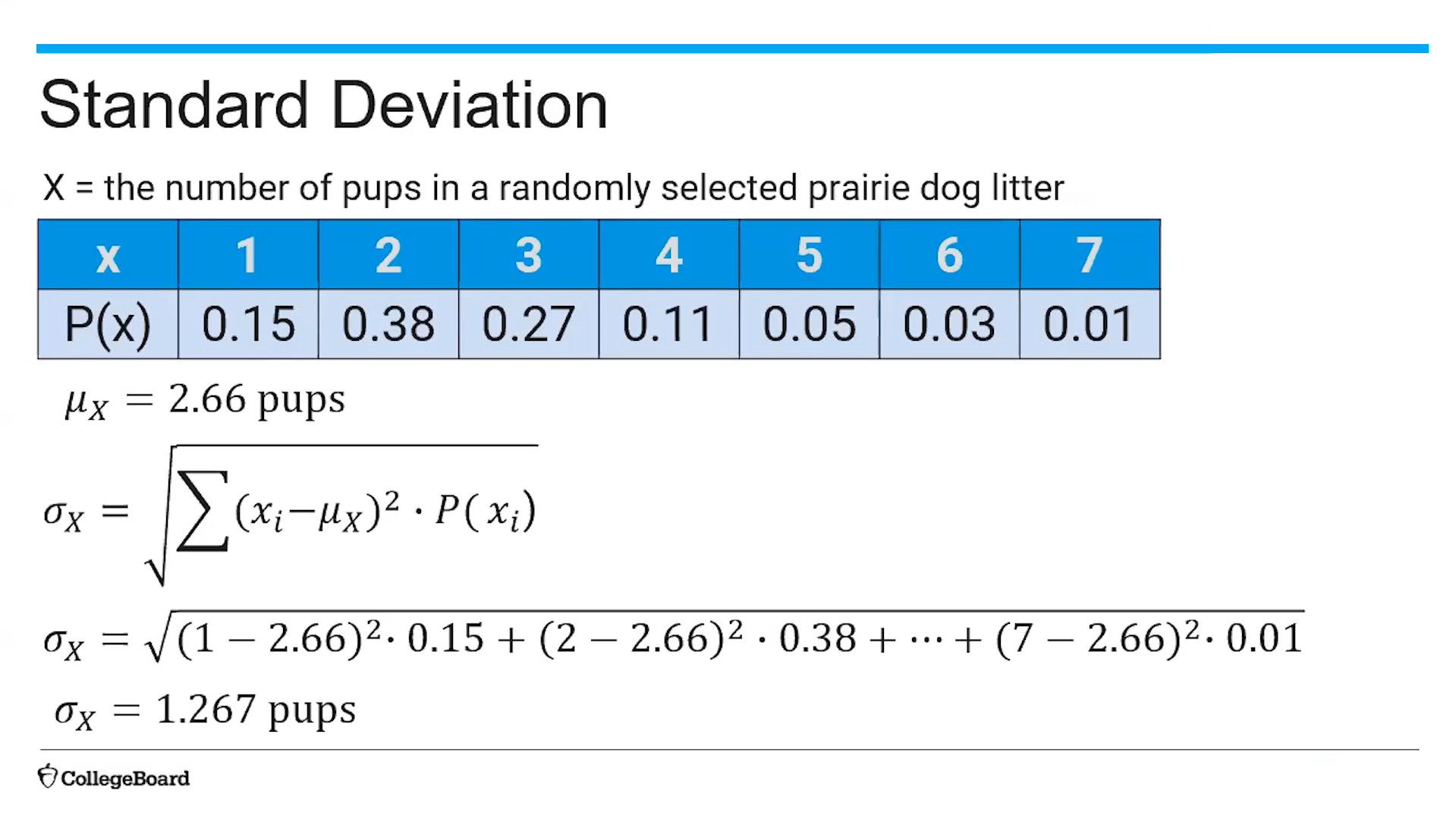
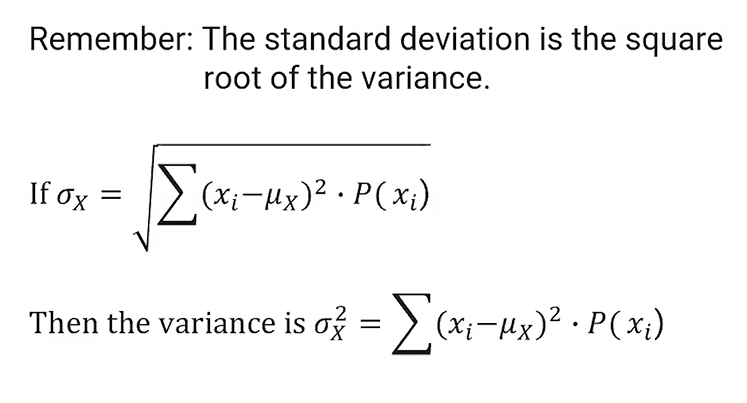
(4.8) Variance Formula (σ_x²)?

(4.9) Finding the mean and standard deviation using a linear transformation

(4.9) When are two random variables independent?

Two random variables are independent if knowing information about one of them does not change the probability distribution of the other.

(4.9) How do you calculate the mean and standard deviation of independent random variables X and Y and real numbers a and b?

(4.10) Definition and conditions for a binomial setting?

A binomial setting involves repeated trials of the same random process, where these conditions are met:

• Two possible outcomes: success or failure

• Independent trials

• Fixed number of trials

• Each trial has the same probability of success

(4.10) Binomial Coefficient Formula

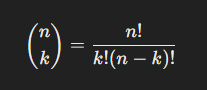
(4.10) Binomial Probability Function

(4.10) Note about calculating binomial probabilities

When calculating binomial probabilities, be sure to define the random variable, identify the distribution and values of interest, calculate the correct probability and answer the question in context

(4.11) Mean of a Binomial Function Formula?

μ_X=np

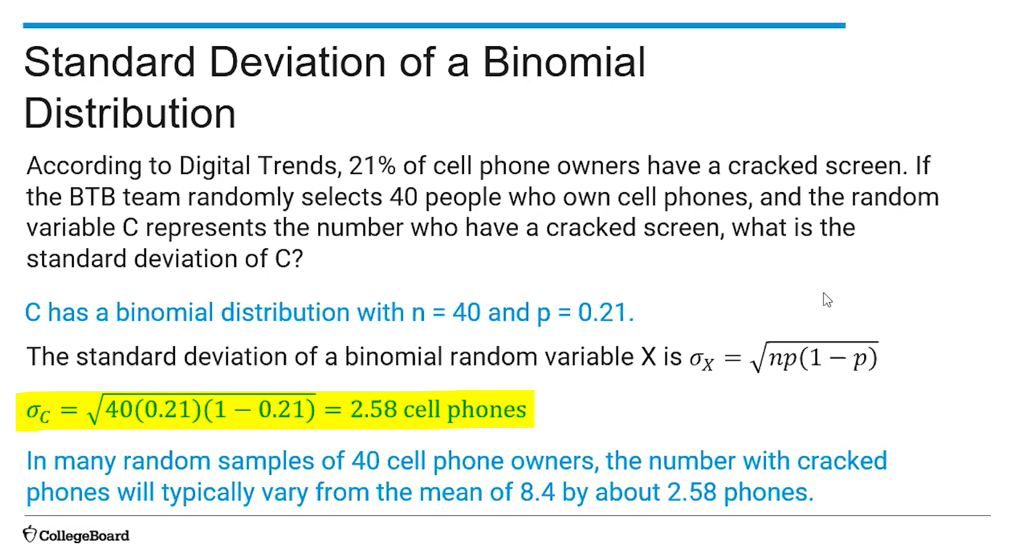
(4.11) Standard Deviation of Binomial Function Formula?

σ_x=√np(1-np)

(4.12) Geometric Setting vs Binomial Setting

A geometric setting involves the same elements as a binomial setting:

• Two possible outcomes: success or failure

• Independent trials

• Each trial has the same probability of success

The only difference is this:

• No fixed number of trials (Trials will be continued until there is success)

(4.12) Geometric Random Variable Probability Formula?

P(X=x) = (1-p)^x-1p

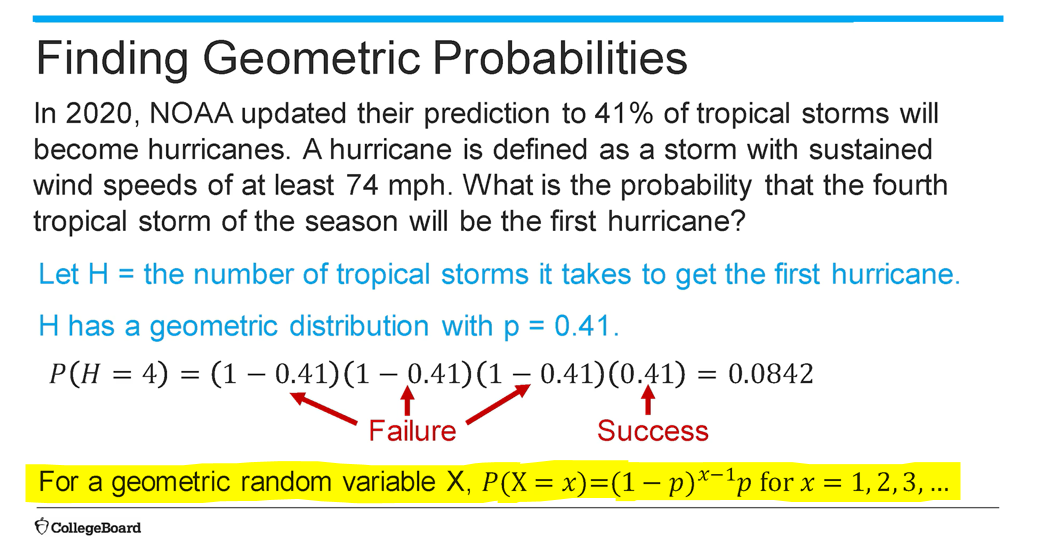
(4.12) Mean of a Geometric Random Variable Formula?

μ_X=1/p

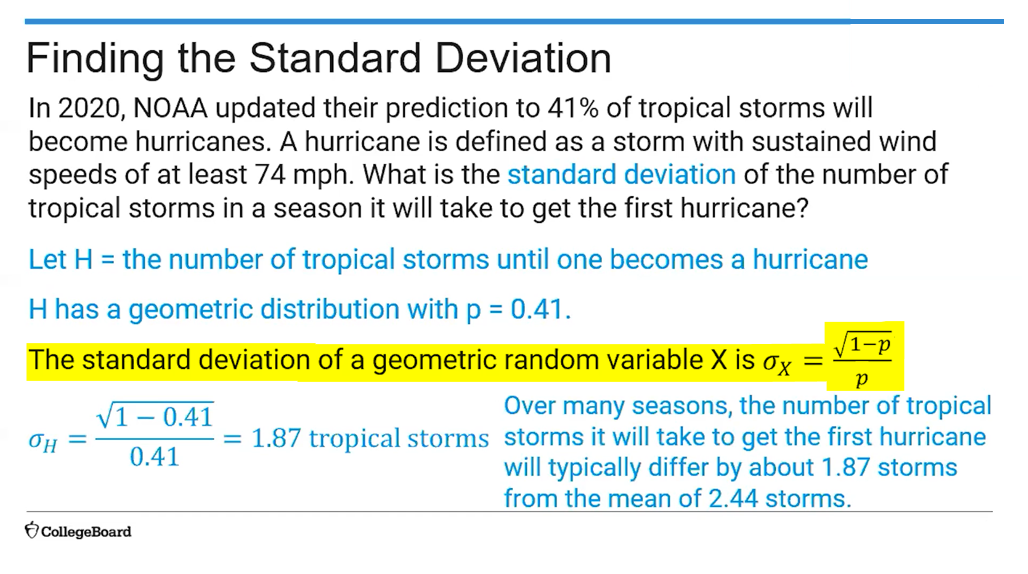
(4.12) Standard Deviation of a Geometric Random Variable Formula?